Complete Solutions to EXERCISE of chapter MCQ of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MCQ |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
A-B =`phi` iff
View SolutionIf n(A)= 3, n (B) = 4 then` (A xx A xx B)` =
View SolutionTotal number of relations that can be defined on set X={p,q,r}is
View SolutionIf P = {-2,-1, 0, 1, 2}, Q = {-6,-5,-3, 0, 3} and the mapping f :`PrarrQ` is defined by `f(x)= 2x^2 + x -6`, state which of the following is the image of (-2)?
View SolutionIf f(x)=`log_3x` and `phi(x)=x^2`,then the value of `f{varphi(3)}`will be
View SolutionFind the minimum value of `Cos^2theta+sec^2theta`
View SolutionIf `tanalpha+secalpha=e^x`,then `cosalpha` equals
View SolutionIf tan A=3/4 and tan B=4/3 ,the value of (A+b) is
View Solution`2sin40^@sin10^@`=
View SolutionShow that `1/(sin10^@)-sqrt3/(cos10^@)=4`
View Solution`sin12^@.sin48^@`=
View SolutionThe general solution of the equation `sinalpha=1`is
View SolutionIf in `/_\ABC`,`angleA=45^@`,`angleB=60^@` ,then the ratio of its sides is
View SolutionThe sum of the cubes of 1st n natural numbers is
View SolutionState which of the following is the value of `(1+i+2i^2+i^3+i^4)`[given `i=sqrt-1]`
View SolutionIf the roots of the equation ` 5x^2 - 7x - k = 0 `are reciprocal to one another, then which of the following is the value of k?
View SolutionSolution sets of the inequation `-2le(3x-1)/2le1[x in Z]`is
View SolutionGiven `^9P_3=`x `^9P_5` then the value of x is
View SolutionIf ``^25C_r=`^25C_(2r+1)`,the value of ``^rC_5`is
View SolutionThe. coefficient of 10th term in the expansion of `(x+1/x)^20`
View SolutionThe 12th term of the scries-n,(n -1), (n - 2),….
View SolutionIf 5.x, 405 are in G.P, then which of the following is the value of x?
View SolutionThe perpendicular distance of the straight line 3x + 4y + 15 = 0 from the origin, is
View SolutionThe angle between the-lines `x+sqrt3y+7=0`and `sqrt3x-y+8=0`is
View SolutionThe distance between the two-parallel lines 3x + 4y + 9 =0and 3x+4y+7=0 is
View SolutionThe equation `x^2+y^2+2gx+2fy+c=0` represents a point -circle when
View SolutionThe-equation of the directrix of the parabola `2x^2=3y` is
View SolutionIf the equation `x^2/(4-m)+y^2/(m-7)+1=0`represents an ellipse then
View SolutionThe length of the latus rectum of the hyperbola `9x^2-25y^2=225`is
View SolutionThe points (5, 2, 4), (6, -1, 2) and (8,-7, k) are collinear if k =
View SolutionIf `| x | le y` then
View SolutionIf `it_(xrarr3)(ax^2-beta)/(x-3)=12`,then
View Solution5f(x)+3f(1/x)=x+2 and y=xf(x),then dy/dx=
View SolutionThe slope of the tangent to the curve `y=2sqrt2x`
View SolutionIf v is the variance and `sigma`is the standard diveation then
View SolutionA and B be two mutually exclusive events such that P(A)=3/8,P(B)=1/3,then `P[AcupB]`is given by
View SolutionDefine power set of a set A. Find the power set.of A = {{1}, [2, 3}}.
View SolutionIf A ={1, 2, 3, 4, 5} and B = {1, 2, 3, 7, 8, 9},find the value of `n{(A xxB)cap(BxxA)}`
View SolutionIf `f(x)=e^(x+a)`,`g(x)=x^(b^2)` and `h(x)=e^(b^2x)`,show that `(g[f(x)])/(h(x))=e^(ab^2)`
View SolutionProve that `tan (pi/12)tan((5pi)/12)tan ((7pi)/12)tan((11pi)/12)=1`
View SolutionProve that `tan62^@=2tan34^@+tan28^@`
View SolutionIf `cosalpha+cosbeta=1/3`and `sinalpha+sinbeta=1/4`,show that `tan ((alpha+beta)/2)=3/4`
View SolutionShow that `1/(sin10^@)-sqrt3/(cos10^@)=4`
View SolutionShow that `secalpha-tanalpha=cot(pi/4+alpha/2)`
View SolutionSolve :`3sin^2x+7cos^2x=6`
View SolutionIn `triangleABC angleB=60^(@), c=2sqrt(3), b =3sqrt(2)` then find `angleA`.
View SolutionIf `(2^(2n)-1)`is divisible by 3, then show that `[2^(2(n+1))-1]`is also divisible by. 3
View SolutionSimplify : `(1+i)^(-2)-(1-i)^(-2)`
View SolutionFind a quadratic equation with rational co-efficient whose one root is`4+sqrt7`
View SolutionState fundamental theorem of algebra
View SolutionIf` `^9P_5 + 5. `^9P_4` =` `^I0P_r`, find r.
View SolutionIf ``^nC_4=21xx`^(n//2)C_3`find n.
View SolutionFind the middle term (or terms) in the following expansions:
(iii) `(x^(2)-1/x)^(9)`
View SolutionThe sum to n terms of an A.P. is `n^(2)`. Find the common difference.
View SolutionIf the slope of the line joining the points (2k,-2) and (1,-k) be (-2) , find k.
View SolutionFind the equation to the circle whose parametric equations are,
`x = (1)/(2)(1+5 cos theta), y = (1)/(2)(-2 + 5sin theta)`
View SolutionFind the equation- of the porabola whose co-ordinates of the vertex and focus are (-2, 3) and (1,3) respectively.
View SolutionFind the the co-ordinates of the point on the ellipse `x^2 + 2y^2 =4` whose eccentric angle is `60^@`.
View SolutionShow that the eccentricities of the two hyperbolas `x^2/16-y^2/9=1`and `x^2/64-y^2/36=1`are equal.
View SolutionFind the co-ordinates of the point of trisection of the line segment joining the points (0, 2, 1) and (2, -1, 5) that is nearer to (0, 2, 1).
View Solutionshow that `lim_(nrarr0)(root(3)(n+1)-1)/n=1/3`
View SolutionIf f(x)=x|x|,prove that f'(x)=2|x|.
View Solutionif `lim_(xrarra)f(x)=lim_(xrarra)g(x)`,then whether f(x)=g(x) is always true?Justify your answer by an example
View SolutionFrom the following array, find out the mean deviation. 7,9,16,24,26,31,39.
View SolutionDefine ‘mutually exclusive’ and ‘mutually exhustive’ events and give examples with the help of a sample space
View SolutionTwo dice are thrown simultaneously. Find the probability that the sum of the numbers obtained will be 10.
View SolutionFor any three sets A,B and C ,prove that `A-(BcupC)=(A-B)cap(A-C)`.
View SolutionFind the domain of definition of `f(x)=log_e ((1-x)/(1+x))` Further show that`f(x_1)+f(x_2)=f((x_1+x_2)/(1+x_1x_2))`,`x_1,x_2 in (-1,1)`
View Solution`sintheta=ksin(theta+phi)`.show that `tan(theta+phi)=(sinphi)/(cosphi-K)`
View SolutionProve that `tan 20^@tan 40^@ tan 60^@ tan 80^@ = 3`
View SolutionIf `a sinalpha+bcosalpha=c` and `a cosecalpha+bsecalpha=c` show that`sin2alpha=(2ab)/(c^2-a^2-b^2)`
View SolutionProve that `4sin27^@=sqrt(5+sqrt5)-sqrt(3-sqrt5)`
View SolutionSolve :tanx+tany=1,`x+y=pi//4`
View SolutionIf `a^4+b^4+c^4+a^2b^2=2c^2(a^2+b^2)`,, then show that`angleC=60^@or120^@`
View SolutionProve using principle of mathematical induction that sum of the cube three consecutive natural number is divisible by 9.
View SolutionIf `x+iy=((a+ib)/(c+id))^(1//2)`,show that `(x^2+y^2)=(a^2+b^2)/(c^2+d^2)`
View SolutionIf one of the root of the equation `px^2 + qx + r = 0` is the cube of the other, prove that.` rp(r + p)^2 = (q^2 - 2rp)^2`.
View SolutionSolve graphically the following system of linear inequations - `x - y le 1`,`x + 2y le 8`, 2x + y ge 2`.` x ge 0`,`yge 0. ‘
View SolutionHow many 4-digit numbers can be formed from the digits 1, 1, 2, 2, 3, 3,4, 5?
View SolutionProve that the middle term in the expansion of `(1+x)^(2n)`is`(1.3.5.......(2n-1))/|___n.2^n.x^n`
View SolutionIf Sn be the sum of n consecutive terms of an A.P. show that `S_(n+4)-4S_(n+3)+6S_(n+2)-4S_(n+1)+S_n=0`
View SolutionIf n th term of a-series be `(2.3^(n-1)+1)`,, find the sum of 1st r terms of the series.
View SolutionShow that the four straight lines `xcosalpha+ysinalpha=P`,`xsinalpha-ycosalpha=-p`and `xsinalpha-ycosalpha=P`form a square.
View SolutionApplying section formulae show that the points A(4. 7,-6), B (2, 5, -4) and C(1, 4, -3) are colinear.
View SolutionEvaluate :`lim_(xrarry)(cos^2x-cos^2y)/(x^2-y^2)`
View SolutionEvaluate:`lim_(xrarr0)(sqrt(1+x^2)-sqrt(1+x))/(sqrt(1+x^3)-sqrt(1+x))`
View SolutionEvaluate: `underset(x rarr 0)(lim)(e^(3x)-1)/(log(1+5x))`
View SolutionEvaluate :`lim_(xrarrpi//2)(xtanx-pi/2 secx)`
View SolutionWater is following into a right circular conical vassel. 45 cm deep and 27 cm in diameter at the rate of `11 cm^3//min`. How fast is the water-level rising when th water is 30 cm deep?
View Solutionif y=f{f(x)},f(0)=0and f'(0)=5 find `[dy/dx]_(x=0)`
View SolutionFind the coefficient of variation of the following data :

View SolutionFind the mean deviation about median from the following data.
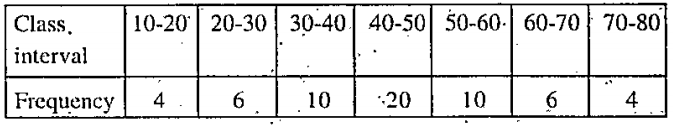
View SolutionFor any two events A and B prove that `P(A cup B) le P{A) + P (B)`.
View SolutionFrom 100 tickets numbered 1, 2,3.. ..... 100.four are drawn at random. What is the probability that 2 of them will bear numbers from1 to 40 and the other 2 will- bear any number from 41 to 100?
View SolutionTwo balls are drawn at random from a bag containing 6 white and 4 black balls. Find the chance that one is white and the other is black?
View SolutionIf the circles `x^2+y^2+2ax+c^2=0`and `x^2+y^2+2by+c^2=0`touch each other,prove that `1/a^2+1/b^2=1/c^2`
View SolutionIf `acosphi=b costheta`,show that `a tantheta+b tanphi=(a+b)tan((theta+phi)/2)`
View SolutionIf `(cot(alpha-beta))/cotalpha+(cos^2gamma)/(cos^2alpha)=1`show that `tan^2gamma+tanalphacotbeta=0`
View SolutionSolve :`cos^3xcos3x+sin^3xsin3x=0`
View SolutionIn any triangle ABC If sinA : sinB: sinC=4:5:6,then prove that cos A :cosB: cosC=12:9:2.
View Solution
EXERCISE questions and complete solutions for chapter MCQ of UNITED BOOK HOUSE of Class 11