Complete Solutions to EXERCISE of chapter MODEL QUESTION PAPERS-SET 4 of Class 11 book with complete answers and questions
| Class 11 | MATHS |
| Chapter | MODEL QUESTION PAPERS-SET 4 |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 47 |
| Academic Year | 2023-24 |
Null set Is
View SolutionThe number of real solution of `x^2-3|x|+2=0` are
View SolutionZ is a complex number and Z.`barZ=0`, it is true only when
View SolutionIf 4th,7th nd 10th term of G.P are x, y and z, then
View SolutionThe co-ordinate of the foot of the perpendicular from the point (4, 3, 5) to z axis is
View SolutionThe equation of the circle passes through the point (0, 4), (0, 0) and (3, 0) is
View SolutionThe value of`lim_(xrarr0)(1-cos4x)/x^2`is
View SolutionIf f(2) = 2, and f'(2) = 1, then the value of `lim_(xrarr2)(xf(2)-2f(x))/(x-2)`
View SolutionThree unbiased dice are thrown at a time. The probability of getting three distinct numbers in three dice is
View SolutionIf the variance of a distribution is 4 coefficient of variation is 5%,then mean of the distribution is ___
View SolutionIf `n(X) = 4` and `n(Y) = 8`. Find the maximum and minimum numbers of.elements of `X cup Y`
View SolutionIf A =` {(a, b) : a + 3b = 12, a, bin N}`, find the range of A.
View SolutionShow that `cos^6theta+sin^6theta=1/8(5+3cos4theta)`
View SolutionIf n be an integer, then find the value of `tan{(npi)/(2)+(-1)^(n).(pi)/(4)}`.
View SolutionIf (1 + i) (2 +i) (3 + i)…..(n+i)=a+ib ,show that 2.5.10….`(n^2+1)=a^2+b^2`
View SolutionIf n is a even number, find the sum of the series upto n terms`1^2-2^2+3^2-4^2+5^2-......`
View SolutionHow many 5 digits telephone number can be formed from the digits from 0 to 9 when every number start with 23 (no digit being repeated in any number)
View SolutionFind the value of `3^2/3. 3^2/9. 3^2/27`…..
View SolutionIf the gradiant of the line joining the points `(2a, -2) ` and `(1, -a) ` is `-2 `. Find the value of `a `.
View SolutionFind the ratio in which the plane 2x+3y+5z=1 divides the line segment joining the points (1,0,-3)and (1,-5,7).
View SolutionEvaluate:`lim_(xrarr0)sinx/x`
View SolutionFind the condition for the existance of `lim_(xrarr0)f(x)`
View SolutionFind the variance of 1, 2, 3, 4.
View SolutionThere are four letters and four envelops with proper address. Find the probability that the letters are not in proper envelops.
View SolutionFor any three sets `A, B, C`. prove that `A xx (B cup C)=" (A xx B) cup(A xx C)`
View SolutionIf `cos alpha = k cos beta` Show that `tan ((alpha+beta)/2)=(1-k)/(1+k)cot((alpha-beta)/2)`
View SolutionSolve : `sectheta-1=(sqrt2-1)tantheta`
View SolutionProve by mathematical induction.`1/1.3+1/3.5+1/5.7+....+1/((2n-1)(2n+1))=n/(2n+1)`
View SolutionHow many different arragements of the letters of the word ALGEBRA can be made so that the two ‘A’ is do not come together.
View SolutionIf the sum of the ` n` term of an ` AP` is `n^2` + ` 2n` . which, term will be 201?
View SolutionIf z=x+iy and `(z-i)/(z+1)`is purely imaginary,then show that the point z always lies on a circle.
View SolutionThe equation of the two adjacent sides of a parallelogram are 4x+ 5y = 0 and 7x + 2y =.0and equation of one of the diagonal is 1 lx + 7y = 9, find the equation of other diagonal.
View SolutionIn a rectangle, the co-ordinates of the end point of a diagonal are (2, 3) and (8, II) and other diagonal is parallel to y axis. Find the co-ordinate of the end points of other diagonal.
View SolutionIf the straight line dx + cy = cd is perpendicular the line joining the points (4, 5) and (3.-8) and also it passes through (6, I). Find the value of c and d
View SolutionFind the equation of a circle which passes through `(0, -3)` and `(3, -4)` and the centre lies on the straight line `2x - 5y + 12 = 0.`
View SolutionEvaluate:`lim_(xrarr0)(sqrt(1+x^2)-sqrt(1+x))/(sqrt(1+x^3)-sqrt(1+x))`
View SolutionIf f(x)=x|x|,prove that f'(x)=2|x|.
View Solution‘If x is a whole number and `x^2` is even, then x is also an even number’. Find the truth value of this statement by contrapositive method.
View Solution‘If a and b are two odd integer, then (a + b) is an even intege.”—examine whether this compound statement is true of false
View SolutionOne cord is drawn at random a pack of 52 c’ards..Find the probability the card will be either a king or a Hearts or red?
View SolutionFind the mean and variance for the following frequency distributions in
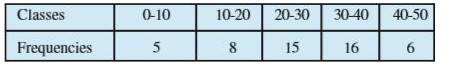
View SolutionSolve : `2cos^2 2thetacos5theta+2=sin^2theta,theta in R.`
View SolutionIf `1/(`^5C_r)+1/(`^6C_r)+1/(~^4C_r)`find the value of r.
View SolutionShow that there are 72 ways of selecting 5 letters from the word INDEPENDENT.
View SolutionThe co-ordinate of vertex and focus of a parabola `x^2 + by^2 + 2hxy+ 2gx + 2fy + c` = 0 are `(2, 4)` and `(6, 2)`. Find the the value of `b, h, g. f, c`.
View SolutionProve that the 4 foci of the two ellipses `25x^2+169y^2=4225` and `400x^2+256y^2=102400` are concyclics.
View SolutionShow that the difference of the focal distances of any point on the hyperbola `9x^2-4y^2 = 36` is equal to its transverse axis.
View Solution
EXERCISE questions and complete solutions for chapter MODEL QUESTION PAPERS-SET 4 of UNITED BOOK HOUSE of Class 11