Complete Solutions to SECTION-J (AAKASH CHALLENGERS QUESTIONS) of chapter WORK, ENERGY AND POWER of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | WORK, ENERGY AND POWER |
| AAKASH INSTITUTE | SECTION-J (AAKASH CHALLENGERS QUESTIONS) |
| Number Of Questions | 14 |
| Academic Year | 2023-24 |
Rain drops each of mass m falling from rest in air experience an upward force given by f= - bv where b is a constant and v is velocity of the drop. Using work energy theorem, derive an expression for
(A) `v=f(t)` i.e. velocity function of time.
(B) `(dE)/(dt)=f(t)` where E = Total mechanical energy of the rain drop
( C) Draw the graphs `(dE)/(dt), (dU)/(dt) and P_("viscous")` as a function of time U is potential energy and P represent power ?
View SolutionA particle is moved foom the orgin A, back to the origin, along the path ABCDEFA as shown in figure

(A) Find the work done by the force `vecF=y hati+zhatj+xhatk` acting on the particle, in the round trip?
(B) Is the force given in part (A) conservative?
View SolutionThree particeles each of mass m are converted by three identical speings each of stiffness k. The rings and springs can slide along a smooth vertical rigid circular wire of radius R. Find the potential energy of the system relative to the centre O.
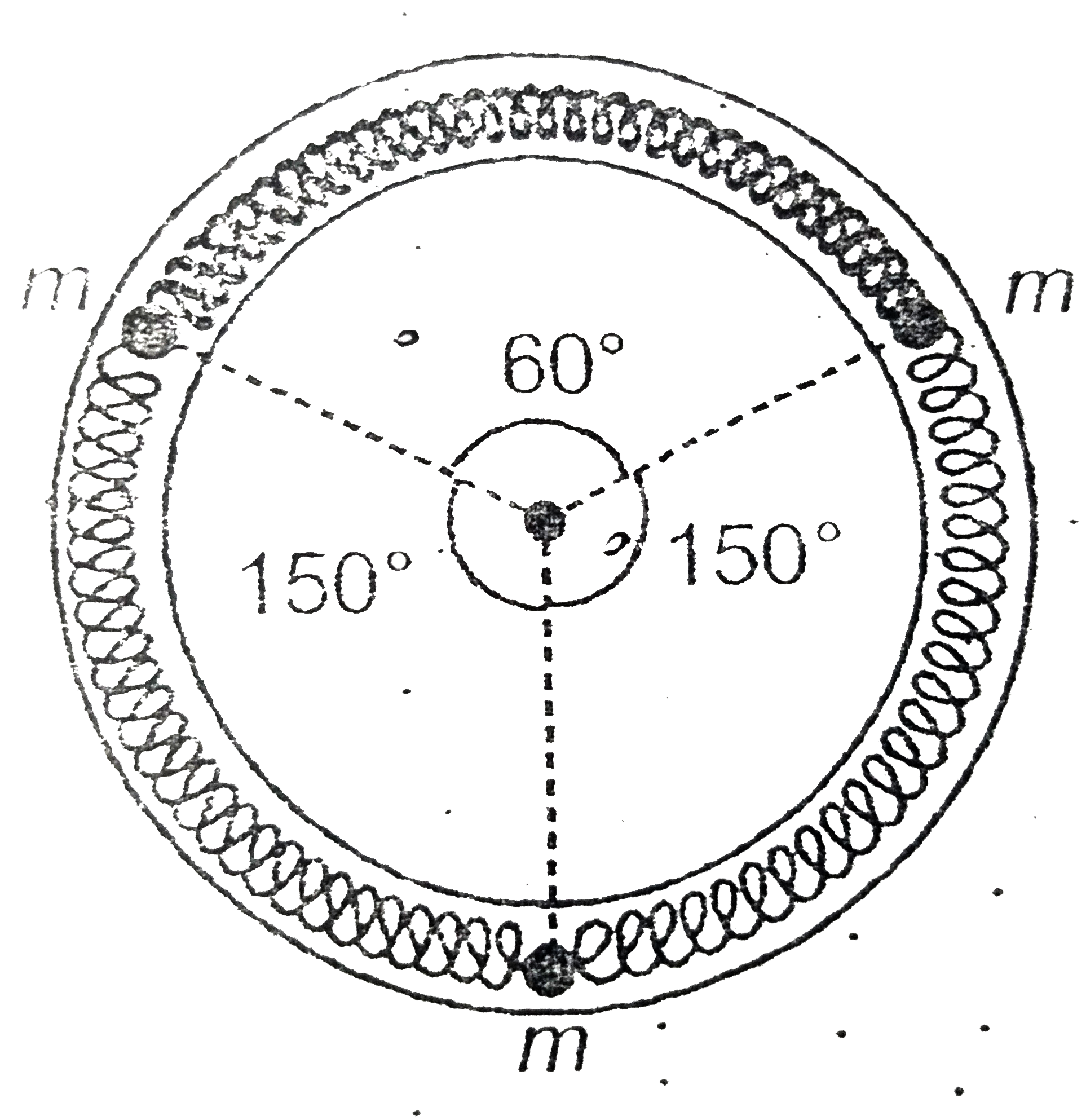
View SolutionIn a simple pendulum, the breaking strength of the string is double the weight of the bob. The bob is released from rest when the string is horizontal. The string breaks when it makes an angle `theta` with the vertical.
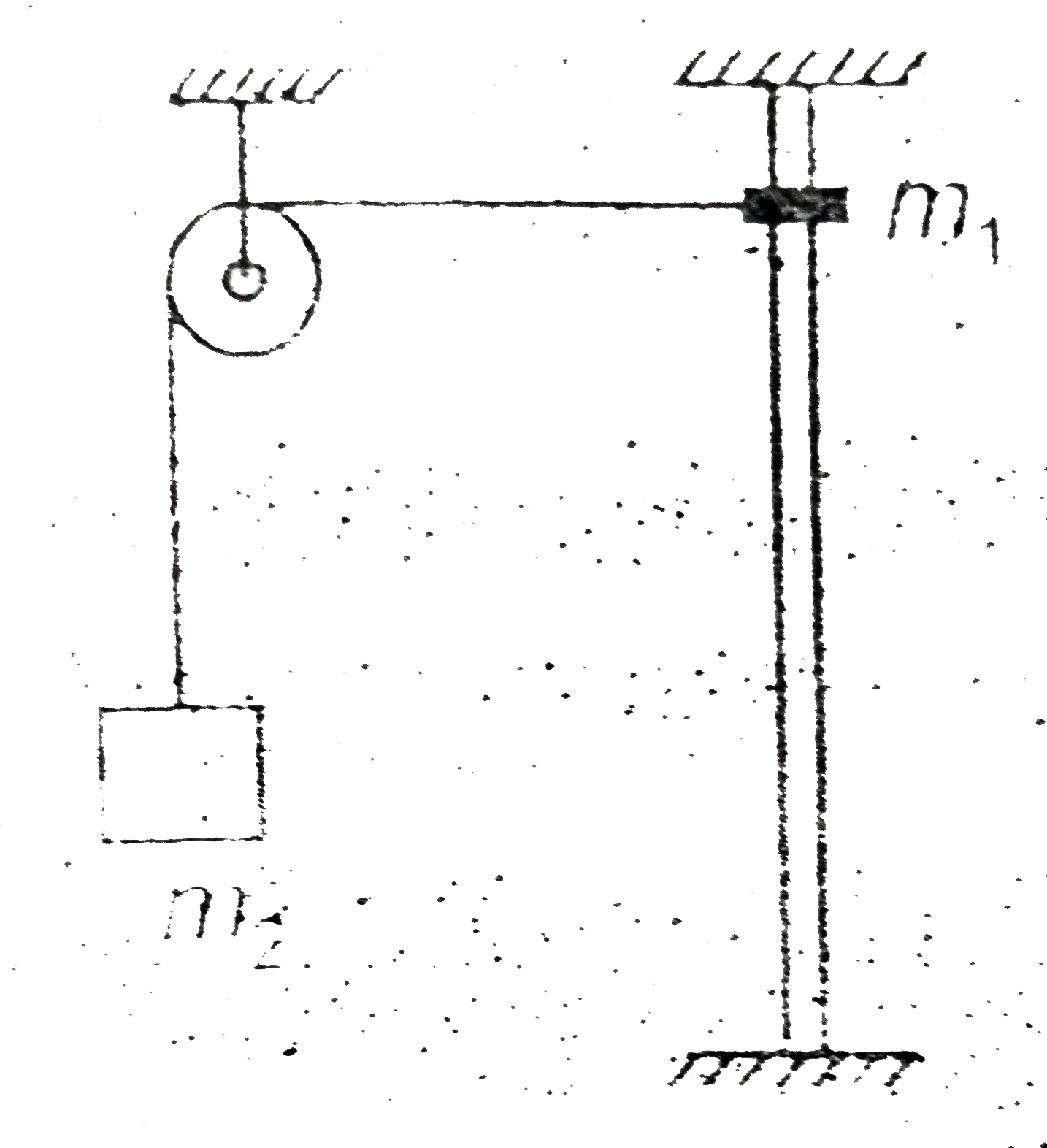
View SolutionA smooth ring of mass `m1` connected with a hanging block of mass `m2,` by a long inextensible string, is released from rest. The ring moves vertically along a fixed rigid rod as shown in figure. Describe the motion of the ring if
(A) `m1=2m2`
(B) `m1=m2`
(C ) `m1=(m2)/(2)`
View SolutionA block is projected with a speed `v_(0)` strikes the point pf projection after describing the path as shown in figure by dotted line. If friction exists for the path of length d and the vertical circular path is smooth, assuming coefficient of frictioin to be `mu`

(A) Find `v_(0)`?
(B) What is the minimum value of `v_(0)` ?
View SolutionA block of mass `m` is released from rest onto a spring. A having stiffness `k_A=mg//2h` as shown in figure. If the block compresses spring B through a distance h, find the:
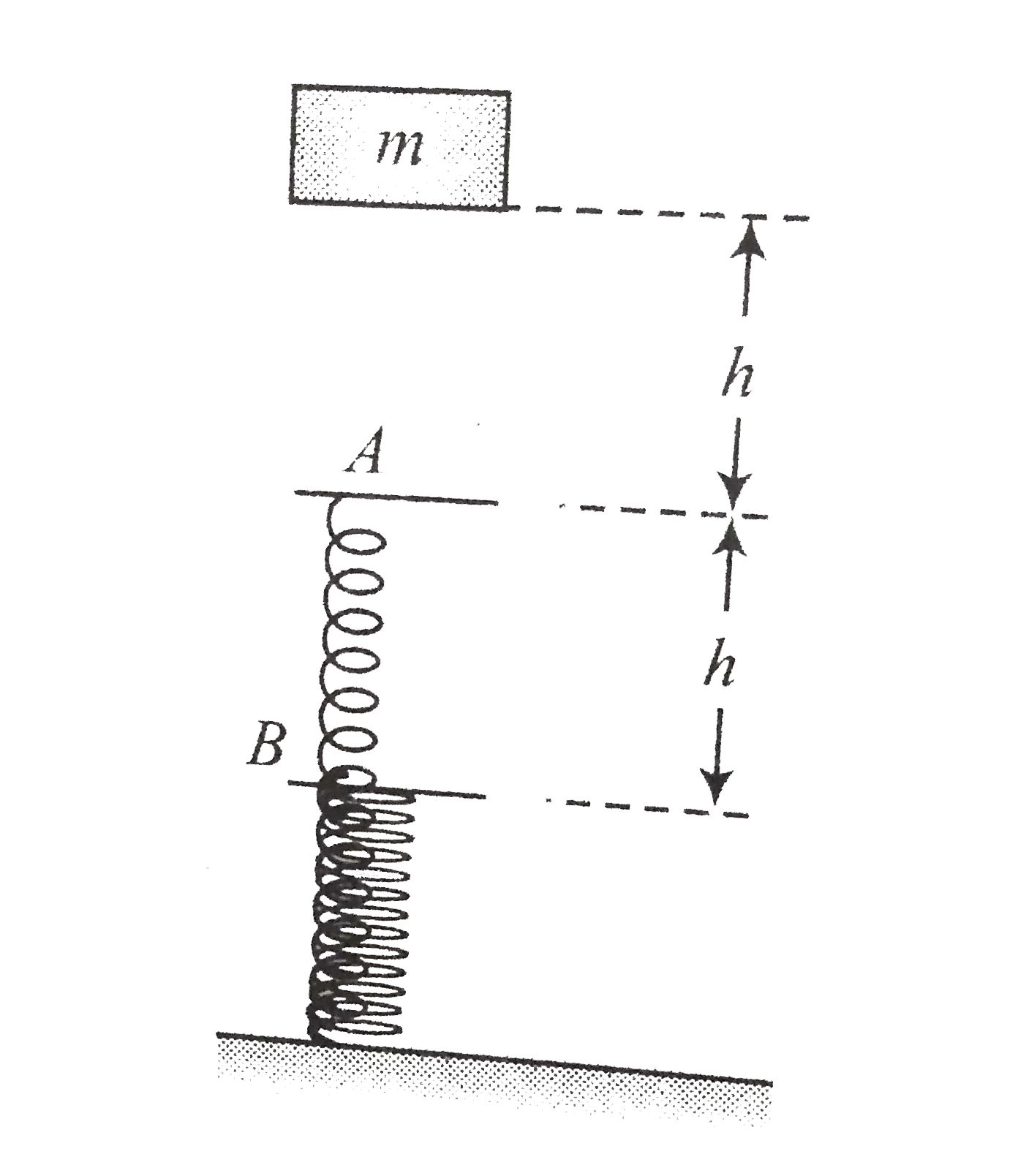
a. stiffness of the spring B
b. equilibrium position of the block
c. maximum velocity of the block
d. maximum acceleration of the block
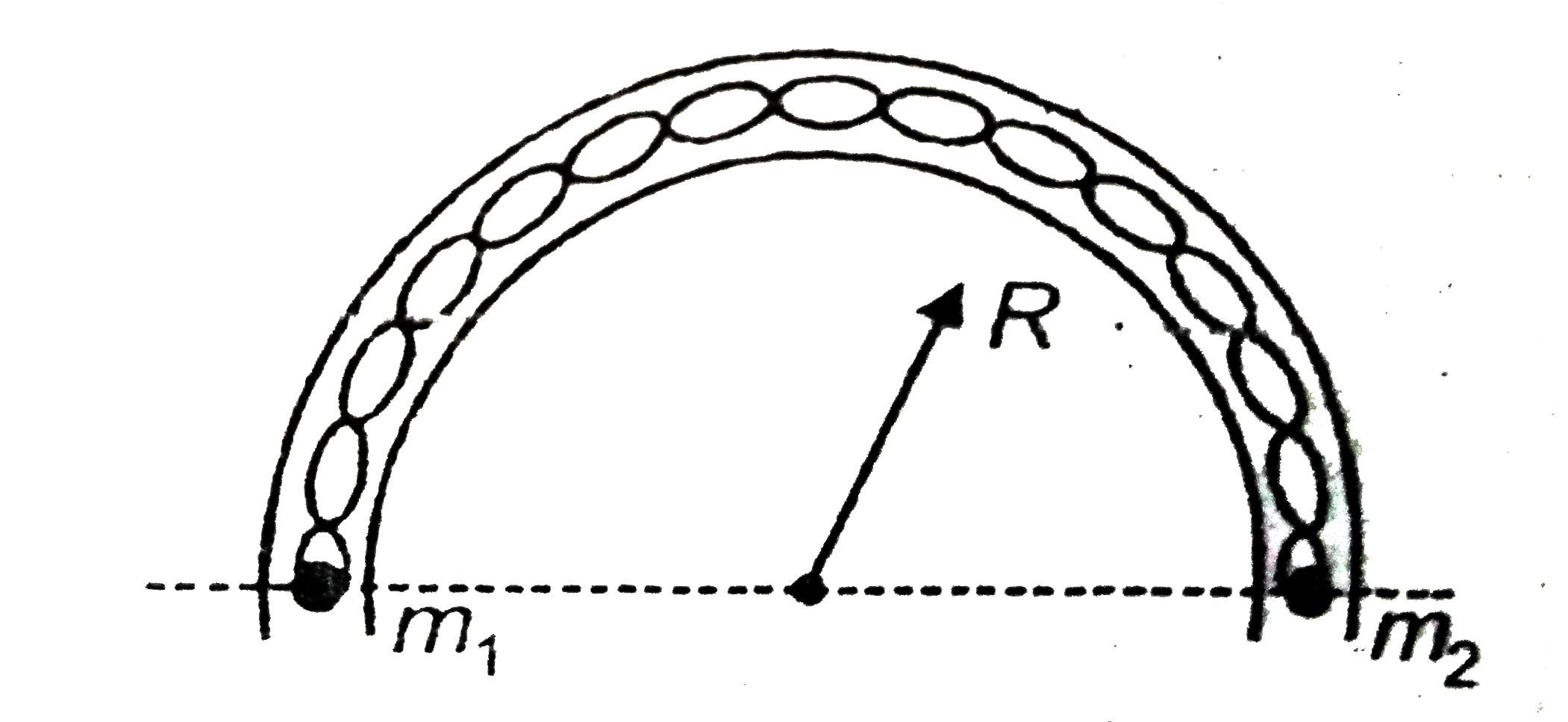
View SolutionTwo beads of masses `m_(1) and m_(2)` connected by an inextensible string are kept at rest at the horizontal diametrical points of a fixed smooth vertical semicircular tube. If the beads are released from the given position, find their speeds as the function of vertical distance y converted by mass `m_(2).`
View SolutionA partical of mass m attached with a massless spring of natural length l and stiffness constant k is releaased from rest from the horizontal positon of the relaxed spring.
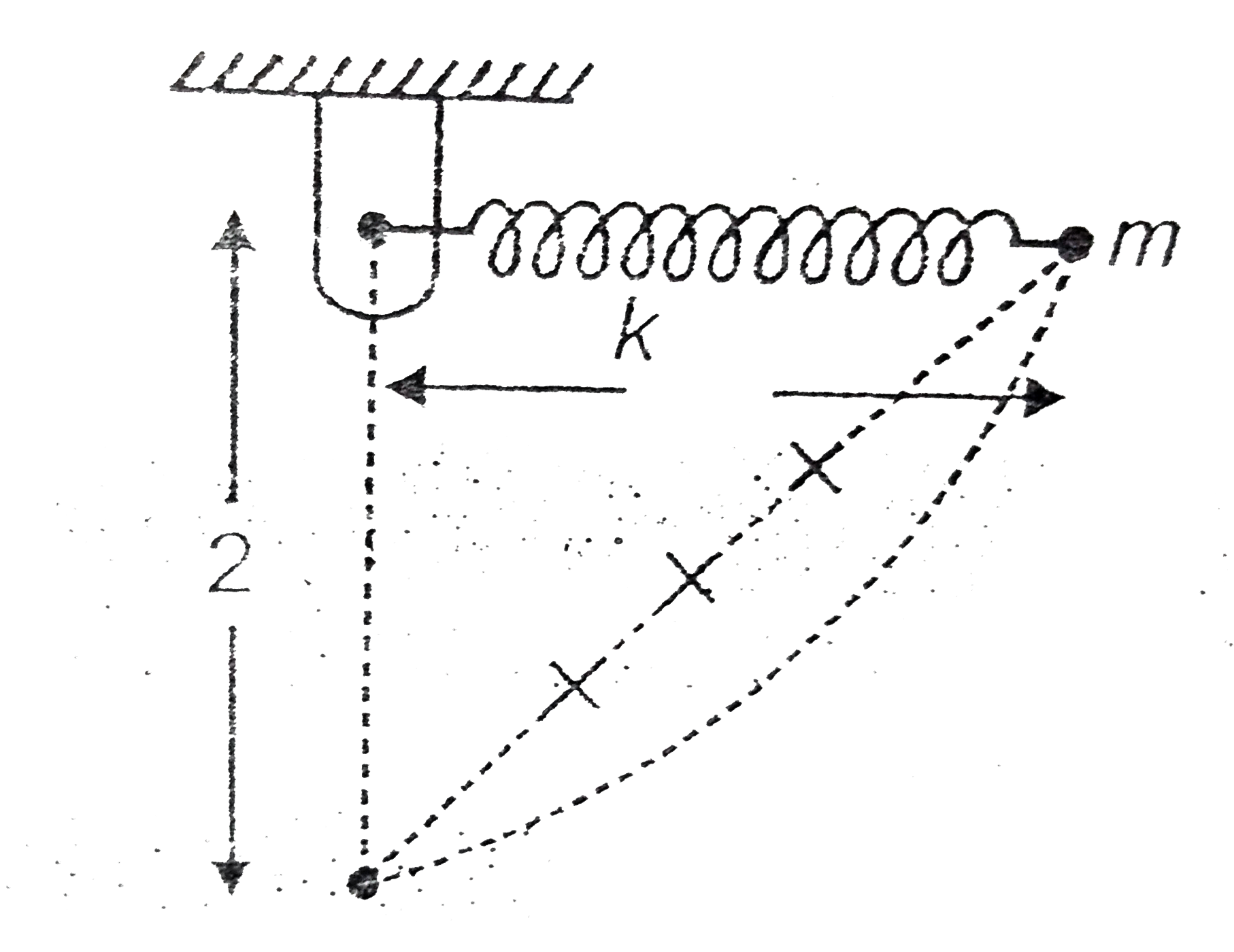
When the particle passes through its lowest point, the maximum length of the spring bocomes 2l find the
(A) Speed of the particle at its lowest point
(B) Acceleration of the particle at its lowest position.
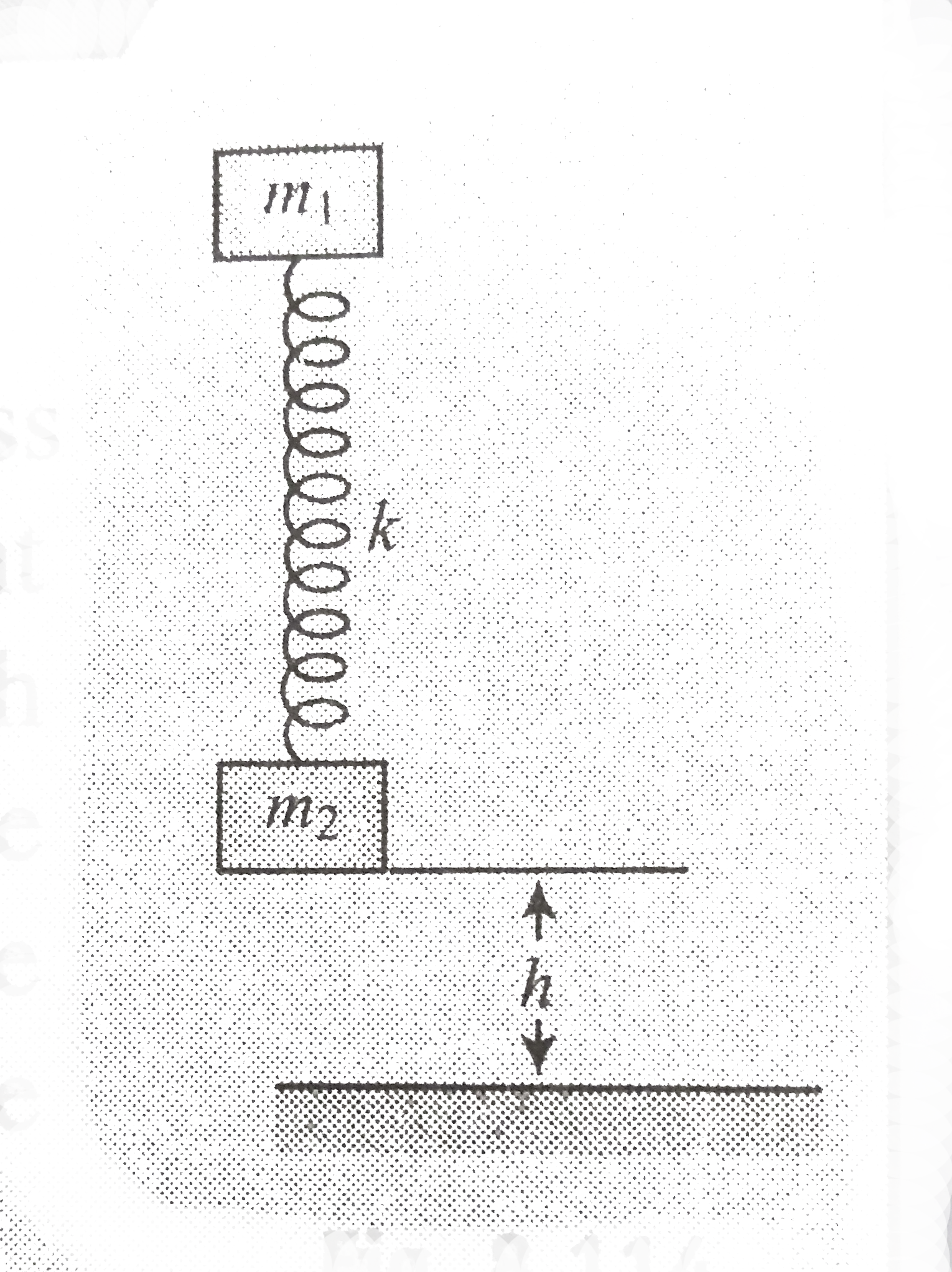
View SolutionA spring-mass system ( `m_1` + massless spring + `m_2`) fall freely from a height `h` before `m_2` colliding inelastically with the ground. Find the minimum value of `h` so that block `m_2` will break off the surface. Assume k=stiffness of the spring.
View SolutionA smooth sledge is moving with constant velocity `v_(0)` toward left. A block is pushed toward right with a velocity `v_(0)’` (relative to ground).

(A) Find the velocity with which the block escapes from the sledge and necessary critical `v_(0)` for escaping from the sledge
(B) Will the block launch onto the sledge again?
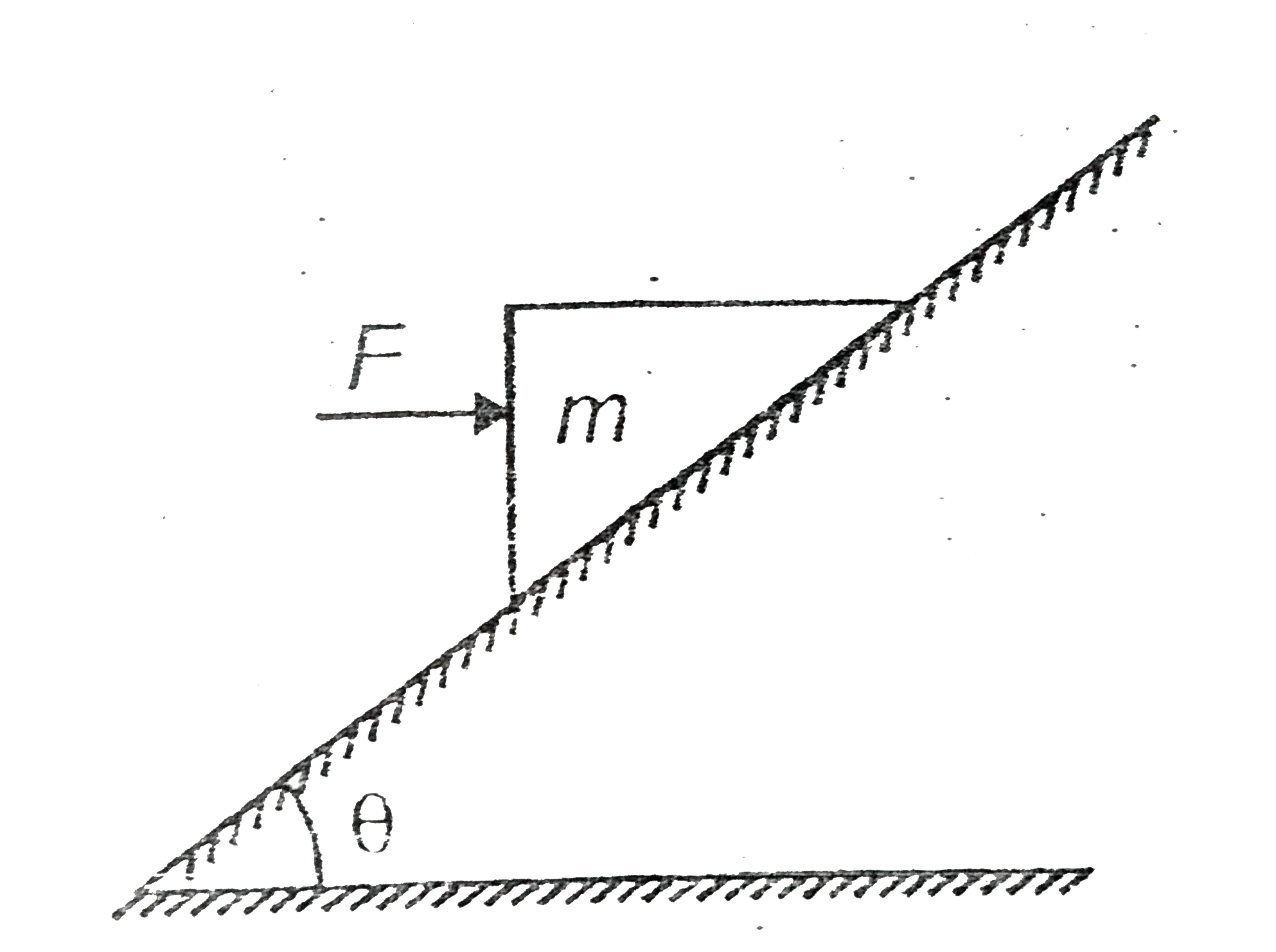
View SolutionA prismatic block of mass m is lifted through a distanced along the slant. If the coefficient of friction between the block and slant surface is 1`mu.` find the
(A) Work done by force F, friction and gravity.
(B) Speed of the block after moving through a distance d.
Assuming,` mu=1//6,theta=45%^(@)and F=2mg`
View SolutionDiscuss the stability of an atom in a molecule possessing Lennard-joners potential energy function
`U(x)=4epsi[((alpha)/(x))^(12)-((alpha)/(x))^(6)]`
where x=separation between the atoms, `alpha=0.263` nm and `epsi=1.5xx10^(-22)J`
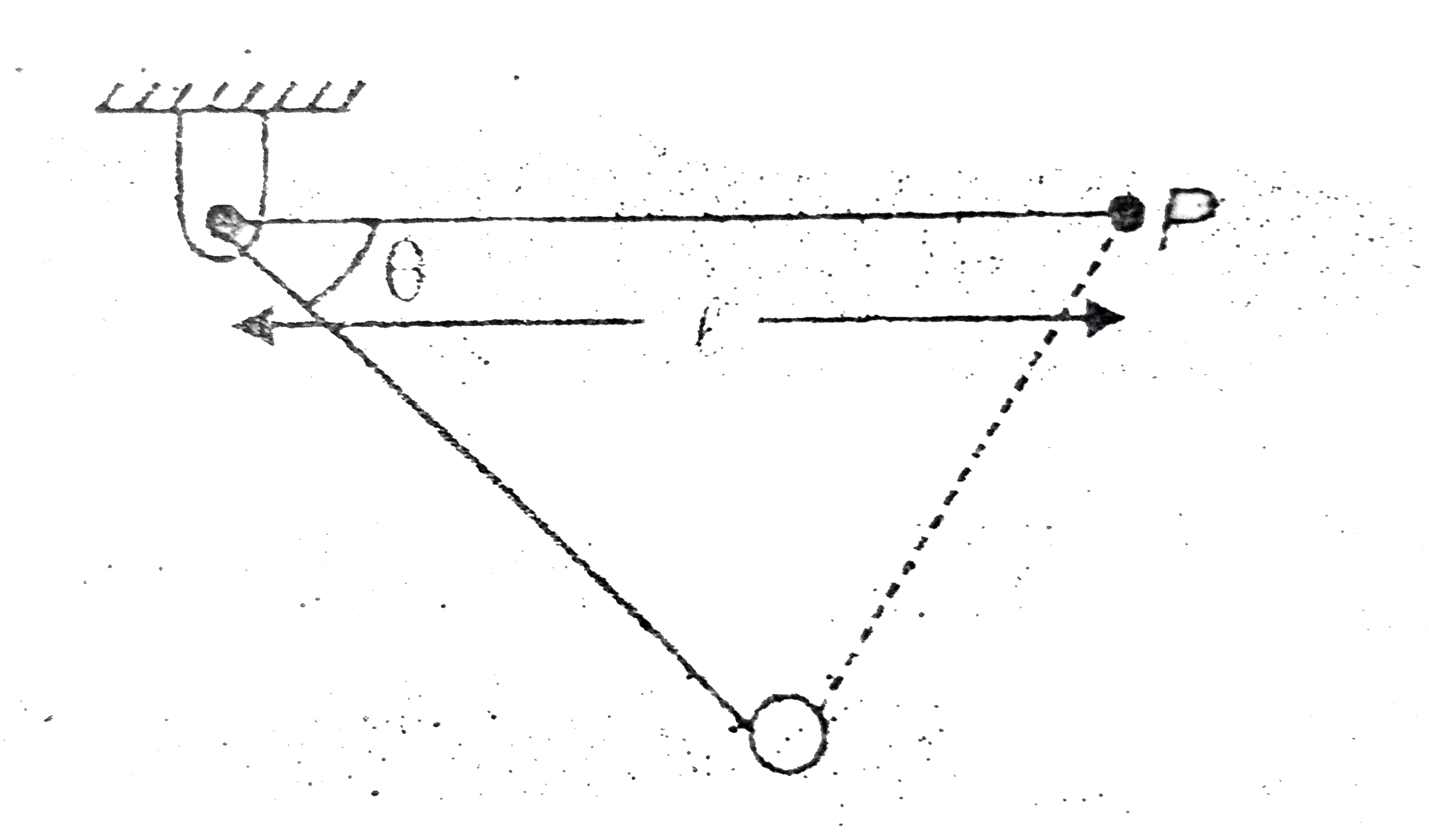
View SolutionA pendulum bob swing from the point P when the ideal string of length l is horizontal. Find the
(A) Speed
(B) Acceleration of the bob
(C ) Power delivered by gravity at an angular position `theta`
View Solution
SECTION-J (AAKASH CHALLENGERS QUESTIONS) questions and complete solutions for chapter WORK, ENERGY AND POWER of AAKASH INSTITUTE ENGLISH of Class 12
All Exercises from AAKASH INSTITUTE Class 12 PHYSICS WORK, ENERGY AND POWER
- Assignment (SECTION - A)
- Assignment (SECTION - B)
- Assignment (SECTION - C)
- Assignment (SECTION - D)
- EXAMPLE
- EXERCISE
- IILUSTRATION
- SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
- SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
- SECTION-C (OBJECTIVE TYPE QUESTIONS) (MORE THAN ONE OPTIONS ARE CORRECT)
- SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-I)
- SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-II)
- SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-III)
- SECTION-D (LINKED COMPREHENSION TYPE QUESTIONS) (COMPREHENSION-IV)
- SECTION-E (ASSERTION-REASON TYPE QUESTIONS)
- SECTION-F (MATRIX-MATCH TYPE QUESTIONS)
- SECTION-G (INTEGER ANSWER TYPE QUESTIONS)
- SECTION-H (MULTIPLE TRUE FALSE TYPE QUESTIONS)
- SECTION-I (SUBJECTIVE TYPE QUESTIONS)
- SECTION-J (AAKASH CHALLENGERS QUESTIONS)
- TRY YOURSELF