Complete Solutions to Exercise For Sesssion 1 of chapter PROPERTIES AND SOLUTION OF TRIANGLES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROPERTIES AND SOLUTION OF TRIANGLES |
| ARIHANT MATHS | Exercise For Sesssion 1 |
| Number Of Questions | 20 |
| Academic Year | 2023-24 |
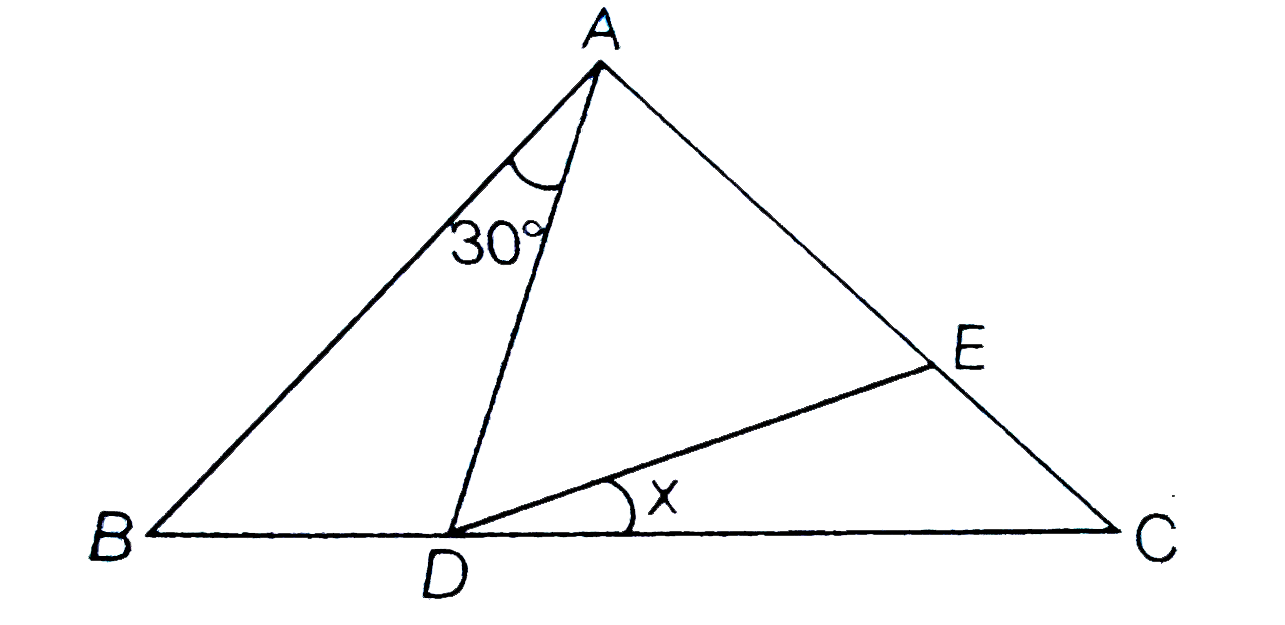
In the given figure,if `AB= AC, angle BAD=30^(@)and AE=AD,` then x is equal to
View SolutionIn `DeltaABC, a=4, b =12 and B=60^(@),` thn the vlaue of sin A is
View SolutionLet ABC be a triangle such that `angle A =45^(@) , angle B =75^(@),` then `a+c sqrt2` is equal to
View SolutionIf the angles A, B, C of `DeltaABC` are in A.P. and `b:c=sqrt(3):sqrt(2)`, show that `A=75^(@)`.
View SolutionIf `cot ""A/2 =(b+c)/(a),`then `DeltaABC` is
View SolutionIn any triangle. `if(a^2-b^2)/(a^2+b^2)=("sin"(A-B))/("sin"(A+B))` , then prove that the triangle is either right angled or isosceles.
View SolutionIn any triangle `A B C ,` prove that: `(a^2sin(B-C))/(sinB+s in C)+(b^2sin(C-A))/(sinC+s in A)+(c^2sin(A-B))/(sinA+s in B)=0`
View SolutionIn any `DeltaABC,` is `2 cos B=a/c,` then the triangle is
View SolutionProve that `((a + b + c) (b + c -a) (c + a -b) (a + b -c))/(4b^(2) c^(2)) = sin^(2)`
View SolutionIf a `cos A =b cos B, ` then the triangle is
View SolutionIn `Delta ABC, (a + b+ c) (b + c -a) = kbc` if
View SolutionIf : `a = 9, b = 8 and c = x` satisfies 3 cos C = 2, then : x =
View SolutionIn `DeltaABC, if sin^2 A+ sin^2 B = sin^2 C`, then the triangle is
View SolutionThe lengths of the sides of a triangle are `alpha-beta, alpha+beta` and `sqrt(3alpha^2+beta^2), (alpha>beta>0)`. Its largest angle is
View SolutionIn any triangle `A B C` , prove that: `(1+cos(A-B)cos C)/(1+cos(A-C)cos B)=(a^2+b^2)/(a^2+c^2)`
View SolutionIf the sides of a triangle ABC are in AP and 'a' is the smallest side, then express cos A in term of b and c.
View SolutionIn a `DeltaABC, a ^(2) cos 2B+b^(2) cos 2A+2ab cos (A-B)=`
View SolutionIn any `DeltaABC, 2 [bc cos A + ca cos B+ ab cos C]=`
View SolutionIn a `DeltaABC, tan ""1/2 (A+B) . Cot""1/2(A-B)` is equal to
View SolutionIf in a `Delta ABC, b =sqrt3, c=1 and B-C =90^(@), ` then `angle A` is
View Solution
Exercise For Sesssion 1 questions and complete solutions for chapter PROPERTIES AND SOLUTION OF TRIANGLES of ARIHANT MATHS (ENGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS PROPERTIES AND SOLUTION OF TRIANGLES
- Examples
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Single Integer Answer Type Questions)
- Exercise (Single Option Correct Type Questions)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Sesssion 1
- Exercise For Sesssion 2
- Exercise For Sesssion 3
- Exercise For Sesssion 4
- Exercise For Sesssion 5
- Exercise For Sesssion 6
- Exercise For Sesssion 7
- Exercise For Sesssion 8
- PROPERTIES AND SOLUTIONS OF TRIANGLES EXERCISE 5: MATCHING TYPE QUESTIONS
- PROPERTIES AND SOLUTIONS OF TRIANGLES EXERCISE 6 : SINGLE INTEGER ANSWER TYPE QUESTIONS
- PROPERTIES AND SOLUTIONS OF TRIANGLES EXERCISE 7 : SUBJECTIVE TYPE QUESTIONS
- PROPERTIES AND SOLUTIONS OF TRIANGLES EXERSISE 1: SINGLE OPTION CORRECT TYPE QUESTIONS
- SOLVED EXAMPLES