Complete Solutions to Examples of chapter SETS, RELATIONS AND FUNCTIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | SETS, RELATIONS AND FUNCTIONS |
| ARIHANT MATHS | Examples |
| Number Of Questions | 62 |
| Academic Year | 2023-24 |
Write the set of the letter of the word 'ALLAHABAD'. Also find the number of subsets of this set.
View SolutionLet `A`, `B` and `C` be the sets such that `A uu B=A uu C` and `A nn B = A nn C`. show that `B=C`
View SolutionLet A and B be sets. If `A nnX = B nnX =varphi`and `A uuX = B uuX`for some set X, show that `A = B`.(Hints `A = A nn(A uuX), B = B nn(B uuX)`and use Distributive law)
View SolutionIf A and B are any two sets, prove that
P(A) = P(B) implies A = B.
View SolutionIf A and B be two sets containing 6 and 3 elements respectively, what can be the minimum number of elements in `AuuB` ? Also, find the maximum number of elements in `AuuB`.
View SolutionSuppose `A_1,A_2….. A_(30)` are thirty sets each having 5 elements and `B_1B_2…..B_n` are n sets each having 3 elements ,Let `overset(30)underset(i=1)bigcupA_1=overset(n)underset(j=1)bigcupB_j=s`
and each element of S belongs to exactly 10 of the `A_1` and exactly 9 of the value of n.
View SolutionIn a group of 1000 people, there are 750 who can speak Hindi and 400 who can speak Bengali. How many can speak Hindi only? How many can speak Bengali only? How many can speak both Hindi and Bengali?
View Solutionabout to only mathematics
View SolutionA class has 175 students. The following table shows the number of students studying one or more of the following subjects in this case.
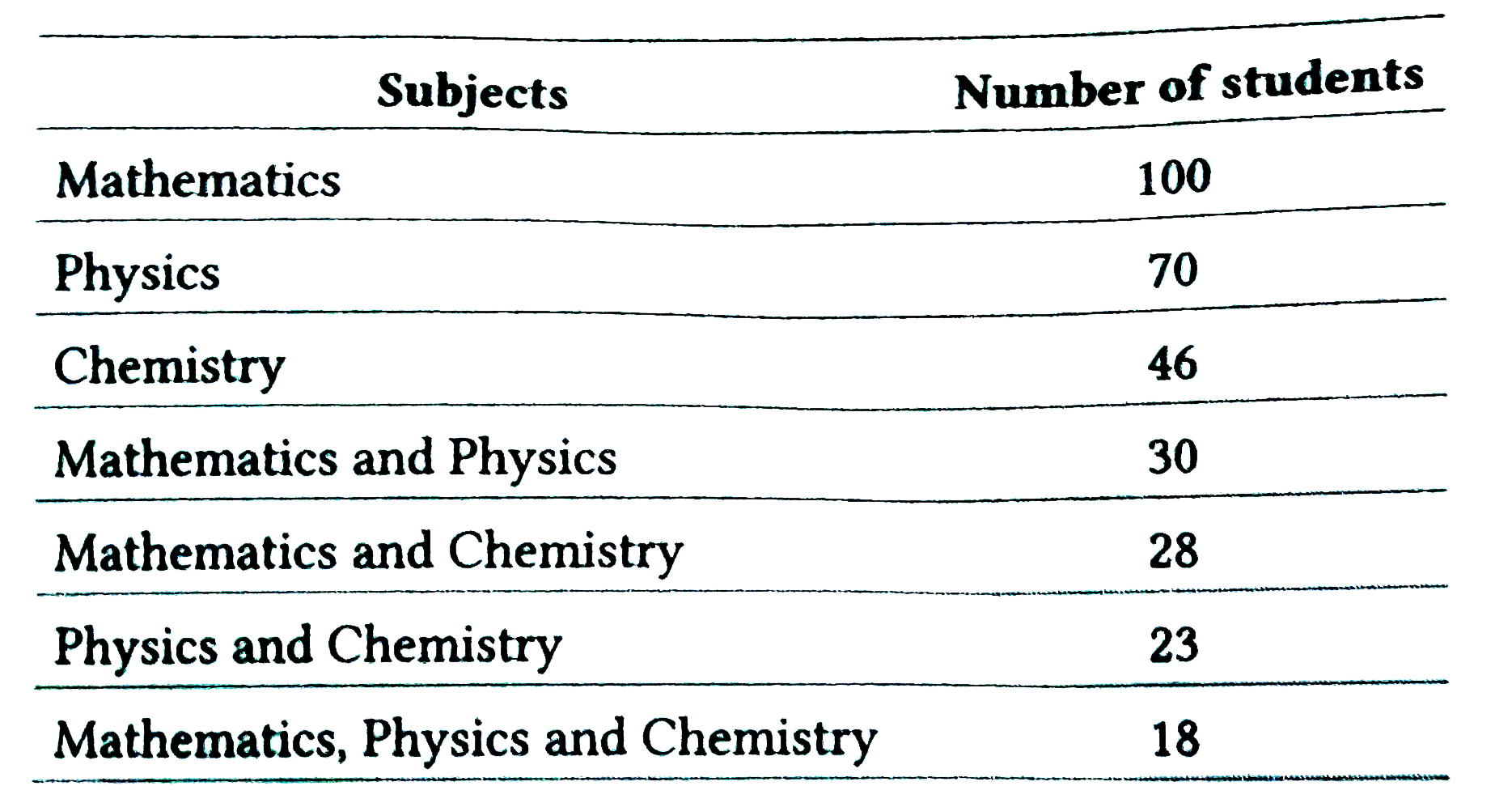
How many students are enrolled in Mathematics alone, Physics alone and Chemistry alone? Are there students who have not offered any one of these subjects?
View SolutionIn a pollution study of 1500 Indian rivers the following data were reported. 520 were polluted by sulphur compounds, 335 were polluted by phosphates, 425 were polluted by crude oil, 100 were polluted by both crude oil and sulphur compounds, 180 were polluted by both sulphur compounds and phosphates, 150 were polluted by both phosphates and crude oil and 28 were polluted by sulphur compounds, phosphates and crude oil. How many of the rivers were polluted by atleast one of the three impurities?
How many of the rivers were polluted by exactly one of the three impurities?
View SolutionIf A = {1, 2, 3} and B = {4, 5}, find `AxxB`, `BxxA` and show that `AxxBneBxxA`.
View SolutionIf A and B be two sets and `AxxB={(3,3),(3,4),(5,2),(5,4)}`, find A and B.
View SolutionIf A and B are two sets given in such a way that `AxxB` consists of 6 elements and if three elements of `AxxB` are (1,5), (2,3) and (3,5), what are the remaining elements?
View SolutionLet A = {1, 2, 3} and R = {(a,b): `a,b in A, a` divides b and b divides a}. Show that R is an identity relation on A.
View SolutionLet A = {3,5}, B = {7,1}.
Let R = {(a,b): `ainA, binB, a-b` is even}.
Show that R is an universal relation from A to B.
View SolutionProve that the relation R defined on the set N of natural numbers by xRy `iff 2x^(2) - 3xy + y^(2) = 0` is not symmetric but it is reflexive.
View SolutionLet `R` be a relation on the set `N` of natural numbers defined by `n\ R\ m` iff `n` divides `mdot` Then, `R` is (a) Reflexive and symmetric (b) Transitive and symmetric (c) Equivalence (d) Reflexive, transitive but not symmetric
View SolutionStatement-1: The relation R on the set `N xx N` defined by (a, b) R (c, d) `iff` a+d = b+c for all a, b, c, d `in` N is an equivalence relation.
Statement-2: The intersection of two equivalence relations on a set A is an equivalence relation.
View SolutionA relation R on set of complex numbers defined by `Z_(1)RZ_(2) hArr (Z_(1)-Z_(2))/(Z_(1)+Z_(2))` is real then which of the following is not true ?
View SolutionLet R be a relation such that `R = {(1,4), (3,7), (4,5), (4,6), (7,6)}`, check R is a function or not ?
View SolutionLet f : N `to N : f(x) =2 x` for all `x in N`
Show that f is one -one and into.
View SolutionLet the function `f:R to R` be defined by `f(x)=cos x, AA x in R.` Show that `f` is neither one-one nor onto.
View SolutionLet `f : R rarr R` be defined by f(x) = cos (5x+2). Is f invertible? Justify your answer.
View SolutionIf `f:R -> R, g:R -> R` defined as `f(x) = sin x and g(x) = x^2`, then find the value of `(gof)(x) and (fog)(x) `and also prove that `gof != fog`.
View SolutionIf `f : R rarr R and g : R rarr R` be two mapping such that f(x) = sin x and g(x) = `x^(2)`, then
find the values of (fog) `(sqrt(pi))/(2) "and (gof)"((pi)/(3))`.
View SolutionIf the mapping `f:{1,3,4}vec{1,2,5}a n dg:{1,2,5}vec{1,3},` given by `f={(1,2),(3,5),(4,1)}a n dg={(2,3),(5,1),(1,3)},` write `fogdot`
View SolutionLet `A={x in Z:0 le x le 12}`. Show that `R={(a,b):a,b in A,|a-b|is divisible by 4}` is an equivalence relation. Find the set of all elements related to 1. Also write the equivalence class [2]
View SolutionFind congruent solutions of `155-=7` (mod 4).
View SolutionFind all congruent solutions of `8x -= 6` (mod 14).
View SolutionTwo finite sets have m and n elements respectively . The total number of subsets of first set is 56 more than the total number of subsets of the second. Find the values of m and n.
View SolutionIf `aN = {ax:x in N} and bNnncN=dN,`where`b,cin N` are relatively prime, then show that `d=bc`.
View SolutionIn a town of 10,000 families it was found that 40 % families buy newspaper A, 20 % buy newspaper B and 10 % buy newspaper C .also 5 % families buy newspaper A and B 3 % buy newspaper B and C and 4 % buy newspaper A can C ,If 2 % families buy all the three newspaper , then number of families which buy newspaper A only is
View SolutionLet `R` be the relation on the set R of all real numbers defined by a `R b` Iff `|a-b| le1.` Then `R` is
View SolutionThe relation R defined in `A= {1, 2, 3}` by `aRb` if `|a^2-b^2| leq 5`. Which of the following is faise
View SolutionIf `f(x)=(1)/((1-x)),g(x)=f{f(x)}andh(x)=f[f{f(x)}]`. Then the value of f(x).g(x).h(x) is
View SolutionIf `l` is the set of integers and if the relation R is defined over l by aRb, iff a - b is an even integer, `a,b inl`, the relation R is:
View SolutionIf `f(x)=(a-x)/(a+x)`, the domain of `f^(-1)(x)` contains
View SolutionIf `f(x)=(sin([x]pi))/(x^2+x+1)` , where `[dot]` denotes the greatest integer function, then
View SolutionIf `A={x: |x|lt2},B={x: |x-5|le2}`,
`C={x: |x|gtx}andD={x: |x|ltx}`
The number of integral values in `AuuB` is
View SolutionIf `A={x: |x|lt2},B={x: |x-5|le2}`,
`C={x: |x|gtx}andD={x: |x|ltx}`
The number of integral values in `AuuC` is
View SolutionIf `A={x: |x|lt2},B={x: |x-5|le2}`,
`C={x: |x|gtx}andD={x: |x|ltx}`
The number of integral values in `AnnD` is
View SolutionIf `A={x:x^(2)-2x+2gt0}andB={x:x^(2)-4x+3le0}`
`AnnB` equals
View SolutionIf `A={x:x^(2)-2x+2gt0}andB={x:x^(2)-4x+3le0}`
A - B equals
View SolutionIf `A={x:x^(2)-2x+2gt0}andB={x:x^(2)-4x+3le0}`
`AuuB` equals
View SolutionIf `f:R^(+)rarrA`, where `A={x:-5ltxltoo}` is defined by f(x) = `x^(2)` - 5 and if
`f^(-1)(13)={-lambdasqrt((lambda-1)),lambdasqrt((lambda-1))}`, the value of `lambda` is
View SolutionIf `A={2,3},B={4,5}andC={5,6}`, then `n{(AxxB)uu(BxxC)}` is
View SolutionState is it reflexive A ={1,2,3} R= { (1,1) , (2,2 ), ( 3,3)}
View SolutionStatement-1 If `AuuB=AuuC` and `AnnB=AnnC`, then B = C.
Statement-2 `Auu(BnnC)=(AuuB)nn(AuuC)`
View SolutionStatement-1 If U is universal set and B = U - A, then n(B) = n(U) - n(A).
Statement-2 For any three arbitrary sets A, B and C, if C = A - B, then n(C ) = n(A) - n(B).
View SolutionIf `A = A uu B`, prove that `B = A nn B`.
View SolutionFind the smallest set `A` such that `Auu{1,2}={1,2,3,5,9}dot`
View SolutionIf P, Q and R are the subsets of a set A, then prove that `Rxx(P^(c )uuQ^(c ))^(c )=(RxxP)nn(RxxQ)`.
View SolutionCheck the following relations `R` and `S` for reflexivity, symmetry and transitivity: `a R b` iff `b` is divisible by `a ,\ a ,\ b in N` (ii) `l_1` `S\ l_2` iff `l_1_|_l_2` , where `l_1` and `l_2` are straight lines in a plane.
View SolutionCheck the relation `rho` for reflexive, symmetry and transitivity:
`alpha rho beta` iff `alpha` is perpendicular to `beta`, where `alpha` and `beta` are straight lines in a plane.
View SolutionLet `f:[0, 1] rarr [0, 1]` be defined by `f(x) = (1-x)/(1+x), 0lexle1` & `g:[0,1]rarr[0,1]` be defined by `g(x)=4x(1-x), 0lexle1`
Determine the functions `fog` and `gof`.
Note that `[0,1]` stands for the set of all real members `x` that satisfy the condition `0lexle1`.
View SolutionIf A, B are two sets, prove that `AuuB=(A-B)uu(B-A)uu(AnnB)`.
Hence or otherwise prove that
`n(AuuB)=n(A)+n(B)-n(AnnB)`
where, n(A) denotes the number of elements in A.
View SolutionIf `A ={ theta : 2cos^2 theta + sintheta <=2}` , and `B = {theta: pi/2<=theta<= (3pi)/2}` then the region for `(AnnB)` is _____
View SolutionAn investigator interviewed 100 students to determine their preferences for the three drinks, milk (M), coffee (C ) and tea (T). He reported the following: 10 students has all three drinks M, C, T, 20 had M and C, 30 had C and T, 25 had M and T, 12 had M only, 5 had C only and 8 had T only. Using a Venn diagram, find how many did not take any of the three drinks?
View SolutionIn a certain city, only 2 newspapers A and B are published. It is known that 25% of the city population read A and 20% read B while 8% reads both A and B. It is also known that 30% of those who read A but not B look into advertisement and 40% of those who read B but not A look into advertisements while 50% of those who read both A and B look into advertisements. What is the percentage of the population who read an advertisement?
View SolutionAn analysis of 100 personal injury claims made upon a motor insurance company revealed that loss or injury in respect of an eye, an arm, a leg occurred in 30, 50 and 70 cases, respectively. Claims involving this loss or injury to two of these members numbered 44. How many claims involved loss or injury to all the three, we must assume that one or another of three members was mentioned in each of the 100 claims?
View SolutionLet `N` denote the set of all natural numbers and R be the relation on `NxN` defined by `(a , b)R(c , d) a d(b+c)=b c(a+d)dot` Check whether R is an equivalence relation on `NxNdot`
View SolutionThe sets S and E are defined as given below:
`S={(x,y): |x-3|lt1and|y-3|lt1}` and
`E={(x,y):4x^(2)+9y^(2)-32x-54y+109le0}`.
Show that `SsubE`.
View Solution
Examples questions and complete solutions for chapter SETS, RELATIONS AND FUNCTIONS of ARIHANT MATHS (ENGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS SETS, RELATIONS AND FUNCTIONS
- Examples
- Exercise (Matching Type Questions)
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Single Integer Answer Type Questions)
- Exercise (Single Option Correct Type Questions)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Session 1
- Exercise For Session 2
- Exercise For Session 3