Complete Solutions to Examples of chapter DIFFERENTIATION of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | DIFFERENTIATION |
| ARIHANT MATHS | Examples |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Differentiate the following functions w.r.t x using first principle.
`f(x) = tan x `
View SolutionDifferentiate the following functions w.r.t x using first principle.
`e^(x^(2)`
View SolutionDifferentiate the following functions w.r.t x using first principle.
`sqrtsinx`
View SolutionIf `y=sinx+e^(x),` Then find `(dy)/(dx)`.
View SolutionIf `Y=x^(2)+sin^(-1)x+log_(e)x,` then find `(dy)/(dx)`
View SolutionIf `y=log_(10)x+log_(e)x+log_(10)10`, then find `(dy)/(dx)`
View SolutionIf `y=x^(-1//2)+log_(5)x+(sinx)/(cosx)+2^(x),` then find `(dy)/(dx)`
View SolutionIf `y=m^(2)sec^(-1)x,` then find `(dy)/(dx)`.
View SolutionIf `y=log_ex^(3)+3sin^(-1)x+kx^(2)`, then find `(dy)/(dx)`
View SolutionIf `y=e^(x)sin x`, then find `(dy)/(dx)`
View SolutionIf `y=e^(x)tanx+x.log_(e)x,` then find `(dy)/(dx)`
View SolutionLet `f, g and h` are differentiable functions. If `f(0) = 1; g(0) = 2; h(0) = 3` and the derivatives of theirpair wise products at `x=0` are `(fg)'(0)=6;(g h)' (0) = 4 and (h f)' (0)=5` then compute the value of `(fgh)'(0)`.
View SolutionIf `y=(1+x)(1+x^2)(1+x^4)(1+x^(2n)),` then find `(dy)/(dx)a tx=0.`
View SolutionIf`y=(x)/(x^(2)+1)`, then find `(dy)/(dx)`
View Solutionif `y=(xsinx)/(log_(e)x),` then find `(dy)/(dx)`
View SolutionIf `y=(3a^(2)x-x^(3))/(a^(3)-3ax^(2))` then find `(dy)/(dx)`
View SolutionIf `y=(e^(x)-tanx)/(x^(n)+cotx)`, then find `(dy)/(dx)`
View SolutionIf `y=(log_ex)/(x)+e^(x)sinx+log_(5)x` then find `(dy)/(dx)`.
View SolutionIf `y=(x^4+x^2+1)/(x^2+x+1)` then `(dy)/(dx)=a x+b` , find `a` and `b`
View SolutionLet `y=(secx+tanx-1)/(tanx-secx+1)`.If `((dy)/(dx))_(x=(pi)/4)=a+sqrt(b)`, then value of `a+b` is equal to
View SolutionIf `y=(tan^(-1)x-cot^(-1)x)/(tan^(-1)x+cot^(-1)x)` , find `((dy)/(dx)])_(x=-1)` 0 (b) 1 (c) `2//pi` (d) `-1`
View SolutionIf `y=e^((tan^(-1)x)^(3)` then find `(dy)/(dx)`
View Solution`y=log_e(tan^(- 1)sqrt(1+x^2))` then `dy/dx` is
View SolutionFind `(dy)/(dx)` , when `y=e^(a x)cos(b x+c)`
View SolutionDifferentiate the following w. r. t. x. `sqrt[log_e{sin(x^(2)/(3)-1)}]`
View SolutionIf `y=xcosy+ycosx,` then find `(dy)/(dx)`
View SolutionIf ` ysqrt(1-x^(2)) +xsqrt( 1-y^(2)) =1,then (dy)/(dx)=`
View SolutionIf `sqrt(1-x^6)+sqrt(1-x^6)=a(x^3-y^3),` then prove that `(dy)/(dx)=(x^2)/(y^2)sqrt((1-y^6)/(1-x^6))`
View SolutionIf `y=sqrt(sinx+sqrt(sinx+sqrt(sinx+ . . .oo)))`, then `(dy)/(dx)` is equal to
View SolutionIf `y=sec^(-1)(sqrt(1+x^(2)))`, when `-1 lt x lt 1,` then find `(dy)/(dx)`
View Solution`y=tan^(- 1)sqrt((1-x)/(1+x))f i n d(dy)/(dx)`
View SolutionProve that `tan^(-1)(sqrt((1-cosx)/(1+cosx))=x/2, x lt pi`.
View SolutionIf `y=tan^(-1)((sqrt(1+x^(2))-1)/(x))`, then find`(dy)/(dx)` when `-1lexle1.`
View SolutionDifferentiate the following functions with respect to `x` : `tan^(-1)((a+x)/(1-a x))` (ii) `tan^(-1)((acosx-bsinx)/(bcosx+asinx)),\ -pi/2-1`
View Solutionयदि ` y= sin ^(-1) {(5x+ 12sqrt (1-x^(2)))/(13)}` तब `(dy)/(dx)` का मान ज्ञात कीजिए|
View SolutionFind `(dy)/(dx)` for `y=tan^(-1)sqrt((a-x)/(a+x),)-a
View SolutionSketch the graph for `y=sin^(-1)(sinx).`
View SolutionSketch the graph for `y=cos^(-1)(cosx).`
View SolutionSketch the graphs for `y=sin (sin^(-1)x)`
View SolutionSketch the graphs for `y=cos(cos^(-1)x)`
View SolutionSketch the graphs for `y=tan(tan^(-1)x)`
View SolutionDraw the graph of `y="cosec"("cosec"^(-1)x)" or "y=sec(sec^(-1)x)`.
View SolutionSketch the graphs for `y=sec(sec^(-1)x)`
View SolutionSketch the graphs for `y=cot(cot^(-1)x)and ` hence find `(dy)/(dx)`
View SolutionIf `x=e^(-t^(2)), y=tan^(-1)(2t+1)`, then `(dy)/(dx)=`
View SolutionFind `(dy)/(dx)` if `x=a(theta-sintheta)` and `y=a(1-costheta)` .
View SolutionIf `x=sqrt(a^sin^((-1)t)), y=sqrt(a^cos^((-1)t))`, show that `(dy)/(dx)=-y/x`
View SolutionIf `x=a(cost+1/2logtan^2t)` and `y=asint` then find `(dy)/(dx)` at `t=pi/4`
View SolutionIf `y=x^(sin x),` then find `(dy)/(dx)`
View Solution`x^y*y^x=1` . Find `dy/dx`
View SolutionIf `(tan^(-1)x)^(y)+y^(cotx)=1,` then find `(dy)/(dx).`
View SolutionIf `f(x)=prod_(n=1)^(100)(x-n)^(n(101-n)` then find `(f(101))/(f,(101))`
View SolutionIf `y=sqrt(logx+sqrt(logx+sqrtlogx+......oo)), " then " dy/dx ` is
View SolutionDifferentiate `sin^(-1)((2x)/(1+x^(2)))` w. r. t. `tan^(-1)x, -1 lt x lt 1`.
View SolutionIf `x in((1)/(sqrt2)1).` Differentiate `tan^(-1)(sqrt(1-x^(2))/(x)) w.r. t. cos^(-1)(2xsqrt(1-x^(2))).`
View SolutionFind the derivative of `sec^(-1)((1)/(2x^(2)-1))" w.r.t. "sqrt(1-x^(2))" at "x-(1)/(2).`
View SolutionIf `y=f(x^(3)),z=g(x^(5)),f'(x)=tanxandg'(x)=secx` then find the value of `lim_(xrarr0)(dy//dz)/(x)`.
View SolutionFind the derivative of `f(tanx)` w.r.t. `g(secx)` at `x=pi/4` , where `f^(prime)(1)=2` and `g^(prime)(sqrt(2))=4` .
View SolutionIf `y=x^(3)log_(e)x,` then find `y'' andy'''.`
View SolutionIf `y=sin(sinx)` then prove that
`(d^2y)/(dx^2)+tanx. (dy)/(dx)+y cos^2x=0`
View SolutionIf `x=a(tsint)andy=(1-cost),` then find `(d^(2)y)/(dx^(2))`.
View Solution112. If f(x)=`[[x,x^2,x^3],[1,2x,3x^2],[0,2,6x]]` find`f'(x)`
View Solution`|[x^2+a^2,ab,ac] , [ab,x^2+b^2,bc] , [ac,bc,x^2+c^2]|=`
View SolutionLet `f(x)=|x^3sinxcosx6-1 0p p^2p^3|,w h e r ep` is a constant. Then `(d^3)/(dx^3)(f(x))` at `x=0` is `p` (b) `p-p^3` `p+p^3` (d) independent of `p`
View Solutionif y= sin mx, them the value of the determinant
`|{:(y,,y_(1),,y_(2)),(y_(3),,y_(4),,y_(5)),(y_(6),,y_(7),,y_(8)):}|" Where " y_(n)=(d^(n)y)/(dx^(n)) " is "`
View SolutionIf `f(x)=|((1+x)^a,(1+2x)^b,1),(1,(1+x)^a,(1+2x)^b), ((1+2x)^b,1,(1+x)^a)|` then find constant term and coefficient of x
View SolutionIf `a_i, b_i in N` for i 1,2,3, then coefficient of x in the determinant;`|((1+x)^(a_1b_1),(1+x)^(a_1b_2),(1+x)^(a_1b_3)),((1+x)^(a_2b_1),(1+x)^(a_2b_2),(1+x)^(a_2b_3)), ((1+x)^(a_3b_1),(1+x)^(a_3b_2),(1+x)^(a_3b_3))|`
View SolutionIf `y=f(x)=x^3+x^5` and `g` is the inverse of `f` find `g^(prime)(2)`
View SolutionLet `f(x) = exp(x^3 +x^2+x)` for any real number and let `g(x)` be the inverse function of `f(x)` then `g'(e^3)`
View SolutionLet `f` be a twice differentiable function such that `f^(x)=-f(x),a n df^(prime)(x)=g(x),h(x)=[f(x)]^2+[g(x)]^2dot` Find `h(10)ifh(5)=11`
View Solutionif `y=((a-x)sqrt(a-x)-(b-x)sqrt(x-b))/(sqrt(a-x)+sqrt(x-b))` then `dy/dx` wherever it is defined is equal to`:`
View SolutionIf `x^2+y^2=R^2(w h e r eR >0)a n dk=(y^n)/((1+y^2)^3)` then find `k` in terms of `R` alone.
View SolutionLet `f(x)=x+ sin x` . Suppose g denotes the inverse function of f. The value of `g'(pi/4+1/sqrt2)` has the value equal to
View SolutionLet `e^(f(x))=lnxdot` If `g(x)` is the inverse function of `f(x),` then `g^(prime)(x)` equal to: `e^x` (b) `e^x+x` . `e^x+e^x` (d)
View SolutionGiven: `f(x)=4x^3 - 6x^2 cos 2 a + 3x sin 2 a sin 6 a+ sqrt (In (2 a - a^2)` then
View SolutionSuppose `f(x)=e^(ax) + e^(bx)`, where `a!=b`, and that `fprimeprime(x)-2fprime(x)-15f(x)=0` for all `x`. Then the value of `ab` is equal to:
View SolutionWhich of the following is not true `log(1+x)ltx` for `xgt0`
View SolutionIf `x^(p) y^(q) = (x + y)^((p + q)) " then " (dy)/(dx)=` ?
View SolutionTwo functions f & g have first & second derivatives at x=0 & satisfy the relations, `f(0) = 2/(g(0)), f'(0)=2g'(0) = 4g(0), g"(0)= 5 f"(0)=6f(0) = 3` then-
View Solution`f(x)=|x^(2)-3x|x|+2|.` Then which of the following is/are true ?
View SolutionConsider `f(x)=(x)/(x^(2)-1)and(x)=f''(x)` Statement I Graph of `g(x)` is cancave up for `xgt1`. Statement II `(d^(n))/(dx^(n))f(x)=((-1)^(n)n!)/(2){(1)/((x-1)^(n+1))+(1)/((x+1)^(n+1))}n"inN`
View SolutionStatement 1: If differentiable function `f(x)` satisfies the relation `f(x)+f(x-2)=0AAx in R ,` and if `((d/(dx)f(x)))_(x=a)=b ,t h e n((d/(dx)f(x)))_(a+4000)=bdot` Statement 2: `f(x)` is a periodic function with period 4.
View SolutionLet `f(x) = x [x],` where [*] denotes the greatest integer function, when x is not an integer then find the value of `f prime (x) `
View SolutionIf f(x) is a polynomial of degree `n(gt2)` and `f(x)=f(alpha-x)`,
(where `alpha` is a fixed real number ), then the degree of f'(x) is
View SolutionThe moment when A is at `(0,0)` and B is at `(1,2)`. The derivative `(dy)/(dx)` of line AB is
View SolutionThe moment when A is at `(1,2) and B is at (0,0)`. The derivative `(dx_(B))/(dx_(A))`, is
View SolutionIf `p''(x)` has real roots `alpha,beta,gamma`. Then , `[alpha]+[beta]+[gamma]` is

View SolutionMatch the Column I with Column II and mark the correct option from the given below.

View SolutionMatch the Column I with Column II and mark the correct option from the given below.
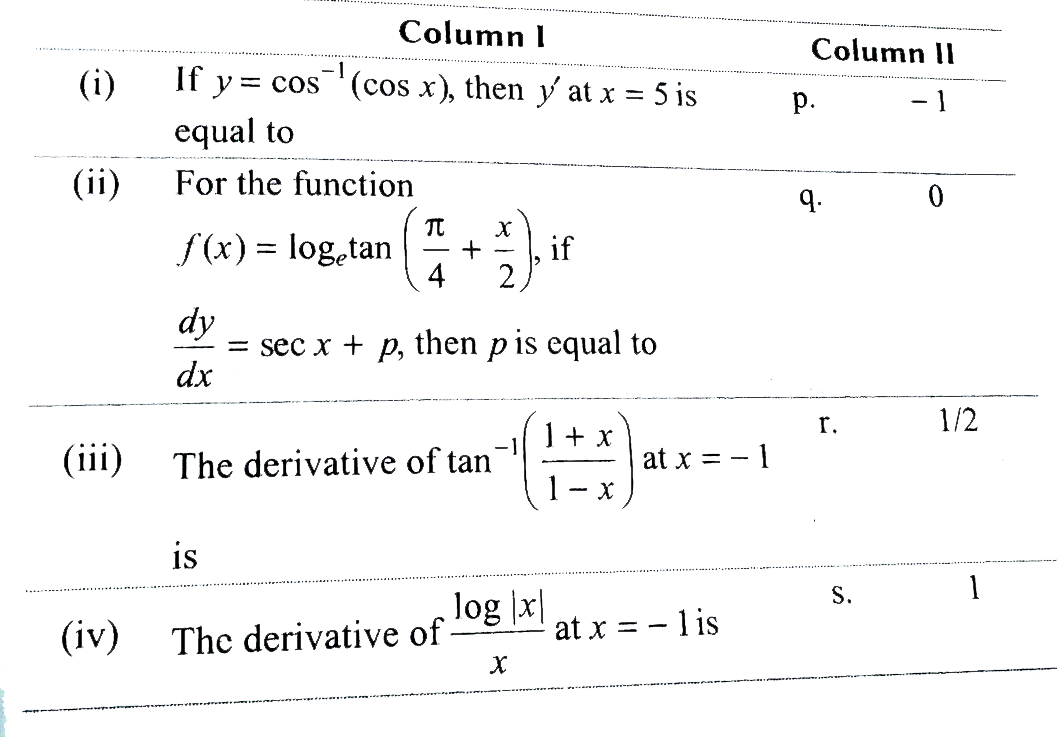
View SolutionIf `y=sqrt((x-sinx)+sqrt(x-sinx)+...),` then `||(dx)/(dy)|_(x=(pi)/(2))^(2)-2pi|=............`
View SolutionLet `f(x) =int_(-2)^xe^((1+t)^2)dt` and `g(x) = f(h(x))`,where h(x) is defined for all `x in R`. If `g'(2) = e^4` and `h' (2)=1` then absolute value of sum of all possible values of h(2), is
View SolutionIf `f(x)=cos{(pi)/(2)[x]-x^(3)},1 lt x lt 2and[x]=` the greatest integer `lex`, then find `f'(root(3)((pi)/(2)))`
View SolutionIf `u=f(x^3),v=g(x^2),f^(prime)(x)=cosx ,a n dg^(prime)(x)=sinx ,t h e n(d u)/(d v)` is `3/2xcosx^3cos e cx^2` `2/3sinx^3secx^2` `tanx` (d) none of these
View Solution`f:RrarrR,f(x)=x^(3)+x^(2)f'(1)+xf''(2)+f'''(3)" for all "x in R.`
The value of f(1) is
View SolutionLet `f(x)=x^2+xg^2(1)+g^''(2) and g(x)=f(1).x^2+xf'(x)+f''(x),` then find `f(x) and g(x).`
View SolutionIf `y=(sinx)/(1+(cosx)/(1+(sinx)/(1+(cosx)/(1+\ ddotto\ oo))))` , prove that `(dy)/(dx)=((1+y)cosx+ysinx)/(1+2y+cosx-sinx)`
View SolutionIf `3sqrt(3sqrt(x-(1)/(3sqrt(x))=2 , then 3sqrt(x)((1)/(3sqrt(x)))`=___________
View SolutionIf `y=tan^(-1)(1/(x^2+x+1))+tan^(-1)(1/(x^2+3x+3))+tan^(-1)(1/(x^2+5x+7))+.....` to nterms, show that `(dy)/(dx)=1/((x+n)^2+1)-1/(x^2+1)`
View Solutionif `f(theta)= Costheta_1.Cos theta_2. cos theta_3….. Cos theta_n, ` show that `{ tan theta_1+ tan theta_2+ tan theta_3+…..+ tan theta_n } = - {(f' (theta))/(f(theta))},`
View SolutionFind the sum of `sinx+3sin3x+5sin5x+...........+(2k-1)sin(2k-1)x.`
View Solution
Examples questions and complete solutions for chapter DIFFERENTIATION of ARIHANT MATHS (HINGLISH) of Class 12
All Exercises from ARIHANT MATHS Class 12 MATHS DIFFERENTIATION
- Examples
- Exercise (More Than One Correct Option Type Questions)
- Exercise (Passage Based Questions)
- Exercise (Questions Asked In Previous 13 Years Exam)
- Exercise (Statement I And Ii Type Questions)
- Exercise (Subjective Type Questions)
- Exercise For Session 1
- Exercise For Session 10
- Exercise For Session 2
- Exercise For Session 3
- Exercise For Session 4
- Exercise For Session 5
- Exercise For Session 6
- Exercise For Session 7
- Exercise For Session 8
- Exercise For Session 9