Complete Solutions to PRACTICE EXERCISE of chapter PROBABILITY of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROBABILITY |
| BITSAT GUIDE | PRACTICE EXERCISE |
| Number Of Questions | 73 |
| Academic Year | 2023-24 |
A single letter is selected at random from the word "PROBABILITY" . The probability that it is a vowel is
View SolutionA five-digit number is formed by the digit 1, 2, 3, 4, 5 without repetition. Find the probability that the number formed is divisible by 4.
View SolutionThree identical dice are rolled. The probability that same number appears on them, is
View SolutionA determinant is chosen at random from the set of all determinants of order two with elements 0 or 1 only. Probability that the determinant chosen has positive value is
View SolutionFrom a well shufflied pacl of 52 playing cards , four cards are accidently dropped . Find the probability that one card is missing from each suit .
View SolutionIf three distinct number are chosen randomly from the first 100 natural numbers, then the probability that all three of them are divisible by both 2 and 3 is `4//25` b. `4//35` c. `4//33` d. `4//1155`
View SolutionA four digit numbers is formed with the digits from 1, 2, 3, 4, 5 without repetition. The probability that it is divisible by 3, is
View SolutionA and B stand in a ring along with 10 other persons. If the arrangement is at random, then the probability that there are exactly 3 persons between A and B, is
View SolutionOne function is selected from all the functions `F: S to S,` where `S = {1,2,3,4,5,6}.` the probability that it is onto function, is
View SolutionThere are 2 teams with n persons in each. The probability of selecting 2 persons from one team and 1 person from the other team is 6/7, then n is equal to
View Solution`x_(1) , x_(2) , x_(3)`… . `x_(50)` are fifty real numbers such that `x_(r) lt x_(r + 1)` for r = 1 ,2 , 3 …….. , 49 . Five numbers out of these are picked up at random . The probability that the five numbers have `x_(20)` as the middle number is :
View SolutionTwo numbers are selected randomly from the set `S={1,2,3,4,5,6}` without replacement one by one. The probability that minimum of the two numbers is less than 4 is `1//15` b. `14//15` c. `1//5` d. `4//5`
View SolutionIf the events A and B are mutually exclusive events such that P(A) = `(3x + 1)/(3)` and P(B) = `(1 - x)/(4)`, then the set of possible real values of x lies in the interval
View SolutionAn urn contains nine balls of which three are red, four are blue and two are green. Three balls are drawn at random without replacement from the urn. The probability that the three balls have different colour, is
View SolutionThree houses are available in a locality. Three persons apply for the houses. Each applies for one house without consulting others. The probability that all the three apply for the same house is
View SolutionIf a number of two digits is formed with the digits 2, 3, 5, 7, 9 without repetition of digits, then the probability that the number formed 35, is
View SolutionIf the papers of 4 students can be checked by anyone of the 7 teachers, then the probability that all the 4 papers are checked by exactly 2 teachers, is equal to
View SolutionIf the word ‘UNIVERSITY’ is arranged randomly, then the probability that both ‘I’ are not together, is
View SolutionIf 6 objects are distributed at random among 6 persons, then the probability that atleast one person does not get any object, is
View SolutionThe probability of not getting a sum of 7 in a single throw with a pair of dice, is
View SolutionThe events `E _(1) and E _(2)` have probabilities `0.25 and 0. 50` respectively. If the probability that both `E _(1) and E _(2)` occur simultaneous is `0. 14.` Then the probability that neither `E _(1)` nor `E _(2)` occurs is
View SolutionFour persons are selected at random from a group of 3 men, 2 women and 4 children. What is the chance that exactly two of them are children?
View SolutionA coin is tossed 3 times by 2 persons. The prbability that both get equal number of heads, is
View SolutionA bag contains 30 balls numbered from 1 to 30. One ball is drawn at random. Find the probability that the number of the ball drawn will be a multiple of 3 or 7.
View SolutionThe probability that a leap year selected ar random contains either 53 sundays or 53 mondays, is
View SolutionFor the three events `"A","B","andC","P"("e x a c t l yo n eo ft h ee v e n t sAorBo c c u r s")="P"("e x a c t l yo n eo ft h ee v e n t sBorCo c c u r s")="P"("e x a c t l yo n eo ft h ee v e n t sCandAo c c u r s")="p"` and`"P"("a l lt h et h r e ee v e n t so c c u rs i m u l t a n e o u s l y")="p"^2,"w h e r e"0<"p"<1//2.` Then, find the probability of occurrence of at least one of the three events `A ,B ,a n dCdot`
View SolutionA, B, C are avents such that
`P _(r) (A) = 0.3 , P _(r) (B)= 0. 4, P _(r) (C) = 0.8`
`P _(r) (AB) = 0. 08 , P _(r) (AC) = 0. 28 and P _(r) (ABC) = 0.09. If P _(r) (A uu B uuC) ge 0. 75,` then `P _(r) (BC)` lies in the interval
View SolutionIf A and B are two events, then the probability that exactly one of them occurs, is given by
View SolutionThe probability that in the toss of two dice we obtain an even sum or a sum less than 5 is
View SolutionA number is chosen at random among the first 120 natural numbers. The probability of the number chosen being a multiple of 5 or 15 is
View SolutionFor any two events A and B, if `P (A uu B) = P ( A nn B, ` then
View SolutionFor any two independent events `E _(1) and E _(2), P { ( E _(1) uu E _(2)) nn (bar E _(1) nn bar E _(2)) }` is
View SolutionFive horses are in race. Mr. X selected two of horses at random and bets on them. The probability that Mr. X selected the winning horse is
View Solution. A bag contains 3 red and 3 white balls. Two balls are drawn one-by-one. The probability that they are of different colours, is
View SolutionLet X be a set containing n elements. Two subsets A and B of X are chosen at random, the probability that `AuuB=X` is
View SolutionIn a hurdle race, a runner has probability `p` of jumping over a specific hurdle. Given that in `5` trials, the runner succeeded `3` times, the conditional probabilit that the runner had succeeded in the first trial is
View SolutionA committee of 4 students is selected at random from a grourp consisting of 8 boys and 4 girls. Given that there is at least one girl in the committee, calculate the probability that there are exactly 2 girls in the committee.
View SolutionIf one Indian and four American men and their wives are to be seated randomly around a circular table. Then, the conditional probability that the Indian man is seated adjacent to his wife given that each American man is seated adjacent to his wife, is
View SolutionIt is given that the events A and B are such that `P(A)=(1)/(4),P((A)/(B))=(1)/(2) and P((B)/(A))=(2)/(3)`. Then `P(B)` is
View SolutionIf `P (A) = (2)/(5), P(B) = (3)/(10) and P (A nnB) = (1)/(5),` then `P ((A')/(B')) . P ((B')/(A'))` is equal to
View SolutionIf A and B are two events such that `P (A) = (1)/(2) P (B) = (1)/(3), P ((A)/(B))= (1)/(4), ` then `P (A' nn B')` is equal to
View SolutionIf `P (A) = (3)/(10) , P (B) = (2)/(5) and P ( A uuB) = (3)/(5),` then `P ((B)/(A)) + P ((A)/(B))` is equal to
View SolutionIf two events A and B are such that `0 lt P (A) , P (B) lt 1` then `P ((A)/(B)) + P ((bar A)/(barB))` is equal to
View SolutionA purse contains 4 copper coins, 3 silver coins, the second purse contains 6 copper coins and 2 silver coins. A coin is taken out of any purse, the probability that it is a copper coin is
View SolutionBag A contains 2 white and 3 red balls and bag B contains 4 white and 5 red balls. One ball is drawn at random from one of the bags and is found to be red. The probability that it was drawn from the bag B is
View SolutionThe chance of defective screws in three boxes `A ,B ,Ca r e1//5,1//6,1//7,` respectively. A box is selected at random and a screw draw in from it at random is found to be defective. Then find the probability that it came from box `Adot`
View SolutionIn three throws of two dice, the probability of throwing doublets not more than twice is
View Solution`Aa n dB` are two independent events. The probability that both `Aa n dB` occur is 1/6 and the probability that neither of them occurs is 1/3. Find the probability of the occurrence of `Adot`
View SolutionThe probability that a 50 year old man will be alive at 60 is 0.83 and the probability that a 45 year old woman will be alive at 55 is 0.87. The probability that atleast one of the will be alive ten years hence, is
View SolutionThe probability that any of the men ` A_(1), A _(2), A _(3), A _(4)` is alive after 95 years of age is `1/2.` The probability that `A _(1)` will die at the age of 95 and will be the first to die is
View SolutionA man throws a fair coin number of times and gets 2 points for each head and 1 point for each tail the probability that he gets exactly 6 points is `(21)/(32)` (2) `(13)/(64)` (3) `(43)/(64)` (4) `(23)/(32)`
View SolutionThere are 5 bails numbered 1 to 5 and 5 boxes numbered 1 to 5. The balls are kept in the boxes one in each box. The probability that exactly 2 balls are kept in the corresponding numbered boxes and the remaining 3 bails in the wrong boxes, is
View SolutionA draws two cards at random from a pack of 52 cards. After returning them to the pack and shuffling it, B draws two cards at random. The probability that their draws contain exactly one common card is
View SolutionA, B, C try to hit a target simultaneously but independently. Their respective probabilities of hitting the target are `3/4,1/2,5/8`. The probability that target is hit by A or B but not by C is
View SolutionLet A and B be two events such that `P bar((A uu B))= (1)/(6) , P (A nn B) = (1)/(4) and P (barA) = (1)/(4)` where `bar A` stands for complement of event A. Then, events A and B are
View SolutionA bag contains 17 tickets numbered 1 to 17. A ticket is drawn and replaced, then one more ticket is drawn and replaced. Probability that first number drawn is even and second is odd, is
View SolutionThree letters are to be sent to different persons and addresses on the three envelopes are also written. Without looking at the addresses, the probability that the letters go into the right envelope is equal to
View SolutionA fair coin is tossed repeatedly. If tail appears on first four tosses, then the probability of head appearing on fifth toss is equal to
View SolutionA die is thrown and a card is selected at random from a deck of 52 playing cards. The probability of getting an even number on the die and a spade card is
View SolutionIf A and B are two independent events such that `P (A) = (7)/(10) , P (barB) = alpha and P (A uu B) = (4)/(5),` then the value of `alpha` is
View SolutionIn a throw of a dice the probability of getting one in even number of throw is
View Solution. Two aeroplanes I and II, bomb a target in succession. The probabilities of I and II scoring a hit correctly are 0.3 and 0.2, respectively. The second plane will bomb only, if the first misses the target. The probability that the target is hit by the second plane, is
View SolutionA discrete random variable X has the following probability distribution
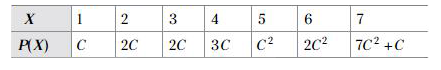
The value of C and the mean of the distribution are
View SolutionA random variable X has the probability distribution
For the events E = {X is a prime number} abd `F={Xlt4},P(EcupF)` is

View SolutionIf `10%` of bolts produced by a machine are defective, then the probability that out of a sample selected at random, of 7 bolts, not more than 1 is defective, is
View SolutionIf the mean and variance of a binomial variate X are α and 1 respectively, then the probability that X takes a value greater than one equal to
View SolutionAn unbiased coin is tossed n times. Let X denote the number of times head occurs. If `P(X=4), P(X=5) and P(X=6)` are in A.P, then the value of n can be
View SolutionThe mean and varaince of binomial distribution are 4 and 3, respectively. Then, the probability of getting exactly six success in this distribution is
View SolutionA multuple choice examination has 5 questions. Each questions has three alternative answers of which exactly one is correct. The probability that a student will get 4 or more correct answers just be guessing, is
View SolutionA die is rolled 20 times . Getting a number greater than 4 is a success.
Find the mean and variance of the number of successes .
View SolutionA fair coin is tossed a fixed number of times. If the probability of getting seven heads is equal to that of getting nine heads, the probability of getting two heads, is
View SolutionA die is thrown 100 times, getting an even number is considered a success. The variance of the number of successes is
View SolutionA die is thrown 100 times, getting an even number is considered a success. The variance of the number of successes is
View Solution
PRACTICE EXERCISE questions and complete solutions for chapter PROBABILITY of BITSAT GUIDE of Class 12