Complete Solutions to Exercise (Matrix) of chapter STRAIGHT LINES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | STRAIGHT LINES |
| CENGAGE NEW | Exercise (Matrix) |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
Consider the lines represented by equation `(x^(2) + xy -x) xx (x-y) =0` forming a triangle. Then match the following lists:
 03:50View Solution
03:50View SolutionConsider the triangle formed by the lines
y+3x+2=0, 3y-2x-5=0, 4y+x-14=0
Match the following lists:
 02:06View Solution
02:06View SolutionA straight line with negative slope passing the point (1, 4) meets the coordinate axes at A and B. The minimum value of OA + OB =
05:21View SolutionIf the point P is symmetric to the point Q(4,-1) with respect to the bisector of the first quadrant then the length of PQ is
02:04View SolutionThe point `A(1,2), B(2,-3), C(-1,-5)` and `D(-2, 4)` in order are the vertices of
02:48View SolutionDifferentiate `y = sin(x^2 + 3)`.
01:45View SolutionConsider the lines given by
`L_(1):x+3y-5=0`
`L_(2):3x-ky-1=0`
`L_(3):5x+2y-12=0`
Match the following lists.
 04:42View Solution
04:42View SolutionConsider a `DeltaABC` in which sides AB and AC are perpendicular to x-y-4=0 and 2x-y-5=0, repectively. Vertex A is (-2, 3) and the circumcenter of `DeltaABC` is (3/2, 5/2).
The equation of the line in List I is of the form ax+by+c=0, where `a,b,c in I.` Match it with the corresponding value of c in list II and then choose the correct code.
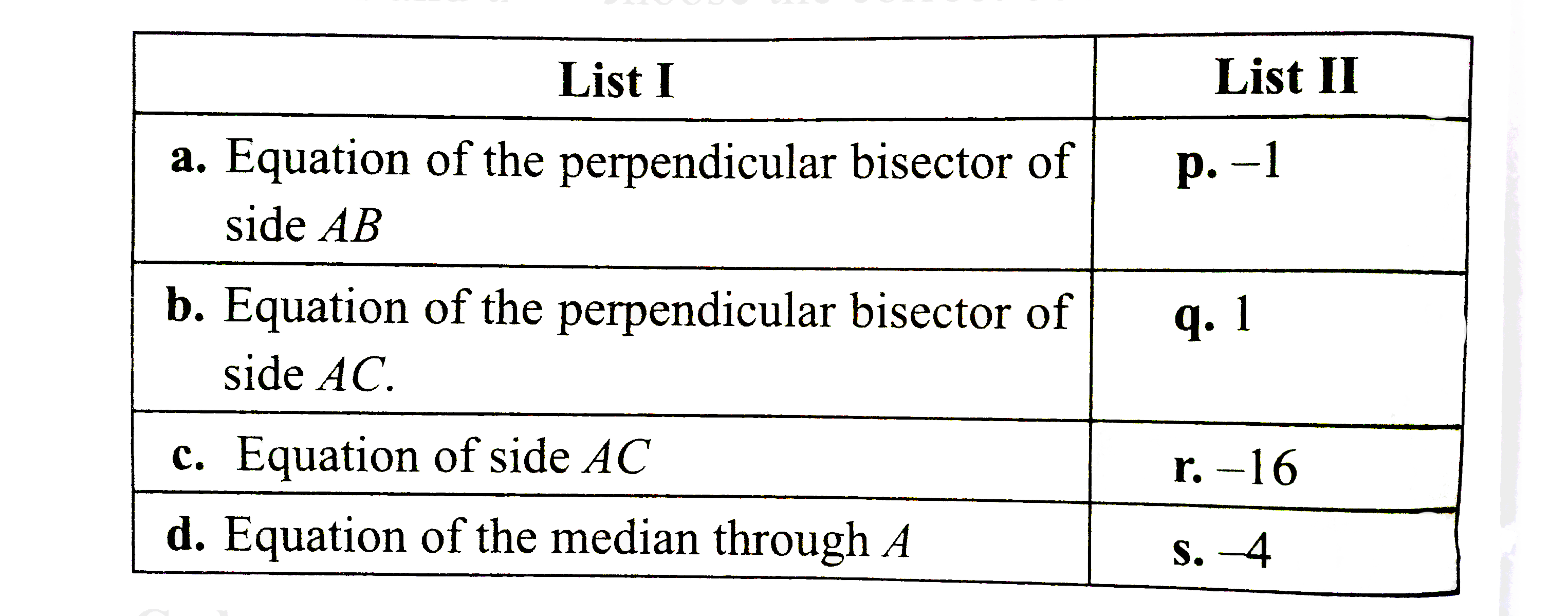
Codes :
`{:(a, b, c, d),(r, s, p, q),(s, r, q, p),(q, p, s, r),(r, p, s, q):}`09:09View Solution
Exercise (Matrix) questions and complete solutions for chapter STRAIGHT LINES of CENGAGE of Class 12
