Complete Solutions to Linked -comprehension type of chapter IIT QUESTIONS 4 of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | IIT QUESTIONS 4 |
| D MUKHERJEE | Linked -comprehension type |
| Number Of Questions | 5 |
| Academic Year | 2023-24 |
When a particle of mass m moves on the x-axis in a potential of the form `V(x) =kx^(2)` it performs simple harmonic motion. The correspondubing time period is proprtional to `sqrtm/h`, as can be seen easily using dimensional analusis. However, the motion of a particle can be periodic even when its potential energy increases on both sides of `x=0` in a way different from `kx^(2)` and its total energy is such that the particle does not escape toin finity. Consider a particle of mass m moving on the x-axis. Its potential energy is `V(x)=ax^(4)(agt0)` for |x| neat the origin and becomes a constant equal to `V_(0)` for |x|impliesX_(0)` (see figure).
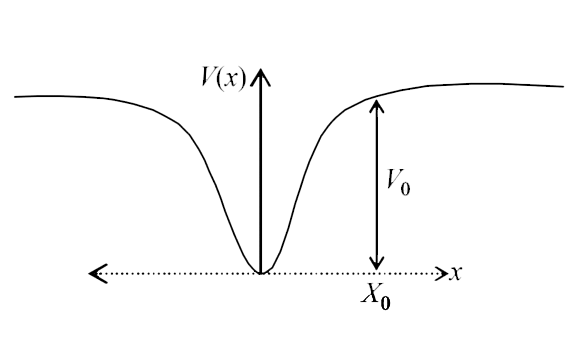
If total energy of the particle is E, it will perform perildic motion only if.
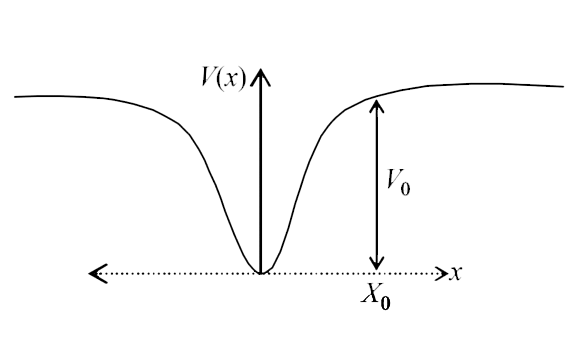
View SolutionWhen a particle of mass m moves on the x-axis in a potential of the form `V(x) =kx^(2)` it performs simple harmonic motion. The correspondubing time period is proprtional to `sqrtm/h`, as can be seen easily using dimensional analusis. However, the motion of a particle can be periodic even when its potential energy increases on both sides of `x=0` in a way different from `kx^(2)` and its total energy is such that the particle does not escape toin finity. Consider a particle of mass m moving on the x-axis. Its potential energy is `V(x)=ax^(4)(agt0)` for |x| neat the origin and becomes a constant equal to `V_(0)` for |x|impliesX_(0)` (see figure).
 .
.
For periodic motion of small amplitude A,the time period (T) of thes particle is proportional to.
View SolutionWhen a particle of mass m moves on the x-axis in a potential of the form `V(x) =kx^(2)` it performs simple harmonic motion. The correspondubing time period is proprtional to `sqrtm/h`, as can be seen easily using dimensional analusis. However, the motion of a particle can be periodic even when its potential energy increases on both sides of `x=0` in a way different from `kx^(2)` and its total energy is such that the particle does not escape toin finity. Consider a particle of mass m moving on the x-axis. Its potential energy is `V(x)=ax^(4)(agt0)` for |x| neat the origin and becomes a constant equal to `V_(0)` for |x|impliesX_(0)` (see figure).
 .
.
The acceleration of this partile for `|x|gtX_(0)` is
(a) proprtional to `V_(0)`
(b) proportional to.
View SolutionElectrical resistance of certain materials, known as superconductors, changes abruptly from a nonzero value of zero as their temperature is lowered below a critical temperature `T_(C) (0)`. An interesting property of super conductors is that their critical temperature becomes smaller than `T_(C) (0)` if they are placed in a magnetic field, i.e., the critical temperature `T_(C) (B)` is a function of the magnetic field strength B. The dependence of `T_(C) (B)` on B is shown in the figure.
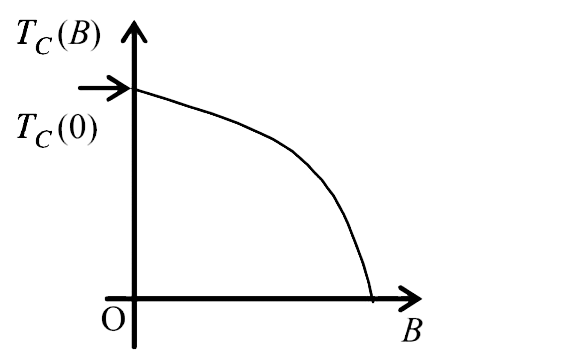 .
.
In the graphs below, the resistance R of a superconductor is shown as a function of its temperature T for two different magnetic fields `B_1`(solid line) and `B_2` (dashed line). If `B_2` is larget than `B_1` which of the following graphs shows the correct variation of R with T in these fields?
View SolutionElectrical resistance of certain materials, known as superconductors, changes abruptly from a nonzero value of zero as their temperature is lowered below a critical temperature `T_(C) (0)`. An interesting property of super conductors is that their critical temperature becomes smaller than `T_(C) (0)` if they are placed in a magnetic field, i.e., the critical temperature `T_(C) (B)` is a function of the magnetic field strength B. The dependence of `T_(C) (B)` on B is shown in the figure.
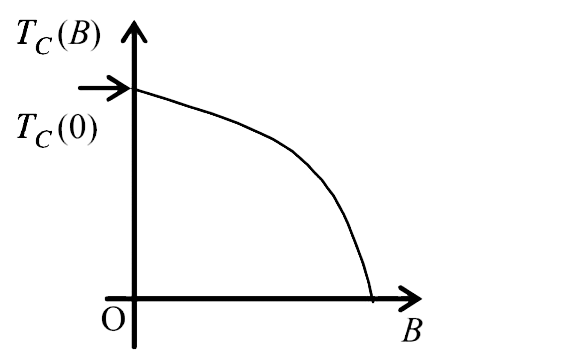 .
.
A superconductor has `T_(C) (0) = 100 K`. When a magnetic field of 7.5 Tesla is applied , its `T_(C)` decreases to 75 K. For this material one can difinitely say that when
View Solution
Linked -comprehension type questions and complete solutions for chapter IIT QUESTIONS 4 of D MUKHERJEE PHYSICS (HINGLISH) of Class 12