Complete Solutions to Physics of chapter GRAVITATION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | GRAVITATION |
| DISHA | Physics |
| Number Of Questions | 55 |
| Academic Year | 2023-24 |
Mass `M` is split into two parts `m` and `(M-m)`, which are then separated by a certain distance. What is the ratio of `(m//M)` which maximises the gravitational force between the parts ?
View SolutionWhat would be the angular speed of earth, so that bodies lying on equator may experience weightlessness ?
( g = `10m//s^(2)` and radius of earth = 6400 km)
View SolutionDetermine the speed with which the earth would have to rotate on its axis , so that a person on the equator would weigh `(3)/(5)` th as much as the person. Take `R = 6400 km`.
View SolutionA planet whose size is the same and mass `4` times as that of Earth, find the amount of energy needed to lift a `2 kg` mass vertically upwards through `2m` distance on the planet. The value of `g` on the surface of Earth is `10 ms^(-2)`.
View SolutionTwo bodies of mass `10^(2)` kg and `10^(3)` kg are lying 1 m apart .
The gravitational potential at the mid-point of the line joining them is
View SolutionA particle of mass 'm' is raised to a height `h = R` from the surface of earth. Find increase in potential energy. `R =` radius of earth. `g =` acceleration due to gravity on the surface of earth.
View SolutionFour particles, each of mass M and equidistant from each other, move along a circle of radius R under the action of their mutual gravitational attraction. The speed of each particle is:
View SolutionThree particles, each of mass m, are situated at the vertices of equilateral triangle of side length a. The only forces. It is desired that each particle moves in a circle while maintaining the original mutual speration a. Find the intial velocity that should be given to each particle and also the time period of the circular motion.
View SolutionWhat will be acceleration due to gravity on the surface of the moon if its radius were `(1//4)^(th)` the radius of earth and its mass `(1//80)^(th)` the mass of earth? What will be the escape velocity on the surface of moon if it is `11.2 km//s` on the surface of the earth? (given that `g=9.8 m//s^(2)`)
View SolutionThe change in the value of `g` at a height `h` above the surface of the earth is the same as at a depth d below the surface of earth. When both `d` and `h` are much smaller than the radius of earth, then which one of the following is correct?
View SolutionAt what height above the earth's surface the acceleration due to gravity will be 1/9 th of its value at the earth’s surface? Radius of earth is 6400 km.
View SolutionIf the radius of the earth were to shrink by one percent its mass remaining the same, the acceleration due to greavity on the earth's surface would
View SolutionAt what height above the earth’s surface does the force of gravity decrease by `10%` ? Assume radius of earth to be 6370 km.
View SolutionA particle hanging from a spring stretches it by 1 cm at earth's surface. How much will the same particle stretch the spring at a place 800 km above the earth's surface/ Radius of the earth=6400 km.
View SolutionThe percentage change in the acceleration of the earth towards the Sun from a total eclipse of the Sun to the point where the Moon is on a side of earth directly opposite to the Sun is
View SolutionThe radius and mass of Earth are `R` and `M`. The acceleration due to gravity at its surface is `g`. Calculate the work required in raising a body of mass `m` to a height `h` from the surface of earth.
View SolutionThe masses and radii of the Earth and the Moon are `M_1, R_1 and M_2,R_2` respectively. Their centres are at a distance d apart. The minimum speed with which a particel of mass m should be projected from a point midway between the two centres so as to escape to infinity is ........
View SolutionWith what velocity must a body be thrown upward form the surface of the earth so that it reaches a height of 10 `R_(e)` ?
earth's mass `M_(e) = 6 xx 10^(24)` kg , radius `R_(e) = 6.4 xx 10^(6)`m and `G = 6.67 xx 10^(-11) N-m^(2)//kg^(2)`.
View SolutionTwo concentric shells of mass `m_(1)` and `m_(2)` are situated as shown. Find the force on a particle of mass `m` when the particles is located at `(a) r=r_(1),(b)r=r_(2),(c)r=r_(3)`. The distance `r` is measured from the centre of the shell.
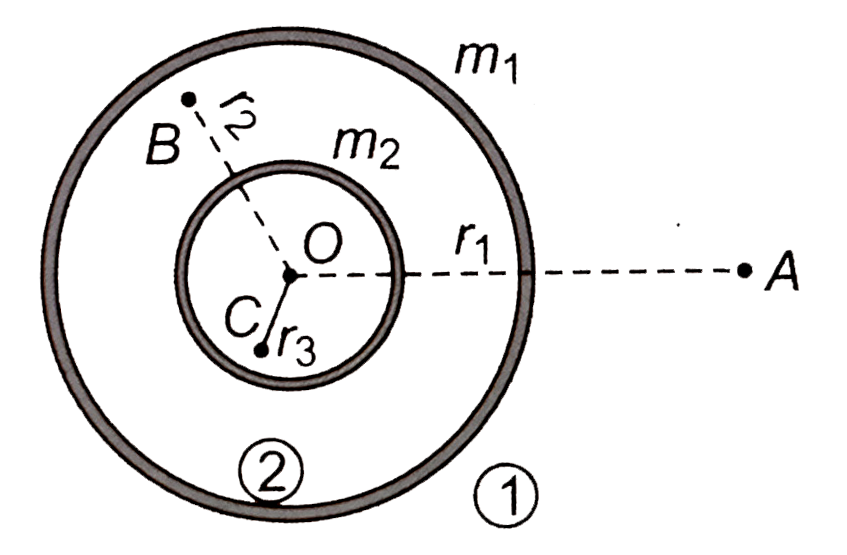
View SolutionWhat is the mass of the planet that has a satellite whose time period is `T` and orbital radius is `r`?
View SolutionThe gravitational force between two point masses `m_(1)` and `m_(2)` at separation r is given by `F = k(m_(1) m_(2))/(r^(2))`
The constant k doesn't
View SolutionWhich of the following statements about the gravitional constant are false ?
View SolutionSpot the correct statements :
The acceleration due to gravity 'g' decreases if
View SolutionThe orbit of Pluto is much more eccentric than the orbits of the other planets. That is, instead of being nearly circular, the orbit is noticeably elliptical. The point in the orbit nearest to the sun is called the perihelion and the point farthest from the sun is called the aphelion.

At perihelion, the gravitational potential energy of Pluto in its orbit has
View SolutionThe orbit of Pluto is much more eccentric than the orbits of the other planets. That is, instead of being nearly circular, the orbit is noticeably elliptical. The point in the orbit nearest to the sun is called the perihelion and the point farthest from the sun is called the aphelion.

At perihelion, the mechanical energy of Pluto’s orbit has
View SolutionThe orbit of Pluto is much more eccentric than the orbits of the other planets. That is, instead of being nearly circular, the orbit is noticeably elliptical. The point in the orbit nearest to the sun is called the perihelion and the point farthest from the sun is called the aphelion.

As Pluto moves from the perihelion to the aphelion, the work done by gravitational pull of Sun on Pluto is
View SolutionStatement -1: Gravitational force between two particles is negligibly small compared to the electrical force.
Statement-2 :The electrical force is experienced by charged particles only.
View SolutionStatement-1 :The universal gravitational constant is same as acceleration due to gravity.
Statement-2 :Gravitional constant and acceleration due to gravity have different dimensional formula
View SolutionAssertion: There is no effect of rotation of a earth on acceleration due to gravity at poles.
Reason : Rotation of earth is about polar axis.
View SolutionA body of mass `100kg` falls on the earth from infinity. Its kinetic energy on reaching the earth is `6.27 xx 10^(n)J`. What is the value of `n`? Given, radius of earth is `6400 km` and `g = 9.8 m//s^(2)`. Air friction is neglected.
View SolutionAn artificial satellite of the earth is to be established in the equatorial plane of the earth and to an observer at the equator it is required that the satellite will move eastward, completing one round trip per day. The distance of the satellite from the centre of the earth will be - ( The mass of the earth is `6.00 xx 10^(24)` kg and its angular velocity = `7. 30 xx 10^(-5) "rad"//"sec".`)
View SolutionTwo satellites `S_(1)` and `S_(2)` revolve round a planet in the same direction in circular orbits. Their periods of revolutions are 1 hour and 8 hour respectively. The radius of `S_(1)` is 104 km. The velocity of `S_(2)` with respect to `S_(1)` will be -
View SolutionIn the above example the angular velocity of `S_(2)` as actually observed by an astronaut in `S_(1)` is -
View SolutionThe moon revolves round the earth `13` times in one year. If the ratio of sun-earth distance to earth-moon distance is `392`, then the ratio of masses of sun and earth will be
View SolutionTwo planets of radii in the ratio `2 : 3` are made from the materials of density in the ratio `3 : 2`. Then the ratio of acceleration due to gravity `g_(1)//g_(2)` at the surface of two planets will be
View SolutionA satellite of mass m is revolving in a circular orbit of radius r. The relation between the angular momentum J of satellite and mass m of earth will be -
View SolutionA spaceship is launched into a circular orbit close to the earth's surface . What additional velocity has now to be imparted to the spaceship in the orbit to overcome the gravitational pull. Radius of earth `= 6400 km`, `g = 9.8m//s^(2)`.
View SolutionThe ratio of the radius of the earth to that of moon is `10`. The ratio of acceleration due to gravity on the earth and on the moon is `6`. What is the ratio (in intergral value) of the escape velocity from the earth's surface to that from the moon?
View SolutionAcceleration due to gravity on a planet is 10 times the value on the earth. Escape velocity for the planet and the earth are `V_(p)` and `V_(e)` respectively. Assuming that the radii of the planet and the earth are the same, then -
View SolutionThe Jupiter’s period of revolution round the Sun is 12 times that of the Earth. Assuming the planetary orbits are circular, how many times the distance between the Jupiter and Sun exceeds that between the Earth and the sun.
View SolutionThe mean distance of Mars from the sun in `1.524` times that of the Earth from the sun. Find the number of years requires for Mars make one revolution about the Sun.
View SolutionThe semi-major axes of the orbits of mercury and mars are respectively 0.387 and 1.524 in astronomical unit. If the period of Mercury is 0.241 year, what is the period of Mars.
View SolutionIf a graph is plotted between `T^(2) and r^(3)` for a planet, then its slope will be be (where `M_(S)` is the mass of the sun)
View SolutionThe masses and radii of the Earth and the Moon are `M_1, R_1 and M_2,R_2` respectively. Their centres are at a distance d apart. The minimum speed with which a particel of mass m should be projected from a point midway between the two centres so as to escape to infinity is ........
View SolutionA satellite has to revolve round the earth in a circular orbit of radius `8 xx 10^(3)` km. The velocity of projection of the satellite in this orbit will be -
View SolutionIf the satellite is stopped suddenly in its orbit which is at a distnace = radius of earth from earth’s surface and allowed to fall freely into the earth, the speed with which it hits the surface of earth will be -
View SolutionA projectile is fired vertically upwards from the surface of the earth with a velocity `Kv_(e)`, where `v_(e)` is the escape velocity and `Klt1`.If `R` is the radius of the earth, the maximum height to which it will rise measured from the centre of the earth will be (neglect air resistance)
View SolutionA satellite is revolving in an orbit close to the earth’s surface. Taking the radius of the earth as `6.4 x× 10^(6)` metre, the value of the orbital speed and the period of revolution of the satellite will respectively be ( g = `9.8 "meter"//sec^(2)`)
View SolutionIf the period of revolution of an artificial satellite just above the earth be T second and the density of earth be `rho , kg//m^(3)` then
(G =`6.67 xx 10^(-11) m^(3)//kg . "second"^(2)`)
View SolutionTwo satellites `A` and `B` of equal mass move in the equatorial plane of the earth, close to earth's surface. Satellite `A` moves in the same direction as the of the rotation of the earth while satellite `B` moves in the opposite direction. Calclate the ratio of the kinetic energy of `B` of that of `A` in the reference frame fixed to the earth `(g=9.8ms^(-2)` and radius of the earth `=6.37xx10^(6)km)`
View SolutionGas escapes from the surface of a planet because it acquires an escape velocity. The escape velocity will depend on which of the following factors:
(1) Mass of the planet
(2) Radius of the planet
(3) Mass of the particle escaping
(4) Temperature of the planet
View Solution`v_(e) "and" v_(p)` denotes the escape velocity from the earth and another planet having twice the radius and the same mean density as the earth. Then which of the following is (are) wrong ?
View SolutionSelect the wrong statements from the following
(1) The orbital velocity of a statellite increases with the radius of the orbit
(2) Escape velocity of a particle from the surface of the earth depends on the speed with which it is fired
(3) The time period of a satellight does not depend on the radius of the orbit
(4) The orbital velocity is inversely proportional to the square root of the radius of the orbit
View SolutionAssertion: The speed of revolution of an artificial satellite revoving very near the earth is `8kms^(-1)`.
Reason: Orbital velocity of a satellite, becomes independent of height near earth.
View SolutionAssertion: if an earth satellite moves to a lower orbit, there is some dissipation of energy but the satellite speed increases.
Reason: The speed of satellite is a constant quantity.
View Solution
Physics questions and complete solutions for chapter GRAVITATION of DISHA PHYSICS (HINGLISH) of Class 12