Complete Solutions to Exercise of chapter STRUCTURE OF ATOM of Class 12 book with complete answers and questions
| Class 12 | CHEMISTRY |
| Chapter | STRUCTURE OF ATOM |
| DISHA PUBLICATION | Exercise |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Which of the following statements is false?
View SolutionIf the shortest wavelength in Lyman series of hydrogen atom is A, then the longest wavelength in Paschen series of `He^(+)` is:
View SolutionThe radius of the second Bohr orbit for hydrogen atom is : (Plank'c const. `h = 6.6262 xx 10^(-34) Js` , mass electron ` = 9.1091 xx 10^(-31)` Kg , charge of electron ` e = 1.60210 xx 10^(-19)` , permittivity of vaccum ` in_(0) = 8.854185 xx 10^(-12) kg^(-1) m^(-3) A^(2))`
View SolutionThe total number of orbitals associated with the principal quantum number 5 is :
View SolutionA stream of electrons from a heated filament was passed between two charged plates kept at a potential difference V esu. If c and m are charge and mass of an electron repectively, then the value of `h//lambda` (where `lambda` is wavelength associated with electron wave) is given by :
View SolutionIf `n = 6`, the correct sequence for filling of electrons will be.
View SolutionAt temperature T , the average kinetic energy of any particles is `(3)/(2)` kT. The de Broglie wavelength follows the order :
View SolutionWhich of the following is the energy of a possible excited state of hydrogen?
View SolutionThe energy of an electron in first Bohr's orbit of H atom is `-13.6eV`. The energy value of electron in the first excited state of `Li^(2+)` is :
View SolutionExcited hydrogen atom emits light in the ultraviolet region at `2.47xx 10^(15)` Hz. With this frequency, the energy of a single photon is:
View SolutionThe de-Broglie wavelength of a particle of mass 6.63 g moving with a velocity of `100 ms^(-1)` is:
View SolutionThe correct set of four quantum numbers for valence electrons of rubidium atom (Z=37) is
View SolutionThe number of neutrons in dipositive zinc ion with mass number `70` is.
View SolutionWhich of the following pairs are isosters ?
View SolutionLet `m_(p)` be the mass of a poton , `M_(1)` the mass of a `_(10)^(20) Ne` nucleus and `M_(2)` the mass of a `_(20)^(40) Ca` nucleus . Then
View SolutionThe ratio of specific charge of a proton and an alpha-particle is :
View SolutionWhich one of the following sets of ions represents the collection of isoelectronic species?
View SolutionThe increasing order (lowest first) for the values of `e//m` (charge//mass) for electron `(e)`, proton `(p)`, neutron `(n)`, and alpha particle `(alpha)` is
View SolutionWhich of the following pairs of nucleides are isodiaphers ?
View SolutionThe discovery of neutron becomes very late because.
View SolutionOf the following sets ,which one does not contain isoeletronic species ?
View SolutionThe pair `NH_(3)` and `BH_(3)` is isoelectronic with
View SolutionThe energy of an electron in the nth Bohr orbit of hydrogen atom is
View SolutionWhich of the following statement does not form part of Bohr's model of the hydrogen atomn?
View SolutionWhen an electron of charge , e and mass , m moves with velocity v around the nuclear charge 'Ze' describing the circular orbit, the potential energy of the electron is
View SolutionBohr's radius for the H-atom (n =1) is approximately 0.53 Ã…. The radius of the first excited state (n=2) is :
View SolutionAccording to Boohr's theory the angular momentum of an electron in 5th orbit is :
View SolutionAn electron from one Bohr stationary orbit can go to next higher orbit:
View SolutionThe potential energy of the electron present in the ground state of ` Li^(2+)` ion is represented by :
View SolutionThe angular speed of electron in the nth orbit of hydrogen atom is
View SolutionThe radius of `1^(st)` Bohr's orbit for hydrogen atom is 'r'. The radius of second Bohr's orbit is
View SolutionEnergy of an electron is givem by `E = - 2.178 xx 10^-18 J ((Z^2)/(n^2))`. Wavelength of light required to excited an electron in an hydrogen atom from level `n = 1` to `n = 2` will be
`(h = 6.62 xx 10^-34 Js` and `c = 3.0 xx 10^8 ms^-1`).
View SolutionThe total energy of the electron revolving round the nucleus is
View SolutionEnergy of an electron in a one-electron system can be calculated as
`E_(n) = (-2.18 xx 10^(-18) Z^(2))/(n^(2))`
Which of the following correctly states the relationship between the n=2 level of `He^(+)` atom (Z=2) and n=2 level of `Li^(2+)` ion (Z=3)?
View SolutionA small particle of mass m move in such a way the potential energy `(U = (1)/(2) m^(2) omega^(2) r^(2))` when a is a constant and r is the distance of the particle from the origin Assuming Bohr's model of quantization of angular momentum and circular orbits , show that radius of the nth allowed orbit is proportional to in
View SolutionThe velocity of an e in excited state of H-atom is `1.093 xx 10^(6)m//s`, what is the circumference of this orbit?
View SolutionMonochromatic radiation of specific wavelength is incident on H-atoms in ground state. H-atoms absorb energy and emit subsequently radiations of six different wavelength. Find wavelength of incident radiations:
View SolutionIf I exciation energy for the H-like (hypothetical) sample is 24 eV, then binding energy in III excited state is :
View SolutionThe energy of seperation of an electron in a hydrogen like atom in excited state is 3.4eV. The de-Broglie wave length (in Å) associtated with the electron is :
View SolutionIf an electron undergoes transition from n = 2 to n =1 in `Li^(2+)` ion , the energy of photon radiated will be best given by
View SolutionThe strength of the photoelectric current depends upon
View SolutionIf `lamda_o and lamda` be the threshold wavelength and wavelength of incident light , the velocity of photoelectron ejected from the metal surface is :
View SolutionThe work function of a photoelectric material is 3.3 eV. The thershold frequency will be equal to
View SolutionZeeman effect refers to the
View SolutionFor any H like system, the ratio of velocities of electron in I, II & III orbit e.e.,`V_(1):V_(2):V_(3)` will be:
View SolutionThe electron in the hydrogen atom undergoes transition from higher orbitals to orbital of radius 211.6 pm. This transition is associated with:
View SolutionWhat is the potential energy of an electron present in `N-` shell of the `Be^(3+)` ion ?
View SolutionWhat is the frequency of revolution of electron present in `2nd` Bohr's orbit of `H-` atom ?
View SolutionWhat is the ratio of time periods `(T_(1)//T_(2))` in second orbit of hydrogen atom to third orbit of `He^(+)` ion?
View SolutionWhich experimental observation correctly account for the phenomenon ?
View SolutionThe energy of a photon is given as, `Delta E`/atom `= 3.03 xx 10^(-19)J "atom"^(-1)` then, the wavelength `(lambda)` of the photon is
View SolutionThe value of Planck's constant is `6.63 xx 10^(-34)Js`. The velocity of light is `3.0 xx 10^(8)ms^(-1)`. Which value is closest to the wavelength in nanometers of a quantum of light with frequency `8xx10^(15)s^(-1)`?
View SolutionThe uncertainty in the position of an electron `(mass = 9.1 xx 10^-28 g)` moving with a velocity of `3.0 xx 10^4 cm s^-1` accurate up to `0.001 %` will be
(Use `(h)/(4 pi)` in the uncertainty expression, where `h = 6.626 xx 10^-27 erg - s`)
View SolutionWave length associated with electron motion
View SolutionThe de-Broglie wavelength associated with a particle of mass `10^-6 kg` moving with a velocity of `10 ms^-1`, is
View SolutionThe momentum `("in" kg-m//s)` of photon having 6 MeV energy is :
View SolutionWhen electronic transition occurs from higher energy state to lower energy state with energy difference equal to `Delta E` electron volts , the wavelength of the line emitted is approxmately equal to
View SolutionWhich of the following statement concerning concerning probability density `(Psi^(2))` and radial distribution function `(4 pir^(2) Psi)` for a s - orbit of H - like species is correct ?
View SolutionThe angular momentum of d electron is
View SolutionThe value of Planck's constant is `6.63 xx 10^(-34)Js`. The velocity of light is `3.0 xx 10^(8)ms^(-1)`. Which value is closest to the wavelength in nanometers of a quantum of light with frequency `8xx10^(15)s^(-1)`?
View SolutionIf uncertainty in position and momentum are equal then uncertainty in velocity is.
View SolutionWhich of the following statement is worong about photon ?
View SolutionExcited hydrogen atom emits light in the ultraviolet region at `2.47xx 10^(15)` Hz. With this frequency, the energy of a single photon is: `(h = 6.63 xx 10^(-34)Js)`
View SolutionPhotons of minimum energy `496k,J. mol^(-1)` are needed to an atoms. Calculate the lowest frequency of light that will ionize a sodium atom.
View SolutionWhich of the following sets of quantum numbers could represent the last electron added to complete the electron added to complete the electron configuation for a ground state atom of `Br(Z=35)` according to Aufbau principle,
View SolutionSuppose the universe were different than it is and that the electron spin quantum number could only have of a value of `+(1)/(2)` . Assume that the Pauli's exclusion principle still holds and that the value of other quantum numbers were the same, which atomic number could correspond to the "noble gases" in this universe?
View SolutionWhich orbital of the following is lower in energy in a many electron atom?
View SolutionCalculate the minimum and maximum number of electrons which may have magnetic quantum number `m=+1` and spin quantum number `s=-(1)/(2)` in chromium (Cr)
View SolutionThe total number of electrons that can be accommodated in all orbitals having principal quantum number 2 and azimuthal in quantum number 1 is
View SolutionFor which one of the following sets of four quantum numbers, an electron will have the highest energy?
View SolutionThe five d-orbitals are designated as `d_(xy), d_(yz), d_(xz), d_(x^(2) - y^(2))` and `d_(z^(2))` . Choose the correct statement.
View SolutionWhich one of the following set of quantum numbers is not possible for 4p electron?
View SolutionIf the magnetic quantum number of a given atom is represented by -3, then what will be its principal quantum number?
View SolutionFor f-orbital, the values of m are
View SolutionHow many nodes are there in 5f orbitals?
View SolutionIf an electron has spin quantum number of `-(1)/(2) ` and magnetic quantum number of -1 it cannot be present in:
View SolutionThe orbital angular momentum for an electron revolving in an orbit is given by `sqrt(l(l + 1))(h)/(2pi)`. What is the momentum of an s-electron?
View SolutionThe energy of the electron in` Be^(3+)` ion depends on
View SolutionWhat are the component values in terms of `h//2pi` ) of the orbital angular momentum along the Z-direction for a 2p electron?
View SolutionThe total number of orbitals associated with the principal quantum number 5 is :
View SolutionThe total spin and magnetic moment for the atom with atomic number `24` are
View SolutionA principal shell having the highest energy subshell to be 'g'can accomodate electrons to a maximum of .
View SolutionThe energy of the electron in the first orbit of `He^+` is `- 871.6 xx 10^-20 J`. The energy of the electron in the first orbit of hydrogen would be.
View SolutionBased on equation `E = -2.178 xx 10^-18 J((Z^2)/(n^2))`, certain conclusions are written. Which of them is not correct ?
View SolutionThe ionization enthalpy of `He^(+)` ion is `19.60 xx 10^(-18)` J `"atom"^(-1)` The ionization enthalpy of `Li^(+2)` ion will be.
View SolutionAn electron in the ground state of hydrogen was excited to a higher energy level using monochromatic radiations of wave length `(lambda )975 Å` . The longest wave length that appears in the resulting spectrum is due to transition from:
View SolutionArrange the wavelengths (a) of the following emission lines of H-atom in an increasing order.
(1) `n = 3 overset(lambda_(1))to n = 1` (2) `n = 5 overset(lambda_(2))to n = 3`
(3) ` n = 12 overset(lambda_(3))to n = 10` (4) `n = 22 overset(lambda_(4)) to n = 20`
View SolutionWhat transition in `He^(o+)` ion shall have the same wave number as the first line in Balmer series of H atom ?
View SolutionFor a hypothetical hydrogen like atom, the potential energy of the system is given by `U(r)=(-Ke^(2))/(r^(3))`, where r is the distance between the two particles. If Bohr's model of quantization of angular momentum is applicable then velocity of particle is given by:
View SolutionA beam of specific kind of particles of velocity `2.1 xx 10^7 m//s` is scattered by a gold `(Z = 79)` nuclei. Find out specific charge (charge/mass) of this particle if the distance of closest approach is `2.5 xx 10^-14 m`.
View SolutionAn electron in a hydrogen atom in its ground state absorbs 1.5 times as much energy as the minimum required for it to escape from the atom. What is the velocity of the emitted electron?
View SolutionH-atom is exposed to electromagnetic radiation of `lambda=1025.6` Å and excited atom gives out induced radiation. What is the minimum wavelength of the induced radiation?
View SolutionIf the lowest energy X-rays have `lambda=3.055xx10^(-8)` m, estimate the minimum difference in energy between two Bohr's orbits such that an electronic transition would correspond to the emission of an X-ray. Assuming that the electrons in other shells exert no influence, at what Z (minimum) would a transition form the second level to the first result in the emission of an X-ray?
View SolutionBalmer gave an equation for wavelegth of visible region of H-spectrum as `lambda=(Kn^(2))/(n^(2)-4).
Where n= pricipal quantum number of energy level, K=constant in terms of R (Rydberg constant).
The value of K in term of R is :
View SolutionIn hydrogen atomic spectrum , a series limit is found at `12186.3 cm^(-1)`. Then it belong to :
View SolutionIf the average life time of an excited state of hydrogen is of the order of `10^(-8) s`, estimate how many whits an alectron makes when it is in the state `n = 2` and before it suffers a transition to state` n = 1 (Bohrredius a_(0) = 5.3 xx 10^(-11)m)`?
View SolutionConsider the following radial distribution function diagrams. Which of the following has the correct matching of curve and orbital ?
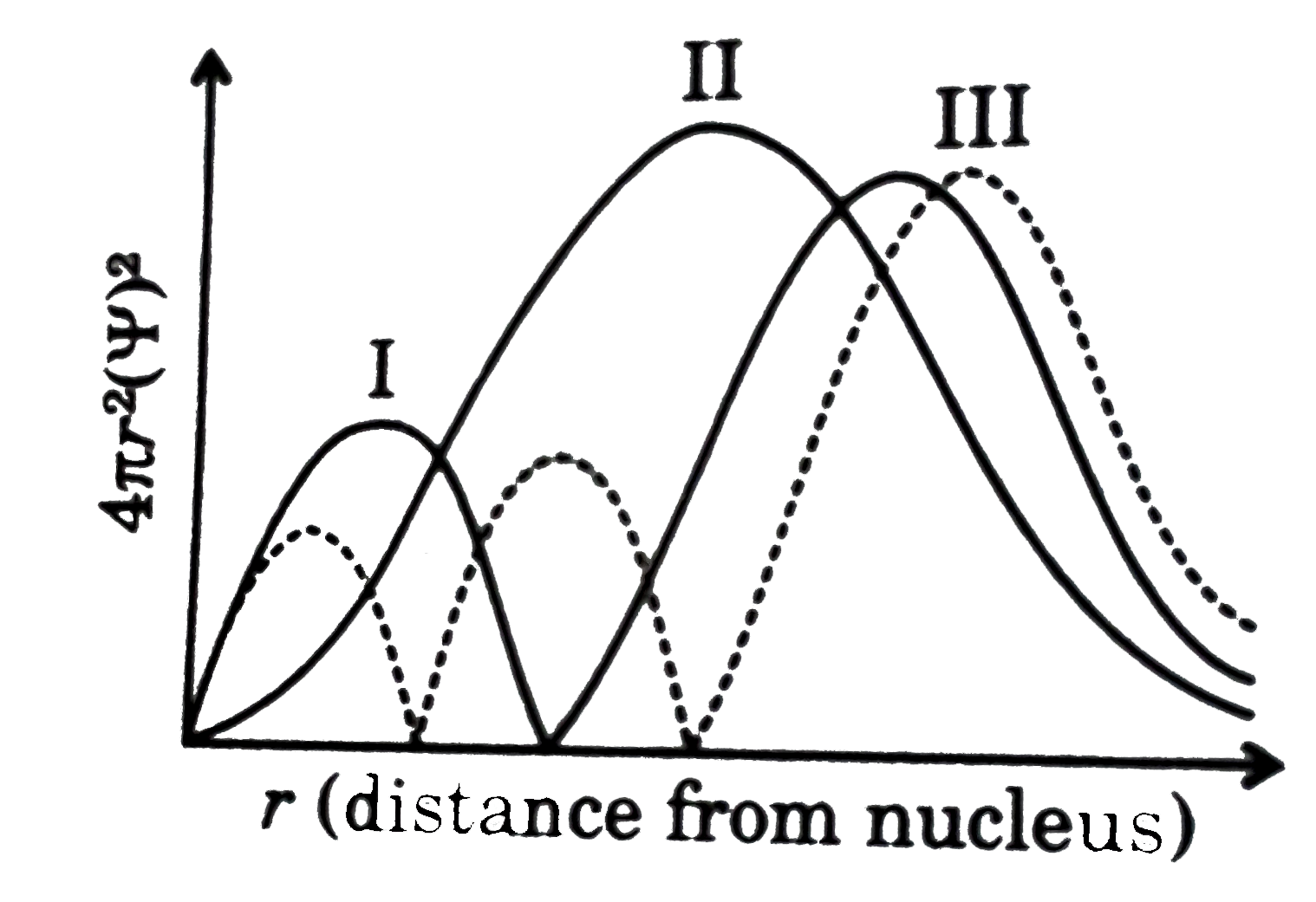
View SolutionWhich statement (s) are correct ?
(1) A 3s sub - shell has a maximum of 8 electrons.
(2) An element with configuration of `1s^(2) 2s^(2)3p^(6)3s^(2)` would be in the same group as beryllium.
(3) The general electronic configuration of the group containing `N_(2)` is `ns^(2) np^(2)`.
(4) A strontium atom has a filled outer shell if it loses tow electrons.
View SolutionIn a multi - electron atom , which of the following orbitals described by the three quantum numbers will have the same energy in the absence of magnetic and electric fields ?
(A) n=l, l=0,m=0 (B) n = 2, l =0 , m= 0
(C) n = 2 , l = 1 , m = 1 (D) n = 3, l =2 , m=1
(E) n=3,l=2,m=0.
View SolutionThe numbers of radial nodes of `3s and 2p` orbitals are respectively:
View Solution
Exercise questions and complete solutions for chapter STRUCTURE OF ATOM of DISHA PUBLICATION CHEMISTRY (HINGLISH) of Class 12