Complete Solutions to EXERCISE - 1 CONCEPT BUILDER of chapter NUCLEI of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | NUCLEI |
| DISHA PUBLICATION | EXERCISE - 1 CONCEPT BUILDER |
| Number Of Questions | 65 |
| Academic Year | 2023-24 |
`M_n` and `M_p` represent mass of neutron and proton respectively. If an element having atomic mass `M` has `N-`neutron and `Z`-proton, then the correct relation will be :
View SolutionIf the distance between nuclei is `2 xx 10^(-13)` cm , the density of nuclear material is (Assume that nuclear mass is order of `(10^(-27))`
View SolutionSize of nucleus is of the order of
View SolutionThe mass number of He is `4` and that for suphur is `32`. The radius of sulphur nuclei is larger than that of helium by
View SolutionA nucleus splits into two nuclear parts which have their velocity ratio equal to 5 : 1 . What will be the ratio of their nuclear radius ?
View SolutionThe volume of a nucleus is directly proportional to
View SolutionThe set which represents the isotope , isobar and isotone respectively is
View SolutionOutside a nucleus.
View SolutionThe mass of neutron is same as that of
View SolutionThe nuclei of which one of the following pairs of nuclei are isotons ?
View SolutionIf the radius of a nucleus `""^(256) X` is 8 fermi , then the radius of `""^(4) He` nucleus will be
View SolutionThe ratio of volume of nuclei (assumed ot be in spherical shape) with respective mass numbers 8 and 64 is
View SolutionAtomic weight of boron is `10.81` and it has two isotopes `._5 B^10` and `._5 B^11`. Then ratio of `._5 B^10` in nature would be.
View SolutionOrder of magnitude of density of uranium nucleus is , [m = 1.67 xx 10^(-27 kg]`
View SolutionThe binding energy per nucleon for `""_(1)H^(2)` and `""_(2)He^(4)` are 1.1 MeV and 7.1 MeV respectively. The energy released when two `""_(1)H^(2)` to form `""_(2)He^(4)` is ………….. MeV.
View SolutionIn the nuclear fusion reaction
`_(1)^(2)H + _(1)^(3)H rarr _(2)^(4)He + n `
given that the repulsive potential energy between the two nuclei is `- 7.7 xx 10^(-14) J` , the temperature at which the gases must be heated the reaction is nearly
[Boltzmann's constant `k = 1.38 xx 10^(-23) J//K]`
View SolutionTwo nucleons are at a separation of 1 fermi. The net force between them is `F_(1)`, if both are neutrons `F_(2)`, if both are protons and `F_(3)`, if one is a proton and the other is a neutron
View SolutionNuclear forces are
View SolutionFrom the following equations pick out the possible nuclear fusion reaction:
View SolutionWhich of the following statements is true ?
View Solution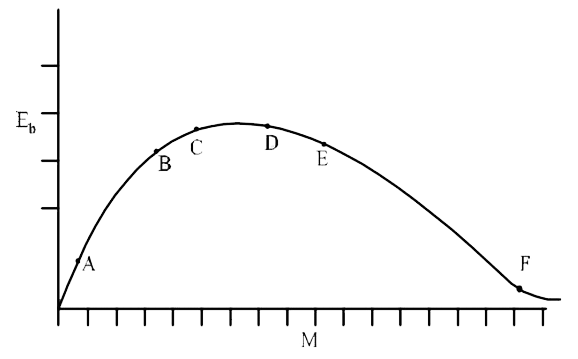
The above is a plate of binding energy per nucleon `E_(0)` against the nuclear mass `M, A, B,C,D,E,F` correspond to different nuclei Consider four reactions:
View SolutionWhen Uranium is bombarded with neutrons , it undergoes fission . The fission reaction can be written as :
`""_(92) U^(235) + ""_(0) n^(1) to ""_(56) Ba^(141) + ""_(36) Kr^(92) + 3x + Q` (energy)
where three particles named x are produced and energy Q is released . What is the name of the particle x ?
View SolutionIn a fission reaction `._92^236 U rarr ^117 X + ^117Y + n + n`, the binding energy per nucleon of `X` and `Y` is `8.5 MeV` whereas of `.^236 U` is `7.6 MeV`. The total energy liberated will be about.
View SolutionComplete the equation for the following fission process `._92 U^235 ._0 n^1 rarr ._38 Sr^90 +....`.
View SolutionWhich one of the following statements is true for nuclear forces?
View SolutionOn an average , the number of neutrons and the energy of a neutron released per fission of a uranium atom are respectively
View SolutionWhich of the following nuclear reaction is not possible?
View SolutionThe rest energy of an electron is.
View SolutionIf `M_(O)` is the mass of an oxygen isotope `._(8)O^(17),M_(p) and M_(n)` are the masses of a proton and a neutron, respectively, the nuclear binding energy of the isotope is
View SolutionEnergy released in the fission of a single `._92 U^235` nucleus is `200 MeV`. The fission rate of a `._92 U^235` fuelled reactor operating at a power level of `5 W` is.
View SolutionThe binding energy of deuteron is `2.2` MeV and that of `._(2)^(4)He` is `28` MeV. If two deuterons are fused to form one `._(2)^(4)He`, th `n` the energy released is
View SolutionIf `M(A,Z)`, `M_(p)and M_(n)` denote the masses of the nucleus `._(Z)X^(A)`, proton and neutron respectively in units of U (where`1U=931MeV//c^(2)`) and B.E. represents its B.E. in MeV, then
View SolutionThe power obtained in a reactor using `U^(235)` disintergration is `1000kW`. The mass decay of `U^(235)` per hour is
View SolutionA reaction between a proton and `._8 O^18` that produces `._9 f^18` must also liberate
View SolutionThe energy released in a typical nuclear fusion reaction is approximately
View SolutionRank the following nuclei in order from largest to smallest value of the binding energy per nucleon:
(i) `._2^4H`, (ii) `_(24)^(52)Cr`, (iii) `._(62)^(152)Sm`, (iv) `._(80)^(100)Hg`, (v) `._(92)^(252)Cf`.
View SolutionWhen the number of nucleons in a nuclues increases the binding energy per nucleon
View SolutionThe curve of blinding energy per nucleon as a function of atomic mass number has a sharp peak for helium nucleus. This implies that helium.
View SolutionBinding energy per nucleon plot against the mass number for stable nuclei is show in the figure. Which curve is correct ?

View SolutionA proton and a neutron are both shot at `100 m//s` towards a`_(6)^(12)C`nucleus. which particle,if either is more likely to be absorbed by the nucleus ?
View SolutionCalculate the binding energy of the deuteron, which consistss of a proton and a neutron, given that the atomic mass of the deuteron is 2.014102 amu,. Take mass of proton `(m_(p))=1.007825` amu, mass of a neutron `(m_(n))=1.008665` amu and 1amu=931.5 MeV
View SolutionThree `alpha-`particle and one `beta-`particle decaying takes place in series from an isotope `._88 Ra^238`. Finally the isotope obtained will be.
View SolutionIn radioactive transformation `""_(92)U^(235) to ""_(82)Pb^(206)` , the number of `alpha` and `beta` particles emitted are
View SolutionAn element X decays into element Z by two -steps process
`X to Y + He_(2)^(4)`
`Y to Z +2e` then
View SolutionNeutron decay in the free space is given follows:
`._0n^1 rarr. _1H^1 +._(-e)^0 +[]`
Then, the parenthesis represents
View SolutionA radioactive element` X` converts into another stable elemnet `Y`. Half-life of `X` is `2h`. Initally, only `X` is present. After time `t`, the ratio of atoms of `X` and `Y` is found to be `1:4` Then `t` in hours is .
View SolutionA radioactive sample contains `10^(-3)` kg each of two nuclear species A and B with half-life 4 days and 8 days respectively . The ratio of the amounts of A and B after a period of 16 days is
View SolutionTwo radioactive materials `X_1` and `X_2` have decay constants `10 lamda` and `lamda` respectively. If initially they have the same number of nuclei, then the ratio of the number of nuclei of `X_1` to that of `X_2` will be `1//e` after a time.
View SolutionIf `N_(0)` is the original mass of the substance of half - life period `t_(1//2) = 5 year` then the amount of substance left after `15` year is
View SolutionA radioactive sample at any instant has its disintegration rate `5000` disintegrations per minute After `5` minutes , the rate is `1250` disintegration per minute. Then , the decay constant (per minute)
View SolutionIf `T` is the half-life of a radioactive material, then the fraction that would remain after a time `(T)/(2)` is
View SolutionA nuclear transformation is denoted by `X(n,alpha) rarr. _(3)^(7)Li`. Which of the following is the nucleus of element `X`
View SolutionUsing a nuclear counter the count rate of emitted particles from a radioactive source is measured. At t=0 it was 1600 counts per second and t = 8 seconds it was 100 counts per second. The count rate observed, as counts per seconds, at t=6, seconds is close to :
View SolutionIn a smaple of rock, the ration `.^(206)Pb` to `.^(238)U` nulei is found to be `0.5.` The age of the rock is (given half-life of `U^(238)` is `4.5 xx 10^(9)` years).
View SolutionA radioactive sample with a half-life of `1` month has the label : 'Activity=
`2` microcurie on 1-8-1991'. What would be its avtivity two months earlier?
View SolutionThe count rate of a Geiger Muller counter for the radiation of a radioactive material of half-life `30` min decreases to `5 s^(-1)` after `2 h`. The initial count rate was
View SolutionThe half-life of `.^215At` is `100mus`. The time taken for the activity of a sample of `.^215At` to decay to `1/16th` of its initial value is
View SolutionThere are n number of radioactive nuclei in a sample that undergoes beta decay. If from the sample, `n'` number of `beta`-particels are emitted every `2s`, then half-life of nuclei is .
View SolutionThe half life of a radio isotope is 5 years . The fraction which will decay in 15 years , will be
View SolutionIn the given reaction
`._z X^A rarr ._(z+1)Y^A rarr ._(z-1) K^(A - 4) rarr ._(z-1) K^(A - 4)`
Radioactive radiations are emitted in the sequence.
View SolutionIn gamma ray emission from a nucleus
View SolutionThe ratio of half-life times of two elements `A` and `B` is `(T_(A))/(T_(B))`. The ratio of respective decay constant `(lambda_(A))/(lambda_(B))`,is
View SolutionConsider a radioactive material of half-life `1.0` minute. If one of the nuclei decays now, the next one will decay
View SolutionA sample of radioactive element has a mass of `10 g` at an instant `t=0`. The approximate mass of this element in the sample after two mean lives is
View Solution`alpha`-particles and `gamma`-rays are all having same energy . Their penetrating power in a given medium in increasing order will be
View Solution
EXERCISE - 1 CONCEPT BUILDER questions and complete solutions for chapter NUCLEI of DISHA PUBLICATION PHYSICS (HINGLISH) of Class 12