Complete Solutions to SECTION - A of chapter MODEL TEST PAPER - 3 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | MODEL TEST PAPER - 3 |
| VIVA PUBLICATION | SECTION - A |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
If A and B are square matrices of order 3 such that |A| = - 1 and |B| = 3 , then the value of |3AB| is a) 27 b) -27 c) -81 d) 81
View SolutionIf `[(a+b,2),(5,ab)]=[(6,2),(5,8)]` , then find the values of a and b respectively.
View SolutionThe range of the function `f(x) = 3x^2+7x+10` is
View SolutionFind the interval in which the function `f(x) = sqrt3 sin 2x - cos 2x + 4` is one - one
View Solution`(d)/(dx)(e^((1)/(2)log(1+tan^(2)x)))` is equal to
View SolutionEvaluate : `int(I+tan^2)/(I+cot^2x)dx`
View SolutionIf A and B be independent events with `P(A) = 1/4 and (P(A cupB) = 2P(B) - P(A) ` , then P(B) is equal to
View SolutionA binary operation `**` defined on `Q^(+)` the set of all positive is given `a**b = (ab) /2` Then the inverse of 3 is :
View SolutionFind the interval where the function f(x) `10 -x^3+6x^2-9x` increases with x.
View SolutionFind the value of k for which the function f(x) `f(x)={(kx+1,xlex),(cosx,xgtpi):}` is continuous at `x = pi`
View SolutionWithout expanding at any stage, prove that `|(0,99,-998),(-99,0,997),(998,-997,0)|=0`
View SolutionIf `sin (sin^(-1)""(1)/5 + cos^(-1)x) = 1`, then find the value of x. a) 0 b) 1/5 c) 2/5 d) 3/5
View SolutionIf `A=[(cosalpha,sinalpha),(-sinalpha,cosalpha)]` prove that `A.A^(T)=1` Hence find `A^(-1)`
View SolutionDetermine a binomial distribution whose mean is 9 and variance is 6.
View SolutionIf 2P(A) = P(B) `=5/13 and P(A/B) = 2/5` find `P(AcupB)`
View SolutionEvaluate : `int(dx)/(xsqrt(x^4-1))`
View SolutionEvaluate : `int((x^3+8)(x-1))/(x^2-2x+4)dx`
View SolutionLet A = R - {3} and B = R - {1}, consider the function `f A rarr B` defined by `=((x-2)/(x-3))` Show that f'is one-one and onto and hence find f inverse
View SolutionIf `y=x^x` then prove that is `(dy)/(dx)=x^(x)log_(e)(ex)` .
View SolutionIf `(d)/(dx)((1+x^2+x^4)/(1+x+x^2)) = a+bx` , find the values of a and b .
View SolutionIf x = sin t , y = sin 2t , prove that `(1-x^2)((dy)/(dx))^2=4(1-y^2)`
View SolutionEvaluate : `int_(pi/5)^((3pi)/(10))(cosx)/(sinx+cosx)dx `
View SolutionProve that : `1/6tan^(-1)""(2x)/(1-x^2)+1/9tan^(-1)""(3x-x^2)/(1-3x^2)+1/12 tan^(-1)""(4x-4x^3)/((1-6x^2+x^4))=` `tan^(-1)x`
View SolutionSolve the following differential equation: `xy(dy)/(dx)-y^2=(x+y)^2e^(-y//x)`
View SolutionUsing differentials, find the approximate value of `log_(e)` (4.01), given that `log_(e)4 = 1.3863.`
View SolutionA particle moves along the curve `y=x^2 + 2x` . At what point(s) on the curve are x and y coordinates of the particle changing at the same rate?
View SolutionThe probability distribution of a random variable X is given as under:
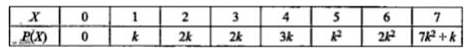
Find k , and P `(X lt 6)` .
View SolutionThe random variable X can take only the values 0, 1, 2. Given that P(X=0) = P(X= 1) = p and `E(X^2) = E(X)` , then find the value of p
View SolutionUsing properties of determinant, if `|(-a^2,ab,ac),(ab,-b^2,bc),(ac,bc,-c^2)| = mua^2b^2c^2`, find `mu `
View SolutionIf a matrix `A=((3,2,0),(1,4,0),(0,0,5))` show that `A^2-7A+10I_(3) = 0` and hence find `A^(-1)`
View SolutionEvaluate: `int(x^2)/(x^4+x^2-2)\ dx`
View SolutionEvaluate : `int_(0)^(1)(log(1+x))/(1+x^2)dx`
View SolutionThe three sides of a trapezium are equal, each being 6 cm long, find the area of the trapezium when it is maximum.
View SolutionA card from a pack of 52 playing cards is lost. From the remaining cards of the pack three cards are drawn at random (without replacement) and found to be all spades. Find the probability of the lost card being a spade.
View Solution
SECTION - A questions and complete solutions for chapter MODEL TEST PAPER - 3 of ARYA MATHS (ENGLISH) of Class 12