Complete Solutions to Section -C of chapter QUESTION PAPER-2018 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | QUESTION PAPER-2018 |
| GURUKUL PUBLICATION | Section -C |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
Given the total cost function for x units of commodity as `C(x)=1/3 x^3+3x^2-16x+2` . Find Marginal cost function .
View SolutionFind the value of `x` and `y` in given equations `x+2y+3=0 and 4x-5y+1=0` .
View SolutionThe average cost function associated with producing and marketing x units of an items is given by `AC=2x-11+(50)/(x)`. Find the range of value of the output x, for which AC is increasing.
View SolutionFind the line of regression of y on x from the following table :
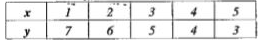
Hence estimate the value of y when x=6.
View SolutionFrom the given data :

and correlation coefficient is `2/3`. Find :
(i) Regression coefficient `b_(yx) and b_(xy)`
(ii) Regression line x on y.
(iii) Most likely value of x when y=14.
View SolutionA product can be manufactured at a total cost `C(x)=x^2/(100) x+100x+40` , where x is the number of units produced. The price at which each unit can be sold is given by `P=(200-x/(400))`. Determine the production level x at which the profit is maximum. What is the price per unit and total profit at the level of production?
View SolutionA manufacturer's marginal cost function is `(500)/sqrt(2x+25)`. Find the cost involved to increase production from 100 units to 300units.
View SolutionA manufacturing companu makes two types of teaching aids A and B of Mathematics for Class X. Each type of A requires 9 labour hours for fabircating and 1 labour for finishing. Each type of B requires 12 labour hours for fabircating and 3 labour hours for finishing. For fabricating and finishing, the maximum labour hours available per week are 180 and 30 respectively. The company makes a profit of 80 on each piece of type A and 120 on each piece of type B. How many pieces of type A and types B should be manufactured per week to get a maximum profit? Formulae this as Linear Programming Problems and solved it. Identify the feasible region from the rough sketch.
View Solution
Section -C questions and complete solutions for chapter QUESTION PAPER-2018 of ARYA MATHS (ENGLISH) of Class 12