Complete Solutions to JEE Main And Advanced of chapter CHEMICAL KINETICS of Class 12 book with complete answers and questions
| Class 12 | CHEMISTRY |
| Chapter | CHEMICAL KINETICS |
| IIT-JEE PREVIOUS YEAR | JEE Main And Advanced |
| Number Of Questions | 66 |
| Academic Year | 2023-24 |
Two reactions `R_(2) and R_(2)` have identical pre - exponential factors. Activations enery of `R_(1)` exceeds that of `R_(2)` by 10 kJ `mol_(-1)` . If `k_(1) and k_(2)` are rate constants for rate constants for reactions `R_(1) and R_(2)`
respectively at 300k , then In `(k_(2)/k_(1))`is equal to `(R=8.314 J mol^(-1)K^(-1))`
View SolutionDecompsition of `H_(2)O_(2)` follows a frist order reactions. In 50 min the concentrations of `H_(2)O_(2)` decreases from 0.5 to 0.125 M in one such decomposition . When the concentration of `H_(2)O_(2)` reaches 0.05 M, the rate of fromation of `O_(2)` will be
View SolutionHigher order `(gt3)` reaction are rare due to :
View SolutionFor the elementary reaction `MrarrN`, the rate of disappearance of `M` increases by a factor of `8` upon doubling the concentration of `M`. The order of the reaction will respect to `M` is
View SolutionFor the non-stoichiometric reaction `2A+BrarrC+D`
The following kinetic data were obtained in theee separate experiment, all at `98 K`
`|{:("Initial concentration (A)","Initial concentration (B)","Initial rate of formation of C" (molL^(-1) s^(-1))),(0.1 M,0.1 M,1.2 xx 10^(-3)),(0.1 M,0.2 M,1.2 xx 10^(-3)),(0.2 M,0.1 M,2.4 xx 10^(-3)):}|`
The rate law for the formation of `C` is:
View SolutionIn the reaction, `P+Q rarr R+S`
the time taken for `75%` reaction of `P` is twice the time taken for `50%` reaction of `P`. The concentration of `Q` varies with reaction time as shown in the figure. The overall order of the reaction is
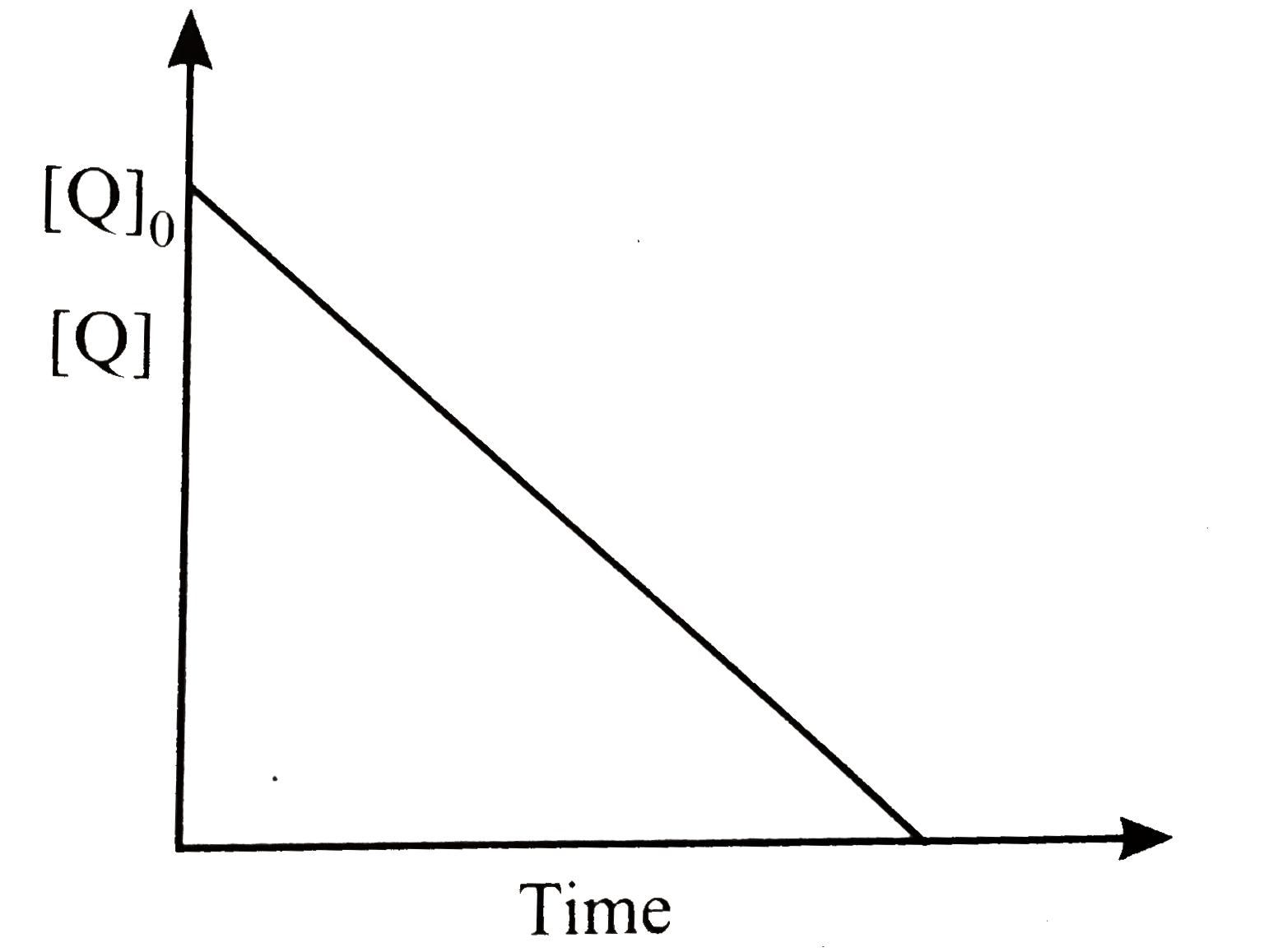
View SolutionThe rate of a reaction doubles when its temperature changes form `300 K` to `310 K`. Activation energy of such a reaction will be:
`(R = 8.314 JK^(-1) mol^(-1) and log 2 = 0.301)`
View SolutionPlots showing the variation of the rate constant `(k)` with temperature `(T)` are given below. The plot that follows the Arrhenius equation is
View SolutionFor a first order reaction `A rarr P`, the temperature `(T)` dependent rate constant `(k)` was found to follow the equation `log k = -2000(1//T) + 6.0`. The pre-exponential factor `A` and the activation energy `E_(a)`, respective, are
View SolutionUnder the same reaction conditions, the intial concentration of `1.386 mol dm^(-3)` of a substance becomes half in `40 s` and `20 s` theough first order and zero order kinetics, respectively.
The ratio `(k_(1)//k_(0))` of the rate constants for first order `(k_(1))` and zero order `(k_(0))` of the reaction is
View SolutionConisder a reaction `aG+bH rarr` Products. When concentration of both the reactants `G` and `H` is doubled, the rate increases eight times. However, when the concentration of `G` is doubled, keeping the concentration of `H` fixed, the rate is doubled. The overall order of reaction is
View Solutionwhich one of the following statements (s) in incorrect about order of reduction?
View SolutionA follows first order reaction.
`(A) rarr Product`
The concentration of `A` changes form `0.1 M` to `0.025 M` in `40 min`. Find the rate of reaction of `A` when the concentration of `A` is `0.01 M`.
View SolutionIn a first order reaction, the concentration of the reactant decreases form `800 "mol dm"^(-3)` to `50 "mol dm"^(-3)` in `2 xx 10^(4) s`. The rate constant of the reaction (in `s^(-1)`) is
View SolutionConisder the chemical reaction
`N_(2)(g) + 3H_(2)(g) rarr 2NH_(3)(g)`
The rate of this reaction can be expressed in terms of time derivatives of the concentration of `N_(2)(g), H_(2)(g)`, or `NH_(3)(g)`. Identify the correct relationship among the rate expresisons.
View SolutionIf `I` is the intenisty of an absorbed light and `c` is the concentration of `AB` for the photochemical process. `AB+hv rarr AB^(**)`, the rate of formation of `AB^(**)` is directly proportional to
View SolutionThe rate constant for the reaction, `2N_(2)O_(5) rarr 4NO_(2) + O_(2)` is `3.0 xx 10^(-5) s^(-1)`. If the rate is `2.40 xx 10^(-5) mol L^(-1) s^(-1)`, then the initial concentration of `N_(2)O_(5)` (in `mol L^(-1)`) is
View SolutionThe half`-` life periof of a radioactive element is 140 days. After 560 days, one gram of the element will reduce to
View SolutionA catalyst is a substance which
View SolutionThe specific rate constant of a first order reaction depends on the
View SolutionThe rate constant of a reaction depends on
View SolutionAccording to the Arrhenius equctions
View SolutionFor the first order reaction
`2N_(2)O_(5)(g) rarr 4NO_(2)(g) + O_(2)(g)`
View Solutionthe following statement (s) is are correct
View SolutionA catalyst
View SolutionWhat should be the age of fossil for meaningful determination of its age?
View SolutionA nuclear explosion has taken place leading to increase in concentration of `C^(14)` in nearby areas. `C^(14)` concentration is `C_(1)` in nearby areas and `C_(2)` in areas far away. If the age of the fossil is determind to be `T_(1) and T_(2)` at the places respectively then
View SolutionIn the Arrhenius equation, `k = A exp^(-Ea//RT)`, A may be termed as the rate constant at…………. .
View SolutionFor the reaction `N_(2)(g)+3H_(2)(g) rarr 2NH_(3)(g)`, under certain conditions of temperature and partial pressure of the reactants, the rate of formation of `NH_(3)` is `0.001 kg h^(-1)`. The same rate of converison of hydrogen under the same condition is................`kg h^(-1)`.
View SolutionThe hydroliss of ethyl acetate in………… medium is a…………..order reaction.
View SolutionThe rate of chemical change is directly proportional to …………… .
View SolutionFor a first order reaction, the rate of the reaction doubles as the concentration of the rection (S)doubles.
View SolutionAn organic compound undergoes first decompoistion. The time taken for its decompoistion to `1//8` and `1//10` of its initial concentration are `t_(1//8)` and `t_(1//10)`, respectively. What is the value of `([t_(1//8)])/([t_(1//10)]) xx 10`? `(log_(10)2 = 0.3)`
View Solution`2X(g) rarr 3Y(g)+2Z(g)`
`{:("Time (in min)",0,100,200,):}`
`|{:("Partial pressure of X (mm Hg)",800,400,200,),("Assuming ideal gas condition, calculate",,,,):}|`
(a) Order of reaction
(b) Rate constant
( c) Time taken for `75%` completion of reaction
(d) Total pressure when `p_(x) = 700 mm`
View SolutionFor the given reaction
`A+B rarr` Products,
the following data are given.
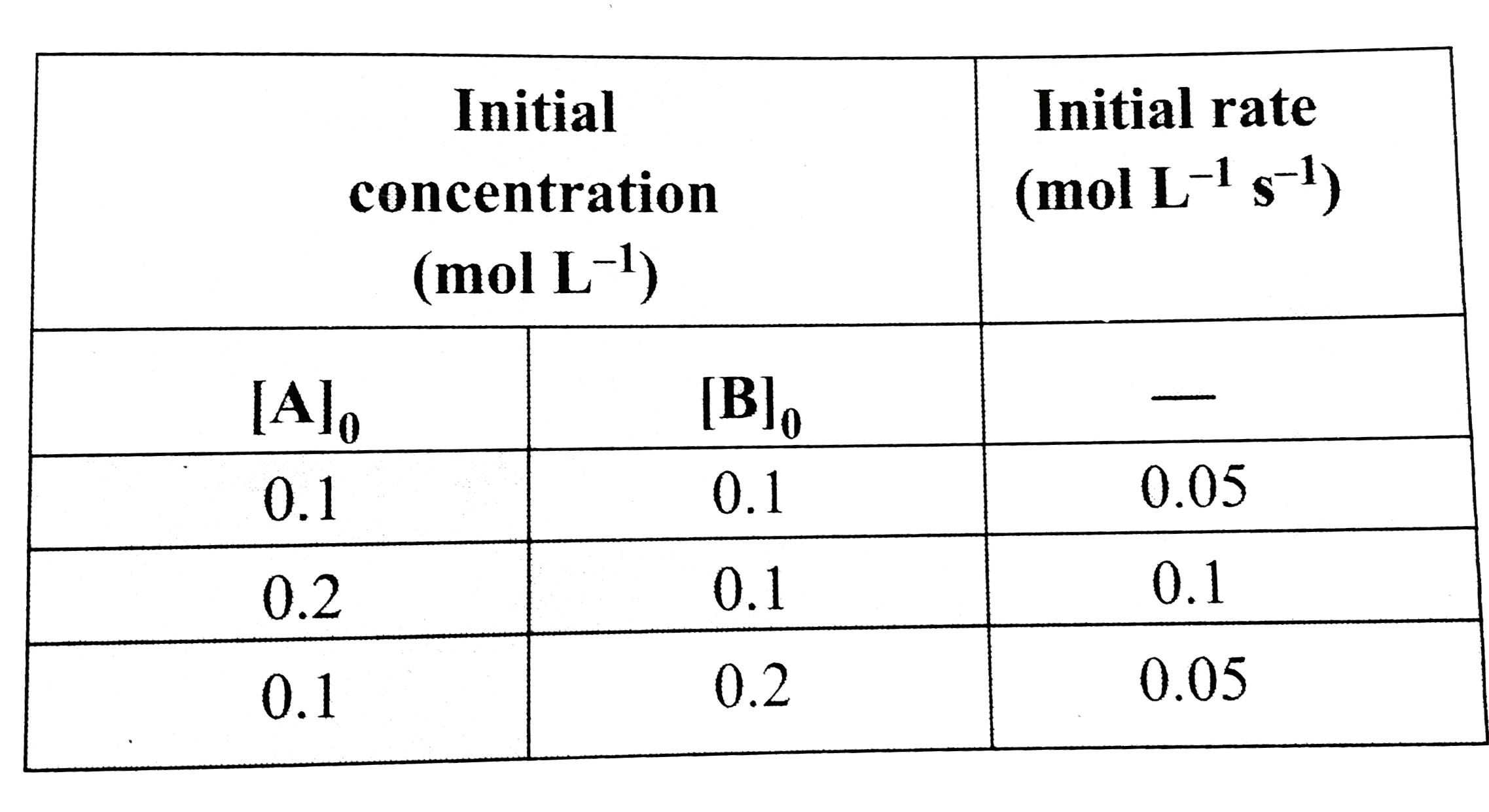
(a) Write the rate equation.
(b) Calculate the rate constant.
View SolutionThe rate of first order reaction is `0.04 mol L^(-1) s^(-1)` at `10 min` and `0.03 mol L^(-1) s^(-1)` at `20 min` after initiation. Find the half life of the reaction.
View SolutionA hydrogenation reaction is carried out at `500 K`. If the same reaction is carried out in the presence of a catalyst at the same rate, the temperature required is `400 K`. Calculate the activation energy of the reaction if the catalyst lowers the activation barrier by `20 kJ mol^(-1)`.
View SolutionThe rate constant for an isomerization reaction, `A rarr B` is `4.5 xx 10^(-3) min^(-1)`. If the initial concentration of `A` is `1 M`, calculate the rate of the reaction after `1 h`.
View Solutionthe rate constant of a reaction is `1.5xx10^(7) s^(-1) at 50^(@)C and 4.5 xx10 ^(7) s^(-1) at 100 ^(@)C.`Evaluate the Arrhenius paraments ` A and E^(a)`.
(ii) for the reaction `N_(2)O_(5)(g) to 2NO_(2)(g) + 1/2 O_(2)(g)`
calculate the mole fractions `N_(2)O_(5)(g)` decomposed at a constant valume and temperature , if the initial pressses os 600 mm Hg and the pressure at any time is 960 mm Hg Assume ideal gas behaviour.
View SolutionThe rate constant for the first order decompoistion of a certain reaction is described by the equation
`log k (s^(-1)) = 14.34 - (1.25 xx 10^(4)K)/(T)`
(a) What is the energy of activation for the reaction?
(b) At what temperature will its half-life periof be `256 min`?
View SolutionOne of the hazards of nuclear explosion is the generation of `.^(90)Sr` and its subsequent incorporation in bones. This nuclide has a half-life of `28.1` year. Suppose one micro-gram was absorbed by a new-born child, how much `Sr^(90)` will remain in his bones after 20 year?
View SolutionAt `380^(@)C` , the half-life periof for the first order decompoistion of `H_(2)O_(2)` is `360 min`. The energy of activation of the reaction is `200 kJ mol^(-1)`. Calculate the time required for `75%` decompoistion at `450^(@)C`.
View Solutionform the following data for the reaction between `A` and `B`,

(a) Calculate the order of the reaction with respect to `A` and with respect to `B`.
(b) Calculate the rate constant at `300 K`.
( c) Calculate the pre-expontential factor.
View SolutionThe gas phase decomposition of dimethyl ether follows first order kinetics.
`CH_(3)-O-CH_(3)(g)rarrCH_(4)(g)+H_(2)(g)+CO(g)`
The reaction is carried out in a constant volume container at `500^(@)C` and has a half life of `14.5 min`. Initially, only dimethyl ether is present at a pressure `0.40 atm`. What is the total pressure of the system after `12min`? (Assume ideal gas behaviour)
View SolutionA first order reaction `A rarr B` requires activation energy of `70 kJ mol^(-1)`. When a `20%` solution of `A` was kept at `25^(@)C` for `20 min`, `25%` decomposition took place. What will be the percentage decomposition in the same time in a `30%` solution maintained at `40^(@)C` ? (Assume that activation energy remains constant in this range of temperature)
View SolutionTwo reaction, `(I)A rarr` Products and `(II) B rarr` Products, follow first order kinetics. The rate of reaction `(I)` is doubled when the temperature is raised form `300 K` to `310K`. The half life for this reaction at `310K` is `30 min`. At the same temperature `B` decomposes twice as fast as `A`. If the energy of activation for reaction `(II)` is twice that of reaction `(I)`, (a) calculate the rate of constant of reaction `(II)` at `300 K`.
View SolutionThe nuclide ratio, `._(1)^(3) H` to `._(1)^(1) H` in a sample of water is `8.0xx10^(-18) : 1` Tritium undergoes decay with a half-life period of `12.3yr` How much tritium atoms would `10.0g` of such a sample contains `40` year after the original sample is collected?
View SolutionThe decompoistion of `N_(2)O_(5)` according to the equation `2N_(2)O_(5)(g) rarr 4NO_(2)(g)+O_(2)(g)`
is a first order reaction. After `30 min`, form the start of the decompoistion in a closed vessel, the total pressure developed is found t be `284.5 mm Hg`. On complete decompoistion, the total pressure is `584.5 mm Hg`. Calculate the rate constant of the reaction.
View SolutionIn a Arrhenius equation for a certain reaction, the values of `A and E_(a)` ( energy of activation)are `4xx10^(13)s^(-1)` and `98.6KJ mol^(-1)`, respectively. If the reaction of first order at, what temperature will its life periof be `10 min`.
View SolutionAN experiment requires minimum beta activity produced at the rate of 346 beta particles per minute. The half- life period of `_(42)Mo^(99)` , which is a beta emitter , is 66.6 h . Find the minimum amount of `_(42)Mo^(99)` required to carry out the experiment in 6.909 h.
View SolutionA first order gas reaction has `k = 1.5 xx 10^(-6) s^(-1)` at `200^(@)C`. If the reaction is allowed to run for `10 h`, what have changed in the Product? What is the half-life of this reaction?
View SolutionWhile studying the decompoistion of gaseous `N_(2)O_(5)`, it is observed that a plot of logarithm of its partial pressure versus time is linear. What kinetic parameters can be obtained form this observation?
View SolutionRadioactive decay is a first`-` order process. Radioactive carbon in wood sample decays with a half`-` life of 5770 years. What is the rate constant `(` in years `)` for the decay ? What fraction would remains after 11540 years ?
View SolutionCalculating time requireed to complete a definite fraction of first order reaction : A first order reaction is `20%` complete in 10 minutes. Calculate the time taken for the reaction to go to `75%` completion.
Stratagy : First calculate the rate constant (k) using frist data and then calculate the required time to complete `75%` of the reaction by using the value of rate constant :
View SolutionRate of a reaction `A + B rarr` Product, is given as a function of different initial concentration of `A` and `B`.
`|{:([A] (mol L^(-1)),(B) (mol L^(-1)),"Initial rate" (mol L^(-1) min^(-1)),),(0.01,0.01,0.005,),(0.02,0.01,0.010,),(0.01,0.02,0.005,):}|`
Determine the order of the reaction with respect to `A` and with respect to `B`. What is the half life of `A` in the reaction ?
View SolutionThe activity of a radioactive isotope is `3000` count per minute at a certai time and `2736` count per minute after `48` h later. The half-life of this isotope is
View SolutionFor a reaction, `Ato` Product, half life measured for two different values of inital concentrations `5xx10^(-3) M` and `25xx10^(-4) M` are 1.0 and 8.0 h respectively. If the initial concentration is adjusted to `1.25xx10^(-3) M`, the new half-life would be
View SolutionIn a consecutive reaction : `A to B to C` starting with only A
View SolutionFor a first order chemical raction, which of the following describe the plot of logarithmic of friction of uncreated reactant vs time?
View SolutionFor a general nth order process,`AtoP` with initial concentration of reactant "a" and rate constant, the expression for time for `75%` completion of reaction is
View SolutionWhich of the following is (are) correct for variation of rate constant with temoperature?
View SolutionWhich of the following is (are) true for the rate constant of a chemical reaction?
View SolutionAssertion : In a chemical reaction, rate of reaction always decreases with time.
Reason In a chemical reation, the amount of reactant remaining always decreases with time.
View SolutionAssertion: A catalyst increases the rate of a given reaction by greater factor if added at lower temperature than at higher temperature.
Reason Rate of reaction always increases on increasing temperature.
View SolutionMatch the statemens of Column I with values of Column II.

View SolutionConsider the following parallel first order reactions:

Half-life of the overall decay of A is 2 ys while half-life for the formation of B is 3yr. Half-life for the formation for C (in years) is :
View Solution
JEE Main And Advanced questions and complete solutions for chapter CHEMICAL KINETICS of IIT-JEE PREVIOUS YEAR (CHEMISTRY) of Class 12