Complete Solutions to Solved Examples of chapter STRAIGHT LINES - FOR BOARDS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | STRAIGHT LINES - FOR BOARDS |
| KC SINHA | Solved Examples |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
What can be said regarding a line if its slope is i. positive ii. zero iii negative?
View SolutionFind the slope of a line whose inclination is `150^@`
View SolutionFind the inclination of the line having slope (i) `-1`
View SolutionFind the inclination of the line having slope (i) `-3`
View SolutionFind the slope of the line through the points `(4,-6)(-2,-5)`
View SolutionShow that the line joining (2,-3) and (-5,1) is parallel to the line joining (7,-1) and (0,3).
View SolutionShow that the line joining `(2, -3) and (-5, 1)` is : Perpendicular to the line joining `(4, 5) and (0, -2)`
View SolutionExamine whether the line joining `(8, 2) and (-5, 3)` is parallel to or perpendicular to or neither parallel nor perpendicular to the line joining `(16, 6) and (3, 15)`
View SolutionWithout using the Pythagoras theorem, show that the points `(4, 4)`, `(3, 5)`and `(1, 1)`are the vertices of a right angled triangle.
View SolutionIf points `(a ,0),\ (0, b)a n d\ (x , y)` are collinear, using the concept of slope prove that `x/a+y/b=1.`
View SolutionA quadrilateral has the vertices at the points `(-4,2),\ (2,6),\ (8,5)\ a n d\ (9,-7)` . Show that the mid points of the sides of this quadrilateral are the vertices of a parallelogram.
View SolutionProve that the line joining the mid-points of the two sides of a triangle is parallel to the third side.
View SolutionIf `A(2,0),B(0,2)a n dC(0,7)` are three vertices, thaken in order, of an isosceles trapezium `A B C D` in which `A B||D Cdot` find the coordinates of `Ddot`
View SolutionIn Figure, time and distance graph of a linear motion is given. Two positions of time and distance are recorded as, when T = 0, D = 2 and when T = 3, D = 8. Using die concept of slope, find law of motion, i.e., how distance depends upon time.
View SolutionConsider the following population and year graph: find the slope of the line AB and using it find what will be the population in the year 2010.
View SolutionFind the equation of the line parallel to the y-axis and 3 units to the right of it.
View SolutionFind the equation of the line parallel to x-axis and passing through the point `(3, -4)`.
View SolutionFind the equation of the line perpendicular to x-axis and having intercept -2 on x-axis.
View SolutionFind the equation of the line which cuts off an intercept `-5` on y-axis and has slope `1/2`.
View SolutionFind the equation of the line which intersects the y-axis at a distance of 2 units above the origin and makes and angle of `30^(@)` with the positive direction of the x-axis.
View SolutionFind the equation of the straight line which makes angle of `15^0` with the positive direction of c-axis and which cuts and intercepts of length 4 length 4 on the negative direction of Y-axis.
View SolutionFind eqn of line which cut off an intercept of 4 units on the x- axis and makes an angle of `30^@` with positive direction of y- axis.
View SolutionFind the equation of the line passing through `(-4, 3)` and having slope = `1/2`.
View SolutionFind the equation of the straight line which passes through the point `(1, 2)` and makes an angle `theta` with the positive direction of x-axis where `costheta = - 1/3`.
View SolutionA line through the point `A(2, 0)` which makes an angle of `30^0` with the positive direction of x-axis is rotated about `A` in clockwise direction through an angle `15^0`. Find the equation of the straight line in the new position.
View SolutionFind the lines through the point (0,2) making angles `pi/3` and`(2pi)/3` with the x-axis. Also, find the lines parallel to the cutting the y-axis at a distance of 2 units below the origin.
View SolutionThe mid-points of the sides of a triangle are `(2,1),(-5,7) and (-5,-5)dot` Find the equations of the sides of the triangle.
View SolutionIf `A(1, 4), B(2, -3) and C (-1, -2)` are the vertices of a `DeltaABC`. Find (i) the equation of the median through A (ii) the equation of the altitude through A. (iii) the right bisector of the side `BC`.
View SolutionFind the equation of the perpendicular bisector of the line segment joining the points (1,1) and (2,3).
View SolutionShow that the perpendicular drawn from the point `(4,1)` on the line segment joining `(6,5)a n d(2,-1)` divides it internally in the ratio `8: 5.`
View SolutionOne side of a square makes an angle `alpha` with x axis and one vertex of the square is at origin. Prove that the equations of its diagonals are `x(sin alpha+ cos alpha) =y (cosalpha-sinalpha)` or `x(cos alpha-sin alpha) + y (sin alpha + cos alpha) = a`, where a is the length of the side of the square.
View SolutionFind the equation of the line joining the points `(-1,3)a n d\ (4,-2)dot`
View SolutionFind the equations to the diagonals of the rectangle the equations of whose sides are `x=a ,x=a^(prime),y=b ` `a n d ` `y=b^(prime)`
View SolutionFind the equation of the internal bisector of angle `B A C` of the triangle `A B C` whose vertices `A ,B ,C` are`(5,2),(2,3)a n d(6,5)` respectively.
View SolutionA rectangle has two opposite vertices at the points (1, 2) and (5,5). It these vertices lie on the line `x=3` , find the other vertices of the rectangle.
View SolutionIn what ratio is the line joining the pints `(2,3)a n d\ (4,-5)` divided by the line passing through the points (6,8) and (-3,-2).
View SolutionFind the coordinates of the vertices of `a` square inscribed in the triangle with vertices `A(0,0),B(3,0) a n d C(2,1);` given that two of its vertices are on the side `A Bdot`
View SolutionFind the equation of the straight line which passes through the point `(3, 4)` and whose intercept on y-axis is twice that on x-axis.
View SolutionA straight line moves so that the sum of the reciprocals of its intercepts made on axes is constant. Show that the line passes through a fixed point.
View SolutionFind the equations of the lines, which cut-off intercepts on the axes whose sum and product are `1` and `-6` , respectively.
View SolutionFind the equation of the lines which passes through the point (3,4) and cuts off intercepts from the coordinate axes such that their sum is 14.
View SolutionA line passes through the point (3,-2). Find the locus of the middle point of the portion the line intercepted between the axes.
View SolutionFind the equation of the line upon which the length of perpendicular `p` from origin and the angle `apha` made by this perpendicular with the positive direction of x-axis are `p=5, alpha = 135^0`
View SolutionSketch roughly the lines satisfying the given conditions and write their equations:
inclination `theta=150^(@)`, and distance from the origin = 3.
View SolutionFind the equation of the straight line upon which the length of perpendicular from origin is `3sqrt(2)` units and this perpendicular makes an angle of `75^0` with the positive direction of x-axis.
View SolutionFind the equation of the straight line upon which the length of the perpendicular from the origin is 2 and the slope of this perpendicular is `5/(12)dot`
View SolutionA straight canal is at a distance of `4 1/2` km from a city and the nearest path from the city to the canal is in the north - east direction . Find whether a village which is at 3 km north and 4 km east from the city lies on the canal or not , then on which side of the canal is the village situated ?
View SolutionFind the equation of the straight line which makes a triangle of area `96sqrt(3)` with the axes and perpendicular from the origin to it makes an angle of `30^0` with y-axis.
View SolutionFind the equation of the line through `(-2, 1)` in symmetric form when the angle made by the line with the positive direction of x-axis is `45^0`
View Solutionfind the equation of the straight line which passes through the point `(3,2)` and whose gradient is `3/4`.find the co-ordinate of the points on the line that are `5` units away from the point `(3,2)`.
View SolutionFind the direction in which a straight line must be drawn through the point `(1,2)` so that its point of intersection with the line `x+ y =4` may be at a distance of 3 units from this point.
View SolutionFind the coordinatse of the points at a distance `4sqrt(2)` units from the point `(-2, 3)` in the direction making an angle of `45^0` with the positive direction of x-axis.
View SolutionThe co-ordinates of the extremities of one diagonal of a square are `(1, 1)` and `(1,-1)` Find the co-ordinates of its other vertices and the equation of the other diagonal
View SolutionIn the given figure, PQR is an euilateral triangle and OSPT is a square. If OT `=2sqrt(2)` units, find the equation of lines OT, OS, SP, QR, PR, and PQ.
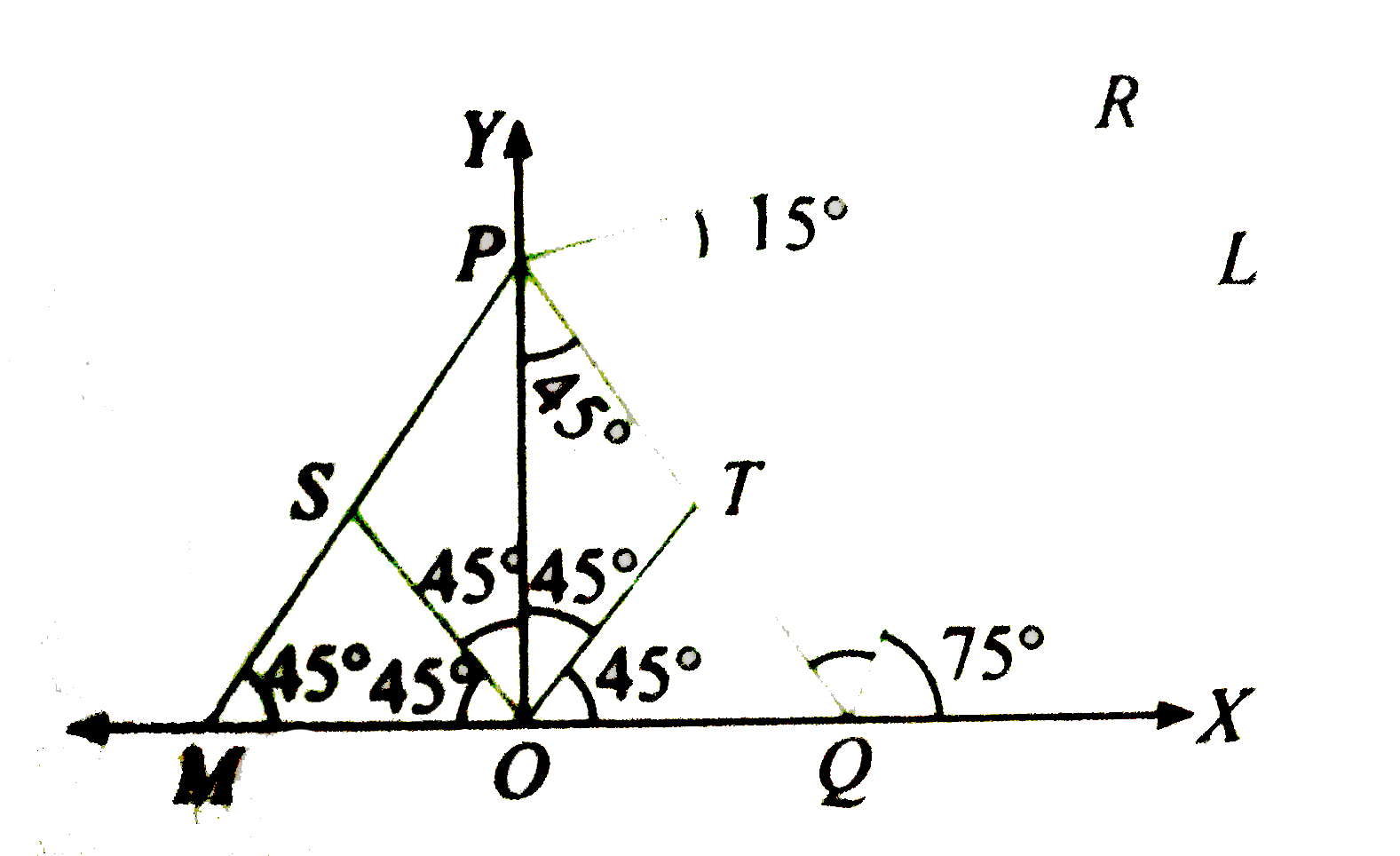
View SolutionThe length L (in centimetre) of a copper rod is a linear function of its Celsius temperature C. In an experiment, if `L" "=" "124. 942` when `C" "=" "20` and `L=" "125. 134` when `C" "=" "110` , express L in terms of C.
View SolutionThe owner of a milk store finds that, he can sell `980` litres of milk each week a `Rs. 14//"litre"` and `1220` litres of milk each week at `Rs 16//"litre"`. Assuming a linear relationship between selling price and demand, how many lire could he sell weekly at `Rs 17//litres`?
View SolutionTransform equation `sqrt(3) y-3x=3` to the slope intercept form and also find the angle which straight line makes with the x-axis.
View SolutionReduce `x+sqrt(3y) + 4 = 0 ` to the :
(ii) Intercept form and find its intercepts on the axes
View SolutionFind the point of intersection of the line, `x/3 - y/4 = 0 and x/2 + y/3 = 1`
View SolutionFind the area of the triangle whose sides are: `3x-2y+1=0, 3x+y+4=0 and 3x-5y+34=0`
View SolutionThe equation of the medians of a triangle formed by the lines `x+y-6=0, x-3y-2=0` and `5x-3y+2=0` is
View SolutionFind the coordinates of the circumcentre of the triangle whose vertices are `(5, 7), (6, 6) and (2, -2)`
View SolutionShow that the lines `4x+y-9=0,x-2y+3=0,5x-y-6=0` make equal intercepts on any line of slope 2.
View SolutionA line is such that its segment between the lines `5x-y+4=0` and `3x+4y-4=0` is bisected at the point (1,5). Obtain its equation.
View SolutionFind the coordinates of the orthocentre of the triangle whose vertices are `(0, 1), (2, -1) and (-1, 3)`
View SolutionTwo vertices of a triangle are `(3,-1)a n d(-2,3)` and its orthocentre is at the origin,. Find the coordinates of eth third vertex.
View SolutionTwo consecutive sides of a parallelogram are 4x+5y = 0 and 7x + 2y = 0. If the equation of one diagonal is 11x + 7y=9, find the equation of the other diagonal.
View SolutionProve that the lines `sqrt(3)x+y=0,\ sqrt(3)y+x=0,\ sqrt(3)x+y=1\ a n d\ sqrt(3)y+x=1` form a rhombus.
View SolutionProve that the straight lines 4x+7y=9, 5x-8y+15=0 and 9x-y+6=0 are concurrent.
View SolutionFind the value of p, so that three lines `3x + y = 2, px + 2y-3=0` and `2x-y=3` are concurrent.
View SolutionIf the lines whose equations are `y=m_1 x+ c_1 , y = m_2 x + c_2 and y=m_3 x + c_3 ` meet in a point, then prove that : `m_1 (c_2 - c_3) + m_2 (c_3 - c_1) + m_3 (c_1 - c_2) =0`
View SolutionIf the lines `p_1x+q_1y=1,p_2x+q_2y=1a n dp_3x+q_3y=1,` be concurrent, show that the point `(p_1, q_1),(p_2, q_2)a n d(p_3, q_3)` are collinear.
View SolutionThe lines 1)`(p-q)x+(q-r)y+(r-p)=0,2)(q-r)x+(r-p)y+(p-q)=0,3)(r-p)x+(p-q)y+(q-r)=0`
View SolutionProve analytically that the medians of a triangle are concurrent.
View SolutionFind the angle between the lines `y-sqrt(3)x-5=0`and `sqrt(3)y-x+6=0`.
View SolutionFind the angle between the lines `x-2y+3=0 and 3x+y-1=0`.
View SolutionFind the angle between the lines `3x=5 and 3x+5y-2=0`.
View SolutionFind the angle between ` x+y=3` and the line joining points (1,1) and (-3,4)
View SolutionProve that the points (2,-1), (0,2), (2,3) and (4,0) are the coordinates other vertices of a parallelogram and find the angle between its diagonals.
View SolutionIs the triangle, whose vertices are `(5, -6), (1, 2) and (-7, -2)`, a right-angled triangle, an acute-angled triangle or an obtuse-angled triangle?
View SolutionProve that that s triangle which has one of the angle as `30^0` cannot have all vertices with integral coordinates.
View SolutionFind the value of `k` if the straight line `2x+3y+4+k(6x-y+12)=0` is perpendicular to the line `7x+5y-4=0.`
View SolutionExamine the following pair of lines are intersecting, parallel, coincident or perpendicular : `x+y+2=0 and 2x+2y-7=0`
View SolutionExamine the following pair of lines are intersecting, parallel, coincident or perpendicular : `x+y+2=0 and 2x-3y+5=0`
View SolutionExamine the following pair of lines are intersecting, parallel, coincident or perpendicular `x+y+2=0 and 2x+2y+4=0`
View SolutionExamine the following pair of lines are intersecting, parallel, coincident or perpendicular `2x+y+2=0 and x-2y+5=0`
View SolutionIf `A(2,0),B(0,2)a n dC(0,7)` are three vertices, thaken in order, of an isosceles trapezium `A B C D` in which `A B||D Cdot` find the coordinates of `Ddot`
View Solutionabout to only mathematics
View SolutionA ,B and C are the points `(2, 0),(5,0) and (5,3)` respectively. Find coordinates of `D` such that `ABCD` is a square.
View SolutionIf the angle between two lines is `pi/4`and slope of one of the lines is `1/2`, find the slope of the other line.
View SolutionFind the slope of the lines which make an angle of `45^0` with the line `3x-y+5=0.`
View SolutionThe line `2x-y = 5` turns about the point on it, whose ordinate and abscissae are equal through an angle of `45^@` in the anti-clockwise direction. Find the equation of the line in the new position.
View SolutionFind the equation of the lines through the point (3, 2) which make an angle of `45o`with the line `x-2y=3`.
View SolutionA vertex of an equilateral triangle is (2,3) and the equation of the opposite side is x+y=2. Find the equation of the other sides of the triangle.
View SolutionWrite the formula for the area of the triangle having its vertices at `(x_1,\ y_1),\ \ (x_2,\ y_2)` and `(x_3,\ y_3)` .
View SolutionA line `4x+y=1` through the point `A(2,-7)` meets the line `B C` whose equation os `3x=4y+1=0` at the point `Bdot` Find the equation to the line `A C` so that `A B=A Cdot`
View Solutionabout to only mathematics
View SolutionA ray of light is sent along the line `x-2y-3=0` upon reaching the line `3x-2y-5=0,` the ray is reflected from it. Find the equation of the line containing the reflected ray.
View SolutionThe equation of the line through (3, 4) and parallel to the line` y =3x+5` is
View SolutionFind the equation of the straight line through (2, 3) and perpendicular to the line `4x-3y=10`
View Solution
Solved Examples questions and complete solutions for chapter STRAIGHT LINES - FOR BOARDS of KC SINHA ENGLISH of Class 12