Complete Solutions to PART-A of chapter ANNUAL EXAMINATION :SAMPLE PAPER of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | ANNUAL EXAMINATION :SAMPLE PAPER |
| KUMAR PRAKASHAN | PART-A |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
if ` F :R to R f (x) = (5 -x^5 )^(1/5) ` then ` (fof) (x) =………`
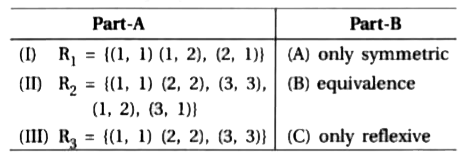
View SolutionIF ` A={1,2,3}` then match following subsets of ` A xx A ` properly
View SolutionIf ` f: N toN , f(x) = 2x +3` then …….
View Solution`tan^(-1)(x/y)-"tan"^(-1)(x-y)/(x+y)` is equal to
View Solution`sin {(pi)/(3) - sin^(-1) ((-1)/(2)) }=…….`
View SolutionIf ` cos^(-1) ((x)/(5)) + "cosec"^(-1) ((5)/(4)) =ppi/2 ` then x=…….
View SolutionIf `x=1/3`, then the value of `cos(2cos^(-1)x+sin^(-1)x)` = ____
View Solutionequal to =………
View Solutionif `[ ( cos alpha, - sin alpha),(sin alpha, cos alpha)]` and `A +A^1 =I` then `alpha =……`
View SolutionIf A is a square matrix such that ` A^2 =A ` then `(I+A)^2 -7A `=……
View SolutionIf A and B are symmetric matrices of same order , then AB +BA is a ……
View SolutionIf the area of the triangle with vertices `(-2,0) ( 0,4) (0,k) ` having 4 sq. units then K=
View SolutionIf `A = [( 1, cos theta , 1),( - cos theta ,1,cos theta ),( -1 , - cos theta ,1)]` where ` 0 le theta le 2 pi` then …..
View SolutionIf `D=[(0,i-100 , i-500 ),(100 -i ,0,1000-i),(500 -i,i-1000 ,0)]` then ` |D| =……`
View SolutionIf ` f (x) =[((1-cos kx)/(x^2) : x ne 0),(8 , x=0)]` is continues at ` x=0` , then K=……
View SolutionIf ` e^x + e^y = e^(x+y) ` then ` (dy )/( dx) =……..`
View Solution`(d)/(dx ) ( e^( tan^(-1) x+ cot^(-1) x)) =……. : (x in R)`
View SolutionThe interval in which `y= x^2 . e^(-x) ` is increasing is -----
View SolutionThe line `y= m x +1 ` is a tangent to the curve `y^2 =4 x ` if the value of m is _____
View SolutionThe normal at the point (2,-2) on the curve ` 3x^2 -y^2 =8` is _____
View SolutionApproximate value of ` (31)^(1/5) ` is ____
View Solution`int_(-1)^(1) log ((2019 -x)/(2019 +x)) dx =………`
View Solution`int_(0)^(1) (dx)/( x+ sqrt(x)) =……`
View Solution`int_(0)^(2) x (2-x ) ^(3/2) dx =……`
View Solution`int sin ( log x) dx =………+c`
View Solution`int(dx)/(sqrt(e^(2x)-1)) =……+c`
View SolutionEvaluate `int_(pi/6)^(pi/3)(dx)/(1+sqrt(tanx))`
View SolutionChoose the correct answers
The value of `int_(0)^(1)tan^(-1)((2x-1)/(1+x-x^(2)))dx` is
View Solution`int_(0)^((2pi)/(3)) sqrt( 1+ cos 2x ) dx=………`
View SolutionArea of the regiion bounded by ` (x^2 )/(16) +(y^2)/(9) = 4 `
View SolutionArea of the region bounded by the curve ` y= sin x and x` -axis `- pi/2 le x le (pi)/(2)` ………
View SolutionSmaller area enclosed by the circle ` x^2 + y^2 = 4 ` and the line ` x+y =2 ` is ……….
View SolutionThe order and degree of differential equation ` xy (d^2 y)/( dx^2) +((dy)/(dx))^2 -y ((dy)/(dx))^3 =0` is …….
View SolutionVerify that the function `y = e^(-3x)` is a solution of the differential equation
`(d^(2)y)/(dx^(2)) + (dy)/(dx) - 6y = 0`
View SolutionThe number of arbitrary constants in the particular solution of a differential equation of third order are …….
View SolutionFind angle `theta` between the vectors `veca=hati+hatj-hatkandvecb=hati-hatj+hatk`.
View SolutionFind `|veca-vecb|`, if two vectors `vecaandvecb` are such that `|veca|=2,|vecb|=3andveca*vecb=4`.
View SolutionThe value of `hati.(hatjxxhatk)+hatj*(hatixxhatk)+hatk*(hatixxhatj)` is
View SolutionIf `vec(a)` and `vec(b)`, are two collinear vectors, then which of the following are incorrect :
(A) `vec(b)=lambda vec(a)`, for some scalar `lambda`
(B) `vec(a)=+-vec(b)`
( C ) the respective components of `vec(a)` and `vec(b)` are not proportional
(D) both the vectors `vec(a)` and `vec(b)` have same direction, but different magnitudes.
View SolutionIf scalar product of vectors ` veca ` with vectors ` 3 hati - 5 hatk , 2 hati + 7 hatj and hati + hatj + hatk ` are respectively `-1,6,5` then ` veca ` =………
View SolutionFor two non - zero vectors ` veca and vecb | veca + vecb| = | veca|` then vectors ` 2 veca + vecb and vecb ` are …..
View SolutionThe coordinates of the foot of the perpendicular drawn from the origin to the plane `2x -3y +4z -6=0` are ……
View SolutionThe angle `"bet"^(n)` two line `( x+3)/(3) = (y-1)/(5) = (z +3)/(4) and ( x+1)/(1) =(4-y)/(-1) =(z-5)/(2) ` is ……..
View SolutionDistance between the two planes ` 2x + 3y +4z -4=0` and `4x + 6 y + 8z = 12 ` is …….
View SolutionThe objective function of a linear programming problem is ..........
View SolutionIn the question of maximum value of z = 800 x + 12000 y subject to constraints ` 9x +12 y le 180, 3x + 4y le 60 , x + 3y le 30 , x ge 30 , x ge 0, y ge 0 ……..` is not a point of feasible region .
View Solutionin solving the L.P problem " minimize ` z= 6 x + 10 y ` subject to ` x ge 6, y ge 2, 2x +y ge 10 , x ge 0 y ge 0"` redundant constraints are ……..
View SolutionChoose the correct answer
The mean of the numbers obtained on throwing a die having written 1 on three faces, 2 on two faces and 5 on one face is
View SolutionE,F are independent events and P(E ) `ne O, P(F ) ne O , `then ……. Is false .
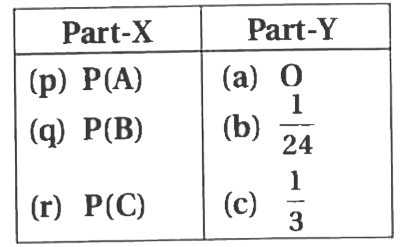
View Solutionwhen four letters are inserted in to four covers (one in each )
A = event that only one letters goes to the proper cover .
B = event that exactly three letters go to the proper covers .
C= event that ll letters go to proper covers and
 then ...... is true
then ...... is true
View Solutionif ` F :R to R f (x) = (5 -x^5 )^(1/5) ` then ` (fof) (x) =………`
View SolutionIF ` A={1,2,3}` then match following subsets of ` A xx A ` properly

View SolutionIf ` f: N toN , f(x) = 2x +3` then …….
View Solution`tan^(-1)(x/y)-"tan"^(-1)(x-y)/(x+y)` is equal to
View Solution`sin{pi/3-sin^(-1)(-1/2)}` is _____
View SolutionIf ` cos^(-1) ((x)/(5)) + "cosec"^(-1) ((5)/(4)) =ppi/2 ` then x=…….
View SolutionIf `x=1/3`, then the value of `cos(2cos^(-1)x+sin^(-1)x)` = ____
View Solutionequal to =………
View Solutionif `[ ( cos alpha, - sin alpha),(sin alpha, cos alpha)]` and `A +A^1 =I` then `alpha =……`
View SolutionIf A is a square matrix such that ` A^2 =A ` then `(I+A)^2 -7A `=……
View SolutionIf A, B are symmetric matrices of same order, then `AB-BA` is a
View SolutionIf the area of the triangle with vertices `(-2,0) ( 0,4) (0,k) ` having 4 sq. units then K=
View SolutionIf `A = [( 1, cos theta , 1),( - cos theta ,1,cos theta ),( -1 , - cos theta ,1)] ` where `0 le theta le 2 pi` then …..
View SolutionIf `D=[(0,i-100 , i-500 ),(100 -i ,0,1000-i),(500 -i,i-1000 ,0)]` then ` |D| =……`
View SolutionIf ` f (x) =[((1-cos kx)/(x^2) : x ne 0),(8 , x=0)]` is continues at ` x=0` , then K=……
View SolutionIf ` e^x + e^y = e^(x+y) ` then ` (dy )/( dx) =……..`
View Solution`(d)/(dx ) ( e^( tan^(-1) x+ cot^(-1) x)) =……. : (x in R)`
View SolutionThe interval in which `y= x^2 . e^(-x) ` is increasing is -----
View SolutionIf the line y = mx + 1 is tangent to the parabola `y^(2) = 4x` then find the value of m.
View SolutionThe normal at the point (2,-2) on the curve ` 3x^2 -y^2 =8` is _____
View SolutionApproximate value of ` (31)^(1/5) ` is ____
View Solution`int_(-1)^(1) log ((2019 -x)/(2019 +x)) dx =………`
View Solution`int_(0)^(1) (dx)/( x+ sqrt(x)) =……`
View Solution`int_(0)^(2) x (2-x ) ^(3/2) dx =……`
View Solution`int sin ( log x) dx =………+c`
View Solution`int(dx)/(sqrt(e^(2x)-1)) =……+c`
View SolutionEvaluate `int_(pi/6)^(pi/3)(dx)/(1+sqrt(tanx))`
View SolutionChoose the correct answers
The value of `int_(0)^(1)tan^(-1)((2x-1)/(1+x-x^(2)))dx` is
View Solution`int_(0)^((2pi)/(3)) sqrt( 1+ cos 2x ) dx=………`
View SolutionArea of the regiion bounded by ` (x^2 )/(16) +(y^2)/(9) = 4 `
View SolutionArea of the region bounded by the curve ` y= sin x and x` -axis `- pi/2 le x le (pi)/(2)` ………
View SolutionSmaller area enclosed by the circle ` x^2 + y^2 = 4 ` and the line ` x+y =2 ` is ……….
View SolutionThe order and degree of differential equation ` xy (d^2 y)/( dx^2) +((dy)/(dx))^2 -y ((dy)/(dx))^3 =0` is …….
View SolutionVerify that the function `y = e^(-3x)` is a solution of the differential equation
`(d^(2)y)/(dx^(2)) + (dy)/(dx) - 6y = 0`
View SolutionThe number of arbitrary constants in the particular solution of a differential equation of third order are …….
View SolutionFind angle `theta` between the vectors `veca=hati+hatj-hatkandvecb=hati-hatj+hatk`.
View SolutionFind `|veca-vecb|`, if two vectors `vecaandvecb` are such that `|veca|=2,|vecb|=3andveca*vecb=4`.
View SolutionThe value of `hati.(hatjxxhatk)+hatj*(hatixxhatk)+hatk*(hatixxhatj)` is
View SolutionIf ` veca and vecb` are two non- zero collinear vectors then …… is correct .
View SolutionIf scalar product of vectors ` veca ` with vectors ` 3 hati - 5 hatk , 2 hati + 7 hatj and hati + hatj + hatk ` are respectively `-1,6,5` then ` veca ` =………
View SolutionFor two non - zero vectors ` veca and vecb | veca + vecb| = | veca|` then vectors ` 2 veca + vecb and vecb ` are …..
View SolutionThe coordinates of the foot of the perpendicular drawn from the origin to the plane `2x -3y +4z -6=0` are ……
View SolutionThe angle `"bet"^(n)` two line `( x+3)/(3) = (y-1)/(5) = (z +3)/(4) and ( x+1)/(1) =(4-y)/(-1) =(z-5)/(2) ` is ……..
View SolutionDistance between the two planes ` 2x + 3y +4z -4=0` and `4x + 6 y + 8z = 12 ` is …….
View SolutionThe objective function of a linear programming problem is ..........
View SolutionIn the question of maximum value of z = 800 x + 12000 y subject to constraints ` 9x +12 y le 180, 3x + 4y le 60 , x + 3y le 30 , x ge 30 , x ge 0, y ge 0 ……..` is not a point of feasible region .
View Solutionin solving the L.P problem " minimize ` z= 6 x + 10 y ` subject to ` x ge 6, y ge 2, 2x +y ge 10 , x ge 0 y ge 0"` redundant constraints are ……..
View SolutionThe mean of the numbers obtained on throwing a die having written ,1 on three faces , 2 on two faces and 5 on one face is ….
View SolutionE,F are independent events and P(E ) `ne O, P(F ) ne O , `then ……. Is false .
View Solutionwhen four letters are inserted in to four covers (one in each )
A = event that only on letters goes to the proper cover .
B = event that exactly three letters go to the proper covers .
C= event that ll letters go to proper covers and
 then ...... is true
then ...... is true
View Solution
PART-A questions and complete solutions for chapter ANNUAL EXAMINATION :SAMPLE PAPER of KUMAR PRAKASHAN KENDRA MATHS (GUJRATI ENGLISH) of Class 12