Complete Solutions to MULTIPLE CHOICE QUESTIONS . (HIGHER LEVEL) of chapter SURFACE TENSION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | SURFACE TENSION |
| MARVEL | MULTIPLE CHOICE QUESTIONS . (HIGHER LEVEL) |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
A mercury drop of radius R splits up into 1000 droplets of equal radii . The change in the surface area is
View SolutionA film of water is formed between two straight parallel wires of length 10 cm each separated by `0.5cm` If their separation is increased by `1mm` while still maintaining their parallelism, how much work will have to be done (Surface tension of water `=7.2xx10^-2(N)/(m)`)
View SolutionTwo small drops of mercury, each of radius `R`, coalesce to form a single large drop. The ratio of the total surface energies before and after the change is
View SolutionA water drop of radius R is split into n smaller drops , each of radius r. If T is the surface tension of water ,then the work done in the process is
View SolutionA metallic wire of diameter `d` is lying horizontally o the surface of water. The maximum length of wire so that is may not sink will be
View SolutionWhich is the fundamental unit that has the same power in the dimensional formulae of surface tension and coefficient of vicosity ?
View SolutionA wooden stick 2m long is floating on the surface of water. The surface tension of water 0.07 N/m. By putting soap solution on one side of the sticks the surface tension is reduced to 0.06 N/m. The net force on the stick will be
View SolutionA thin metal disc of radius `r` floats on water surface and bends the surface downwards along the perimeter making an angle `theta` with vertical edge of the disc of the disc. If the disc dispplaces a weight of water `W` and surface tension of water is `T`, then the weight of metal disc is
View SolutionA large number of liquid drops each of radius 'a' coalesce to form a single spherical drop of radish b. The energy released in the process is converted into kinetic energy of the big drops formed. The speed of big drop will be
View SolutionA certain number of spherical drops of a liquid of radius `r` coalesce to form a single drop of radius `R` and volume `V`. If `T` is the surface tension of the liquid, then
View SolutionOne end of a glass capillary tube with a radius `r=0.05cm` is immersed into water to a depth of `h=2cm`.Excess pressure required to blow an air bubble out of the lower end of the tube will be `(S.T` of water `=70"dyne"//cm`).Take `g=980cm//s^(2)`.
View SolutionA capillary tube having 1 mm and 2.5 mm as internal an external radii hangs vertically from the arm of a balance . The lower end of the tube just touches a liquid of surface tension 40 dyne/cm .If the touches a liquid of surface tension 40 dyne/cm . If the angle of contact is zero, then the change in weight due to surface tension is
View SolutionIf a capillary tube of radius 1 mm is immersed in water , the mass of water rising in the capillary tube is M . If the radius tube is doubled , then the mass of water , that rises in the capillary tube will be
View SolutionPure water rises through a height h in a capillary tube of internal radius (r ). If T is the S.T of water , then the pressure diference between water level in the container and the lowest point of the concave meniscus is
View SolutionFor which of the two pairs, the angle of contact is same
View SolutionA capillary tube of radius r is dipped in a liquid of density `rho ` and surface tension S. if the angle of contanct is `theta`, the pressure difference between the two surface in the beaker and the capillary ?
View SolutionWater rises upto a height h in a capillary tube of radius r. What is the network done in this process if the density of water is `rho` ?
View SolutionWater rises in a capillary tube to a certain height such that the upward force due to surface tension is balanced by `75xx10^-4` newton force due to the weight of the liquid. If the surface tension of water is `6xx`10^-2` newton/metre the inner circumference of the capillary must be:
View SolutionWater rises to a height of `10 cm` in a capillary tube and mercury falls to a depth of `3.42 cm` in the same capillary tube. If the density of mercury is `13.6 g//c.c.` and the angles of contact for mercury and for water are `135^@` and `0^@`, respectively, the ratio of surface tension for water and mercury is
View SolutionThe height upto which water will rise in a capillary tube will be:
View SolutionThe lower end of a capillary tube is dipped in water. Water rises to a height of 8 cm. The tube is then broken at a height of 6 cm. The height of water colume and angle of contact will be
View SolutionA capillary tube of radius r is immersed in water and water rises in to a height h. The mass of water in the capillary tube is 5g. Another capillary tube of radius 2 r is immersed in water. The mass of water that will rise in this tube is
View SolutionThe lower end of a capillary tube of radius r cm is placed vertically in water. What is the heat evolved if water rise upto a height h in the capillary tube ?
View SolutionTwo narrow bores of diameters 3.0mm and 6.0 mm are joined together to form a U-shaped tube open at both ends. If th U-tube contains water, what is the difference in its levels in the two limbs of the tube? Surface tension of water at the temperature of the experiment is `7.3xx10^(-2)Nm^(-1)`. Take the angle of contact to be zero. and density of water to be `1.0xx10^(3)kg//m^(3)`.
`(g=9.8 ms^(-2))`
View SolutionIf h is the rise of water in a capillary tube of radius r, then the work done by the force of surface tension is
`(rho ` is density g = `("acc")^(n)` due to gravity )
View SolutionLiquid rises to a height of 2 cm in a capillary tube and the angle of contact between the solid and the liquid is zero. If the tube is depressed more now so that top of capillary is only 1 cm above the liquid, then the apparent angle of contact between the solid and the liquid is
View SolutionWater rises upto a height x in capillary tube immersed vertically in water . When this whole arrangement is taken to a depth d in a mine , the water level rises upto a height y . If R is the radius of the earth , then the ration `x/y` is given by
View SolutionIf two soap bubbles of equal radii r coalesce then the radius of curvature of interface between two bubbles will be
View SolutionThe pressure inside two soap bubbles is 1.01 and 1.02 atmosphere. The ration of their respective volumes is
View SolutionA soap bubble assumes a spherical surface . Which one of the following statement is wrong ?
View Solution`W_(1)` is the work done in blowing a soap bubble of radius r from a soap solution at room temperature . The soap solution is then heated and second soap bubble of radius 2r is blown from the heated solution . If `W_(2)` is the work done in forming the second bubble , then
View Solution_E01_021_Q01.png)
The adjoining diagram shows three soap bubbles, A , B and C prepared by blowing the capillary tube fitted with stop cocks S, `S_(1),S_(2)` and `S_(3)` with stop cock S closed and stop cocks `S_(1),S_(2)` and `S_(3)` opened-
View SolutionA glass tube of uniform internal radius `(r)` has a valve separating the two identical ends. Initially, the valve is in a tightly closed position. End `1` has a hemispherical soap bubble of radius `r`. End `2` has sub-hemispherical soap bubble as shown in figure. Just after opening the valve.
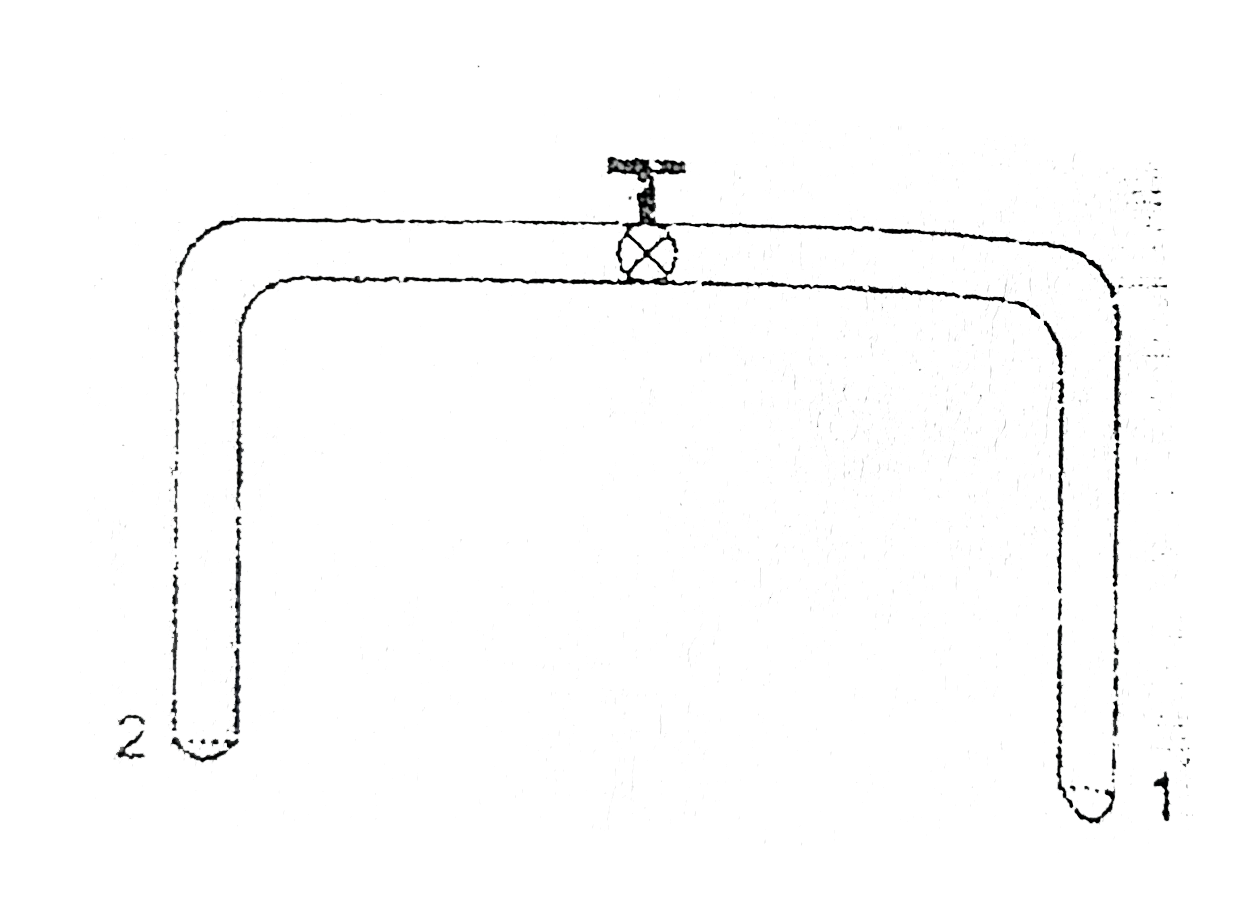
View SolutionThe surface tension and vapour pressure of water at `20^(@)C` is `7.28xx10^(-2)Nm^(-1)` and `2.33xx10^(3)Pa`, respectively. What is the radius of the smallest spherical water droplet which can form without evaporating at `20^(@)C`?
View SolutionIn air , a charged soap bubble of radius 'r' is in equilibrium having outside and inside pressures being equal . The charge on the drop is (`epsilon_(0)` = permittivity of free space , T = surface tension of soap solution )
View Solution
MULTIPLE CHOICE QUESTIONS . (HIGHER LEVEL) questions and complete solutions for chapter SURFACE TENSION of MARVEL PHYSICS (HINGLISH) of Class 12