Complete Solutions to EXAMPLE of chapter RELATIONS AND FUNCTIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | RELATIONS AND FUNCTIONS |
| MAXIMUM PUBLICATION | EXAMPLE |
| Number Of Questions | 100 |
| Academic Year | 2023-24 |
Show that the relation R in the set is given by `A={x in Z,0lexle12}`
`R={(a,b):a-b` is a multiple of 4} is an equivalence relation.
View SolutionLet R be a Relation in the set
`A={1,2,3,4,5,6}` define as
`R={(x,y):y=2x-1}`
Write R in roster form and find it's domain and range
View SolutionLet R be a Relation in the set
`A={1,2,3,4,5,6}` define as
`R={(x,y):y=2x-1}`
Is R an equivalance relation? Justify.
View SolutionLet `f:R-{(-4)/3}toR` be a function defined b `f(x)=frac(4x)(3x+4),xne-4/3`. Show that `f^-1(y)=frac(4y)(4-3y)`
View SolutionLet '`ast`' is a binary operation on the set Q of rational numbers as follows,
`aastb=a-b`
Check whether the binary operation are commutative and associative. Also find the identify element if exists.
View SolutionLet '`ast`' is a binary operation on the set Q of rational numbers as follows,
`aastb=a+ab`
Check whether the binary operation are commutative and associative. Also find the identify element if exists.
View SolutionLet '`ast`' is a binary operation on the set Q of rational numbers as follows,
`aastb=a^2+b^2`
Check whether the binary operation are commutative and associative. Also find the identify element if exists.
View SolutionLet '`ast`' is a binary operation on the set Q of rational numbers as follows,
`aastb=(a-b)^2`
Check whether the binary operation are commutative and associative. Also find the identify element if exists.
View SolutionShow that the relation R on the set of natural numbers defined as `R:{(x,y):y-x`is a multiple of 2} is an equivalance relation
View SolutionConsider `f:[3,infty)to[1,infty)` given by
`f(x)=x^2-6x+10`. Find `f^-1`
View Solution`ast`' be a binary operation on `NxxN` defined as `(a,b)ast(c,d)=(ac,bd)`
Show that `ast` is commutative.
View Solution`ast`' be a binary operation on `NxxN` defined as `(a,b)ast(c,d)=(ac,bd)`
Find the identity element of `ast` if any
View Solution`ast`' be a binary operation on `NxxN` defined as `(a,b)ast(c,d)=(ac,bd)`
Write an element of `NxxN`which has an inverse.
View SolutionShow that the relation R in the set R of Real numbers defined as `R={(a,b):aleb^2}` is neither reflexive, nor symmetric, nor transitive.
View SolutionConsider `f:R^+to[-5, infty)` given by
`f(x)=9x^2+6x-5`. Show that f is invertible with `f^-1(y)=frac(sqrt(y+6)-1)(3)`
View SolutionLet `A=R-{3}` and `B=R-{1}` consider the function `f:AtoB` defined by `f(x)=frac(x-2)(x-3)`. Is f one-one and onto? Justify your answer.
View SolutionLet `f:R-{(-4)/3}toR` be a function defined b `f(x)=frac(4x)(3x+4),xne-4/3`. Show that `f^-1(y)=frac(4y)(4-3y)`
View SolutionThe relation R defined in the set
`A={-1,0,1}` as
`R={(a,b):a=b^2}`
Chech whether R is reflexive, symmetric and transitive.
View SolutionThe relation R defined in the set
`A={-1,0,1}` as
`R={(a,b):a=b^2}`
Is R an equivalance relation
View SolutionLet `A={1,2,3}`. Give an example of a relation on A which is
Symmetric but neither reflexive nor transitive.
View SolutionLet `A={1,2,3}`. Give an example of a relation on A which is
Transitive but neither reflexive nor symmetric.
View SolutionFind `fog` and `gof` if
`f(x)=abs(x)` and `g(x)=abs(3x+4)`
View SolutionFind `fog` and `gof` if
`f(x)=16x^4` and `g(x)=x^(1/4)`
View SolutionConsider the binary operation `ast` : `QtoQ` where Q is the set of rational numbers as defined as `aastb=a+b-ab`
Find `2ast3`
View SolutionConsider the binary operation `ast` : `QtoQ` where Q is the set of rational numbers as defined as `aastb=a+b-ab`
Is identity for `ast` exist? If yes, find the identity element.
View SolutionConsider the binary operation `ast` : `QtoQ` where Q is the set of rational numbers as defined as `aastb=a+b-ab`
Are elements of Q invertible? Is yes, find the inverse of an element in Q
View Solution`ast`' is a binary operation on R defined as
`aastb=2ab`
Determine whether `ast` is commutative and associative
View Solution`ast`' is a binary operation on R defined as
`aastb=2ab`
Find the identity element if exists.
View Solution`ast`' is a binary operation on R defined as
`aastb=2ab`
Find the inverse element, if exists
View SolutionCheck if the following function satisfies the condition `f^-1nef`.
`f:R-{0}toR-{0},f(x)=1/x`
View SolutionWhich of the following satisfies the condition `f^-1nef`.
`f:RtoR,f(x)=-x`
View SolutionWhich of the following satisfies the condition `f^-1nef`.
`f:R-{-1}toR-{1},f(x)=frac(x)(x+1)`
View SolutionWhich of the following satisfies the condition `f^-1nef`.
`f:R-{2}toR-{2},f(x)=frac(2x-3)(x-2)`
View SolutionIf `f:RtoR` is a function defined by
`f(x)=3x-2`
Show that `f` is one-one.
View SolutionLet `A=NtimesN` and '`ast`' be the binary operation. On A defined by `(a,b)ast(c,d)=(a+c,b+d)`. Show that '`ast`' is commutative and associative. Find the identity for '`ast`' on A if any.
View SolutionIf `f(x)=frac(4x+3)(6x-4),xne2/3`.
Show that `fof(x)=x`, for all `xne2/3`.
View SolutionIf `f(x)=frac(4x+3)(6x-4),xne2/3`.
What is the inverse of '`f`'?
View SolutionLet be a function defined by `f(x) =sqrtx` is a function if it defined from f : N`to`N`
View SolutionLet be a function defined by `f(x) =sqrtx` is a function if it defined from
`f:RtoR`
View SolutionLet be a function defined by `f(x) =sqrtx` is a function if it defined from
`f:RtoR^+`
View SolutionLet be a function defined by `f(x) =sqrtx` is a function if it defined from
`f:R^+toR^+`
View SolutionCheck the injectivity and surjectivity of the following functions
`f:NtoN` defined by `f(x)=x^3`
View SolutionCheck the injectivity and surjectivity of the following functions
`f:RtoR` given by `f(x)=[x]`
View SolutionConsider the function `f(x)=frac(x+1)(x-1),xne1`
`fof(2)` =__
View SolutionConsider the function `f(x)=frac(x+1)(x-1),xne1`
what is the inverse of `f`?
View SolutionConsider the function `f(x)=frac(x+1)(x-1),xne1`
`f(3)+f^-1(3)`
View SolutionShow that the function `f:RtoR` defined by `f(x)=2x-3` is one-one and onto. Find `f^-1`
View SolutionWhich of the following figure represents the graph of a function on R which is onto but not one-one.
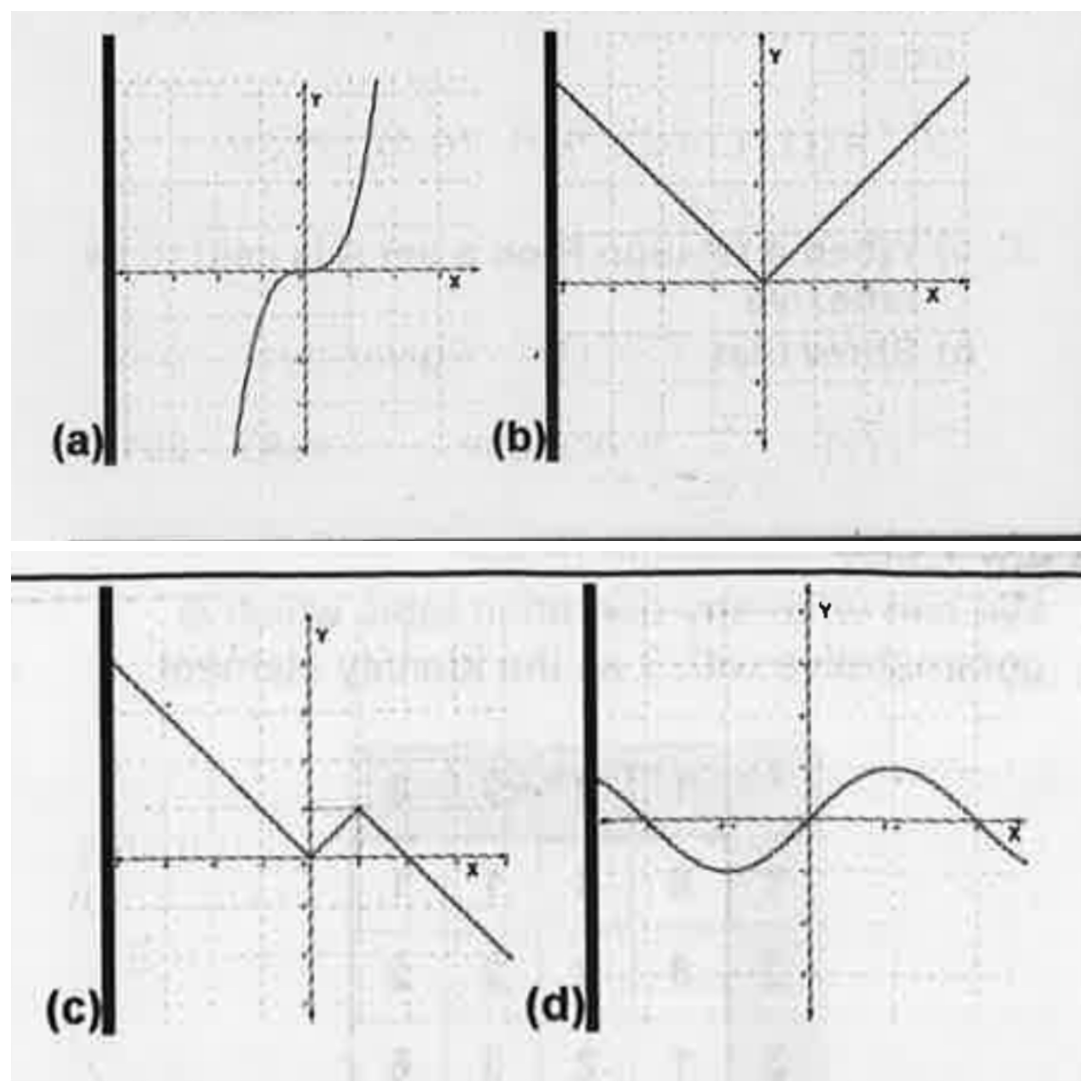
View SolutionWrite a function on R which is onto but not one-one.
View Solution`A={1,2,3,4,6},ast` is a binary operation on A is defined as `aastb=HCF` of a and b.
Represent `ast` with the help of an operation table.
View Solution`A={1,2,3,4,6},ast` is a binary operation on A is defined as `aastb=HCF` of a and b.
Find the identity element.
View Solution`A={1,2,3,4,6},ast` is a binary operation on A is defined as `aastb=HCF` of a and b. Draw its operation table.
Write a commutative binary operation on A with 3 as the identity element. ( Hint: Operation table may be used.
View SolutionLet `ast` be a binary operation on the set of all real numbers R defined by `aastb=a+b+a^2b` for a, b R.
Find `2ast6` and `6ast2`.
View SolutionLet `ast` be a binary operation on the set of all real numbers R defined by `aastb=a+b+a^2b` for a, b belongs to R.
Prove that `ast` is neither commutative nor associative.
View SolutionLet `ast` be a binary operation on the set of all real numbers R defined by `aastb=a+b+a^2b` for a, b R.
Find the identity elements in R if exists.
View SolutionConsider `f:{3,4,5,6}to{8,10,12,13,14}` and `f={(3,8),(4,10),(5,12),(6,14)}`. State whether `f` has inverse? Give reason.
View SolutionConsider `f:RtoR` given by
`f(x)=3x+2` show that `f` is invertible. Find the inverse of `f`
View SolutionConsider the set `A={1,2,3,4,5}`, and `B={1,4,9,16,25}` and a function `f:AtoB` defined by `f(1)=1`, `f(2)=4`, `f(3)=9`, `f(4)=16 ` and `f(5)=25`.
Show that `f` is one-to-one.
View SolutionConsider the set `A={1,2,3,4,5}`, and `B={1,4,9,16,25}` and a function `f:AtoB` defined by `f(1)=1`, `f(2)=4`, `f(3)=9`, `f(4)=16 ` and `f(5)=25`.
Show that `f` is onto.
View SolutionConsider the set `A={1,2,3,4,5}`, and `B={1,4,9,16,25}` and a function `f:AtoB` defined by `f(1)=1`, `f(2)=4`, `f(3)=9`, `f(4)=16 ` and `f(5)=25`.
Does `f^-1` exists? Explain.
View SolutionWhen a relation R on a set A is said to be reflexive
View SolutionShow that `f:[-1,1]toR` given by
`f(x)=frac(x)(x+2)` is one-one.
View SolutionThe function `f:NtoN` given by `f(x)=2x`
View SolutionFind `gof(x)`, if `f(x)=8x^3` and `g(x)=x^(1/3)`
View SolutionLet `ast` be an operation such that `aastb=LCM` of a and b defined on the set `A={1,2,3,4,5}`. Is `ast` a binary operation? Justify your answer.
View Solution`f:{1,2,3,4}to{5}` defined by
`f={(1,5),(2,5),(3,5),(4,5)}`.
Does the function is invertible?
View Solution`A=R-{7/5},B=R-{3/5}`
`f:AtoB` defined by `f(x)=frac(3x+4)(5x-7)`
`g:BtoA` defined by `g(y)=frac(7y+4)(5y-3)`
Find `gof`
View SolutionLet `A=NtimesN`, N-set of natural numbers and '`ast`' be a binary operation on A defined by `(a,b)ast(c,d)=(ac-bd,ad+bc)`.
Show that '`ast`' is commutative on A.
View SolutionLet N be the set of Natural numbers. Consider the function `f:NtoN` defined by `f(x)=x+1, x in N`
Prove that `f` is not onto.
View SolutionLet N be the set of Natural numbers. Consider the function `f:NtoN` defined by `f(x)=x+1, x in N`
If `g(x)={(x-1,,,,x>1),(1,,,,x=1):}` then find `gof`
View SolutionLet N be the set of Natural numbers. Consider the function `f:NtoN` defined by `f(x)=x+1, x in N`
Check whether `f` is an onto function.
View SolutionGive a relation on a set `A={1,2,3,4}` which is reflexive, symmetric and not transitive.
View SolutionShow that `f:[-1,1]toR` given by `f(x)=frac(x)(x+2)` is one-one.
View SolutionLet '`ast`' be a binary operation on `Q^+` defined by '`aastb=frac(ab)(6)`'. Find the inverse of 9 with respect to '`ast`'.
View Solution`ast:Rto R` given by `aastb=3a^2-b`
Find the value of `2ast3` . Is '`ast`' commutative? Justify your answer.
View Solution`f:RtoR` is defined by `f(x)=x^2-3x+2` Find `fof(x)` and `fof(1)`.
View SolutionConsider `f:RtoR` given by `f(x)=5x+2`
Show that `f` is one-one.
View SolutionConsider `f:RtoR` given by `f(x)=5x+2`
Is `f` invertible? Justify your answer.
View SolutionLet `ast` be a binary operation on N defined by `aastb=HCF` of a and b
Is `ast` commutative?
View SolutionLet `ast` be a binary operation on N defined by `aastb=HCF` of a and b
Is `ast` associative?
View SolutionLet `f:RtoR` be given by `f(x)=frac(2x+1)(3)` find
`fof` and show that `f` is invertible.
View SolutionFind the identity element of the binary operation `ast` on N defined by `aastb=ab^2`.
View SolutionWhat is the minimum number of pairs to form a non - zero reflexive relation on a set of n elements?
View SolutionOn the set R of real numbers, S is a relation defined as
`S={(x,y)//x inR,y inR,x+y=xy}`. Find `a inR` such that 'a' is never the first element of an ordered pair in S. Also find `b inR` such that 'b' is never the second element of an ordered pair in S.
View SolutionConsider the function `f(x)=frac(3x+4)(x-2),xne2`. Find a function on a suitable domain such that `gof(x)=x=fog(X)`.
View SolutionIf `f:RtoR` and `g:RtoR` defined by `f(x)=x^2` and `g(x)=x+1`, then `gof(x)` is
View SolutionConsider the function `f:NtoN`, given by `f(x)=x^3`. Show that the function '`f`' is injective but not surjective.
View SolutionThe given table shows an operation `ast`on `A={p,q}` (Figure)
Is `ast` a binary operation?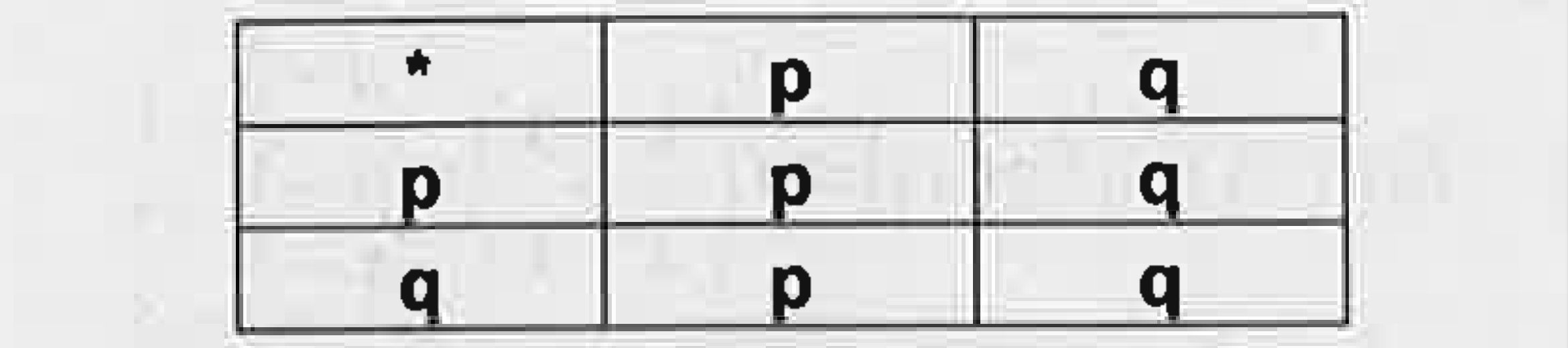
View SolutionThe given table shows an operation `ast`on `A={p,q}` (Figure)
Is `ast` commutative? Give reason.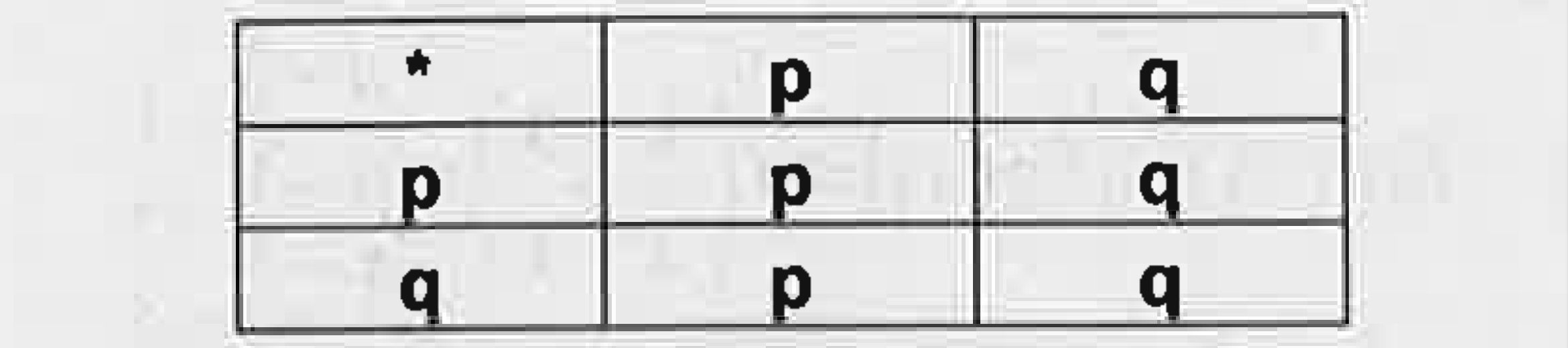
View SolutionLet R be relation defined on `A={1,2,3}` by `R={(1,3),(3,1),(2,2)}` is
View SolutionFind `fog` and `gof` if `f(x)=abs(x+1)` and `g(x)=2x-1`
View SolutionLet `ast` be a binary operation defined on `NtimesN` by `(a,b)ast(c,d)=(a+c,b+d)`
Find the identity element for `ast` if it exists.
View SolutionIf `R={(x,y):x,y inZ,x-y inZ}`, then the relation R is
View SolutionLet `ast` be a binary operation on the set Q of rational numbers by `aastb=2a+b`. Find `2ast(3ast4)` and `(2ast3)ast4`.
View SolutionLet `f:RtoR,g:RtoR` be two one-one functions. Check whether `gof` is one-one or not.
View SolutionA function `f:XtoY` is onto if range of `f`=……….
View SolutionLet `f:{1,3,4}to{3,4,5}` and `g:{3,4,5}to{6,8,10}` be functions defined by
`f(1)=3,f(3)=4,f(4)=5`,
`g(3)=6,g(4)=8,g(5)=8`,then `(gof)(3)`=……...
View SolutionLet Q be the set of Rational numbers and '`ast`' be the binary operation on Q defined by '`aastb=frac(ab)(4)`' for all a,b in Q.
What is the identity element of '`ast`' on Q?
View SolutionLet Q be the set of Rational numbers and '`ast`' be the binary operation on Q defined by '`aastb=frac(ab)(4)`' for all a,b in Q.
Find the inverse element of '`ast`' on Q.
View SolutionShow that `aast(bastc)=(aastb)astc,AAa,b,c inQ`.
View Solution
EXAMPLE questions and complete solutions for chapter RELATIONS AND FUNCTIONS of MAXIMUM PUBLICATION of Class 12