Complete Solutions to EXAMPLE of chapter INVERSE TRIGNOMETRY of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | INVERSE TRIGNOMETRY |
| MAXIMUM PUBLICATION | EXAMPLE |
| Number Of Questions | 42 |
| Academic Year | 2023-24 |
prove the following
`sin^(-1)frac(8)(17)+sin^-1frac(3)(5)=sin^(-1)frac(77)(85)`
View Solutionprove the following
`tan^(-1)frac(63)(16)=sin^(-1)frac(5)(13)+cos^(-1)frac(3)(5)`
View Solutionprove the following
`cot^(-1)9+cosec^(-1)frac(sqrt41)(4)=pi/4`
View SolutionFind the value of
`tan^(-1)(frac(1)(sqrt3))+cos^(-1)(-frac(sqrt3)(2))+sin^(-1)(frac(1)(2))`
View SolutionIf `tan^-1x+tan^-1y+tan^-1z=pi`,show that
`x+y+z=xyz`
View SolutionMatch the following
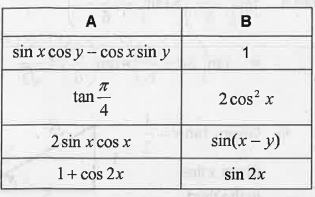
View SolutionSolve `2tan^-1(cosx)=tan^-1(2cosx)`
View SolutionSolve the following
`2tan^-1(cosx)=tan^-1(2cosecx)`
View SolutionSolve the following
`tan^(-1)2x+tan^(-1)3x=pi/4`
View SolutionSolve `2tan^-1(cosx)=tan^-1(2cosx)`
View SolutionProve that `sin^-1frac12(13)+cos^-1frac4(5)+tan^-1frac63(16)=pi`
View SolutionFind the principal value of `sec^-1(-frac(2)(sqrt3))`
View SolutionIf `sin(sin^-1(frac(1)(5))+cos^-1(x))=1`
View SolutionSolve the following
`tan^-1(frac(1-x)(1+x))=1/2tan^-1x`
View SolutionSolve the following
`sin^-1x+sin^-1 2x=pi/3`
View SolutionChoose the correct answer from the
bracket.
`cos(tan^-1x),|x|<1` is equal to
View SolutionProve that `sin^-1frac(4)(5)+sin^-1frac(5)(13)=sin^-1frac(63)(65)`
View SolutionIn which quadrants are the graph of
`cos^-1(x)` lies,`x in [-1,1]`
View SolutionIf `cos^-1x+cos^-1y=pi/3`,then
`sin^-1x+sin^-1y=………..`
View Solutionif `tan^-1x+tan^-1y=pi/4` then prove that
`x+y+xy=1`
View Solution`sin(tan^-1(1))` is equal to
View Solutionif `x in(o,pi/2)`,show that
`cot^-1(frac(sqrt(1+sinx)+sqrt(1-sinx))(sqrt(1+sinx)-sqrt(1-sinx)))=x/2`
View Solutionshow that `sin^-1frac(3)(5)-sin^-1frac(8)(17)=cos^-1frac(84)(85)`
View SolutionChoose the correct answer from the
bracket.
if `cos^-1x=y`,then y is equal to
View Solutionfind the value of `cos^-1cos((7pi)/3)`
View SolutionSolve for x if,`tan^-1(frac(1+x)(1-x))=2tan^-1x`
View SolutionFind the principal value of `cos^-1(frac(sqrt3)(2))`
View SolutionProve that `2 sin^-1(frac(3)(5))=tan^-1(frac(24)(7))`
View SolutionProve `2tan^-1frac(1)(2)+tan^-1frac(1)(7)=tan^-1frac(31)(17)`
View SolutionFind the principal value of `cos^-1(-frac(1)(2))`
View SolutionShow that `tan^-1(frac(cosx)(1-sinx))=pi/4+x/2`
View SolutionThe principal value of `cos^-1(-frac(1)(2))`
View SolutionExpress `tan^-1(frac(cosx)(1-sinx))` in the simplest
Form.
View SolutionWrite the principal value of `sin^-1(frac(1)(2))`
View Solutionshow that
`sin^-1(frac(3)(5))-sin^-1(frac(8)(17))=cos^-1(frac(84)(85))`
View SolutionThe principal value of `tan^-1(-1)` is
`[pi/4,-pi/4,pi-pi/4,pi+pi/4]`
View SolutionIf `tan^-1(frac(x-1)(x-2))+tan^-1(frac(x+1)(x+2))=pi/4`,
then find the value of x
View SolutionGive an expression for `tan(x+y)`
View SolutionProve that `xy<1`,
`tan^-1x+tan^-1y=tan^-1(frac(x+y)(1-xy))`
View SolutionUsing the above result prove that
`tan^-1(frac(1)(2))+tan^-1(frac(1)(3))=pi/4`
View SolutionShow that
`tan^-1frac(1)(5)+tan^-1frac(1)(7)+tan^-1frac(1)(3)+tan^-1frac(1)(8)=pi/4`
View SolutionGiven that `cot3theta=frac(3cot^2theta-1)(cot^3theta-3cottheta)`,show
that `cot^-1frac(3x^2-1)(x^3-3x)`,`|x| < sqrt3` is `3cot^-1x`
View Solution
EXAMPLE questions and complete solutions for chapter INVERSE TRIGNOMETRY of MAXIMUM PUBLICATION of Class 12