Complete Solutions to EXERCISES LEVEL-2 (Single Correct Answer Type Questions) of chapter PROGRESSIONS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROGRESSIONS |
| MCGROW HILL PUBLICATION | EXERCISES LEVEL-2 (Single Correct Answer Type Questions) |
| Number Of Questions | 11 |
| Academic Year | 2023-24 |
The rth term of the series `2(1/2)+1(7/13)+1(1/9)+20/23+.....` is
View SolutionH.M. between `(1)/(28) and (1)/(10)` is
View SolutionIf `a\ (1/b+1/c),\ b(1/c+1/a),\ c(1/a+1/b)` are in A.P. prove that `a ,\ b ,\ c` are in A.P.
View SolutionIf `a_1,a_2,a_3,...a_n` are in A.P with common difference `d` then find the sum of the following series `sind(coseca_1coseca_2+coseca_2coseca_3+....coseca_(n-1)coseca_n)`
View SolutionIf `H_n=1+1/2+1/3+.... +1/n,` then value of `1+3/2+5/3+....+(2n-1)/n` is
View SolutionIf `a,b,c` are in H.P., then prove that `a/(b+c-a), b/(a+b-c), c/(a+b-c)` are in H.P.
View SolutionSum of all the terms in the nth row of the triangle
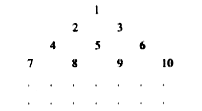 is
is
View SolutionLet `alpha` and `beta` be two positive real numbers. Suppose `A_1, A_2` are two arithmetic means; `G_1 ,G_2` are tow geometrie means and `H_1 H_2` are two harmonic means between `alpha` and `beta`, then
View SolutionLet `a(n) =1-(1)/(2)+(1)/(3)+…+(-1)^(n-1)(1)/(n)," then "(1)/(n+1)+(1)/(n+2)+…+(1)/(2n)` is equal to
View SolutionIn Fig. 8.3 each square is filled with the arithmetic mean of the numbers in the boxes sharing a side with it. If the first square is filled with 5, then value of x is

View SolutionLet a,b, and c be three real numbers satistying `[a,b,c][(1,9,7),(8,2,7),(7,3,7)]=[0,0,0]`Let b=6, with a and c satisfying (E). If alpha and beta are the roots of the quadratic equation `ax^2+bx+c=0 then sum_(n=0)^oo (1/alpha+1/beta)^n` is (A) 6 (B) 7 (C) `6/7` (D) oo
View Solution
EXERCISES LEVEL-2 (Single Correct Answer Type Questions) questions and complete solutions for chapter PROGRESSIONS of MCGROW HILL EDUCATION MATHS (HINGLISH) of Class 12
All Exercises from MCGROW HILL PUBLICATION Class 12 MATHS PROGRESSIONS
- EXERCISES (Numerical Answer Type Questions)
- EXERCISES CONCEPT-BASED (Single Correct Answer Type Questions)
- EXERCISES LEVEL-1 (Single Correct Answer Type Questions)
- EXERCISES LEVEL-2 (Single Correct Answer Type Questions)
- Questions from Previous Years. AIEEE/JEE Main Papers
- Questions from Previous Years. B-Architecture Entrance Examination Papers
- SOLVED EXAMPLES CONCEPT -BASED (SINGLE CORRECT ANSWER TYPE QUESTIONS)
- SOLVED EXAMPLES LEVEL (Numerical Answer Type Questions)
- SOLVED EXAMPLES LEVEL -1 (SINGLE CORRECT ANSWER TYPE QUESTIONS)
- SOLVED EXAMPLES LEVEL -2 (SINGLE CORRECT ANSWER TYPE QUESTIONS)