Complete Solutions to Paper 2 (Mathematics ) of chapter PRACTICE SET 02 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PRACTICE SET 02 |
| MHTCET PRACTICE PAPER | Paper 2 (Mathematics ) |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
`intsqrt(1+sin((x)/(4)))dx` is equal to
View SolutionThe angle between two planes `x+2y+2z=3` and `-5x+3y+4z=9` is
View SolutionWhat must be the matrix X , is `2X+[[1,2],[3,4]]=[[3,8],[7,2]]?`
View SolutionLet truth values of p be F and q be T. then , truth vlaue of `~(~p v q)` is
View Solution`(d)/(dx)[sin^(-1)(xsqrt(1 - x)- sqrt(x)sqrt(1 - x^(2)))]` is equal to
View SolutionA vertex of common graph of inequalities `2x+y ge 2` and `x-y le 3` , is
View SolutionThe derivative of `sin^2x` with respect to `cos^2x` is equal to....
View SolutionIf `A xx(BxxC)=Bxx(CxxA)` and [ABC] = 0, then `A xx(BxxC)` is equal to
View Solution`int cos (log_e x)dx` is equal to
View SolutionThe switching function ffor switching network is
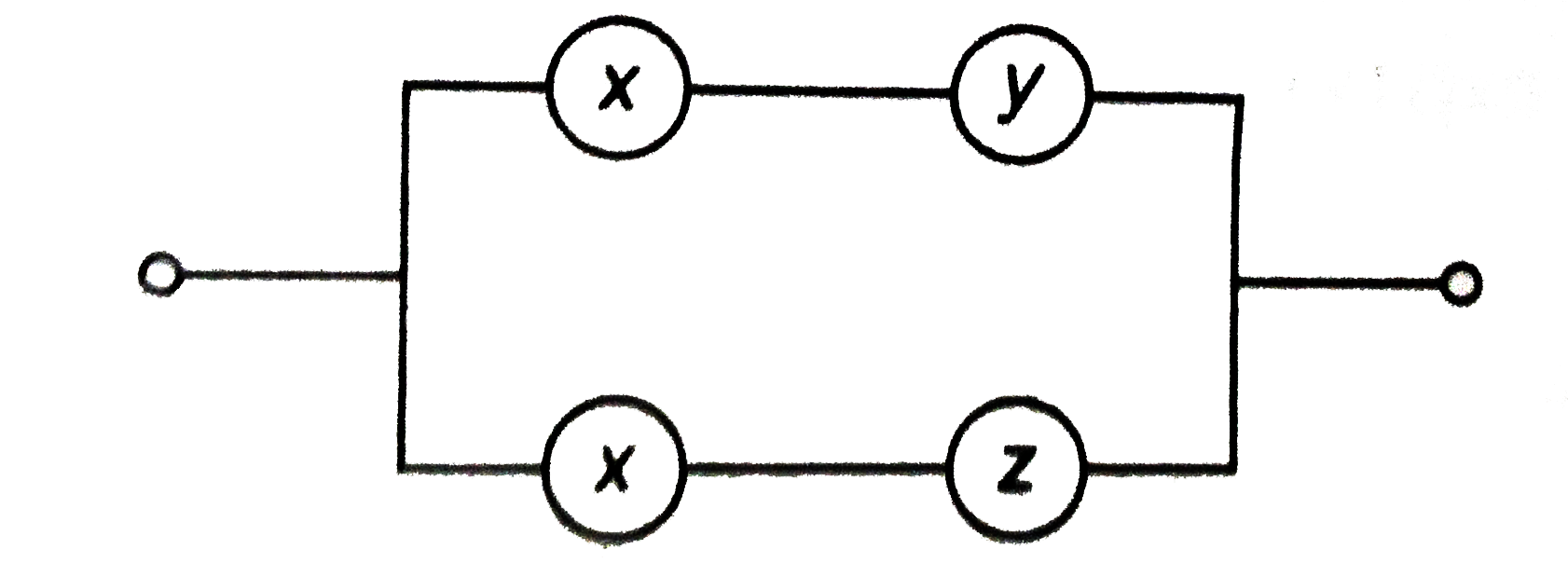
View SolutionThe difference of the slopes of the lines given by the equation `x^2(sec^2theta-sin^2theta)-2xytantheta+y^2sin^2theta=0` is.
View SolutionLet `p ^^ (q vv r)=(p ^^ q) vv(p ^^ r)`. Then this law is known as
View SolutionIf `|a|=|b|=1 and |a+b|=sqrt(3)`, then the value of `(3a-4b)(2b+5b)` is
View SolutionA particle moves in a straight line so that `s=sqrt(t)`, then its acceleration is proportional to
View SolutionLe t `f(x) ={{:((sinpix)/(5x)",",xne0),(" "k",",x=0):}` if (x) is continous at `x=0`, then k is equal to
View Solution`lim_(xto0)(log_e(1+x))/(3^x-1)` is equal to
View SolutionThe differential equation for which `sin^(-1) x + sin^(-1) y = c` is given by
View SolutionThe probability that in a family of 5 members,exactly two members have birthday on sunday is:-
View SolutionThe solution of `y'-y = 1 , y(0)=-1` is given by `y(x) =`
View SolutionThere is a group of 265 persons who like either singing or dancing or painting. In this group 200 like singing, 110 like dancing and 55 like painting. If 60 persons like both singing and dancing, 30 like both singing and painting and10 like all three activities, then the number of persons who like only dancing and painting is
View SolutionLet R be a relation on N defined by `R = {(x, y): 2x + y = 10}`, then domain of R is
View SolutionThe period of `sin^2theta` is
View SolutionSix numbers are in AP such that their sum is 3 . The first term is 4 times the third term . Then , the fifth term is
View SolutionThe value of k such that the lines `2x-3y+k=0,3x-4y-13=0` and `8x-11y-33=0` are concurrent is
View SolutionIf one end of a diameter of the circle `x^(2)+y^(2)-4x-6y+11=0` is (3, 4), then find the coordinates of the other end of the diameter.
View SolutionA man and his wife appear for an interview for two posts. The probability of the man's slection is 1/5 and that of his wife's slection is `1//7`. The probability that atleast one of them is selected , is
View SolutionThe area bounded by the curve `x=3y^2-9` and the line `x=0,y=0 and y=1` is
View SolutionThe vector `hat(i)+xhat(j)+3hat(k)` is rotated through an angle `theta` and doubled in magnitude then it becomes `4hat(i)+(4x-2)hat(j)+2hat(k)`. The value of x is
View SolutionIf `lim_(xto oo) (1+(a)/(x)+(b)/(x^2))^(2x)=e^2`, then
View SolutionFor the function `f(x)=xe^x,` the point
View Solution`intx logx dx` is equal to
View SolutionA die is thrown 100 times. Getting an even number is considered a successes. Find the mean and variance of successes.
View SolutionThe angle between the line `(x-3)/(2)=(y-1)/(1)=(z+4)/(-2)` and the plane, `x+y+z+5=0` is
View SolutionIf in a triangle `ABC, 3 sin A = 6 sin B=2sqrt3sin C`, then the angle A is
View SolutionThe differential equation of all circles which passes through the origin and whose centers lie on Y-axis is
View SolutionThe value of the integral `int _(0)^(pi//2)(sin^100x-cos^100x)dx` is
View SolutionIf `8f(x)+6f(1/x)=x+5` and `y=x^2(f(x),` then `(dy)/(dx)` at `x=-1` is equal to 0 (b) `1/(14)` (c) `-1/4` (d) None of these
View SolutionIf `2a+3b+6c=0,` then prove that at least one root of the equation `a x^2+b x+c=0` lies in the interval (0,1).
View Solution`int(sin^(-1)x)/(sqrt(1-x^(2)))dx` is equal to
Where, C is an arbitrary constant.
View SolutionIf `X and Y` are independent binomial vatiates `B(5,1/2) and B(7,1/2)` and the value of `P(X+Y=3)` is
View Solution`sin((1)/(2)cos^(-1).(4)/(5))` is equal to
View SolutionIn a `Delta ABC `, if A,B,C are in AP, then `(a)/(c) sin2C+(c)/(a)(sin2A)` is equal to
View SolutionThe general solution of the differential equation `(dy)/(dx)+(1+cos2y)/(1-cos2x)=0` is given by
View SolutionThe number of values of x in the interval `[0,5pi]` satisfying the equation `3sin^2x-7sinx+2=0` is
View SolutionLet `A(1,-1,2) and B(2,3-1)` be two points. If a point P divides AB internally in the ratio `2:3`, then the position vector of P is
View SolutionIf the line ` y cos alpha = x sin alpha +a cos alpha ` be a tangent to the circle `x^(2)+y^(2)=a^(2)`, then
View SolutionThe value of `lambda` for which the curve `(7x + 5)^2 + (7y + 3)^2 = lambda^2 (4x + 3y-24)^2` represents a parabola is
View Solutionif `y=4x+3` is parallel to a tangent to the parabola `y^2=12x`, then its distance from the normal parallel to the given line is
View SolutionLet the equation of an ellipse be `(x^2)/(144)+(y^2)/(25)=1`,Then , the radius of the circle with centre `(0,sqrt(2))` and passing through the foci of the ellipse is
View SolutionThe value of `sin50^(@)-sin70^(@)+sin10^(@)` is
View Solution
Paper 2 (Mathematics ) questions and complete solutions for chapter PRACTICE SET 02 of MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS of Class 12