Complete Solutions to Mathematics of chapter MHTCET 2016 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | MHTCET 2016 |
| MHTCET PREVIOUS YEAR PAPERS | Mathematics |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
Let `X~B(n,p)`, if E(X)=5,Var(X)`=2.5` then `P(X lt 1)` is equal to
View SolutionDerivative of ` tan^(-1)((x)/(sqrt( 1 - x^(2))))` with respect to
` sin^(-1) (3x - 4x^(3)) ` is
View SolutionForm the differential equation of the family of circles touching the y-axis at origin.
View SolutionIf `A=[(1,1,0),(2,1,5),(1,2,1)]` then `a_(11)A_(21)+a_(12)A_(22)+a_(13)A_(23)` is equal to
View SolutionIf the Rolle's theorem for `f(x)=e^(x)(sin x-cosx)` is verified on `[(pi)/4,(5pi)/4]` then the value of `C` is
View SolutionThe joint equation of lines passing through the origin and trisecting the first quadrant is
View SolutionIf `2 tan^-1 (cosx)=tan^-1 (2 cosecx)` then `sinx + cosx=`
View SolutionFind the direction cosines of teh line `(x-2)/2=(2y-5)/(-3),z=-1.` Also, find the vector equation of the line.
View Solution`int(dx)/(sqrt(8+2x-x^(2)))`
View SolutionThe a[[rpxo,ate va,ie pf `f(x)=x^3+5x^(2)-7x+9" at "x=1` is
View SolutionIf a random variable waiting time in minutes for bus and probability density function of x is given by
`f(x)={{:(1/5","0lexle5),(0",""otherwise"):}`
Then probability of waiting time not more than 4 minutes is equal to
View SolutionIn `DeltaABC,(a-b)^(2)"cos"^(2)(C)/(2)+(a+b)^(2)"sin"^(2)(C)/(2)` is equal to
View SolutionDerivative of ` log (sec theta + tan theta )` with respect top ` sec theta ` at
` theta = (pi)/(4) ` is
View SolutionThe joint equation of bisectors of angles between lines `x=5` and `y=3` is
View Solutionएक कण वक्र `6y= x^3+ 2` के अनुगत गति कर रहा है वक्र पर उन बिंदुओं को ज्ञात कीजिए जबकि x-निर्देशांक की तुलना में y-निर्देशांक 8 गुना तीव्रता से बदल रहा है
View SolutionIf the function f(x) defined by
`f(x)={(x"sin"(1)/(x)",", "for "x ne 0),(k",", "for " x=0):}`
is continuous at x = 0, then k is equal to
View SolutionIf `y = e^(m) sin^(-1) x and (1-x^(2)) ((dy)/(dx))^(2) = At^(2)` , then A is equal to
View Solution`int((4e^x-25)/(2e^x-5))dx=A x+B log/(2e^x)-5/(+c)` then
View Solution`(tan^(-1)(sqrt3)-sec^(-1)(-2))/(cosec^(-1)(-sqrt2)+cos^(-1)(-(1)/(2)))` is equal to
View SolutionFor what value of k, the function defined by
`f(x)={((log(1+2x)sin x^(@))/(x^(2))",", "for "x ne 0),(k",","for "x =0):}`
is continuous at x = 0 ?
View SolutionIf `log_(10)((x^(2)-y^(2))/((x^(2))+y^(2)))=2" then "(dy)/(dx)` is equal to
View Solution`((int (x)/(2))/(-(x)/(2)))log((2-sinx)/(2+sin x))` dx is equal to
View Solution`int ((x^(2)+2)(a^((x+tan^(-1)x)))/(x^(2)+1))` dx is equal to
View SolutionThe degree and order of the differential equation `[1+((dy)/(dx))^3]^(7/3)=7((d^2y)/(dx^2))` respectively are
View SolutionThe acute angle between the line `r=(hati+2hatj+hatk)+lambda(hati+hatj+hatk)` and the plane `r.(2hati-hatj+hatk)=5`
View SolutionFind by integration the area of the region bounded by the curve `y=2x-x^2` and the x-axis.
View SolutionIf `int(f(x))/(logsinx)dx=log*logsinx,` then f(x) is equal to
View SolutionIf A and B are foot of perpendicular drawn from point Q(a,b,c) to the planes yz and zx, then equation of plane through the point A,B, and O is
View SolutionIf `a=hati+hatk-2hatk, b=2hati-hatj+hatk and c=3hati-hatk c =ma+nb` then m+n ios equal to
View Solution`int_(0)^(pi//2) ((nsqrt(sec x))/(nsqrt(sec x)+nsqrt(cosecx)))dx` is equal to
View SolutionIf the probability density function of a random varaible X is given as
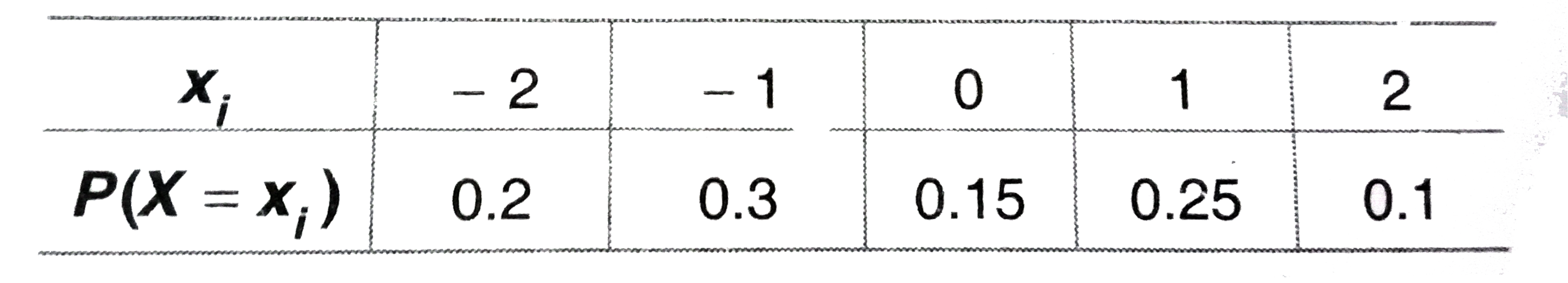
then F(0) is equal to
View SolutionFind the particular solutions of the following differential equation :
(1) ` y(1+ log x) dx/dy - x log x = 0 , when , x =e , y= e^(2)`
View SolutionIf M and N are the mid-points of the diagonals AC and BD respectively of a quadrilateral ABCD, then the of `vec (AB)+ vec(AD)+vec(CB)+vec(CD)` equals
View SolutionIf `sinx` is an integrating factor of the differential equation `(dy)/(dx)+P y=Q` , then write the value of `Pdot`
View SolutionWhich of the following equation does not represent a pair of lines ?
View SolutionProbability of guessing correctly atleast 7 out of 10 answers in a 'True' or 'False' test is equal to
View SolutionPrincipal solutions of the equation `sin 2x + cos 2x = 0`, where `pi < x < 2pi `
View SolutionIf line joining points A and B having position vectors `6 bar a-4 bar b+4 bar c` and `-4 bar c` respectively, and the line joining the points C and D having position vectors `-bar a-2 bar b-3 bar c` and `bar a+2 bar b -5 bar c` intersect, then their point of intersection is:
View SolutionIf `A=[(2,2),(-3,2)], B=[(0,-1),(1,0)]` then `(B^(-1)A^(-1))^(-1)` is equal to
View SolutionIf p : Every square is a rectangle.
q : Every rhombus is a kite, then truth values of `p to q` and `p harr q` are _________ and ________ respectively.
View SolutionIf `G(barg), H(barh)` and `P(barp)` are centroid, orthocenter and circumcenter of a triangle and `xbarp + ybarh + zbarg =0` then `(x, y, z)=`
View SolutionWhich of the following quantified statement is true?A) The square of every real number is positive
View SolutionThe general solution of the equation `tan^(2)x=1` is
View SolutionThe shaded part of given figure indicates in feasible region.
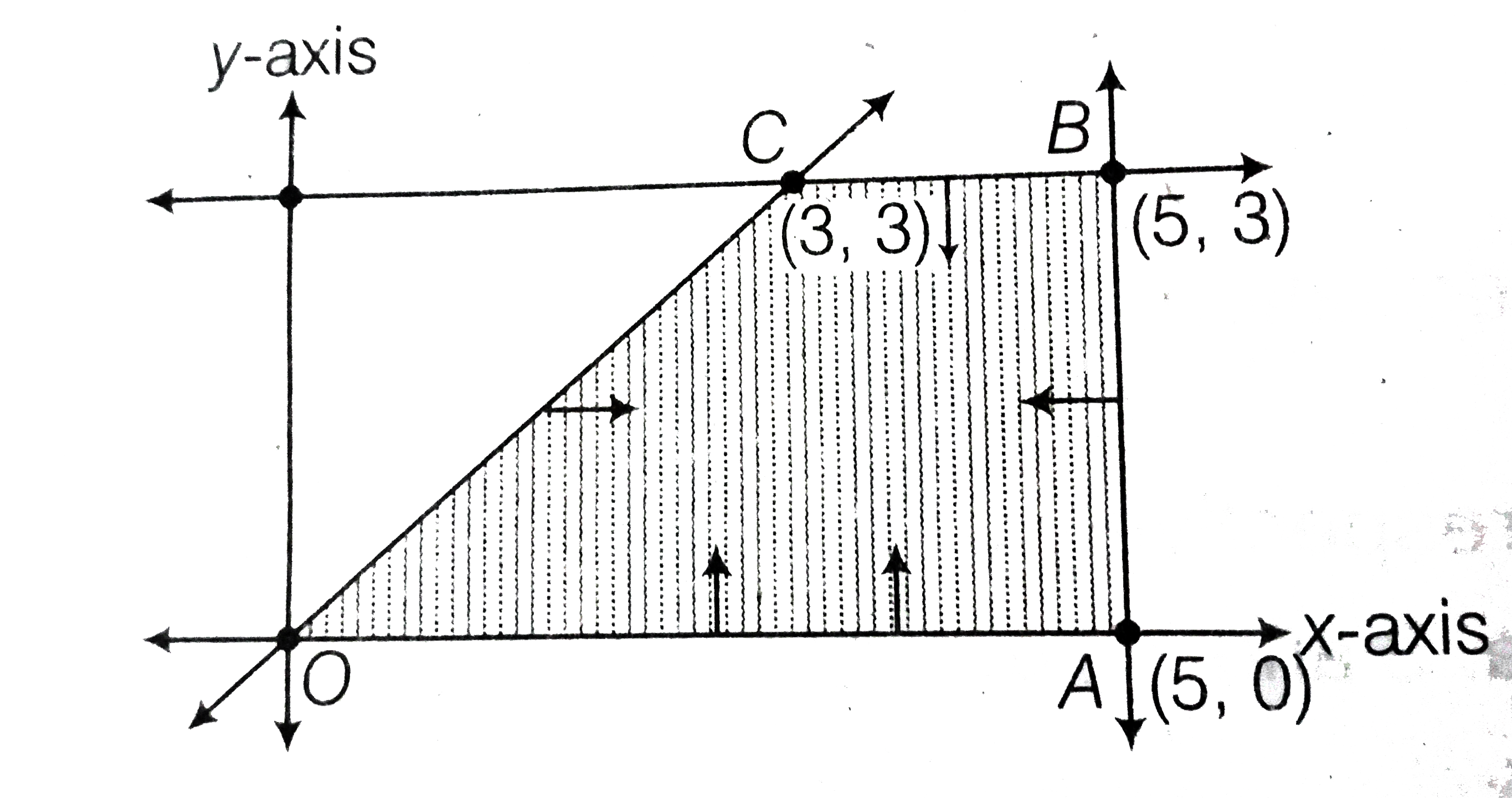
then the constraints are
View SolutionDirection ratios of the line which is perpendicular to the lines with direction ratios (-1,2,2) and (0,2,1) are
View SolutionIf matrix `A=[(1,2),(4,3)]`, such that `AX=l`, then `X` is equal to
View SolutionIf `a=hati+hatj+hatk, b=2hati+lambda hati+lambdahatj+hatk, c=hati-hatj+4hatk` and `a.(bxxc)=10` then `lambda` is equal to
View SolutionIf random variable `X~B(n=5,P=(1)/(3))`, then `P(2 lt X lt 4) is equal to
View SolutionThe objective function `z = x_1 + x_2`, subject to `x_1 + x_2 leq 10,-2x_1 + 3x_2 leq 15,x_1 leq 6,x_1 ,x_2 leq 0` has maximum value of the feasible region.
View Solution
View Solution
Mathematics questions and complete solutions for chapter MHTCET 2016 of MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS of Class 12