Complete Solutions to MHT CET Corner of chapter ROTATIONAL MOTION' of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ROTATIONAL MOTION' |
| MHTCET PREP GUIDE | MHT CET Corner |
| Number Of Questions | 35 |
| Academic Year | 2023-24 |
A circular disc of radius `R` and thickness `R//6` has moment of inertia `I` about an axis passing through its centre and perpendicular to its plane. It is melted and recast into a solid sphere. The `M.I` of the sphere about its diameter as axis of rotation is
View SolutionLet M be the mass and L be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is
View SolutionA cord is wound round the circumference of wheel of radius `r`. The axis of the wheel is horizontal and fixed and moment of inertia about it is `I`. A weight `mg` is attached to the end of the cord and falls from rest. After falling through a distance `h`, the angular velocity of the wheel will be.
View SolutionA satellite of mass `m` is in a circular orbit of radius `r` round the Earth. Calculate its angular momentum with respect to the centre of the orbit in terms of the mass `M` of the Earth and `G`.
View SolutionA ring and a disc roll on the horizontal surface without slipping, with same linear velocity. If bolh have same mass and radius and total kinetic energy of the ring is 4 J, then total kinetic energy of the disc is
View SolutionA circular disc of radius `R` and thickness `R//6` has moment of inertia `I` about an axis passing through its centre and perpendicular to its plane. It is melted and recast into a solid sphere. The `M.I` of the sphere about its diameter as axis of rotation is
View SolutionLet M be the mass and L be the length of a thin uniform rod. In first case, axis of rotation is passing through centre and perpendicular to the length of the rod. In second case, axis of rotation is passing through one end and perpendicular to the length of the rod. The ratio of radius of gyration in first case to second case is
View SolutionAn object of radius R and mass M is rolling horizontally without slipping with speed v . It then rolls up the hill to a maximum height `h = (3v^(2))/(4g)` . The moment of inertia of the object is ( g = acceleration due to gravity)
View SolutionThe moment of inertia of a uniform rod about a perpendicular axis passing through one end is `I_(1)`. The same rod is bent into a ring and its moment of inertia about a diameter is `I_(2)`. Then `I_(1)//I_(2)` is
View SolutionThree identicle particle each of mass `1 kg` are placed with their centres on a straight line. Their centres are marked `A, B and C` respectively. The distance of centre of mass of the system from `A` is.
View SolutionA rod PQ of mass M and length L is hinged at end P. The rod is kepts horizontal by a massless string tied to point Q as shown in the figure. When string is cut, the initial angular acceleration of the rod is

View Solution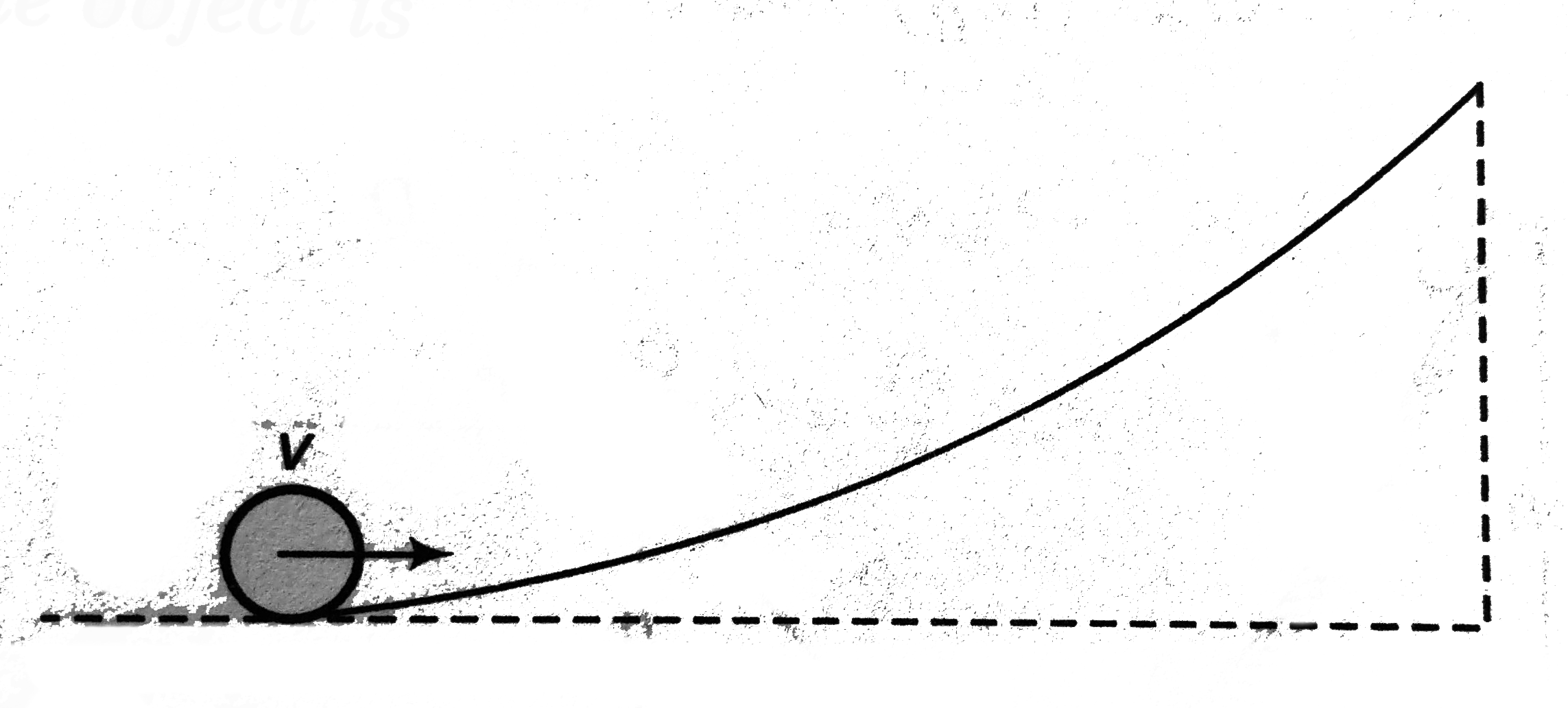
A small object of uniform density rolls up a curved surface with an initial velocity `v`. It reaches up to a maximum height of `(3v^(2))/(4g)` with respect to the initial position. The object is
(a). Ring
(b). solid sphere
(c). hollow sphere
(d). disc
View SolutionA circular disc is to be made by using iron and aluminium, so that it acquires maximum moment of inertia about its geometrical axis. It is possible with
View SolutionWhen a disc is rotating with angular velocity `omega` , a particle situated at a distance of 4 cm just begins to slip . If the angular velocity is doubled , at what distance will the particle start to slip ?
View SolutionWhich relation is not correct of the following ?
View SolutionThe moment of inertia of a uniform thin rod of length `L` and mass `M` about an axis passing through a point at a distance of `L//3` from one of its ends and perpendicular to the rod is
View SolutionMoment of inertia of a uniform circular disc about a diameter is `I`. Its moment of inertia about an axis perpendicular to its plane and passing through a point on its rim will be.
View SolutionThe moment of inertia of two freely rotating bodies A and B are `l_(A) and l_(B)` respectively. `l_(A) gt l_(B)` and their angular momenta are equal. If `K_(A) and K_(B)` are their kinetic energies,then
View SolutionMoment of inertia of big drop is l. If 8 droplets are formed from big drop, then moment of inertia of small droplet is
View SolutionMoment of inertia of a rod of mass M and length L about an axis passing through a point midway between centre and end is
View SolutionFrom a disc of radius R, a concentric circular portion of radius r is cut out so as to leave an annular disc of mass M. The moment of inertia of this annular disc about the axis perpendicular to its plane and passing through its centre of gravity is
View SolutionTwo spheres of equal masses, one of which is a thin spheical shelll and the other a solid, have the same moment of inertia about their respective diameters. The ratio of their radii well be
View SolutionThe moment of inertia of a uniform circular disc of radius `R` and mass `M` about an axis passing from the edge of the disc and normal to the disc is.
View SolutionThe moment of inertia of a solid sphere about an axis passing through the centre radius is `1/2MR^(2)` , then its radius of gyration about a parallel axis at a distance `2R` from first axis is
View SolutionMoment of inertia of a disc about an axis which is tangent and parallel to its plane is I . Then the moment of inertia of disc about a tangent, but perpendicular to its plane will be
View SolutionBy keeping moment of inertia of a body constant, if we double the time period, then angular momentum of body
View SolutionA disc of moment of inertia `9.8//pi^(2)kg m^(2)` is rotating at 600 rpm. If the frequency of rotation changes from 600 rpm to 300 rpm, then what is the work done ?
View SolutionThe center of mass of a system of two particles divides the distance between them.
View SolutionA thin circular ring of mass `m` and radius `R` is rotating about its axis with a constant angular velocity `omega`. Two objects each of mass `M` are attached gently to the opposite ends of a diameter of the ring. The ring now rotates with an angular velocity `omega' =`
View SolutionMoment of inertia depends on
View SolutionMoment of inertia of a disc about its own axis is I. Its moment of inertia about a tangential axis in its plane is
View SolutionA sphere of mass 0.5 kg and diameter 1m rolls without sliding with a constant velocity of 5 m/s . What is the ratio of the rotational K.E. to the total kinetic energy of the sphere?
View SolutionA body of moment of inertia of `3 kg m^(2)` rotating with an angular velocity of 2 rad/s has the same kinetic energy as that that of mass 12 kg moving with a velocity of
View SolutionThe moment of inertia of a thin circular disc of mass M and radius R about any diameter is
View SolutionThe radius of gyration of a disc of mass 100 g and radius 5 cm about an axis pasing through centre of gravity and perpendicular to the plane is
View Solution
MHT CET Corner questions and complete solutions for chapter ROTATIONAL MOTION' of MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS of Class 12