Complete Solutions to Level-II of chapter SOLUTION OF TRIANGLES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | SOLUTION OF TRIANGLES |
| MODERN PUBLICATION | Level-II |
| Number Of Questions | 50 |
| Academic Year | 2023-24 |
If in `DeltaABC` , `cotA`, `cotB`, `cotC` are in A.P., then
View SolutionThe sides of a triangle are in A.P and its area is `(3)/(5)xx` (area of equilateral triangle of same perimeter). Then the ratio of the sides to :
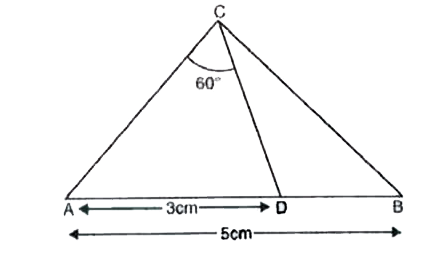
View SolutionIn the figure, `ABC` is a triangle in which `/_C=90^(@)` and `AB=5cm`. `D` is a point on `AB` such that `AD=3cm` and `/_ACD=60^(@)`. Then the length of `AC` is
View SolutionIn `DeltaABC`, `(acosA+bcosB+ccosC)/(a+b+c)` equals: where R and r are circum-radius and in -radius respectively
View SolutionThe ratio of R : r of an equilateral triangle is : where R and r are circumradius and inradius respectively
View SolutionH.M of exradii of a triangle is
View SolutionIn `DeltaABC`, the inradius and exradii are `r,r_(1),r_(2)` and `r_(3)` respectively. Then `r.r_(1)r_(2)r_(3)` equals
View SolutionIn any triangle `ABC`, `sin.(A)/(2)` is
View SolutionIn `DeltaABC`, `cosA+cosB+cosC gt 1` only if the triangle is
View SolutionLet `A_(0),A_(1),A_(2),A_(3),A_(4)` and `A_(5)` be the consecutive vertices of a regular hexagon inscribed in a circle of radius `1` unit. Then the product of the lengths of `A_(0)A_(1)`, `A_(0)A_(2)` and `A_(0)A_(4)` is
View SolutionIn a `DeltaABC` , if D is the middle point of BC and AD is perpendicular to AC, then `cos C` equals
View SolutionIf the angles of a triangle ABC satisfy the equation :
`81^(sin^(2)x)+81^(cos^(2)x)=30`, then the triangle can't be
View SolutionIf `n,n+1,n+2` where `n in N`, represent the sides of a triangle ABC, in which the largest angle is twice the smallest, then n is equal to
View SolutionIf in `DeltaABC`, `(cosA)/(7)=(cosB)/(19)=(cosC)/(25)=k`, then :
`|{:(-(1)/(k),25,19),(25,-(1)/(k),7),(19,7,-(1)/(k)):}|` equals
View SolutionIn a `DeltaABC`, if `|{:(a,b,c),(b,c,a),(c,a,b):}|=0`, then : `sinAsinB+sinBsinC+sinCsinA` equals
View SolutionIn a `DeltaABC`, if `A=18^(@)`, `b-a=2`, `ab=4`, then the triangle is
View SolutionThe perimeter of a triangle, right-angled at `C`, `70`, and the in-radius is `6`, then `|a-b|` equals
View SolutionIf in a `DeltaABC`, sines of angles A and B satisfy :
`4x^(2)-2sqrt(6)x+1=0` ,then `cos(A-B)` equals
View SolutionIn `DeltaABC`, if the median AD makes an angle `theta` with `AC`, and `AB=2AD`, then `sintheta` equals
View SolutionIn a `DeltaABC`, if `Re(sinA+sinB+sinC)=96`, then the area of the triangle in square units, equals
View SolutionIn a `DeltaABC`, if `cot.(A)/(2)cot.(B)/(2)=c`, `cot.(B)/(2)cot.(C )/(2)=a` and `cot.(C)/(2)cot.(A)/(2)=b`, then `(1)/(s-a)+(1)/(s-b)+(1)/(s-c)` equals
View SolutionThe distance of the incentre of `DeltaABC` from A is
View SolutionIn a `DeltaABC`, if `cotA=(x^(3)+x^(2)+x)^(1//2)`, `cotB=(x+x^(-1)+1)^(1//2)` and `cotC=(x^(-3)+x^(-2)+x^(-1))^(-1//2)` , then the triangle is
View SolutionIf in `DeltaABC`, `(b+c)/(11)=(c+a)/(12)=(a+b)/(13)`, then `cosA` equals :
View SolutionIf `p_(1),p_(2),p_(3)` are respectively the perpendiculars from the vertices of a triangle to the opposite sides, then `p_(1)p_(2)p_(3)` equals
View SolutionIf `(a)/(cosA)=(b)/(cosB)=(c )/(cosC)`, then triangle is
View SolutionIf `c^(2)=a^(2)+b^(2)`, then `4s(s-a)(s-b)(s-c)` equals
View SolutionIf the lengths of the sides of a `DeltaABC` are `3,4` and `5cm` , then the distance between its incentre and circumcentre is
View SolutionIf the lengths of the sides of a `DeltaABC` are `3,4` and `5cm` , then the distance between its orthocentre and circumcentre is
View SolutionIf A,B,C are the angles of a triangle, then the value of `cot.(A)/(2)+cot.(B)/(2)+cot.(C )/(2)` is
View SolutionThe area of a circle is `A_(1)` and the area of a regular pentagon inscribed in the circle is `A_(2)`. Then `A_(1)//A_(2)` is
View SolutionIn a triangle ABC , `2acsin.(1)/(2)(A-B+C)` is equal to :
View SolutionIn a triangle ABC, let `/_C=pi//2`. If r is the inradius and R is the circum-radius of the triangle, then `2(r+R)` is equal to :
View SolutionThe number of distinct real roots of `|(sinx,cosx,cosx),(cosx, sinx,cosx),(cosx,cosx,sinx)|=0` in the interval `(-pi)/4lexlepi/4` is :
View SolutionIn a triangle ABC, `2casin.(A-B+C)/(2)=`
View SolutionIn a `DeltaABC`, `tan.(A)/(2)=(5)/(6)`, `tan.(C)/(2)=(2)/(5)`, then
View SolutionIn a triangle ABC, `a=4`, `b=3`, `/_A=60^(@)`, then `c` is the root of the equation
View SolutionIn a triangle with sides `a,b,c,r_(1) gt r_(2) gt r_(3)` (which are extradii), then
View SolutionThe sides of a triangle are `3x+4y`, `4x+3y` and `5x+5y` , where `x,y gt 0`, then the triangle is
View SolutionWhich of the following pieces of data does NOT uniequely determine an acute angled triangle ABC (`R` being the radius of the circumcircle) ?
View SolutionIf in a triangle `ABC`, `acos^(2).(C)/(2)+cos^(2).(A)/(2)=(3b)/(2)`, then the sides `a,b,c`
View SolutionIn a triangle ABC, medians AD and BE are drawn . If `AD=4`, `/_DAB=(pi)/(6)` and `/_ABE=(pi)/(3)`, then the area of the `DeltaABC` is
View SolutionThe sum of the radii of inscribed and circumscribed circles for an n-sided regular polygon of side 'a' is
View SolutionThe angles of a triangle are in the ratio `4 : 1 :1`, then the ratio of the largest side to the perimeter is
View SolutionThe sides of a triangle are `sinalpha,cosalpha` and `sqrt(1+sinalphacosalpha)` for some `0 lt alpha lt (pi)/(2)`. The the greatest angle of the triangle is
View SolutionThe sides of a triangle are in the ratio `1 : sqrt(3) : 2`, then angles of the triangle are in the ratio
View SolutionIf in a `DeltaABC`, the altitudes from the vertices A,B,C on opposite sides are in H.P., then `sinA,sinB,sinC` are in
View SolutionIf a,b,c are sides opposite to the angles A,B,C, then which of the following is correct ?
View SolutionA triangular park is enclosed on two sides by a fence and on the third side of straight river bank. The two sides having fence of same length `x`. The maximum area enclosed by the park is
View SolutionInternal bisector of `/_A` of triangle `ABC` meets side `BC` at `D.A.` line drawn through `D` perpendicular to `AD` intersects the side `AC` at `E` and the side `AB` at `F`. If `a`, `b`, `c` represent the sides of `DeltaABC`, then :
View Solution
Level-II questions and complete solutions for chapter SOLUTION OF TRIANGLES of MODERN PUBLICATION MATHS (KANNADA ENGLISH) of Class 12