Complete Solutions to Example of chapter APPLICATION OF INTEGRALS of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | APPLICATION OF INTEGRALS |
| NCERT TAMIL | Example |
| Number Of Questions | 15 |
| Academic Year | 2023-24 |
Find the area of the circle `x^(2) + y^(2) = a^(2)` using integration.
08:27View Solution(1) Draw the rough sketch of the ellipse `(x^(2))/(a^(2)) + (y^(2))/(b^(2)) = 1`.
Find the area enclosed by the ellipse `(x^(2))/(a^(2)) + (y^(2))/(b^(2)) = 1`.09:01View SolutionFind the area of the region bounded by the curve `y = x^(2)` and the line y = 4.
02:46View SolutionFind the area of the region in the first quadrant enclosed by the x-aixs, the line y = x, and the circle `x^(2) + y^(2) = 32`.
12:49View SolutionFind the area of the region BOB'RESB is enclosed by the ellipse and the lines x = 0 and x = ae , where `b^2` = `a^2` (1- `e^2`) and e<1
07:29View SolutionFind the area of the region bounded by the two parabolas `y = x^(2)` and `y^(2) = x`.
09:25View SolutionFind the area lying above x-axis and included between the circle `x^(2) + y^(2) = 8x` and inside the parabola `y^(2) = 4x`.
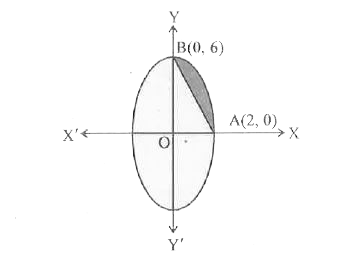
12:45View SolutionIn the figure given below, AOBA is the part of the ellipse `9x^(2) + y^(2) = 36` in the first quadrant such that OA = 2 and OB = 6. Find the area between arc AB and the chord AB
 11:50View Solution
11:50View SolutionUsing integration, find the area of the region bounded by the triangle whose vertices are (0, 1), (2,2) and (3, 1).
16:19View SolutionFind the area enclosed between the circles `x^(2) + y^(2) = 4` and `(x - 2)^(2) + y^(2) = 4`.
02:18View SolutionConsider the parabola `y^(2) = 4ax`
(i) Write the equation of the rectum and obtain the x co-ordinates of the point of intersection of latus rectum and the parabola.
(ii) Find the area of the parabola bounded by the latus rectum.07:32View SolutionFind the area of the region bounded by the line y = 3x +2, the x-axis and the ordinates x = -1 and x = 1.
07:11View SolutionFind the area bounded by the curve y = sin x between x = 0 and x = `2pi`.
04:28View SolutionProve that the curves `y^(2) = 4x` and `x^(2) = 4y` divide the area of the square bounded by x = 0, x = 4 and y = 0 into three equal parts.
11:50View SolutionFind the area of the region `{(x,y): y^(2) le 4x, 4x^(2) + 4y^(2) le 9}`.
13:15View Solution
Example questions and complete solutions for chapter APPLICATION OF INTEGRALS of FULL MARKS MATHS(TAMIL) of Class 12
