Complete Solutions to EXAMPLES of chapter PROBABILITY of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PROBABILITY |
| NCERT TELUGU | EXAMPLES |
| Number Of Questions | 36 |
| Academic Year | 2023-24 |
If `P(A)=(7)/(13), P(B)=(9)/(13)` and `P(A cap B)=(4)/(13)`, evaluate P(A|B).
View SolutionA family has two children. What is the probability that both the children are boys given that at least one of them is a boy ?
View SolutionTen cards numbered 1 to 10 are placed in a box, mixed up thoroughly and then one card is drawn randomly. If it is known that the number on the drawn card is more than 3, what is the probability that it is an even number?
View SolutionIn a school, there are 1000 students, out of which 430 are girls. It is known that out of 430, 10% of the girls study in class XII. What is the probability that a student chosen randomly studies in Class XII given that the chosen student is a girl?
View SolutionA die is thrown three times. Events A and B are defined as below:
A : 4 on the third throw
B : 6 on the first and 5 on the second throw
Find the probability of A given that B has already occurred.
View SolutionA die is thrown twice and the sum of the numbers appearing is observed to be 6. What is the conditional probability that the number 4 has appeared at least once?
View SolutionConsider the experiment of tossing a coin. If the coin shows head, toss it again but if it shows tail, then throw a die. Find the conditional probability of the event that the die shows a number greater than 4' given that there is at least one tail.
View SolutionAn urn contains 10 black and 5 white balls. Two balls are drawn from the urn one after the other without replacement. What is the probability that both drawn balls are black?
View SolutionThree cards are drawn successively, without replacement from a pack of 52 well shuffled cards. What is the probability that first two cards are kings and the third card drawn is an ace?
View SolutionA die is thrown. If E is the event the number appearing is a multiple of 3' and F be the event 'the number appearing is even' then find whether E and F are independent ?
View SolutionAn unbiased die is thrown twice. Let the event A be 'odd number on the first throw' and B the event 'odd number on the second throw'. Check the independence of the events A and B.
View SolutionThree coins are tossed simultaneously. Consider the event E 'three heads or three tails', F 'at least two heads' and G ‘at most two heads'. Of the pairs (E,F), (E,G) and (F,G), which are independent? which are dependent?
View SolutionIf A and B are two independent events, then the probability of occurrence of at least one of A and B is given by 1- P(A') P(B').
View SolutionA person secures a job in a construction company in which the probability that the workers go on strike is 0.65 and the probability that the construction job will be completed on time if there is no strike is 0.80. If the probability that the construction job will be completed on time even if there is a strike is 0.32, determine the probability that the constructed job will be completed on time.
View SolutionBag I contains 3 red and 4 black balls. Bag II contains 5 red and 6 black balls. If one ball is drawn at random from one of the bags and it is found to be red, then the probability that it was drawn from Bag II, is
View SolutionGiven three identical boxes I, II and III, each containing two coins. In box I, both coins are gold coins, in box II, both are silver coins and in the box III, there is one gold and one silver coin. A person chooses a box at random and takes out a coin. If the coin is of gold, what is the probability that the other coin in the box is also of gold?
View SolutionSuppose that the reliability of a HIV test is specified as follows:
Of people having HIV, 90% of the test detect the disease but 10% go undetected. Of people free of HIV, 99% of the test are judged HIV-ive but 1% are diagnosed as showing HIV+ive. From a large population of which only 0.1% have HIV, one person is selected at random, given the HIV test, and the pathologist reports `"him" // "her"` as HIV+ive. What is the probability that the person actually has HIV?
View SolutionIn a factory which manufactures bolts, machines A, B and C manufacture respectively 25%, 35% and 40% of the bolts. Of their outputs, 5, 4 and 2 percent are respectively defective bolts. A bolt is drawn at random from the product and is found to be defective. What is the probability that it is manufactured by the machine B?
View SolutionA doctor is to visit a patient. From the past experience, it is known that the probabilities that he will come by train, bus, scooter or by other means of transport are respectively `(3)/(10), (1)/(5), (1)/(10)` and `(2)/(5)`. The probabilities that he will be late are `(1)/(4), (1)/(3)` and `(1)/(12)`, if he comes by train, bus and scooter respectively, but if he comes by other means of transport, then he will not be late. When he arrives, he is late. What is the probability that he comes by train?
View SolutionA man is known to speak the truth 3 out of 4 times. He throws a die and reports tht it a six. The probability that it is actually a six is
View SolutionA person plays a game of tossing a coin thrice. For each head, he is given Rs 2 by the organiser of the game and for each tail, he has to give Rs 1.50 to the organiser. Let X denote the amount gained or lost by the person. Show that X is a random variable and exhibit it as a function on the sample space of the experiment.
View SolutionA bag contains 2 white and 1 red balls. One ball is drawn at random and then put back in the box after noting its colour. The process is repeated again. If X denotes the number of red balls recorded in the two draws, describe X.
View SolutionTwo cards are drawn successively with replacement from a well shuffled pack of 52 cards. The probability of drawing two aces is
View SolutionFind the probability distribution of number of doublets in three throws of a pair of dice.
View SolutionLet X denote the number of hours you study during a randomly selected school day. The probability that X can take the values x, has the following form, where k is some unknown constant.
`P(X=x)={{:(0.1, "if "x =0),(kx, "if "x = 1 or 2 ),(k(5-x), "if " x=3 or 4),(0,"otherwise"):}`
(a) Find the value of k.
(b) What is the probability that you study at least two hours ? Exactly two hours? At most two hours?
View SolutionLet a pair of dice be thrown and the random variable X be the sum of the numbers that appear on the two dice. Find the mean or expectation of X.
View SolutionFind the variance of the number obtained on a throw of an unbiased die.
View SolutionTwo cards are drawn simultaneously (or successively without replacement) from a well shuffled pack of 52 cards. Find the mean, variance and standard deviation of the number of kings.
View SolutionSix balls are drawn successively from an urn containing 7 red and 9 black balls. Tell whether or not the trials of drawing balls are Bernoulli trials when after each draw the ball drawn is
(i) replaced (ii) not replaced in the urn.
View SolutionIf a fair coin is tossed 10 times, find the probability of
(i) exactly six heads
(ii) at least six heads
(iii) at most six heads
View SolutionTen eggs are drawn successively with replacement from a lot containing 10% defective eggs. Find the probability that there is at least one defective egg.
View SolutionColoured balls are distributed in four boxes as shown in the following table:
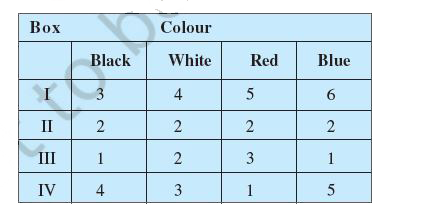
A box is selected at random and then a ball is randomly drawn from the selected box. The colour of the ball is black, what is the probability that ball drawn is from the box III?
View SolutionIf the mean and variance of a binomial distribution are 4 and `4//3`. Then the distribution is
View SolutionThe probability of a shooter hitting a target is `(3)/(4)`. How many minimum number of times must `"he" // "she"` fire so that the probability of hitting the target at least once is more than 0.99?
View SolutionA and B throw a die alternatively till one of them gets a '6' and wins the game. Find their respective probabilities of winning, if A starts first.
View SolutionIf a machine is correctly set up, it produces 90% acceptable items. If it is incorrectly set up, it produces only 40% acceptable items. Past experience shows that 80% of the set ups are correctly done. If after a certain set up, the machine produces 2 acceptable items, find the probability that the machine is correctly setup.
View Solution
EXAMPLES questions and complete solutions for chapter PROBABILITY of NCERT MATHEMATICS(TELUGU) of Class 12