Complete Solutions to MATHEMATICS of chapter NTA TPC JEE MAIN TEST 37 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | NTA TPC JEE MAIN TEST 37 |
| NTA MOCK TESTS | MATHEMATICS |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
The coefficient of `x^10` in the expansion of: `(3x^(2) - 1/x^(2))^(15)` is:
View SolutionGiven that `z_(1), z_(2)` and `z_3` are complex numbers with `|z_(1)| = |z_(2)| = |z_(3)| = 1, z_(1) + z_(2) + z_(3) =1` and `z_(1)z_(2)z_(3)=1`, then `|(z_(1) +2)(z_(2)+2)(z_(3)+2)|` is equal to:
View SolutionLet `f(x) = ln(x+ sqrt(x^(2)+1)`, then the value of determinant: `|{:(f(sin 2017 pi),f(sin pi/6), f(e^(x))),(f(cos (2pi)/3), f(cos (2017pi)/2), f(tan pi/3)),(f(-e^(x)), f(cot (5pi)/6), f(0)):}|` is:
View SolutionWhich of the following is correct ?
View SolutionA line is drawn from A(—4, 0) to intersect the curve `x^(2)/8 + y^(2)/4 =1` at P and Q above z-axis. If `1/(AP) + 1/(AQ) ge sqrt(3)/2`, then the maximum value of the slope of line is:
View SolutionIf `n(A) = 1000, n(B) = 500` and if `n(A cap B) ge 1` and `n(A cup B) =p`, then
View SolutionA circle passes through the points of intersection of the parabola `y+1 =(x-4)^(2)` and x-axis. Then the length of tangent from origin to the circle is:
View SolutionAn equilateral triangle is inscribed in ellipse whose equation is `x^(2) + 4y^(2) = 4`, one vertex of triangle is (0, 1) and one altitude is contained in y -axis and length of each side is `sqrt(m/n)` (where m and n are relatively prime), then m + n is:
View SolutionThe number of integral values of a for which three distinct chords of the ellipse `x^(2)/(2a^(2)) + y^(2)/a^(2) =1` passing through the point `(20a, -a^(2)/2)` are bisected by the parabola `y^(2) = 4ax` is:
View SolutionIf `ax^(2) + 2hxy - ay^(2) =0, a gt 0`, represents a pair of straight lines forming with `2x + 3y = -8` an isosceles triangle which is right angled at origin, then (a + h) is:
View SolutionIf `x cos alpha + y sin alpha =p` is tangent to the curve `y =-sqrt(x)` , then range of a is:
View SolutionLet P = (-1,0), Q = (0,0) and `R = (3, 3sqrt(3))` be three point. The equation of the bisector of the angle PQR is:
View Solutionlf `sum_(n=1)^k [1/3 + n/90] = 21`, where [x] denotes the integral part of x, then is equal to
View SolutionIf the tangent at any point on the curve `x^(4) + y^(4) = a^(4)` cuts off intercepts p and q on the co-ordinate axes then the value of `p^(-4//3) + q^(-4//3)` is:
View SolutionA continuous function `f : R to R` satisfies the differential equation `f(x) = (1+x^(2)) [1 + f_(0)^(x) (f(t))^(2)/(1+t^(2)) dt]`, then f(-3) is:
View SolutionIf `int sqrt(2) sqrt(1+ sinx) dx = -4 cos(ax + b) + C` then the value of (a,b) is :
View Solutionp : Every quadratic equation has one real root and q Every quadratic equation has two real roots, then truth value of p and q are:
View SolutionThe S.D. of the following data is nearly:
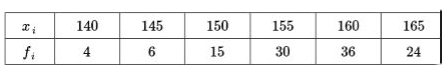
View SolutionNumber of solutions of the equation
`(sqrt(6) - sqrt(2))/(sin x) + (sqrt(2) + sqrt(6))/(cos x) = 8` in `(0,pi/2)` is/are:
View SolutionNumber of solutions of the equation `sin^(-1)(sin 6x) =x` in `x in [0,pi]` is:
View SolutionThe tens digit of 1! + 2! + 3! + ... + 49! Is:
View SolutionLet `k_(1)` and `k_2` are two values of k for which the equation `4x^(2) -4(5x+1) + k^(2)=0` has one root equals to two more than the other, then find the value of: `(k_(1)^(2) - k_(2)^(2))`
View SolutionLet `veca, vecb` and `vecc` be three vectors such that `|vecb| =2|veca|` and `3|veca|`.The Angle between each pair of vectors is 60° such that `|veca +2vecb+3vecc| = sqrt(21)`, then `sqrt(7)|vecc|` is equal to:
View SolutionLet `("lim")_(h to 0)(1/(hroot(3)(8+h)) - 1/(2h)) = k`, then find `96k + 3`.
View SolutionLet `L = "lim"_(n to infty) 1/n^(3) sum_(k=1)^(n) k^(2)e^(k/n)`, then find the value of e-L
View SolutionIf `f(x) ge 0AA x in (0,2)` and y = f (x) makes positive intercepts having length 2 and 1 unit(s) on coordinate axes respectively and encloses an area of `3/4` sq. units with axes, then the value of `3-4 int_(0)^(2) xf(x) dx` is:
View SolutionFind `f(x) =(a^(x)-1)^(3)/(sin(x log a) log(1+x^(2) log a^(2))` and `f(0) =m log a`. Find m such that f is continuous at `x=0`.
View SolutionIf x = 9 is the chord of contact of the hyperbola `x^(2) - y^(2) =9`, then the equation of the pair of tangents forming the chord of contact is `ax^(2) - by^(2) - 18x + 9=0`. Find the value of a + b.
View SolutionIf `tan(pi cos theta) = cot(pi sin theta)`, then `2 cos^(2)(theta - pi/4)` is:
View SolutionAn urn contains 6 white and 4 black balls. A fair die is rolled and that number of balls are chosen from the urn. Find the probability that balls selected are white.
View Solution
MATHEMATICS questions and complete solutions for chapter NTA TPC JEE MAIN TEST 37 of NTA MOCK TESTS of Class 12