Complete Solutions to MATHEMATICS of chapter NTA TPC JEE MAIN TEST 58 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | NTA TPC JEE MAIN TEST 58 |
| NTA MOCK TESTS | MATHEMATICS |
| Number Of Questions | 30 |
| Academic Year | 2023-24 |
The term independent of x in the expansion of `[(t^(-1) - 1)x + (t^(-1) + 1)^(-1)x^(-1)]^8` is :
View SolutionLet `alpha_1, alpha_2` and `beta_1, beta_2` are roots of the equation `ax^2 + bx + c = 0` and `px^2 + qx + r = 0` respectively. If the system of equations `alpha_1 y + alpha_2 z = 0`and `beta_1 y + beta_2 z = 0 `has a non trivial solution, then
View SolutionThe value of `sum_(r = 1)^(50) (1^3 + 3^3 + 5^3 + ..... + (2r - 1)^3)/(1 + 3 + 5+.... + (2r - 1))` :
View SolutionIf `P = {x in R : f(x) = 0}` and `Q = {x in R : g(x) =0}`, then `P uu Q `is
View SolutionIf the length of a focal chord of parabola `y^ = 4x `is `25/4` and has a positive slope, then the slope of the focal chord will be
View SolutionIf `(a,b), (c,d), (e, f)` are the vertices of a triangle such that a, c, e are in G. P. with common ratio r and b, d, f are in G. P. with common ratio s, then the area of the triangle is
View SolutionLet `|veca| = 2, |vecb| = 5` . The possible value of k for which the vectors `veca + k vecb` and `veca - k vecb`are perpendicular is
View SolutionWhich of the following function is surjective but not injective?
View SolutionIf `lim_(x to 1) (ax^3 + bx^2 + cx + d)/((x - 1)^3)` exists and finite, then `(b + c + d)/(a)` is equal to
View SolutionIf `y = f(x)` satisfies the condition `f(x) = f(4 – x) AA x in (0, 4), f (x) = f(14 – 2) AA x in [4, 10] f(x) = {{:([x^2 - 2x + 5]"," , x in (0,1]),(3 + sqrt(2x - x^2)",", x in (1,2]),((20 - 2x)/(3)",", x in [4,7]):}`
Then the area bounded by `y = f(x), x = 0, x = 10 ` and the x -axis isView SolutionIf `int (2 cos x + 3 sin x)/(3 cos x + 4 sin x) dx = Ax + B` , then `ln |3 cos x + 4 sin x| + C (A + B)` is equal to
View Solution`~ (p
View SolutionIf the standard deviation of the numbers 2, 3, a and 11 is 3.5, then which of the following is true?
View SolutionEach side of an equilateral triangle subtends an angle of `60^@` at the top of a tower h meters high located at the centre of the triangle. If a meters is the length of each side of the triangle, then
View SolutionThe value of x satisfying the equation `(sin^(-1)x)^3 - (cos^(-1)x)^3 + (sin^(-1)x) (cos^(-1)x)(sin^(-1)x - cos^(-1)x) = (pi^3)/(16)` is
View SolutionThe number of points where the function, `f(x) = cos|2018pi – x| + sin|2020pi "is" -x | + (x - pi)|x^2 - 3pi x + 2pi^2|` non-differentiable is/are
View SolutionThe hyperbola `(x^2)/(a^2) - (y^2)/(b^2) = 1`, the area of the triangle formed by the asymptotes and the tangent drawn to it at (a, 0) is
View SolutionLet `f (x)` is a differentiable function such that `f (x + y) = f (x) + f (y) + 2xyAAx, y in R` and `lim_(x to 0) (f(x))/(x) = 210`, then f (2) is equal
View SolutionIf `270^@ lt theta lt 360^@,` then `sqrt(2 + sqrt(2 + 2cos theta))` is equal to
View SolutionThe general solution of the trigonometric equation `sin x – cos x = 1` is given by
View SolutionIf `omega` is non real root of equation `x^3 – 1 = 0` then value of `sum_(r - 1)^(5)(1+ omega^r + omega^(2r))` is
View SolutionLet A be the set of all `3 xx 3` skew symmetric matrices whose entries are either –1, 0 or 1. If there are exactly three O's three l's and three (-1)'s, then the number of such matrices is
View SolutionThe number of the rectangle in the following figure is
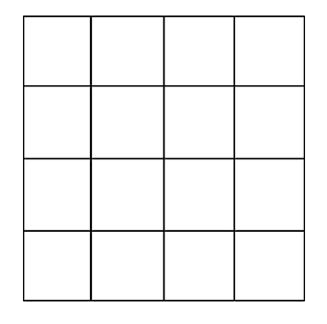 View Solution
View SolutionIf `alpha, beta and gamma` are the roots of the cubic equation `x^3 – 3x^2 + 1 = 0`, then `(alpha − 2)(beta – 2) (gamma – 2) = `
View SolutionA circle C having center at (1, 2) and radius equal to 3, cuts the members of the family of circles passing through two fixed points P(2, 6) and Q(4, 5), such that the common chords pass through a fixed point (21, yı), then the value of `((y_1 - x_1)/(7))` is
View SolutionOrigin O is the centre of two concentric circles whose radii are a and b respectively, `a lt b`. A line OPQ iş drawn to cut the inner circle in P and the outer circle in Q. PR is drawn perpendicular to x – axis and QR is drawn perpendicular to the y- axis. The locus of R is an ellipse touching the two cireles. If the foci of the ellipse lie on the inner circle, if eccentricity is `sqrt(2) K`, then the value of K is
View SolutionIf the minimum and maximum value of function `3x^4 – 8x^3 + 12x^2 – 48x + 25` on the interval (0,3] is b and a respectively, then the value a – b is
View SolutionThrough the point P(1, 2, 2) a plane is drawn at right angles to OP, O being the origin, to meet the axes in A, B, C. If the area of triangle ABC is `(240+lambda)/8` sq. units , then `lambda` equals
View SolutionA normal is drawn at a point A (x, y) of a curve. It meets the X -axis and Y - axis at point P and Q respectively such that `1/(OP) + 1/(OQ) = 1` where O is the origin. Then, the equation of such a curve passing through (5, 4) is
View Solution`int_0^5 [x] {x}dx` (where [.]denotes greatest integer function and{.} denotes fractional part of function is
View Solution
MATHEMATICS questions and complete solutions for chapter NTA TPC JEE MAIN TEST 58 of NTA MOCK TESTS of Class 12
