Complete Solutions to PHYSICS of chapter NTA NEET SET 41 of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | NTA NEET SET 41 |
| NTA MOCK TEST | PHYSICS |
| Number Of Questions | 45 |
| Academic Year | 2023-24 |
A moving positron and electron both with kinetic energy 1 Me V annihilate with each other and emits two gamma photons. If the rest mass energy of an electron is 0.51 MeV, the wavelength of each photon is ?
View SolutionIn `Li^(++)` , electron in first Bohr orbit is excited to a level by a radiation of wavelength `lamda` When the ion gets deexcited to the ground state in all possible ways (including intermediate emissions), a total of six spectral lines are observed. What is the value of `lamda` (Given : `h= 6.63 xx 10^(-34) Js, c = 3 xx 10^(8) ms^(-1)`)
View SolutionA mass ‘m’ moves with a velocity ‘v’ and collides inelastically with another identical mass at rest. After collision the 1st mass moves with velocity `(v)/(sqrt(3))` in a direction perpendicular to the initial direction of motion. Find the speed of the `2^(nd)` mass after collision :
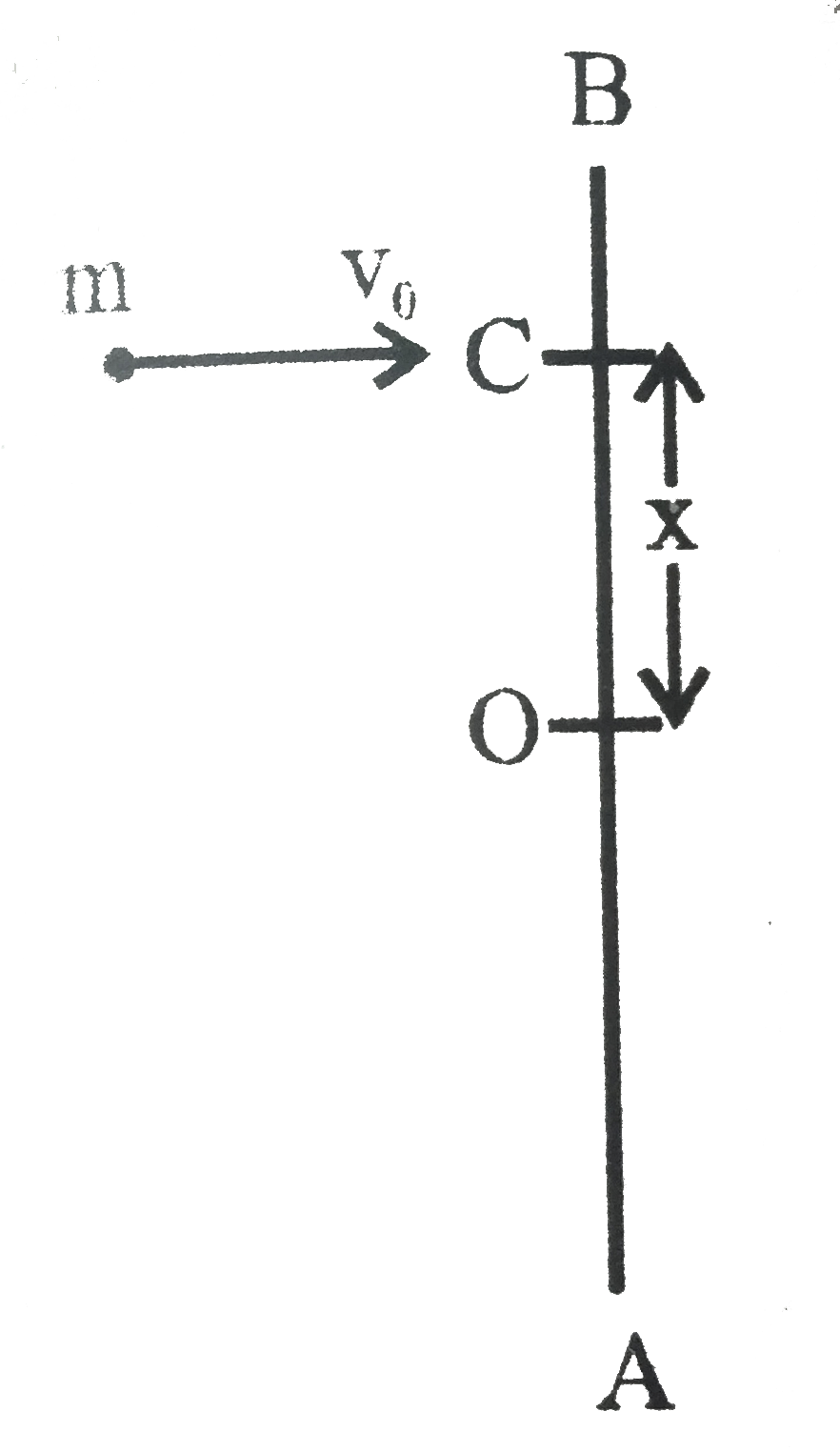
View SolutionA uniform rod `AB` of length `L` and mass `M` is lying on a smooth table. A small particle if mass `m` strike the rod with velocity `v_(0)` at point `C` at a distance comes to rest after collision. Then find the value of `x`, so that point `A` of the rod remains stationary just after collision.
 .
.
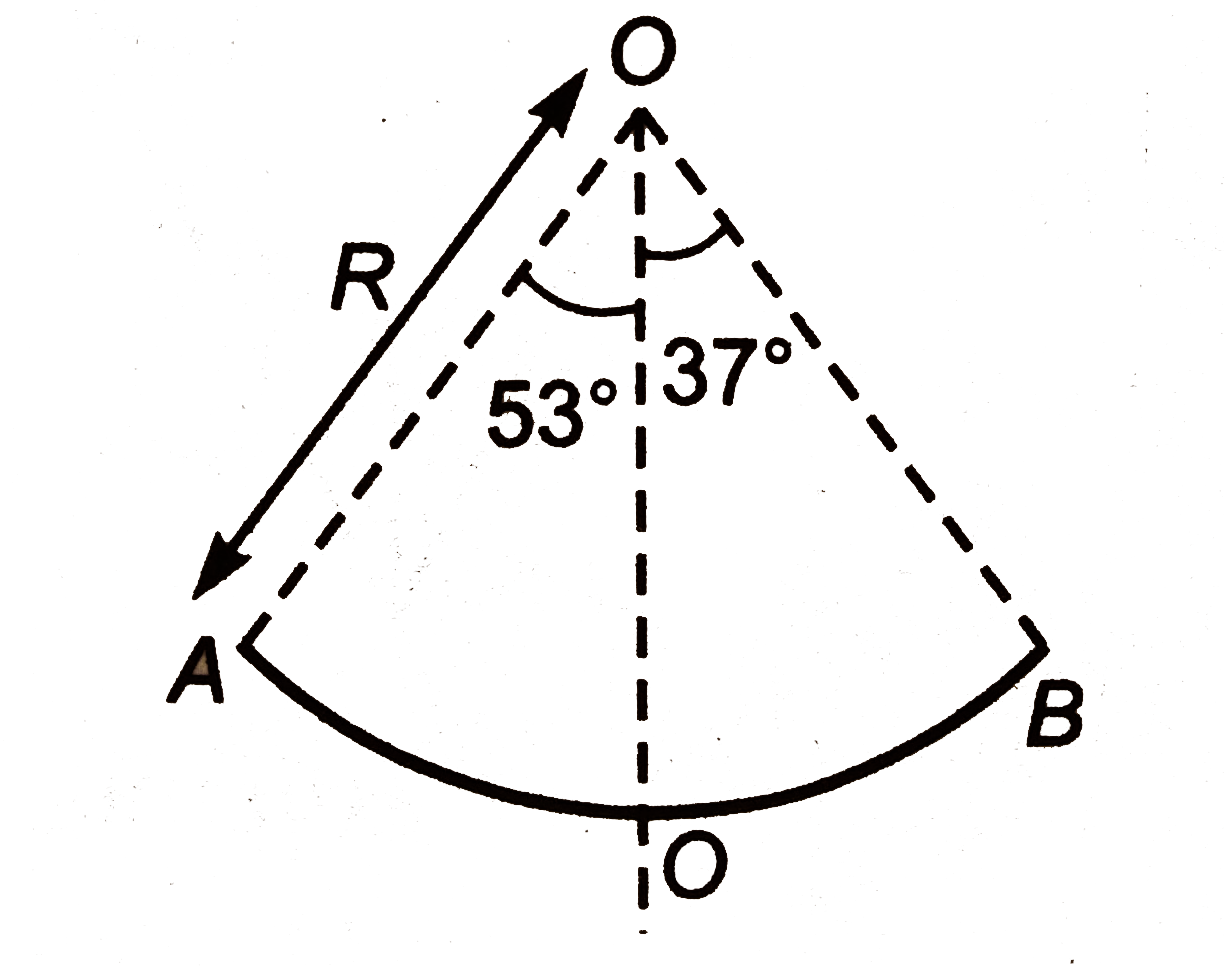
View SolutionA section of fixed smooth circular track of radius `R` in vertical plane is shown in the figure. A block is released from position `A` and leaves the track at `B` The radius of curvature of its trajectory just after it leaves the track `B` is ?
View SolutionA particle of charge q and mass m is projected with a velocity `v_0` toward a circular region having uniform magnetic field B perpendicular and into the plane of paper from point P as shown in Fig. 1.136. R is the radius and O is the center of the circular region. If the line OP makes an angle `theta` with the direction of `v_0` then the value of `v_0` so that particle passes through O is

View SolutionThe resistance of a wire at `20^(@)C` is `20Omega` and at `500^(@)C` is `60Omega`. At which temperature its resistance will be `25Omega`?
View SolutionThe ratio of the resistances of a conductor at a temperature of `15^(@)C` to its resistance at a temperature of `37.5^(@)C` is `4:5`. The temperature coefficient of resistance of the conductor is
View SolutionA power transmission line feeds input power at 2300 V a step down transformer with its primary windings having 4000 turns. The output power is delivered at 230 V by the transformer. If the current in the primary of the transformer is 5A and its efficiency is `90%`, the output current would be :
View SolutionA coil of inductanece `5H` is joined to a cell of emf `6V` through a resistance `10 Omega` at time t=0. The emf across the coil at time `t=ln sqrt(2)` s is:
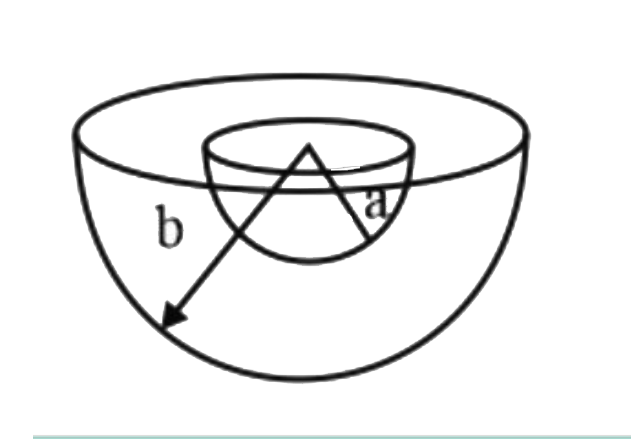
View SolutionA uniform charged hemisphere of radius b and charge density `rho` has a hemispherical cavity of radius `a(a=b/2)` cut from its centre . If the potential at the centre of the cavity is `(nrhob^2)/(16 in_0)` then n = ?
View SolutionA charge 'Q' is distributed over two concentric hollow spheres of radii 'r' and 'R' (gtr) such that the surface densities are equal. Find the potential at the common centre.
View SolutionA battery of emf 12 V and internal resistance `2Omega` is connected in series with a tangent galvanometer of resistance `4Omega` . The deflection is `60^@` when the plane of the coil is along the magnetic meridian . To get a deflection of `30^@`, the resistance to be connected in series with the tangent galvanometer is
View SolutionA projectile of mass `m` is fired from the surface of the earth at an angle `alpha = 60^(@)` from the vertical. The initial speed `upsilon_(0)` is equal to `sqrt((GM_(e))/(R_(e))`. How high does the projectile rise ? Neglect air resistance and the earth's rotation.
View SolutionA satellite of mass m is launched vertically upwards with an initial speed `sqrt((GM)/®)` from the surface of the earth. After it reaches height R (R = radius of the earth), it ejects a rocket of mass `m/10` in a direction opposite to the initial direction of the satellite, so that subsequently the satellite escapes to infinity. The minimum kinetic energy of the rocket at ejection needed is (G is the gravitational constant, M is the mass of the earth):
View SolutionThe intensity of radiation emitted by the sun has its maximum value at a wavelength of 510 nm and that emitted by the North star has the maximum value at 350 nm. If these stars behave like black bodies, then the ratio of the surface temperatures of the sun and the north star is
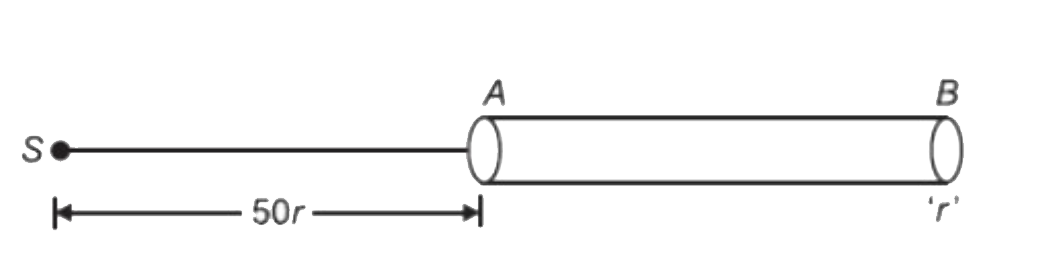
View SolutionA cylindrical rod of length l and cross - sectional radius r is placed at a distance of 50 r from a infrared point source S of power 1.25 kw as shown in the figure. The lateral surface of the rod is perfectly insulated from the surroundings . If the cross - section A absorbs 80 % of the incident energy and the temperature difference between the ends of the rod is constant , then the rate of heat flow through the rod in steady state is
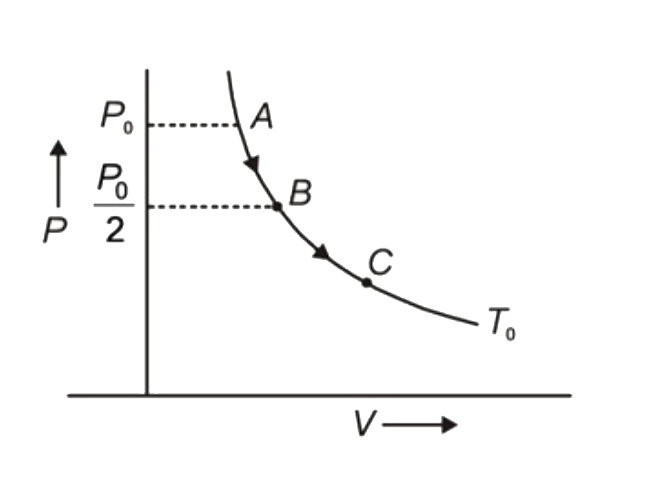
View SolutionThe state of an ideal gas changed as shown, at constant temperature `T_0` If heat supplied to the gas in process `BrarrC` is thrice of work done by gas in `ArarrB` , then the pressure of gas in state C is `P_0/(2n)` . Find the value of n.
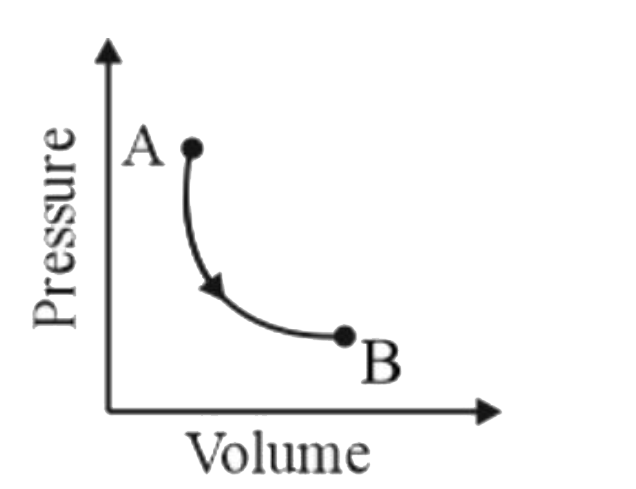
View SolutionThe pressure and volume of an ideal gas are related as ` p alpha 1/v^2` for process `A rarrB` as shown in figure . The pressure and volume at A are `3p_0 and v_0` respectively and pressure `B` is `p_0` the work done in the process `ArarrB` is found to be `[x-sqrt(3)]p_0v_0` find
View SolutionA cylindrical wire of radius R has current density varying with distance r form its axis as `J(x)=J_0(1-(r^2)/(R^2))` . The total current through the wire is
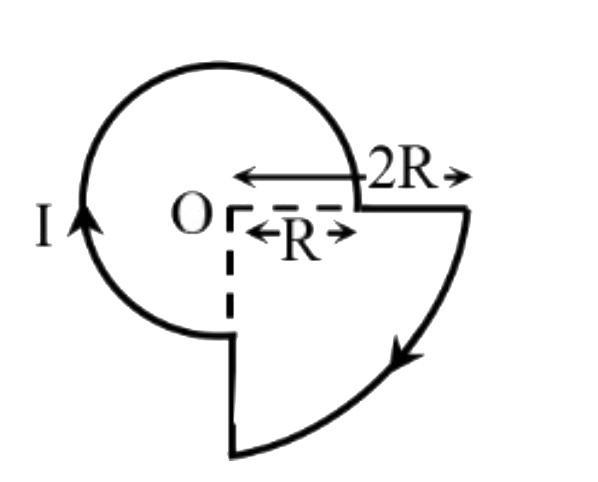
View SolutionA current I is flowing through the loop , as shown in the figure . The magnetic field at centre O is
View SolutionOn a particle moving on a circular path with constant speed `v`, light is thrown from a projectors placed at the centre of the circular path. The shadow of the particle is formed on the wall. The velocity of shadow up the wall is
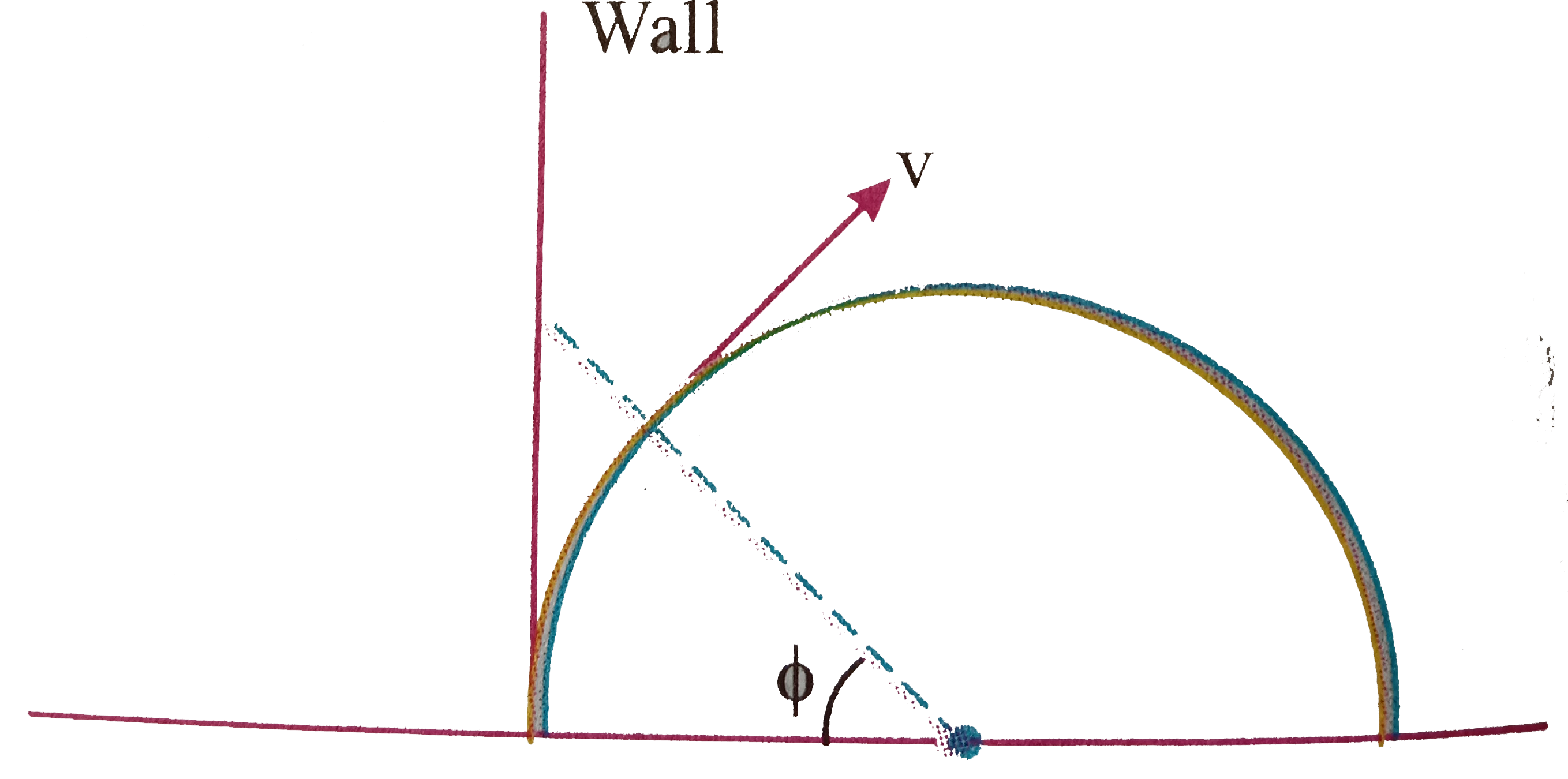
View SolutionA fighter plane enters inside the enemy territory, at time `t=0`, with velocity `upsilon_(o)=250m//s` a moves horizontally with constant acceleration `a=20m//s^(2)` (see figure) An enemy tank at the border, spot the plane and fire shots at an angle `theta=60^(2)` with the horizontal and with velocity `u=600 m//s`.At what altitude `H` of the plane it can be hit by the shot?
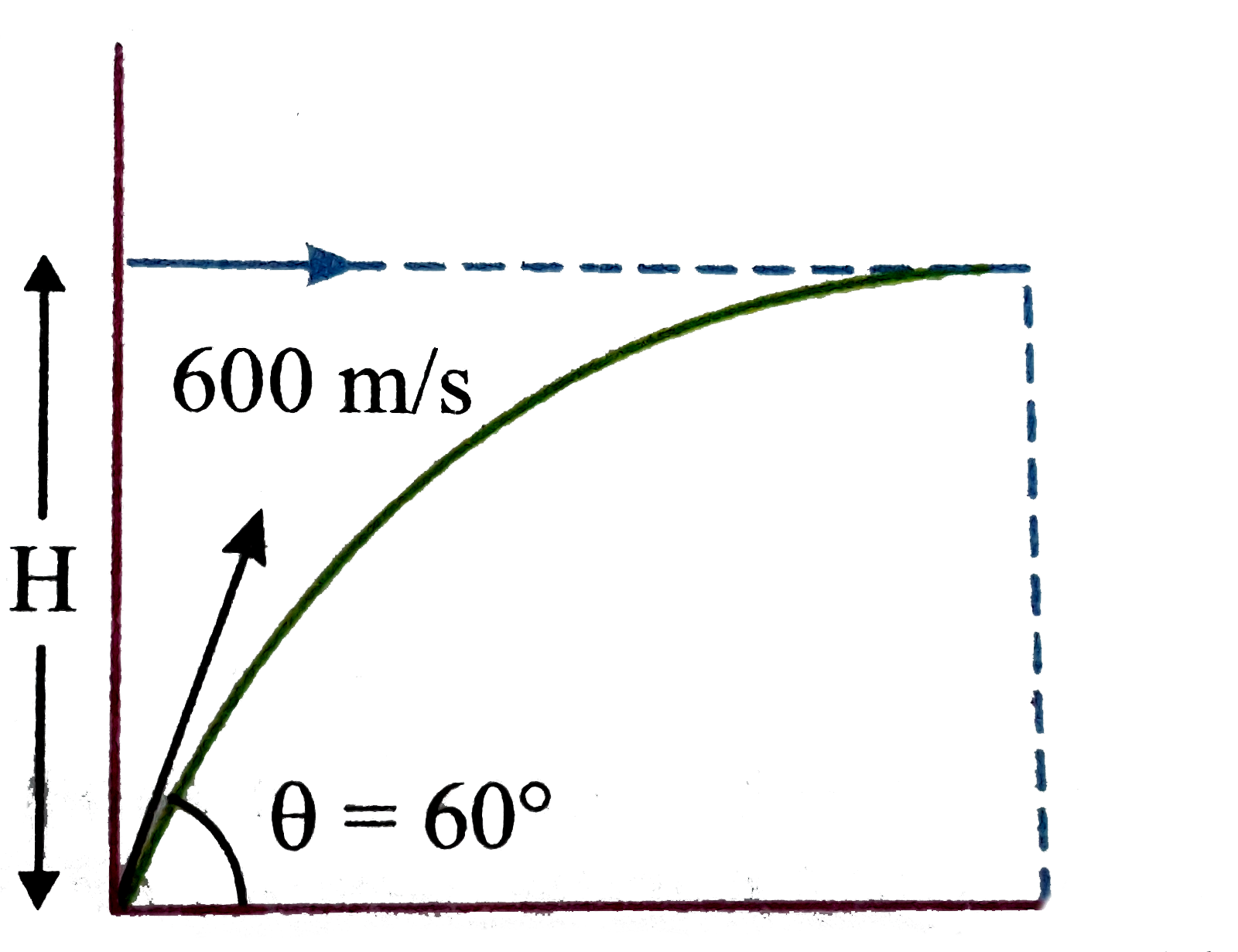
View Solution
Three blocks are connected as shown in the figure. Valculate the minimum force required to move the body with constant velocity. The coefficient of friction at all surfaces is 0.25.
View SolutionTwo blocks A and B of masses m and 2m, respectively , are held at rest such that the spring is in natural length. Find out the acceleration of both the blocks just after relese.
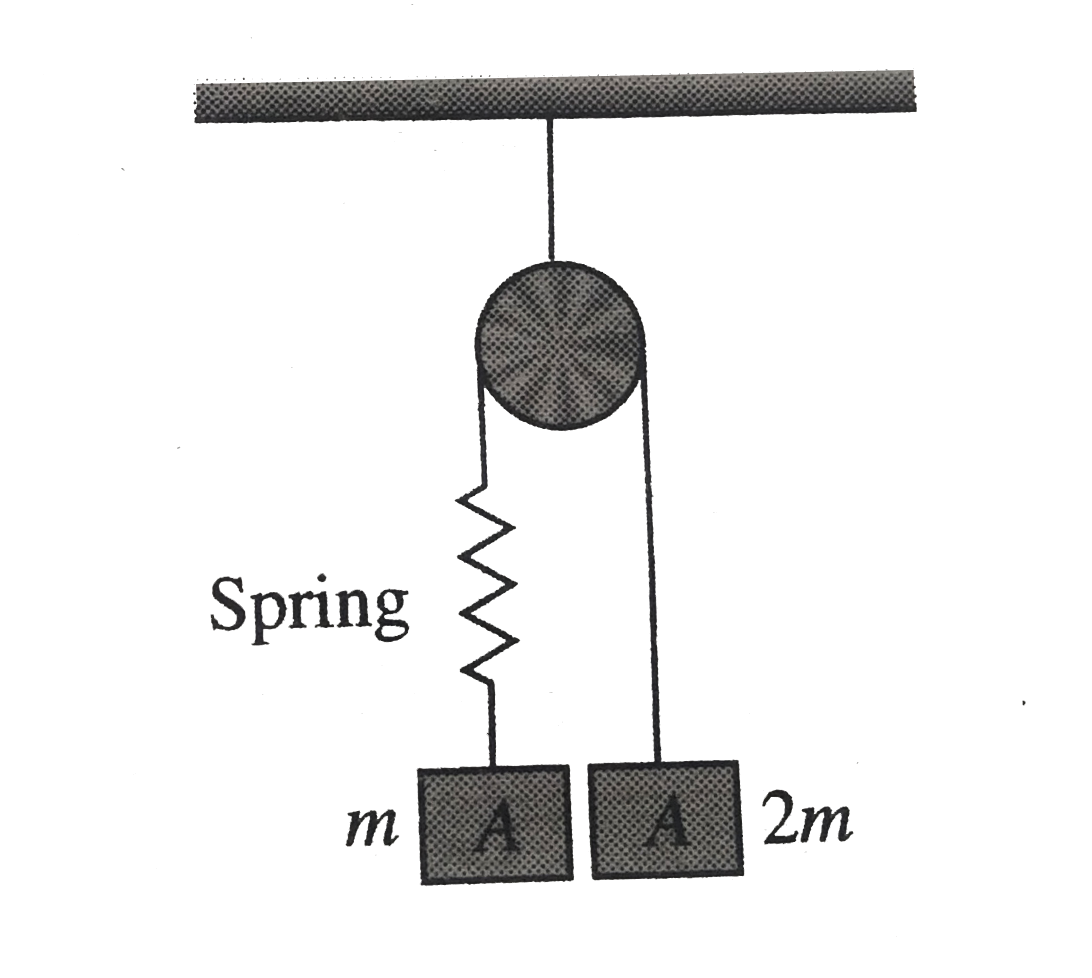
View SolutionA radioactive nucleus (initial mass number A and atomic number Z) emits `3alpha`-particles and 2 positrons. The ratio of number of neutrons to that of protons in the final nucleus will be
View SolutionNe nucleus , the after absorbing energy , decays into two `alpha` particle and an unknown nucleus . The unknown nucleus is
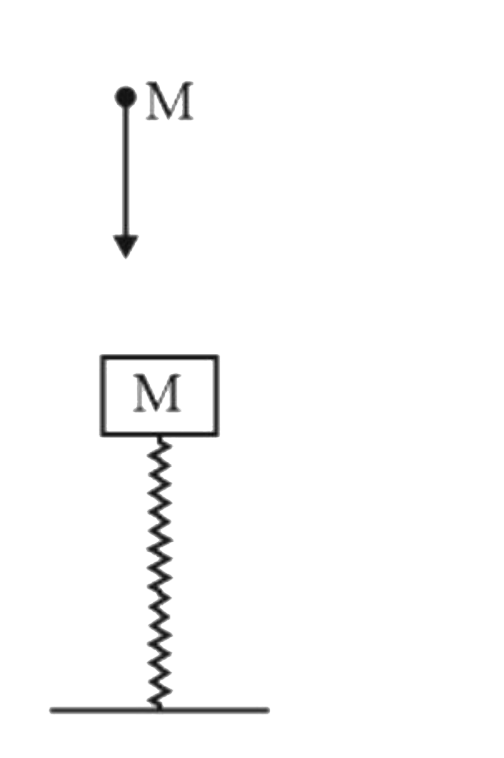
View SolutionAn ideal spring supports a disc of mass M. A body of mass m is released from a certain height from where it falls to hit M. The two masses stick together at the moment they touch and move together from then on . The oscillations reach to a height a above the original level of the disc and depth b blow. it The constant of the force of the spring is
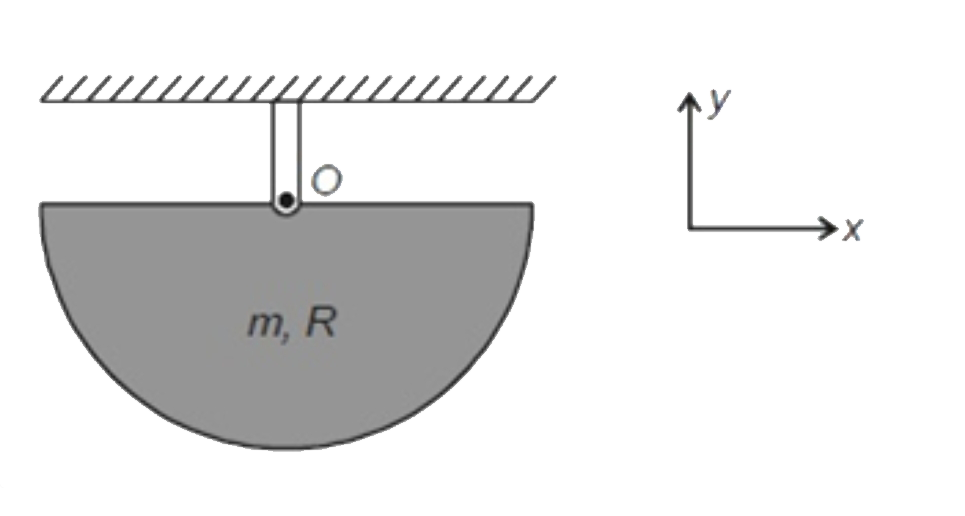
View SolutionTime period for small oscillations in vertical x - y plane of uniform semi - circular disk of mass m and radius R , about horizontal axis through point O, is equal to
View SolutionThe kinetic energy of the most energetic photoelectrons emitted from a metal surface is doubled when the wavelength of the incident radiation is reduced from `lamda_1 " to " lamda_2` The work function of the metal is
View SolutionWhen ultraviolet light is incident on a photocell, its stopping potential is `V_(0)` and the maximum kinetic energy of the photoelectrons is `K_(max)`. When X-rays are incident on the same cell, then:
View SolutionIn a U-tube the radii of two columns are respectively `r_(1) and r_(2)` and if a liquid of density d filled in it has level difference of h then the surface tension of the liquid is -
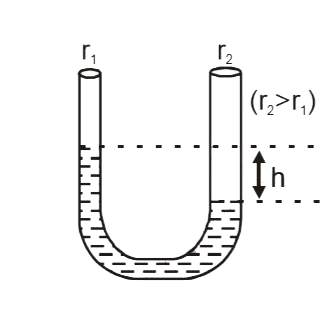
View SolutionAn open glass tube is immersed in mercury in such a way that a length of 8 cm extends above the mercury level. The open end of the tube is then closed and sealed and the tube is raised vertically up by additional 46 cm. what will be length of the air column above mercury in the above now ?
(Atmospheric pressure = 76 cm of Hg)
View SolutionConsider a concave mirror and a convex lens (refractive index 1.5) of focal length `10 cm` each separated by a distance of `50 cm` in air (refractive index = 1) as shown in the Fig. An object is placed at a distance of `15 cm` from the mirror. Its erect image formed by this combination has magnification `M_1`. When this set up is kept in a medium of refractive index `7//6`, the magnification becomes `M_2`. The magnitude `((M_2)/(M_1))` is :
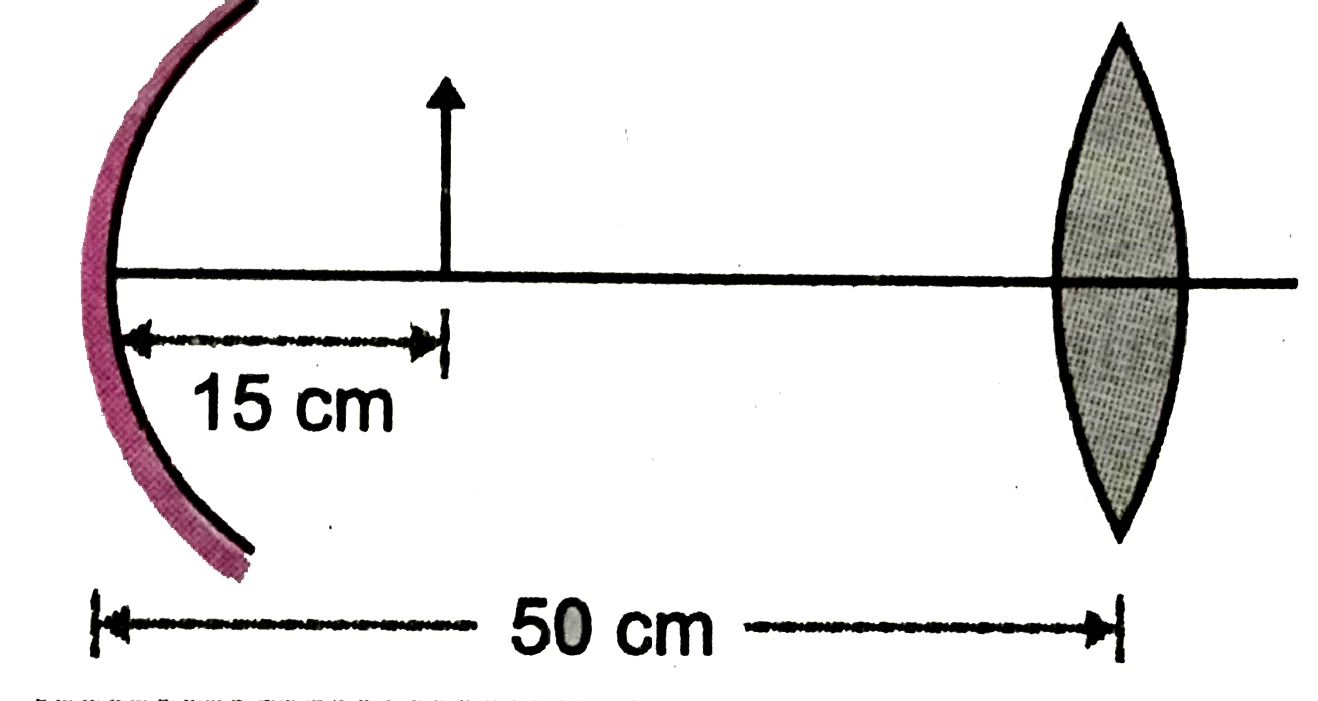 .
.
View SolutionA circular beam of light of diameter `d = 2 cm` falls on a plane refractive of glass. The angle of incidence is `60^(@)` and refractive index of glass is `mu = 3//2`. The diameter of the refracted beam is
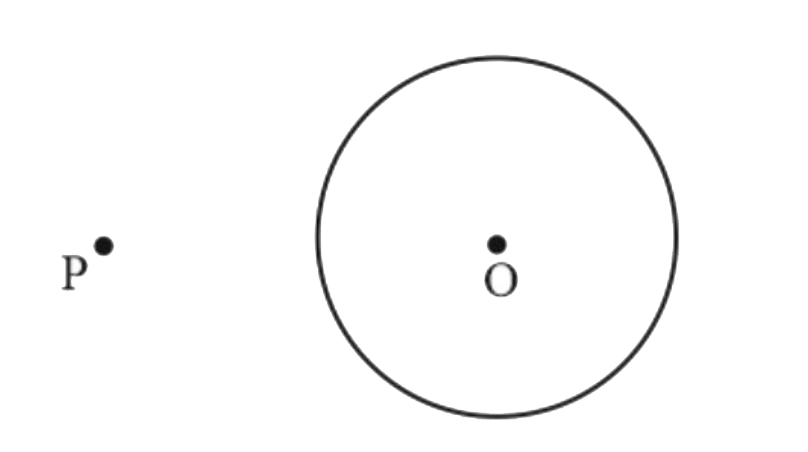
View SolutionConsider a particle , moving in a circle with constant speed . P is a point outside the circle , in the same plane . Then , the angular momentum of the particle about the point P is
View SolutionTwo particles A and B are moving , as shown in the figure .
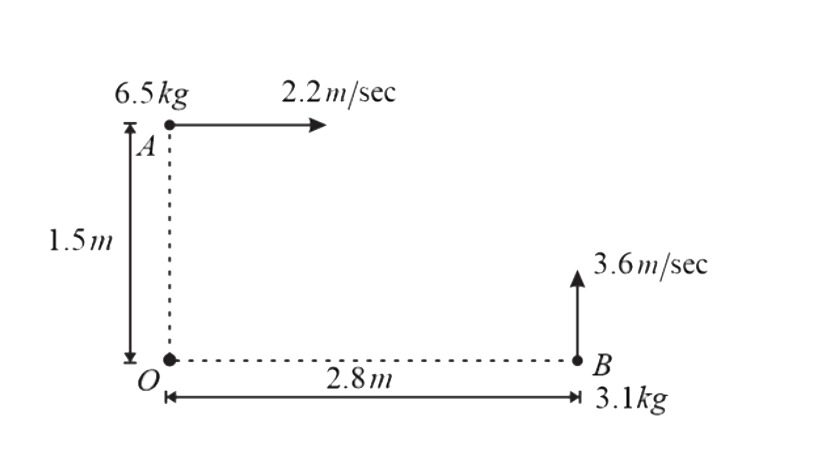
Their total angular momentum about the point O is
View SolutionThe value of transistor parameters , `beta and alpha` respectively, for the given figure is
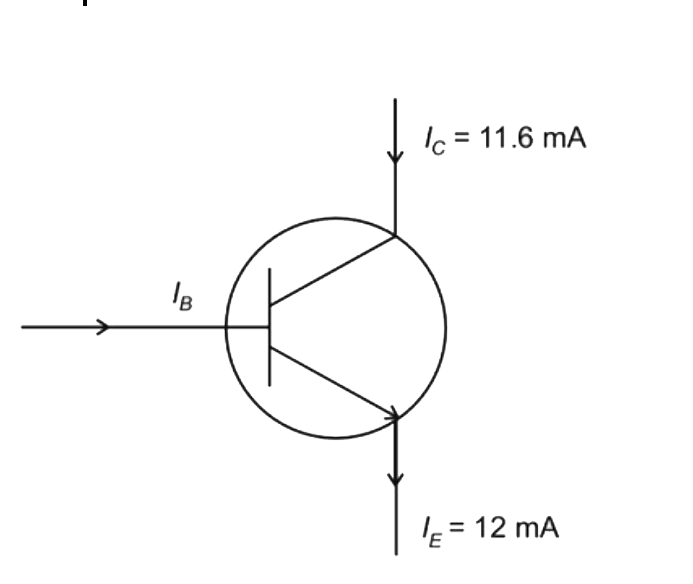
View SolutionFor a transistor , the correct statement is
View SolutionTemperature of 100 gm water is changed from `0^@C " to " 3^@C` . In this process , heat supplied to water will be (specified heat of water `1 cal g^(-1) .^@C^(-1))`
View SolutionIf the velocity of light c, gravitational constant G and Planck's constant h be taken as fundamental units the dimension of mass in the new system will be-
View SolutionA thin slice is cut out of a glass cylinder along a plane parallel to its axis. The slice is placed on a flat glass plate with the curved surface downwards. Monochromatic light is incident normally from the top. The observed interference fringes from the combination do not follow on of the following statements.
View SolutionIn the Young's double slit experiment, the intensities at two points `P_(1)` and `P_(2)` on the screen are respectively `I_(1)` and `I_(2)` If `P_(1)` is located at the centre of a bright fringe and `P_(2)` is located at a distance equal to a quarter of fringe width from `P_(1)` then `I_(1)/I_(2)` is
View SolutionA simple wave motion represented by `y=5(sin 4pi t+sqrt3 cos 4pit)`. Its amplitude is
View SolutionThe end correction of a resonance column is 1 cm. If the shortest length resonating with the tunning fork is 10 cm, the next resonating length should be :
View Solution
PHYSICS questions and complete solutions for chapter NTA NEET SET 41 of NTA MOCK TESTS of Class 12