Complete Solutions to MULTIPLE CHOICE QUESTIONS of chapter K-CET-MATHEMATICS-2015 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | K-CET-MATHEMATICS-2015 |
| SUNSTAR PUBLICATION | MULTIPLE CHOICE QUESTIONS |
| Number Of Questions | 60 |
| Academic Year | 2023-24 |
Write the set builder form `A= {-1,1}`
02:06View SolutionIf the operation `oplus ` is defined by `a oplus b = a^(2)+b^(2) ` for all real numbers 'a' and 'b' then `(2 oplus 3) oplus 4` = __________
01:17View SolutionIf `Z= ((sqrt3 +1)^(3) (3i + 4)^(2))/((8+ 6i)^(2))` then |Z| is equal to
03:17View Solution`f(x)= (1)/(2)- tan ((pi x)/(2)) -1 lt x lt 1 and g(x)= sqrt((3+4x-4x^(2)))`. Find domain of `(f + g)`
04:48View SolutionIf the `2^(nd) `and `5^(th)` terms of G.P. are 24 and 3 respectively then the sum of `1^(st)` six terms is `:`
02:04View SolutionThe middle term of expansion `(10/x+x/10)^(10)` is
01:28View SolutionIf `|(2a,x_(1),y_(1)),(2b,x_(2),y_(2)),(2c,x_(3),y_(3))| = (abc)/2 != 0 ` , then the area of the triangle whose vertices are `((x_(1))/a,(y_(1))/a),((x_(2))/b,(y_(2))/b)and ((x_(3))/c,(y_(3))/c)`
04:16View SolutionIf `alpha and beta ` are the roots of `x^(2)-ax+b^(2)=0` , then `alpha^(2) +beta^(2)` is equal to_______
02:26View Solution`~[(~p)^^q]` is logically equivalent to :
01:59View SolutionThe value of `sin^(-1) ((2 sqrt2)/(3)) + sin^(-1) ((1)/(3))` is equal to
02:41View SolutionThe shaded region shown in fig. is given by the inequation
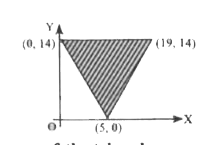 10:28View Solution
10:28View SolutionIf `veca=hati + 2 hatj + 2hatk, |vecb|=5` and angle between `veca and vecb` is `(pi)/(60,` then the area of the triangle formed by these two vectors as two side is :
01:08View SolutionIf `vec(a)=i-2 j + 3k, vec(b)` is a vector such that `vec(a).vec(b)= |vec(b)|^(2) |vec(a)-vec(b)|= sqrt7`, then `|vec(b)|`= ________
04:51View SolutionIf direction cosines of a vector of magnitude 3 are `(2)/(3), -(a)/(3), (2)/(3) and a gt 0`, then vector is ________
02:27View SolutionIf the eccentricity of the hyperbola `(x^(2))/(a^(2))-(y^(2))/(b^(2))=1` is `(5)/(4) and 2x+3y-6=0`
is a focal chord of the hyperbola, then the length of transverse axis is equal to _________03:11View SolutionFoot of perpendicular drawn from the origin to the plane `2x-3y + 4z=29` is_________
03:47View SolutionIf two dice are thrown simultaneously, then the probability that the sum of the numbers which come up on the dice to be more than 5 is_________
04:11View SolutionIf `f(y)=f(x^(2)+2)` and `f'(3)=5`, then `(dy)/(dx)` at `x=1` is :
01:18View SolutionIf `x= a cos^(3) theta, y= a sin^(3) theta`, then `1 + ((dy)/(dx))^(2)` is______
02:41View SolutionEquatin of line passing through the point (2,3,1) and parallel to the line of intersection of the planes x−2y−z+6=0 and x+y+3z=5 is
02:06View Solution`int (1)/(x^(2)(x^(4)+1)^(3//4))dx` is equal to___________
03:24View SolutionIf `f: R rarr R` is defined by `f(x)=(x)/(x^(2)+1)` find f(f(2))
04:29View SolutionEvalute `|(cos 15^(@) sin 15^(@)),(sin 75^(@) cos 75^(@))|`
01:27View SolutionSlope of normal to the curve `y=x^(2)-(1)/(x^(2))` at `(-1, 0)` is
02:25View Solution`underset(0)overset(pi//4)int log ((sin x+cos x)/(cos x))dx`
06:03View SolutionArea bounded by `y=x^(3),y= 8 and x=0` is _______
06:11View SolutionLet `veca = hati + hatj + hatk, vecb = hati - ahtj + hatk and hati - hatj - hatk ` be three vectors. A vector `vecv` in the plane of `veca and vecb,` whose projection on `vecc` is `(1)/(sqrt3),` is given by :
02:50View SolutionThe mean deviation of the data 3, 10, 10, 4, 7, 10, 5 from the mean is :
03:52View SolutionA man takes a step forward with probability 0.4 and one step backward with probability 0.6, then the probability that at the end of eleven steps he is one step away from the starting point is
08:59View SolutionIf the function g(x) is defined by `g(x)= (x^(200))/(200) + (x^(199))/(199)+ …..+ (x^(2))/(2)+ x + 5`, then g'(0)=________
04:08View SolutionA box contains o red marbles number from 1 through 6 and 4 white marbles 12 through 15 .Find the probability that a marble drawn at random is white and old number :
01:06View Solution`lim_(x rarr 0) (1- cos x)/(x^(2))` is :
01:20View SolutionThe probability distribution of x is

Find the value of k03:01View SolutionIf `f(x)=2x^(2)`, find `(f(3.8)-f(4))/(3.8-4)` :
01:26View SolutionIf x=ct and `y= (c )/(t)`, find `(dy)/(dx)` at t=2
04:16View SolutionA balloon which always remains spherical is being inflated by pumping in 10 cubic centimeters of gas per second. Find the rate at which the radius of the balloon is increasing when the radius is 15cm.
06:49View Solution`f(x)= {(3x-8,"If " x le 5),(2k,"if" x gt 5):}` is continuous, find k
02:34View Solution`int e^(x)(1+sin x)/(1+cos x)` dx is equal to
02:49View SolutionIf `1, w, w^(2)` are three cube roots of unity, then `(1 - w+ w^(2)) (1 + w-w^(2))` is _______
05:15View SolutionSolve for x `tan^(-1) ((1-x)/(1+x))=(1)/(2) tan^(-1)x, x gt 0`
09:59View SolutionThe system of linear equations :
`x+y+z=6,x+2y+3z=1 and x+2y+az=6` has no solutions when :04:05View SolutionEvaluate `int(sin^(2)x)/(1+cosx)dx`
03:13View SolutionIf `((x+1)^(2))/(x^(3)+x)=(A)/(x)+(Bx+C)/(x^(2)+1)`, then `"cosec"^(-1) ((1)/(A)) + cot^(-1) ((1)/(B))+sec^(-1)C=`______
07:13View SolutionThe remainder obtained when `1! + 2! + 3!+….+ 11!` is divided by 12 is_______
04:06View SolutionIf `alpha le 2 sin^(-1) x + cos^(-1) x le beta`, then
05:14View SolutionIf `A= [(0,1),(1,0)]`, then `A^(2)` equal to______
02:42View SolutionThe value of `tan (1^(@)) + tan (89^(@))` is _______
02:34View SolutionIf `y= log ((1-x^(2))/(1+ x^(2)))`, then `(dy)/(dx)` is equal to _______
06:24View SolutionThe two curves `x^(3)-3xy^(2)+2=0 and 3x^(2)y-y^(3)-2=0`:
02:00View SolutionIf x is real, the minimum value of `x^(2)-8x+17` is :
01:04View Solution`underset(-pi//4)overset(pi//4)int (dx)/(1+cos 2x)` is equal to
07:25View SolutionThe function `f(x)= [x]`, where [x] denotes greatest integer function is continuous at __________
03:23View SolutionThe solution of differential equation :
`x(dy)/(dx)+2y=x^2` is :01:52View SolutionIf `sin x + sin y= (1)/(2) and cos x + cos y=1`, then `tan (x+y)` =______
05:01View SolutionIf `A= [(alpha,2),(2,alpha)] and |A^(3)|= 27`, then `alpha`= _______
02:03View SolutionThe order of differential equation of all circles of given radius 'a' is _______
08:23View SolutionA line passes through (2,2) and is perpendicular to the line `3x+y=3`, its y-intercepts is__________
03:45View SolutionLet `f: R rarr R` be defined by `f(x)= (1)/(x) AA x inR`, then f is _______
03:19View SolutionThe solution set of the inequation `(x^(2) + 6x-7)/(|x+4|) lt 0` is ______
07:21View SolutionIf `P= |(x,1),(1,x)| and Q= |(x,1,1),(1,x,1),(1,1,x)| " then " (dQ)/(dx)=` _______
04:23View Solution
MULTIPLE CHOICE QUESTIONS questions and complete solutions for chapter K-CET-MATHEMATICS-2015 of SUNSTAR MATHS (KANNADA ENGLISH) of Class 12
