Complete Solutions to MCQs of chapter K-CET- MATHEMATICS - 2017 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | K-CET- MATHEMATICS - 2017 |
| SUNSTAR PUBLICATION | MCQs |
| Number Of Questions | 59 |
| Academic Year | 2023-24 |
If A and B are finite sets and `A sub B` then
03:06View SolutionThe value of `cos^(2)45^(@)-sin^(2)15^(@)` is
03:16View Solution`3+5+7+` …..sum to n terms
02:15View SolutionIf `((1+i)/(1-i))^(m)=1`, then find the least positive integral value of m.
03:50View SolutionIf `|x-2|le 1`, then
01:44View SolutionIf `""^nC_(12)=""^nC_8` , then n is equal to :
02:08View SolutionThe total number of terms in the expansion of `(x+a)^(47)-(x-a)^(47)` after simplification is
07:55View SolutionEquation of line passing through the point (1, 2) and perpendicular to the line `y=3x-1` is
06:13View SolutionThe eccentricity to the ellipse `(x^(2))/(36)+(y^(2))/(16)=1` is
04:38View SolutionThe perpendicular distance of the point P (6, 7, 8) from XY - plane is
03:34View SolutionThe value of `lim_(theta to 0)(1-cos 4theta)/(1-cos 6 theta)` is
05:09View SolutionWrite the contrapositive and converse of the following statements.
If x is a prime number, then x is odd.01:16View SolutionIf the coefficient of variation and standard deviation are 60 and 21 respectively, the arithmetic mean of distribution is
01:41View SolutionThe range of the function `f(x)=sqrt(9-x^(2))` is
04:06View SolutionLet f : R `to` R be defined by `f(x)=x^(4)`, then
08:17View SolutionThe range of `sec^(-1)x` is
01:23View SolutionIf `tan^(-1)x + tan^(-1)y=4pi//5`, then `cot^(-1)x + cot^(-1)y` is equal to
04:37View SolutionIf `f(x)=8x^(3), g(x)=x^(1//3)`, then fog (x) is
02:52View SolutionIf `A = 1/pi [(sin^(-1)(xpi),tan^(-1)(x/pi)),(sin^(-1)(x/pi),cot^(-1)(pix))]`
`B = 1/pi [(-cos^(-1)(xpi),tan^(-1)(x/pi)),(sin^(-1)(x/pi),-tan^(-1)(pix))] `
then `A-B ` is equal to :02:12View SolutionIf the matrix A is both symmetric and skew symmetric, then
02:55View SolutionFind x and y, if `2[(1,3),(0,x)]+[(y,0),(1,2)]=[(5,6),(1,8)]`
03:07View SolutionBinary operation * on R `-{-1}` defined by `a ** b = (a)/(b+1)` is
05:29View Solutionfind the value of x for which `|(3,x),(x,1)|=|(3,2),(4,1)|`
03:54View SolutionIf A is a square matrix of order `3xx3`, then |KA| is equal to
05:36View SolutionThe area of triangle with vertices (K, 0), (4,0), (0, 2) is 4 square units, then value of K is
03:23View SolutionLet `Delta=|(Ax,x^(2),1),(By,y^(2),1),(Cz,z^(2),1)|` and `Delta_(1)=|(A,B,C),(x,y,z),(zy,zx,xy)|` then
05:31View SolutionIf `f(x)={{:(Kx^(2),"if x" le 2),(3,"if x" gt 2):}}` is continuous at x = 2, then the value of K is
05:01View SolutionThe value of C in mean value theorem for the function `f(x)=x^(2)` in [2, 4] is
05:24View SolutionThe point on the curve `y^(2)=x`, where the tangent makes an angle of `(pi)/(4)` with x-axis is :
01:21View SolutionThe function `f(x)=x^(2)+2x-5` is strictly increasing in the interval
03:16View SolutionThe rate of change of volume of a sphere with respect to its surface area when the radius is 4 cm is
05:24View SolutionIf `y=tan^(-1)((sin x + cos x)/(cos x - sin x))`, then `(dy)/(dx)` is equal to
04:29View SolutionIf `y=|(f(x),g(x),h(x)),(1,m,n),(a,b,c)|`, then `(dy)/(dx)` is equal to
05:37View SolutionIf `sin x = (2t)/(1+t^(2)), tan y = (2t)/(1-t^(2))`, then `(dy)/(dx)` is equal to
02:47View SolutionThe derivative of `cos^(-1)(2x^(2)-1)` w.r.t `cos^(-1)` x is
06:09View SolutionIf y = log (log x) then `(d^(2)y)/(dx^(2))` is equal to
05:30View Solution`int((x+3)e^(x))/((x+4)^(2))dx` is equal to
06:47View SolutionEvalute : `int (cos 2 x - cos2 alpha)/(cos x - cos alpha)dx`.
02:17View Solution`int sqrt(x^(2)+2x+5)dx` is equal to
07:38View Solution`int_(0)^(pi//2)(tan^(7)x)/(cot^(7)x + tan^(7)x)dx` is equal to
05:54View Solution`int_(-5)^(5)|x+2|dx` is equal to
05:42View Solution`int_(-pi//2)^(pi//2)(dx)/(e^(sin x)+1)` is equal to
05:10View Solution`int_(0)^(pi//2)(1)/(a^(2).sin^(2)x+b^(2).cos^(2)x)dx` is equal to
07:31View SolutionFind the area of the region bounded by the curve `y = x^(2)` and the line y = 2.
03:03View SolutionArea of the region bounded by the curve y = cos x, x = 0 and `x = pi` is
06:04View SolutionThe degree of the differential equation `[1+((dy)/(dx))^(2)]^(2)=(d^(2)y)/(dx^(2))`
02:58View SolutionGeneral solution of differential equation `(dy)/(dx)+y=1(y ne 1)` is
01:49View SolutionThe integrating factor of the differential equation `x.(dy)/(dx)+2y=x^(2)` is `(x ne 0)`
02:29View SolutionIf `vec(a)=2hat(i)+lambda hat(j)+hat(k)` and `vec(b)=-hat(i)+2hat(j)-3hat(k)` are orthogonal, then value of `lambda` is
02:02View SolutionIf `vec(a),vec(b),vec(c )` are unit vectors such that `vec(a)+vec(b)+vec(c )=0`, then the value of `vec(a).vec(b)+vec(b).vec(c )+vec(c ).vec(a)` is equal to
02:32View SolutionIf `veca and vec b` are unit vectors, then what is the angle between `veca and vecb` for `sqrt3 veva - vecb` to be a unit vectors ?
03:08View SolutionReflexion of the point `(alpha, beta, gamma)` in XY plane is
05:11View SolutionThe plane `2x-3y+6z-11=0` makes an angle `sin^(-1)(alpha)` with X - axis. The value of `alpha` is equal to
05:00View SolutionThe distance of the point (-2, 4, -5) from the line `(x +3)/(3)= (y - 4)/(5)= (z +8)/(6)` is
05:19View SolutionA box has 100 pens of which 10 are defective. The probability that out of a sample of 5 pens drawn one by one with replacement and atmost one is defective is
05:35View SolutionTwo events A and B will be independent if
03:11View SolutionThe probability distribution of x is
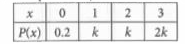 01:00View Solution
01:00View SolutionIf an LPP admits optimal solution at two consecutive vertices of a feasible region, then
00:45View Solution`int_(0.2)^(3.5)[x]dx` is equal to
05:38View Solution
MCQs questions and complete solutions for chapter K-CET- MATHEMATICS - 2017 of SUNSTAR MATHS (KANNADA ENGLISH) of Class 12
