Complete Solutions to MATHEMATICS of chapter TS EAMCET 2018 (7 MAY SHIFT 1) of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | TS EAMCET 2018 (7 MAY SHIFT 1) |
| TS EAMCET | MATHEMATICS |
| Number Of Questions | 80 |
| Academic Year | 2023-24 |
If `f:[0, oo) to [0,oo)` is defined by `f (x) = (x)/(1+ x) ,` then f is
View SolutionThe range of the function `f (x) =- sqrt( -x ^(2) -6x -5)` is
View SolutionIf `A = [{:(1,1),(0,1):}] and I = [{:(1,0),(0,1):}],` then for all `n in N`
View SolutionThe rank of the matrix `[{:(3,2,1,-4),(2,3,0,-1),(1,-6,3,-8):}]` is
View SolutionLet A,B,C be `3 xx3` non-sinigular matrices and I be the identity matrix of order three. If `ABA=BA ^(2)B and A ^(3)=I,` then `AB^(4) - B^(4)A=`
View SolutionIf the system of equation
`x+y+2z =3, x + 2y + 3z =4 and x + cy + 2xz =5` is inconsistent, then
View SolutionIf `z =x + iy` is complex number satisfying `|(z-2i)/(z + 2i)|=2` and the locus of z is a circle, then its radius is
View SolutionIf `(x-iy ) ^(1/3) = a + ib,` then `(ax -by)/(a-b) =`
View SolutionIf `z =x` is is a complex number and `|1 + iz|=|1-iz|,` then
View SolutionThe sum of the least positive arguments of the distinct cube roots of the complex number `(1-i sqrt3) is
View SolutionLet R-`(alpha, beta),` be the range of `(x +3)/((x -1) (x +2)).` Then the sum of the intercepts of the line `alphax + beta y + 1 =0` on the coordinate axis is
View SolutionIf `alpha, beta `are the roots of the equation `x ^(2) + 5x+ 2=0,` then `((alpha )/(2 + 5 alpha ))^(2)+ ((beta)/(2 + 5 beta))^(2) =`
View SolutionIf the roots of
`x ^(4) -10 x ^(3) + 37 x ^(2) - 60 x + 36 =0` are ` alpha, alpha , beta, beta (alpha lt beta), ` then `2 alpha + 3 beta - 2 alpha beta=`
View SolutionThe polynomial equation of degree 5 whose roots are the translates of the roots of `x ^(5) - 2x ^(4) + 3x ^(3) -4x ^(2) + 5x -6=0 by -2` is
View SolutionThe number of natural numbers less than 1000, in which no two digits are repeated is
View SolutionThe coefficient of `x ^(3)` in the expansion of `(1-2x)/((2x +1)(2-x))` is
View SolutionIf the coefficient of rth, (r + 1)th and (r+ 2)th terms in the expansion of `(1 + x) ^(n)` are respectively in the ratio `2:4:5,` then (r,n)=
View SolutionIf n is positive integer greater than 1, then `3 (""^(n) C_(0)) -8(""^(n) C_(1)) + 13 (""^(n)C_(2)) +…` upto `(n+1)` terms =
View Solution`(3x-2)/((x +1) ^(2) (x +2))=(A)/(x+1) + (B)/((x +1)^(2))+ (C )/(x +3),` then ` A + B+C` =
View SolutionIf `sin theta + sin ^(2) theta =1 and cos ^(12) theta + a cos ^(10) theta + bcos ^(8) theta + c cos ^(6) theta + d =0,` then
View Solution`cos 20^(@)* cos 40^(@) * cos 60^(@) * cos 80^(@) = `
View Solution`If A+B +C= pi /4` then
` 4 cos ""A/2 cos ""B/2 cos ""C/2 - cos ""pi/8=`
View SolutionLet `A = {x in, R//|sqrt3 cos x - sin x| ge 2,0 le x le 2pi}.If x _(1) in A, x_(2) in A,` then `(x _(1))/(x _(2))=`
View SolutionAll the values of x satisfying the equation `2 tan^ (-1) 2x = sin ^(-1)""((4x )/(1+ 4 x ^(2)))` lie in the interval
View Solution`sin h ^(-1) 2 + cos h ^(-1) 2- tanh ^(-1) ""(2)/(3) + cot h ^(-1) (2)=`
View SolutionThe greatest angle of the triangle whose sides are `x ^(2) + x + 1,2x + 1 and x ^(2) -1` is
View SolutionIn a `Delta ABC,` if `a:b:c=4:5:6,` then the ratio of the radius of its circumcircle to that of its incircle
View SolutionIn triangle ABC, D is mid point of BC, if 'AD' is perpendicular to 'AC then `cos A cos C`
View SolutionThe position vectors of three points A,B and C `(1,3,x ) (3,5,8) and (y,-1,-6) ` respectively. IF A, B and C are collinear, then (x,y) =
View SolutionA line L passes through the poins `hati+ 2 hatj + hatk and - 2 hati + 3 hatk.` A plane P passes through the origin and the points `4 hatk, 2 hati + hatj.` The point where the line L meets the plane P is
View SolutionIf P, Q, R are the mid-point of the sides AB, BC and CA of `Delta ABC` respectively, then `PC-BQ=`
View SolutionLet `a = hati + hatj, b = hatj+ hatk and c = hati+ hatk.` If d is unit vector such that a.d = 0 and b . `(c xxd)=0 and d= `
View SolutionIf `a= hati + hatj and b = 3 hati - 2 hatj,` then the vector r satisfying the equation `r xxa =b xxa and r xxb = a xx b` is
View SolutionThe shortest distance between the lines
`r = (3t-4) hati -2thatj -(1+ 2t) hatkand `
`r ( 6 + s) hati + (2- 2s) hatj + 2 (1 + s) hatk` is
View SolutionLet `sigma_(1) , sigma _(2)` be the standard deviations of two distributions `D _(1)and D_(2)` respectively and `D_(1)` be more consistent then `D_(2)` If the means of `D_(1) and D_(2)` are same, then the percentage increase in the standard deviation of `D_(2)` over the standard deviation of `D_(1)` is
View SolutionConsider the following distribution

The sum of the mean deviation from the mean and the mean deviation from median of this distribution is
View SolutionTen persons with badges numbered 1 to 10 are in a room. If three of them are asked to leave the room. Then, the probability to have the person with the smallest badge number as 5 among the three persons that left the room, is
View SolutionA bag P contains 3 blue and 5 red balls. Another bag Q contains 4 blue and 6 red balls. A ball is drawn at random from one of the bags and is found to be red. The probability that it is from bag Q is
View SolutionMatch the items from List-I that of List-II A bag contains 4 red , 3 white and 5 blue balls. Three balls are drawn at a time randomly from the bag.

The correct answer is
View SolutionIn a game of throwing 3 coins, a plater will loose Rs.5/-for each head and gain Rs.10/-for each tail. IF a random variable `X:StoR` is defined as X(a)=net gain (a E s) the mean of random variable is (in rupees)
View SolutionA person fails 4 times in a game when he plays 9 times. If he plays 15 times, the probability of having success at most one is
View SolutionIf `A = (-1,2) and B=(1,-2)` are two points and P is a variable point such that the area of `Delta PAB` is always one, then the equation of the locus of P is
View SolutionThe point `(4,1)` undergoes the following transformations successively
I. Reflection about the line `y =x`
II. Translation through a distance 2 units in the direction of positive X-axis.
III. Rotation through an angle `pi/4` about origin in the anticlock wise direction.
Then, the final position of the point is
View SolutionThe image of the point `(2,4)` with respect to the straight line `2x + 3y -6 =0` is
View SolutionThe equation of the base of an equilateral triangle is `12 x + 5y-65=0.` If one of its verices is `(2,3)` then the length of the side is
View SolutionA triangle is formed by Y-axis, the straight line L passing through the points `(3,0)(1, (4)/(3))` and the straight line perpendicular to the line L and passing through the point `(8,1).` Then, the area of the triangle (in sq units) is
View SolutionFor `c ne 0, c ne 1` if the straight line
`x + y =1,2 x -y =c and bx +2by=c` have one common point, then
View SolutionIf the lines represented by `x ^(2) - 2hxy - y ^(2) =0 ` are rotated about (0,0) through an angle `alpha` one in clockwise direaction and the other in the counter clockwise direction, then the combined equation of the bisectors of the angle between the lines thus obtained is
View SolutionThe normal to a circle `S =0 at P (1,3) is x + 2y -7` and it has another normal at `Q(3,5)` which is the polar of the point ` A(7, -(1)/(2))` with respect to the circle `x ^(2) + y ^(2)- 4x + 6y -12 =0.` Then the equation of the circle S =0 is
View SolutionIf `x + ky -4 =0 and x+ y -5=0` are conjugate lines with respect to the circle
`(x -1) ^(2) + (y-1) ^(2) =3,` then ` k = `
View SolutionThe length of the chord intercepted by the circle `x ^(2) + y ^(2) + 2x + 4y =20=0` on the line `3x + 4y -6=0` is
View SolutionLet Q be a point on the circle `B : x ^(2) + y ^(2)=a ^(2)` and p(h,k) be a fixed point. If the locus of the point which divides the join of P and Q in the ratio p:q is a circle C, then the centre of C is
View SolutionThe equation of the circle passing through (2a,0) and whose radical axis w.r. to the circle ` x^(2) +y^(2) = a^(2) ` is ` x = a//2` is
View SolutionIf two distinct chords drawn from the point `A (4,4)` on the parabola `y ^(2) =4x` are bisected by the lince `y = ax,` then the interval in which a lies is
View SolutionThe locus of the mid-point of the line segment joining the focus to a moving point on the parabola, `y ^(2 ) = 4ax ` is a conic. The equation of the directrix of that conic is
View SolutionFor the ellipse `4x ^(2)+ y ^(2) - 8x + 2y + 1=0,` the focus and the equation of the directrix are respectively
View SolutionIf `4x + y+ p= 0( p gt 0)` is tangent to the ellipse `x ^(2) + 3y ^(2) =3 and 16x + qy + 14 =0 (1 gt 0)` is a normal to the ellipse `x ^(2) + 8y ^(2) =33,` then `p + q=`
View SolutionThe locus of the mid-points of the chords of the circle `x^(2) + y^(2) = 16` which are the tangents to the hyperbola `9x^(2) - 16y^(2) = 144` is
View SolutionIf the line segment joining the points `P (2,4,1) and Q (3,8,1)` is divided by the plane `3x -ky-6z=0` externally in the ratio `4 :5,` then k =
View SolutionIf the directin cosines of two lines satisfy the equation `l + m + n =0 and 2lm + 2ln - m n =0,` then the acute angle between those two lines is
View SolutionThe perpendicular distance of the point `(1,-1,2)` from the plane `x + 2y + z=4,` is
View SolutionIf f (x) satisfies `97 f (x) + mf ((1)/(x)) =0,` where `f (x) = lim _( n to oo) n(x ^(1//n)-1). X gt 0,` then the value of m is
View SolutionIf `f (x) = {{:((sqrt(1 + ax )- sqrt(1- ax ))/(x)"," -1 le x lt 0), ((x ^(2) +2)/(x-2)"," 0 le x le 1):}`
continuous on `[-1,1],` then a =
View SolutionThe function that is not differentiable at `x =1` is
View Solutionmatch the items of List-I with those of List-II.
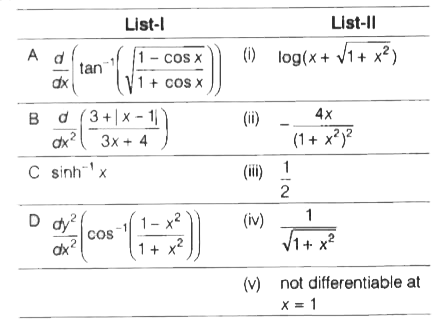
View SolutionIf `y = 2 cos (2 log x) + 3 sin (2log x),` then `x ^(2) y^(2) + xy^(1)+ 2y=`
View SolutionConsider the following statements A is relative error in the area of a square when the relative error in its side os `0.4` B is relative error in the volume of a sphere when the relative error in its radius is `0.3` C is relative error in the surface area of a closed cylinder whose height is equal to its radius, when the relative error in its height is `0.2` D is approximate error in `y = x ^(2)+ x-3` when `x =2 and delta x =0.1`
The ascending order of the values of errors in these statements is
View SolutionIf the curves `(x ^(2))/(4) + (y ^(2))/(9) =1 and (x ^(2))/(16) -(y ^(2))/(k)=1` cut each other orthogonally, then k =
View SolutionThe value C of the Lagrange's mean value theorem for the function `f (x) =x (x - 2)` in the interval `[0,(1)/(2)]` is
View SolutionIf Q is the point on the parabola `y ^(2) =4x` that is nearest to the point `P (2,0)` then PQ =
View SolutionIf `int sin ^(-1) ( sqrt((x)/(a + x)))dx =A (x)+` constant, then `A (x) =`
View Solution`int (cos 2 x. sin 4x)/(cos ^(4) x(1+ cos ^(2) 2x))dx =`
View Solution`int (log x) ^(3) x ^(4) dx`, where ` rho = log x`
View SolutionIf `int (sqrt3)/(sqrtx- root 3x) dx =x + Ex ^(5//6) + Dx ^(1//2) + Bx ^(1//3) + Ax^(1//6)+ log (root6x-1)^(6) +K,` then `A +B+C+D+E=`
View Solution`int _(0) ^(1//2)[sin 4 pix] dx=`
View SolutionIf `f(n)= 1/n {(n+1)(n+2)....2n}^(1//n)` then `{:(" "Lt),(n rarr oo):} f(n)=`
View SolutionThe area of the region bounded by `y = |x| and y =1 - |x|` is
View SolutionConsider the following differential equations.
`D_(1) : y=4((dy)/(dx) )^2+ 3x , D_(2), (d ^(2)y)/(dx ^(2))= (3+ ((dy)/(dx)) ^(2) )^(4/3)D_(3):[1+ ((dy)/(dx))]^(2) = ((dy)/(dx)) ^(2)`
The ratio of the sum of the orders of `D_(1),D_(2)and D_(3)` to the sum of their degrees is
View SolutionThe general solution fo the differential equation `(dy)/(dx)=1 + x +y + xy` is
View SolutionThe general solution of the differential equation `(1 + y^(2)) dx = (tan ^(-1) y-x)dy` is
View Solution
MATHEMATICS questions and complete solutions for chapter TS EAMCET 2018 (7 MAY SHIFT 1) of TS EAMCET PREVIOUS YEAR PAPERS of Class 12