Complete Solutions to MATHEMATICS of chapter AP EAMCET SOLVED PAPER 2019 (22-04-2019, SHIFT-2) of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | AP EAMCET SOLVED PAPER 2019 (22-04-2019, SHIFT-2) |
| TS EAMCET | MATHEMATICS |
| Number Of Questions | 77 |
| Academic Year | 2023-24 |
Let f be a function such that `f(xy)=(f(x))/y` for all positive real numbers x, y. If f(20) = 15, then f(50) =
View SolutionIf `f:A rarr B` is a function defined by `f(x)=(x^(2)-x)/(x^(2)+2x)`, then which one of the following is true?
View SolutionThe statement "`n^(5)-5n^(3)+4n` is divisible by 120" is true for
View SolutionLet `A=[{:(7,5), (4, 8):}], B=[{:(4, 3), (7, 5):}] " and "C=[{:(-5, 3), (7, -4):}]`
IF Tr(S) denotes the trace of a square matrix S then
`sum_(k=0)^(infty)1/(3^(k))Tr{A(BC)^(k)}=`
View SolutionIf the inverse of the matrix `A=[{:(3, 4, 5), (2, -1, 8), (5, -2, 7):}]` is B, then `B^(T)=`
View SolutionIf `x=alpha, y=beta, z=gamma` is the solution of the system of equations `x+y+z=4, 2x-y+3z=9, 3x+y+2z=8, " then "4alpha+2beta+3gamma=`
View Solution`(2/(i+sqrt(3)))^(100)+(2/(i-sqrt(3)))^(100)=`
View SolutionIf `a=3+4i, z_(1) " and "z_(2)` are two complex numbers such that `abs(z_(1))=3 " and "abs(z_(2)-a)=2`, then the maximum value of `abs(z_(1)-z_(2))` is
View SolutionIf `alpha` is the real root and `beta, gamma` are the complex roots of the equation `x^(3)+3x^(2)+3x+28=0, " then " 2alpha+3beta+3gamma=`
View SolutionGiven that `alpha, beta, gamma, delta` are in a geometric progression. If `alpha, beta` are the roots of `x^(2)-x+p=0 " and "gamma, delta` are the roots of `x^(2)-4x+q=0`, where p and q are integers, then the ordered pair (p, q) =
View SolutionIf A, B, C are the sets of all values of x, for which `x^(2)-5x-14` is positive, `-6x^(2)+2x-3` is negative and `4x-5x^(2)+2` is negative respectively, then `A cap B cap C=`
View SolutionThe complex solution set of the inequation `sqrt(x^(2)-3x+2) gt (3-x)` is
View SolutionSolve the equation `6x^4-35x^3+62x^2-35x+6=0` .
View SolutionI. The number of all ten digited numbers that can be formed with all the distinct digits and which ar divisble by 4 is `15 times 81`.
II. The number of positive integers that can be formed by using the digits 0, 1, 2, 3, 4, 5 without any repetition is 630.
View SolutionA man has 5 male and 4 female relatives. His wife has 4 male and 5 female relatives. The number of ways in which they can invite 5 male and 5 female relatives so that 5 of them are man's relatives and remaining 5 are his wife's relatives
View SolutionIf the coefficients of `r^(th), (r+1)^(th) " and "(r+2)^(th)` terms in the expansion of `(1+x)^(14)` are in an arithmetic progression, then r =
View SolutionIf `x=(5)/((2!).3)+(5.7)/((3!).3^(2))+(5.7.9)/((4!).3^(3))+….`
then find the value of `x^(2)+4x.`
View SolutionIf the periods of the functions sin(ax+b) and tan(cx+d) are respectively `4/7 " and "2/5`, then `sin(abs(a)+abs(c))+cos(abs(a)-abs(c))=`
View SolutionThe smallest positive value of x (in degress) for whiich `tan (x + 100^(@)) = tan (x + 50^(@)) .tan xtan (x-50^(@))` is
View SolutionFor `alpha ne 0, " if "cos(theta+alpha), costheta " and "cos(theta-alpha)` are in harmonic progression, then `sec^(2)theta*cos^(2)""alpha/2=`
View SolutionIf `cos2theta+alphasintheta=2alpha-7` has a solution, then
View SolutionIf x = a is a solution of the equation `sin^(-1)""x/3+sin^(-1)""(2x)/3=sin^(-1)x`, then the roots of the equation `x^(2)-ax-1=0` are
View SolutionThe set of all real values of x for which `f(x)=log_(e)sqrt((1+x)/(1-x))+log_(e)((1+sqrt(1-x^(2)))/x)+coth^(-1)x+log_(e)((1+sqrt(1+x^(2)))/x)+sinh^(-1)x` is defined is
View SolutionIf ` A = 60 ^(@) " then " ( 1)/(a+b) + (1) /( a+ c) = `
View SolutionIn a `DeltaABC, 8R^(3)sumsin^(3)Acos(B-C)=`
View SolutionIn a `DeltaABC, " if "a:b:c=4:5:6`, then the ratio of the radius of the circumcircle to the radius of the incircle is
View Solutiona, b, c are three mutually perpendicular unit vectors in the right handed system. If the points P, Q, R with position vectors `2a+5b-4c, a+4b-3c " and "ka+7b-6c` respectively lie on a line, then the ratio in which the point P divides QR is
View SolutionLet `pi` be the plane passing through the points `hat(i), hat(j), hat(i)+hat(j)+hat(k)` and L be the line passing through the point `hat(i)+2hat(j)+3hat(k)` and parallel to the vector `hat(i)-hat(j)+hat(k)`. If `P(alpha, beta, gamma)` is the point of intersection of the plane `pi` and line L, then `sqrt((alpha^(2)+beta^(2))gamma^(2))=`
View SolutionIf `a=hat(i)-hat(j)-hat(k), b=2hat(i)-3hat(j)+hat(k)" and "p_(1), p_(2)` are the orthogonal projection vectors of a on b and b on a respectively, then `(p_(1)+p_(2))*(p_(1)-p_(2))=`
View SolutionLet a, b, c be three non-coplanar vectors and `a^(')=(b times c)/([abc]), b^(')=(c times a)/([abc]), c^(')=(a times b)/([abc])`. The length of the altitude of the parallelopiped formed by a `a^('), b^('), c^(')` as coterminous edges, with respect to the base having `a^(') " and "c^(')` as its adjacent sides is
View SolutionLet a, b, c be three non-coplanar vectors. Let `S_(i)(i=1, 2, 3, 4, 5, 6)` denonte the six scalar triple products formed by all possible permutations of `a, b,c `. If i, j, k, l are randomly chosen distinct numbers from 1 to 6 and if `x=S_(i)/S_(j)+S_(k)/S_(l), y=S_(i)/S_(j)-S_(k)/S_(l)`, then `x^(2)+y^(2)=`
View SolutionIf `a=hat(i)-2hat(j)+hat(k), b=hat(i)+3hat(j)-2hat(k), c=2hat(i)+hat(j)-hat(k) " and "d=hat(i)+hat(j)+hat(k)`, then the volume (in cubic units) of the tetrahedron having `(a times b) times c, b, d` as its coterminuous edges is
View SolutionThe mean deviation of the data 3, 5, 11, 13, 17, 19, 23, 29 about its arithmetic mean is
View SolutionIf the weights of 10 persons (in kgs) are observed as : 45, 49, 55, 50, 41,44 , 60, 58, 53, 55, then the variance of their weights is
View SolutionIf two dice are rolled at a time, then the probability of getting an odd number on the first die or a total of 7 on both dice is
View SolutionIf A and B are two events of a random experiment such that `P(bar(A))=0.3, P(B)=0.4 " and "P(A cap bar(B))=0.5, " then "P(A cup B)+P(B| A cup bar(B))=`
View SolutionA speaks truth in 4 out of 5 times. A die is tossed. If A reports that there is 4 on the die, then the probability that there was 4 on the die, is
View SolutionLet S = {1, 2, 3,…., 50} and `A_(k)` be the set of multiples of k in S for `k in N`. IF `x_(k)` is a number chosen from `A_(k)`, then match the items of List-I with the items of List-II.
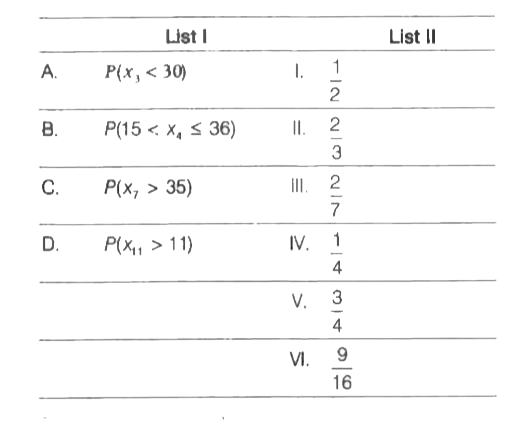
The correct match is
View SolutionIf X is a Poisson variate such that `P(X=2)=9P(X=4)`, then the mean and variance of X are
View SolutionLet P be the point (4, 1) and Q be its image in the line y = x. If Q is translated through a distance 2 units along the negative Y-axis to reach the point R, then the co-ordinates of R are
View SolutionThe normal form of the line x+y+1=0 is
View SolutionThe vetices of a triangle are O(0, 0), B(-3, -1), C(-1, -3). The equation of the line parallel to BC and intersecting the sides OB and OC whose perpendicular distance from O is `1//2` is
View SolutionA variable line passing through a fixed point `(alpha,beta)` intersects the coordinate axes at A and B. If O is the origin, then the locus of the centroid of the `DeltaOAB` is
View SolutionIf A is the orthocentre of the triangle formed by `2x^(2)-y^(2)=0, x+y-1=0` and B is the centroid of the triangle formed by `2x^(2)-5xy+2y^(2)=0, 7x-2y-12=0`, then the distance between A and B is
View SolutionThe distance between the pair of lines represented by
`x^(2)+2sqrt(2)xy+2y^(2)+4x+4sqrt(2)y+1=0`, is
View SolutionThe number of integers `alpha`, for which a chord of the circle `x^(2)+y^(2)=75` is bisected at `(8, alpha)` and that the slope of the chord is an integer, is
View SolutionIf the line `x-6y-12=0` meets the circle `S equiv x^(2)+y^(2)-4x+8y+6=0` at A and B, then the point of intersection of the tangents at A and B to S = 0 is
View SolutionA circle of radius 5 units passes through A(-5, 0) and B(5, 0). If `P(5cosalpha, 5(sinalpha), Q(5cosbeta, 5sinbeta)` are two points on this circle such that `alpha-beta=pi/2`, then the locus of the point of intersection of the line AP and BQ is
View SolutionThe condition that the circles which passes through the points `(0,a),(0,-a)` and touch the line `y= mx+c` will cut orthogonally is
View SolutionFor the system of circles given by `(x^(2)+y^(2)+2gx)+lambda(x^(2)+y^(2)+2fy+l)=0, " where " g ne 0, f ne 0 " and " lamda` is a parameter, if the line joining the points circles of the system subtends a right angle at the origin, then `k/f^(2)=`
View SolutionFor the parabola `y^(2)+2x+2y-3=0`, match the items in List-I with those from List-II.

The correct match is
View SolutionThe area (in sq units) of the triangle formed by the normal to the parabola `y^(2)=16x` whose slope is `1/2` with the co-ordinates axes is
View SolutionIf the major axis of an ellipse lies on the Y-axis, its minor axis lies on the X-axis and the length of its latusrectum is equal to `2/3` of its minor axis, then the eccentricity of that ellipse is
View SolutionIf `y=x+c` is a normal to the ellipse `x^(2)/25+y^(2)/9=1, " then "c^(2)=`
View SolutionThe area (in sq units) of the quadrilateral formed by the four common tangents drawn to the two hyperbolas `x^(2)/a^(2)-y^(2)/b^(2)=1 " and "y^(2)/a^(2)-x^(2)/b^(2)=1(a gt b)` is
View SolutionThe direction cosines of the line which is perpendicular to the lines with direction cosines proportional to (1, -2, -2) and (0, 2, 1) are
View SolutionThe number of lines passing through (0, 0, 0) and making an angle of `45^(@)` with each of the three co-ordinate axes is
View SolutionThe equation of the plane which passes through the point (2, 5, -8) and perpendicular to each of the planes 2x-3y+4z+1=0 and 4x+y-2z+6=0 is
View Solution`lim_(x to pi/6)(Sin(x-pi/6))/(sqrt(3)/2-cosx)=`
View SolutionThe integer 'n' is for which `Lt_(x to 0)((cosx-1)(cosx-e^(x)))/(x^(n))` is a finite non zero numberis
View SolutionIf `f(x)=int_(-1)^(x)abs(t)dt, x ge 1`, then
View SolutionIf `x=sinh^(-1)[log(1+sqrt(y)], " then "(dy)/(dx)=`
View SolutionIf `f(x)=((7x+1)sinx)/(e^(x)logx) " and "f^(')(x)=f(x)g(x)`, then `g^(')(x)=`
View SolutionIf `f(x)=|{:(cosx,1,0),(1,2cosx,1),(0,1,2cosx):}|" then "int_(0)^(pi//2)f(x)dx=`
View SolutionThe angle between the curves `y^(2)=8(x+4) " and "y^(2)=24(4-x)` is
View SolutionThe function `f(x)=x^(1//x) " for " x gt 0`, is
View SolutionLet `f:[0, 1/2] rarr R` be given by `f(x)=x(x-1)(x-2)`. The value 'c', when Lagrange's mean-value theorem is applied for f(x), is
View SolutionIf a tangent to the ellipse `x^(2)/a^(2)+y^(2)/b^(2)=1(a gt b gt 0)` having slope `1/3` is a normal to the circle `x^(2)+y^(2)+2x+2y+1=0`, then the maximum value of ab is
View Solution`int``(sin^(8)x-cos^(8)x)/(1-2sin^(2)x+2sin^(4)x)`dx`=`
View Solution`int(2x^(12)+5x^(9))/((x^(5)+x^(3)+1)^(3))dx=(x^(p))/(q(x^(5)+x^(3)+1)^(r))+c`, then `p-q-r`=
View Solution`int(x^(2)+cos^(2)x)/((1+x^(2))sin^(2)x)dx=`
View SolutionIf `I_(n)=intsin^(n)xdx, " for "n=1, 2, 3... " then "8I_(8)+7(I_(7)-I_(6))-6I_(5)=`
View Solution`lim_(n to infty) (1+32+243+…+n^(5))/n^(6)=`
View SolutionLet `a gt 1 " and "b gt 1`. If f(t) is a periodic function of period T and `int_(0)^(infty)a^(-bi)f(t)dt=kint_(0)^(T)a^(-bt)f(t)dt` then k =
View SolutionThe area (in sq units) enclosed by the curves `y=sinx+cosx " and "y=abs(cosx-sinx)` over the interval `[0, pi/2]` is
View SolutionThe degree and order respectively of the differential equation of the family of the curves respresented by `y=sqrt(c(x+sqrt(c)))` are (Here, C is a parameter)
View SolutionThe solution of the differential equation `(x+y-1)/(x+y-2)(dy)/(dx)=(x+y+1)/(x+y+2)`, given that y = 1 when x = 1, is
View Solution
MATHEMATICS questions and complete solutions for chapter AP EAMCET SOLVED PAPER 2019 (22-04-2019, SHIFT-2) of TS EAMCET PREVIOUS YEAR PAPERS of Class 12