Complete Solutions to MATHEMATICS of chapter TS EAMCET 2015 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | TS EAMCET 2015 |
| TS EAMCET | MATHEMATICS |
| Number Of Questions | 79 |
| Academic Year | 2023-24 |
An equation of a line whose segment between the coordinates axes is divided by the point `((1)/(2),(1)/(3))` in the ratio `2:3` is
View SolutionFind the value of `k` if the angle between the straight lines `4x-y+7=0, kx-5y-9-0` is `45^(@)`
View SolutionThe combined equation of the straight lines passing through the point (4,3) and each line making intercepts on the coordinate axes whose sum is - 1, is
View SolutionIf the coordinates of a pont P are transformed to `(sqrt(2), - sqrt(2))` when the axes are rotated through an angle `45^(@)`, then P
View SolutionThe locus of the point p which is equidstant from 3x+4y+5=0 and 9x+12y+7=0, is
View SolutionThe probability of a coin showing head is p and then 100 such coins are tossed. If the probability of 50 coins showing head is same as the probalility of 51 coins showing head then p equals
View SolutionX is a binomial variate with parameters `n=6` and p. If `4P(X=4)=P(X=2)`, then p is s
View SolutionIn a certain colleage, 4% of men and 1% of women are taller than 1.8m. Also, 60% of students are women. If a student selected at random is found to be taller than 1.8 m, then the probability that the student being a woman is
View SolutionIf A and B are two events such that `P(A//B)=0.6P(B//A)=0.3andP(A)=0.1"then"P(bar(A)capbar(B))` equals
View SolutionIf A and B are events such that `P(AcupB)=(5)/(6),Pbar((A))=(1)/(4)andP(B)=(1)/(3),"then"AandB` are
View SolutionTwo teams A and B have the same mean and their coefficients of variance are 4, 2 respectively. If `sigma_(A), sigma_(B)` are the standard deviations of teams A, B respectively then the relation between item is
View SolutionIn a data the number I is repeated I times for i=1, 2, …,n. Then the mean of the data is
View SolutionIf `a=2hat(i)-3hat(j)+5hat(j)," " b=3hat(i)-4hat(j)+5hat(k)andc=5hat(i)-3hat(j)-2hat(k)`, then the volume of the parallelpiped with contermiouns edges a+b, b+c, c+a, is
View SolutionThe shortest distance between the skew - lines
`(x-3)/(-1)=(y-4)/(2)=(z+2)/(1),(x-1)/1=(y+7)/(3)=(z+2)/(2)` is
View SolutionIf the position vectors of the vertices of `DeltaABC" ""are" " "3hat(i)+4hat(j)-hat(k),hat(i)+3hat(j)+hat(k)and5(hat(i)+hat(j)+hat(k))`, respectively. Then, the magnitude of the altitude from A onto the side BC is
View SolutionABCD is a parallelogram and P is a point on the segment `overset(-)(AD)` dividing it internally in the ratio 3:1. The line `overset(-)(BP)` meets the diagonal AC in Q. Then AQ: QC=
View SolutionIf M and N are the mid - points of the sides BC and CD respectively of a parallelogram ABCD, then AM + AN equals
View SolutionP is the point of intersection of the diagonals of the parallelogram ABCD, If S is any point in space and SA + SB + SC + SD = `lambdaSP`, then `lambda` equals
View SolutionIf `DeltaABC,ifr_(1)=2r_(2)=3r_(3) "then"b:c` equals
View Solution`(1)/(r^(2))+(1)/(r_(1)^(2))+(1)/(r_(2)^(2))+(1)/(r_(3)^(2))` equals
View SolutionThe angle of a triangle ABC are in an arithmetic progression . The larger sides a,b satisfy the relation ` (sqrt(3))/(2) lt (b)/(a) lt 1 .` then the possible values of the smallest side are
View SolutionIf cosh 2x = 199, then coth x equals
View SolutionIf `cos(cot^(-1)(1/2))=cot(cos^(-1)x)`, then a value of x is
View SolutionThe number of solutions of sec x cos 5x + 1 = 0 in the interval `[0,2pi]` is
View SolutionIf `DeltaABC, ifangleC=(pi)/(3), "then"(3)/(a+b+c)-(1)/(a+c)` equals
View SolutionIf `A=sin^(2) theta + cos^(4) theta ` , then for all values of ` theta ` , where
View SolutionIf f(x) is a real function defined on [-1,1], then the function g(x) = f(5x+4) is defined on the interval
View SolutionIf `f:NtoR` is defined by f(1) = - 1 and f(n+1) = 3f(n) + 2 for `nge1`, then f is
View SolutionThe remainder of `n^(4)-2n^(3)-n^(2)+2n-26` when divided by 24, is
View Solution`A(x)=|{:(1,2,3),(x+1,2x+1,3x+1),(x^(2)+1,2x^(2)+1,3x^(2)+1):}|rArrint_(0)^(1)A(x)dx=`
View SolutionLet `|{:(x^(2)+x+1,x+1,2x-3),(3x^(2)-1,x+2,x-1),(x^(2)+5x+1,2x+3,x+4):}|`
`=ax^(4)+bx^(3)+cx^(2)+dx+e` be an identity in x.
If a,b,c,d are known, then the value of e is
View SolutionThe system of equations 4x+y+2z=5, x-5y+3z=10, 9x-3y+7z=20 has
View SolutionIf `1,omega,omega^(2)` are the cube roots of unity and `alpha=omega+2omega^(2)-3, "then"alpha^(3)+12alpha^(2)+48alpha+3` equals
View SolutionIf `alpha, beta` are the roots of 1 + x + x^(2)= 0, then the value of `alpha^(4) + beta^(4) + alpha^(-4)beta^(-4)` is
View SolutionIf `alpha,beta` are the roots of the equation `x^(2) - 4x + 8 = 0`. Them for any `ninN,alpha^(2n)+beta^(2n)` equals
View SolutionIf `alpha,beta` are non - real cube roots of 2, then `alpha^(6) + beta^(6)` equals
View SolutionLet `alpha!=beta` satisfy `alpha^(2)+1=6alpha,beta^(2)+1=6beta.` Then, the quadratic equation whose roots are `(alpha)/(alpha+1),(beta)/(beta+1)` is
View SolutionThe set of solutions of ` |x| ^2-5|x|+4 lt 0` is
View SolutionLet `alpha,beta,gamma` be the roots of `x^(3)+x+10=0` and `alpha_(1)=(alpha+beta)/(gamma^(2)),beta_(1)=(beta+gamma)/(alpha^(2)),gamma_(1)=(gamma+alpha)/(beta^(2))`. Then, the value of `(alpha_(1)^(3)+beta_(1)^(3)+gamma_(1)^(3))-(1)/(10)(alpha_(1)^(2)+beta_(1)^(2)+gamma_(1)^(2))` is
View SolutionSuppose `alpha,beta.gamma` are the root of `x^(3)+x^(2)+x+2=0`. Then, the value of `((alpha+beta-2gamma)/(gamma))((beta+gamma-2alpha)/(alpha))((gamma+alpha-2beta)/(beta))` is
View Solution`overset(10)underset(r=0)sum^(40-r)C_(5)` is equal to
View SolutionIF a polygon has 35 diagonals , then the number of sides of the polygon is
View SolutionIf ``x=`1+(3)/(1!)xx(1)/(6)+(3xx7)/(2!)((1)/(6))^(2)+(3xx7xx11)/(3!)((1)/(6))^(3)+.... `"then"x^(4)` equals
View SolutionIf |x| is so small that `x^2` and higher powers of x may be neglected, then an approximately value of `((1+(2)/(3)x)^(-3) (1-15x)^(-1//5))/((2-3x)^4)` is
View SolutionThe coefficient of `x^(n)` in the expansion of `(1)/(x^(2)-5x+6)"for"|x|lt1` is
View SolutionIn a ` Delta ABC,` the value of `angle A ` is obtained from the equation `3 cos A + 2=0` . The quandratic equation , whose roots are `sin A` and `tan A` is
View SolutionMatch the differential equations in List I to their integrating factors in List II.
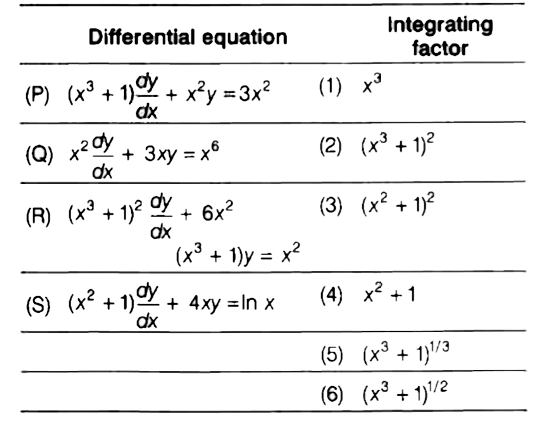
View SolutionThe solution of the differential equation `xy' = 2xe^(-y//x) + y` is
View SolutionThe differential equation of the family of curve `y = ax + (1)/(a)`, where `a ne 0` is an arbitrary constant, has the degree
View SolutionThe area of the region bounded by the curves `y=9x^(2)andy=5x^(2)+4` (in square units) is
View Solution`int_(0)^(pi//2) (16sin x. cos x)/(sin^(4) x + cos^(4)x)dx=`
View Solution`int_(0)^(1)sqrt((1-x)/(1+x))dx`
View Solution`int(x+5)/(x^(2)+4x+5)dx=alog(x^(2)+4x+5)+btan^(-1)(x+k)+C`, then (a,b,k) equals
View SolutionEvaluate `intsqrt(e^(x)-4)dx`
View SolutionIf `inte^(-x)tan^(-1)(e^(x))dx`
View SolutionIf `intsqrt((2+x)/(2-x))dx` is equal to
View SolutionTwo particles P and Q located at the points with coordinates `P(t,t^(3)-16t-3), Q(t+1,t^(3)-6t-6)` are moving in a plane. The minimum distance between them in their motion is
View SolutionIf `f(x)={{:(x,olexle1),(2-x,1lexle2'):}` then Rolle's theorem is not applicable to f(x) because
View SolutionAn equilateral triangle is of side 10 units. In measuring the side, if an error of 0.05 unit is made. Then, the percentage error in the area of the triangle is
View SolutionIf the line y = - 4x + b is tangent to the curve `y = (1)/(x)`, then b equals
View SolutionIf `x^(2) + y^(2) = t + (2)/(t) and x^(4) + y^(4) = t^(2) + (4)/(t^(2))`, then `x^(3)y(dy)/(dx) = `
View SolutionIf `y = tan^(-1)((3x-x^(3))/(1-3x^(2))) + tan^(-1) ((4x-4x^(3))/(1-6x^(2) + 4x^(4)))` then `(dy)/(dx) =`
View SolutionThe value of that should be assigned to f(0) so that the function f(x) `=(x+1)^(cotx)` is continuous at x = 0, is
View Solution`lim_(xrarr0)[tan(x+(pi)/(4))]^(1//x)` is equal to
View SolutionA plane meets the coordinate axes at P,Q,R respecticely. If the centroid of `DeltaPQR` is `(1,(1)/(2),(1)/(3))`, then the equation of the plane is
View SolutionIf the extremities of a diagonal of a square are (1,2,3) and (2,-3,5), then its side is of length
View SolutionA (4,3,5), B(0,-2,2) and C( 3,2,1) are three points. The coordinates of the point in which the bisector of `angleABC " meets the side " bar(BC)` is
View SolutionThe product of lenghts of perpendicular from any point on the hyperbola `x^(2) - y^(2) = 16` to its asymptotes, is
View SolutionThe centre of the ellipse `(x+y-3)^(2)/9+(x-y+1)^(2)/16=1` is
View SolutionFor the ellipse `(x^(2))/(25)+(y^(2))/(16) = 1`, a list of lines given in List I are to be matched with their equation given in List II.

View SolutionIf P is point on the parabola `y ^(2) =8x and A` is the point `(1,0),` then the locus of the mid point of the line segment AP is
View SolutionThe equation of the parabola with focus (1,-1) and directrix x + y + 3 = 0, is
View SolutionThe equation of the circle passing through (1,2) and the points of intersection of the circles `x^2+y^2-8x-6y+21=0` and `x^2+y^2-2x-15=0` is
View SolutionThe length of the common chord of the circles `(x-a)^2+y^2 = r^2` and `x^2 +(y-b)^2=r^2` is
View SolutionIf (4,2) and (k,-3) are conjugate points with respect to `x^(2)+y^(2)-5x+8y+6=0` them k=
View SolutionThe area (in sq units) of the triangle formed by the tangent, normal at `(1,sqrt(3))` to the circle `x^(2) + y^(2) =4` and the X-axis, is
View SolutionThe value of a, such that the power of the point (1, 6) with respect to the circle `x^(2)+y^(2)+4x-6y-a=0" is -16 is"`
View SolutionThe line x+y=k meets the pair of straight lines `x^(2)+y^(2)-2x-4y+2=0` in two points A and B. If O is the origin and `angleAOB=90^(@)` then the value of `kgt1` is
View SolutionIf two pairs of straight lines with combined equations xy + 4x - 3y - 12 = 0 and xy - 3x + 4y - 12 = 0 form a square. Then, the combined equation of its diagonals is
View Solution
MATHEMATICS questions and complete solutions for chapter TS EAMCET 2015 of TS EAMCET PREVIOUS YEAR PAPERS of Class 12