Complete Solutions to EXERCISE of chapter SET 8 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | SET 8 |
| UNITED BOOK HOUSE | EXERCISE |
| Number Of Questions | 29 |
| Academic Year | 2023-24 |
For any one empty set A the identity mapping on A will be
View SolutionIf `2 tan^(-1)x= sin^(-1)K` then the value of k is
View SolutionCHOOSE the correct answer from the following alternative :if two rows or two columns of a determinant are identical then value of the determinant is
View SolutionIf f(2a-x)=-f(x), then `overset(2a)underset(0)intf(x)dx `=
View SolutionIf `f(x) = x+ 1/x` , x>0 then the greatest value of f(x) is (a) -2 (b)0 (c) 3 (d) none of these
View SolutionIf `|veca|=4,|vecb|=2 sqrt3` and `|vecaxxvecb|=12` then the angle between the vectors `veca` and `vecb` is
View Solutionthe direction cosines of the line joining the points (1,2-3) and (-2,3,1) are
View SolutionIf the probability of occurrence of an event is `5/8` then the odds in fovour of the event are-
View SolutionA fair coin is tossed 99 times. Let X be the number of times head occurs. The P(X=r) maximum when r is
View SolutionEvaluate : `sec^2(tan^(-1)2)+cosec^2(cot^(-1)3)`.
View SolutionProve that , `|[1,log_x^y,log_x^z],[log_y^x,1,log_y^z],[log_z^x,log_z^y,1]|=0`
View SolutionIf `A = [[2,2],[4,3]]` show that A`A^(-1)`=`I_2`where `I_2` is the unit matrix of order 2.
View Solution`f(x) = { (kx+1, x le pi),( cos x, x >pi):}` If f(x) is continous at `x = pi` then find k.
View SolutionIf `ye^y=x` prove that `(dy)/(dx)= y/(x(1+y))`
View SolutionEvaluate: `int e^x/x(x log x+1)dx`
View SolutionFind the integrating factor of the differential equation `xlogx (dy)/(dx)+y= 2/(xlogx)`.
View SolutionFind the acute angle between the pair of straight lines whose direction cosines are `sqrt3/4,-1/4,-sqrt3/2` and `-sqrt3/4,1/4,-sqrt3/2`.
View SolutionFind the equation of the plane which passes through the points (1,2,3) ,(2,3,1) and (3,1,2).
View SolutionA random variable X has the following probability distribution:
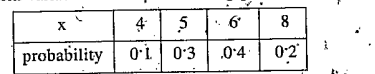
Find the expectation of the random variable X.
View SolutionIf `cos^(-1)x+cos^(-1)y= theta`, show that `x^2-2xycos theta+y^2 = sin^2 theta`.
View SolutionIf `A = [[cos alpha,sin alpha],[-sin alpha,cos alpha]]` then prove that, AA'=I. Hence find `A^(-1)`.
View SolutionIf `tan y = (tan x+sec x-1)/(tanx -sec x+1)` show that `(dy)/(dx) = 1/2`
View SolutionEvaluate: `int (dx)/(1-cos alpha cos x)(0< alpha < pi/2)`
View SolutionEvaluate: `int ((sinx+cos x)dx)/(sin(x-a))`
View SolutionThe vectors `vec a` and `vec b` are non collinear. If `vec p = (x+4y)veca+ (2x+y+1)vec b` and `vec q= (-2x+y+2)vec a+ (2x-3y-1)vec b` satisfy the relation `3vec p= 2vec q` find the values of x and y.
View Solutionevaluate `overset(1)underset(-1)int (2x+3)/(4)dx`
View SolutionShow that the standard deviation of a binomial distribution (with parameters n and p) cannot exceed `sqrt(n)//2`.
View SolutionProve that, a conical tent of given capacity will required the least amount of canvas, when the height is `sqrt2` times the radius of the base.
View SolutionShow that the normal at any point `theta` to the curve `x=acostheta+athetasintheta,\ y=asintheta-a\ thetacostheta` is at a constant distance from the origin.
View Solution
EXERCISE questions and complete solutions for chapter SET 8 of UNITED BOOK HOUSE of Class 12