Complete Solutions to PREVIOUS YEAR QUESTION of chapter PREVIOUS YEAR QUESTION 2020 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | PREVIOUS YEAR QUESTION 2020 |
| USHA PUBLICATION | PREVIOUS YEAR QUESTION |
| Number Of Questions | 46 |
| Academic Year | 2023-24 |
If `veca` and `vecb` are unit vectors and `veca-vecb` is also a unit vector, then write the measure of the angle between `veca` and `vecb`.
02:31View SolutionWrite the axis to which the plane by+cz+d=0 is parallal.
03:01View SolutionWrite down all the partitions of the set {a,b,c}.
01:19View SolutionWrite the domain of the function defined by f(x)=`sin^(-1)x+cosx`
02:00View SolutionA is a square matrix of order 3. write the value n, `|2A|=n|A|`.
02:06View SolutionA discrete random variable X has the probability distribution as given below:
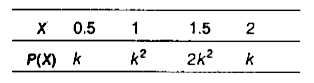
Then, find the value of k.03:25View SolutionFind the derivative of `tan^(-1)((cosx+sinx)/(cosx-sinx))` w.r.t. x.
03:27View SolutionIf f(x) = sin x + 2 in the interval `[-pi/2,pi/2]`, what can you say about the greatest value of f(x)?
04:16View SolutionIf `int_(-1/2)^(1/2) cosx In(1+x)/(1-x) dx = kIn^2` then write the value of k.
05:31View SolutionWrite the differential equation of all non-horizontal lines in a plane.
02:07View SolutionProve that for any `f:X rarr Y , f o id_x = f =id_Y` of.
03:15View SolutionSolve equation `3"tan"^(-1)1/((2+sqrt3)) - "tan"^(-1) 1/x ="tan"^(-1) 1/3`
06:40View SolutionProve that `tan{(pi)/(4)+(1)/(2) "cos"^(-1)(a)/(b)}+tan{(pi)/(4)-(1)/(2) cos^(-1)((a)/(b))}=(2b)/(a)`.
03:21View SolutionA man plans to start a poultry farm by investing at most ₹ 3000. He can buy old hens for ₹80 each and young ones for ₹ 140 each, but he cannot house more than 30 hens. Old hens lay 4 eggs per week ,each ell bing sold at ₹5. It costs ₹ 5 to feed an old hen and ₹8 to feed a young hen per week. Formulate his problem determining the number of hens of each type he should buy so as to earn a proft of more than ₹ 300 per week.
06:03View SolutionTest whether the relation : ` R = {(m, n):2|(m+n)}` on `ZZ` is reflexive, symmetric or transitive.
06:24View SolutionThere are two families A and B. There are `4` men, `6` women and `2` children in family A and `2` men, `2` women and `4` children in family B. The recommended daily amount of calories is `2400` for men, `1900` for women and `1800` for children, and `45` g of proteins for men, `55` g for women and `33` g for children. Represent the above information by matrices. Using matrices multiplication, calculate the total requirement of calories and proteins for each of the `2` families.
05:49View SolutionEliminate x,y,z from
a=x/y-z, b=y/z-x, c=z/x-y02:34View SolutionThere are 25 girls and 15 boys in class XI and 30 boys and 20 girls in class XII. If a student chosen from a class, selected at random, happens to be a boy, find the probability that he has been chosen from class XII.
04:05View SolutionFour cards are drawn successively with replacement from a well shuffled pack of 52 cards. Find the probability distribution of the number of aces. Calculate the mean and variance of the number of aces.
10:19View SolutionFind the inverse of the matrix `[{:(4,-2),(3,1):}]`
02:14View SolutionIf sin (x + y) = y cos (x + y) then prove that
`dy/dx =-(1 + y^2)/y^2`05:42View SolutionWhat is the derivative of ` sec^(-1)(1/(2x^2-1))`,with respect to`(sqrt(1-x^2))`?
06:01View SolutionFind the approximate value of `sqrt(0.24)`.
02:11View SolutionShow that the tangent to the curve `x = a (t - sin t), y = at (1 + cos t)` at
`t = pi/2` has slope.(1- pi/2)`04:11View SolutionExamine the contiunity of the following functions at the indicated points .`f(x)`=`{[2x+1 ,if xle0],[x, if 0 < x < 1],[2x-1,if xge1]:}`at `x=0,1.`
03:42View SolutionEvaluate the following integrals :
`int_(0)^(pi//2)log|(4+3sinx)/(4+3cosx)|dx`.04:52View SolutionThe area between `x=y^(2)` and `x=4` is divided into two equal parts by the line `x=a`. Find the value of a.
05:54View SolutionSolve the following differential equation:- `x(dy)/(dx) + y = y^2 lnx`
05:58View SolutionSolve : ` In((dy)/(dx)) = 3x+4y` given that y=0, when x=0.
05:36View SolutionEvaluate the following integrals `int(3sinx+28cosx)/(5sinx+6cosx)dx`
08:33View SolutionIf `veca = 3hati+hatj-2hatk, vecb = 2hati-3hatj+4hatk` then verify that `vecaxxvecb` is perpendicular to both `veca` and `vecb`.
06:19View SolutionPassing throughthe point `(2,-3,1)` and `(-1,1-7)` and perpendicular to the plane `x-2y+5z+1=0`.
04:27View SolutionFind the perpendicular distance of the point `(-1,3,9)` from the line `(x-13)/5=(y+8)/(-8)=(z-31)/1`
07:39View SolutionProve that the measure of the angle between two main diagonals of a cube is `cos^(-1)frac[1][3]`.
08:03View SolutionProve that the four points with position vectors `2veca+3vecb-vecc, veca-2vecb+3vecc, 3veca+4vecb-2vecc` and `veca-6vecb+6vecc` are coplanar.
05:48View SolutionIf A,B,C are matrices of order `2xx2` each and `2A+B+C =[[1,2],[3,0]]`
`A+B+C=[[0,1],[2,1]]`
`A+B-C =[[1,2],[1,0]]`find A,B and C.06:51View SolutionThe probability of a shooter hitting a target is `(3)/(4)` Find the minimum number of times he must fire, so that the probability of hitting the target atleast once is greater than `0.999`.
05:39View SolutionProve the following:
`[[(b+c)^2,a^2,bc],[(c+a)^2,b^2,ca],[(a+b)^2,c^2,ab]]`
=`(a^2+b^2+c^2)(a+b+c)(b-c)(c-a)(a-b)`10:05View SolutionShow that the semivertical angle of a cone of given slant height is `tan^1 sqrt2` when its volume is maximum.
08:27View SolutionIf `y=x^(sinx)+x^3 (sqrt(x^2+4))/(sqrt(x^3+3))` find `(dy)/(dx)`.
07:53View SolutionEvaluate : `int(x^5+x^4+x^3+x^2+4x+1)/(x^2+1) dx`
04:39View SolutionFind the solution of the following differential equations:
(4x+6y+5)dx-(2x+3y+4)dy=006:47View SolutionFind the area of the smaller region bounded by the ellipse `(x^(2))/(9)+(y^(2))/(4)=1` and the line `(x)/(3)+(y)/(2)=1`.
10:43View SolutionFind the shortest distance between the lines `(x-3)/3=(y-8)/(-1)=(z-3)/1` and `(x+3)/(-3)=(y-7)/2=(z-6)/4` Find also the equation of the line of shortest distance.
10:18View SolutionSolve the following LPP graphically Optimize `Z=5x_1+25x_2` subject to `-0.5x_1+x_2 le 2, x_1+x_2 ge 2, -x_1+5x_2 ge 5, x_1, x_2 ge 0`
10:35View SolutionIf `"sin"^(-1)x + "sin"^(-1)y +"sin"^(-1)z = pi "prove that" x^4+y^4+z^4 +4x^2y^2z^2=2(x^2y^2+y^2z^2+z^2x^2)`
08:14View Solution
PREVIOUS YEAR QUESTION questions and complete solutions for chapter PREVIOUS YEAR QUESTION 2020 of USHA MATHS (ODIA ENGLISH) of Class 12
