Complete Solutions to SECTION - C (Long Answer Type Questions) of chapter MODEL PAPER 2 of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | MODEL PAPER 2 |
| VGS PUBLICATION-BRILLIANT | SECTION - C (Long Answer Type Questions) |
| Number Of Questions | 7 |
| Academic Year | 2023-24 |
If ` alpha, beta` are the roots of the equation ` x^(2) - 2x + 4 = 0 ` then for any ` n in N` show that ` alpha^(n) + beta^(n) = 2^(n+1) cos ((n pi)/3)`.
09:45View SolutionSolve `18x^3+81x^2+121x+60=0` given that one roots is equal to half the sum of the remainging roots .
04:52View SolutionProve that : If the coefficients of `x^(10)` in the expansion of `(ax^(2)+(1)/("bx"))^(11)` is equal to the coeffi cient of `x^(-10)` in the expansion of `(ax-(1)/("bx"^(2)))^(11)`, find the relation between a and b where a and b are real numbers.
09:33View SolutionIf `x=(1.3)/(3.6)+(1.3.5)/(3.6.9)+(1.3.5.7)/(3.6.9.12)+....` then prove that `9x^(2)+24x=11`.
07:20View SolutionCalculate the variance and standard deviation for the following discrete frequency distribution:
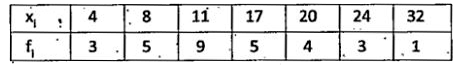 06:54View Solution
06:54View SolutionState and explain Boyle's law.
01:32View SolutionThe probability distribution of a random variable Xis given below:
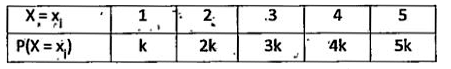
Find the value of k and the mean and variance of X.06:14View Solution
SECTION - C (Long Answer Type Questions) questions and complete solutions for chapter MODEL PAPER 2 of VGS PUBLICATION-BRILLIANT of Class 12
