Complete Solutions to TEXTUAL EXERCISES of chapter CURRENT ELECTRICITY of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | CURRENT ELECTRICITY |
| VIKRAM | TEXTUAL EXERCISES |
| Number Of Questions | 34 |
| Academic Year | 2023-24 |
The storage battery of a car has an emf of 12 V. If the internal resistance of the battery is `0.4 Omega`, what is the maximum current that can be drawn from the battery?
View SolutionA battery of emf 10 V internal resistance `3 Omega` is connected to a resistor R.
(i) If the current in the circuit is 0.5 A. calculate the value of R.
(ii) What is the terminal voltage of the battery when the cirucit is closed.
View SolutionThree resistors `1 Omega, 2 Omega` and `3 Omega` are combined in series. What is the total resistance of the comination?
View SolutionIf the combination is connected to a battery of emf 12 V and negligible internal resistance, obtain the potential drop across each resistor.
View SolutionThree resistors `2 Omega, 4 Omega` and `5 Omega` are combined in parallel. What is the total resistance of the combination?
View SolutionIf the combination is connected to a battery of emf 20 V and negligible internal resistance, determine the current through each resistor, and the total current drawn from the battery.
View SolutionAt room temperature `(27.0^(@)C)` the resistance of a heating element is `100 Omega`. What is the temperature of the element if the resistance is found to be `117 Omega`, given that the temperature coefficeint of the material of the resistor is `1.70 xx 10^(-4) .^(@)C^(-1)`
View SolutionA negligibly small current is passed through a wire of length 15 m and unifrom cross-section `6.0 xx 10^(-7) m^(2)` and its resistance is measured to be `5 Omega`. What is the resistivity of the material at the temperature of the experiment?
View SolutionA silver wire has a resistance of `2.1 Omega` at `27.5^(@)C`, and a resistance of `2.7 Omega` at `100^(@)C`. Determine the temperature coefficient of resistivity of silver.
View SolutionA heating element using nichrome connected to a 230V supply draws an initial current of 3.2 A which settles after a few seconds to a steady value of 2.8A. What is the steady temperture of the heating element if the heating the room temperature is `27.0^(@)C`? Temperature coefficient of resistance of nichrome averaged over the temperature range involved is `1.70 xx 10^(-4) .^(@)C^(-1)`.
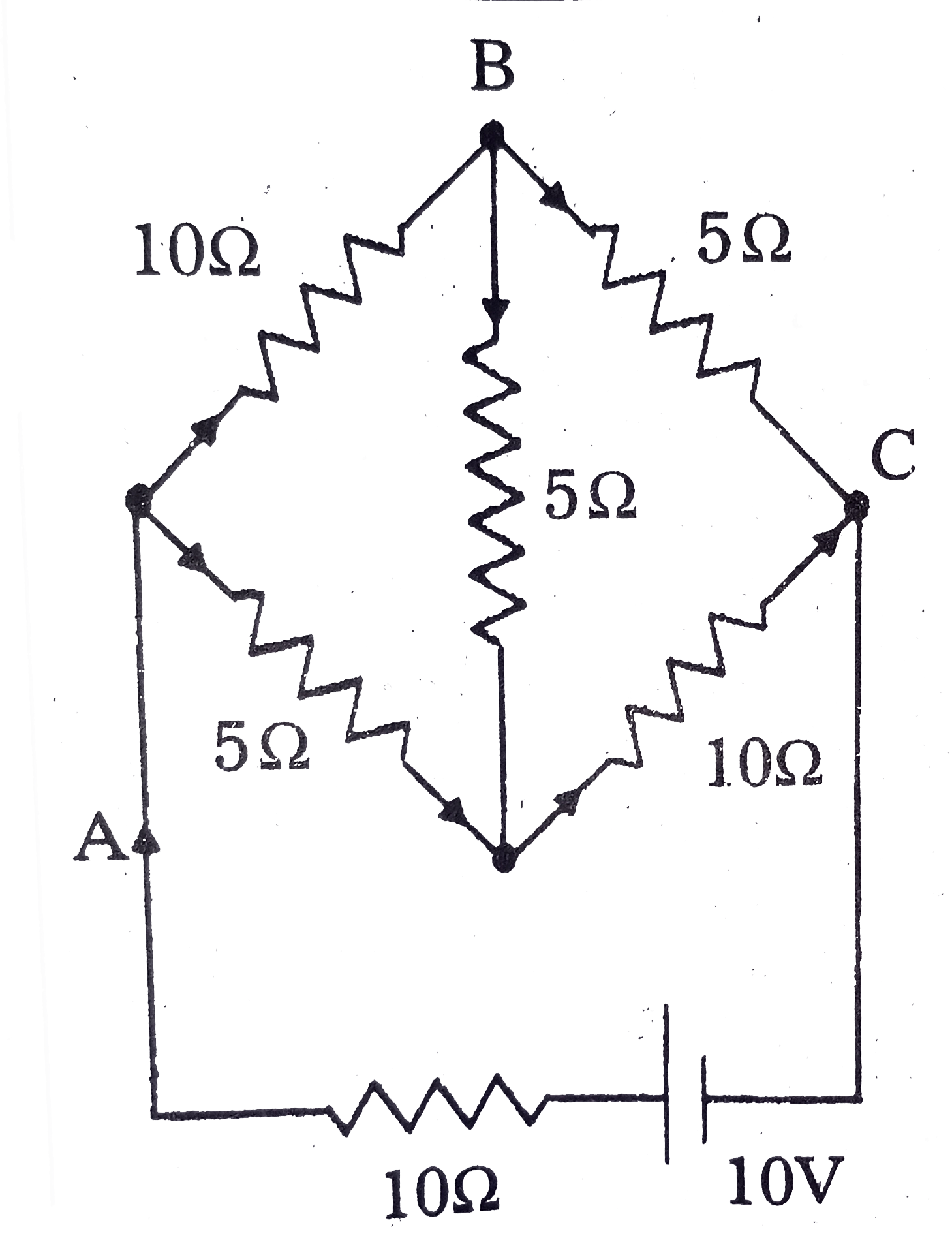
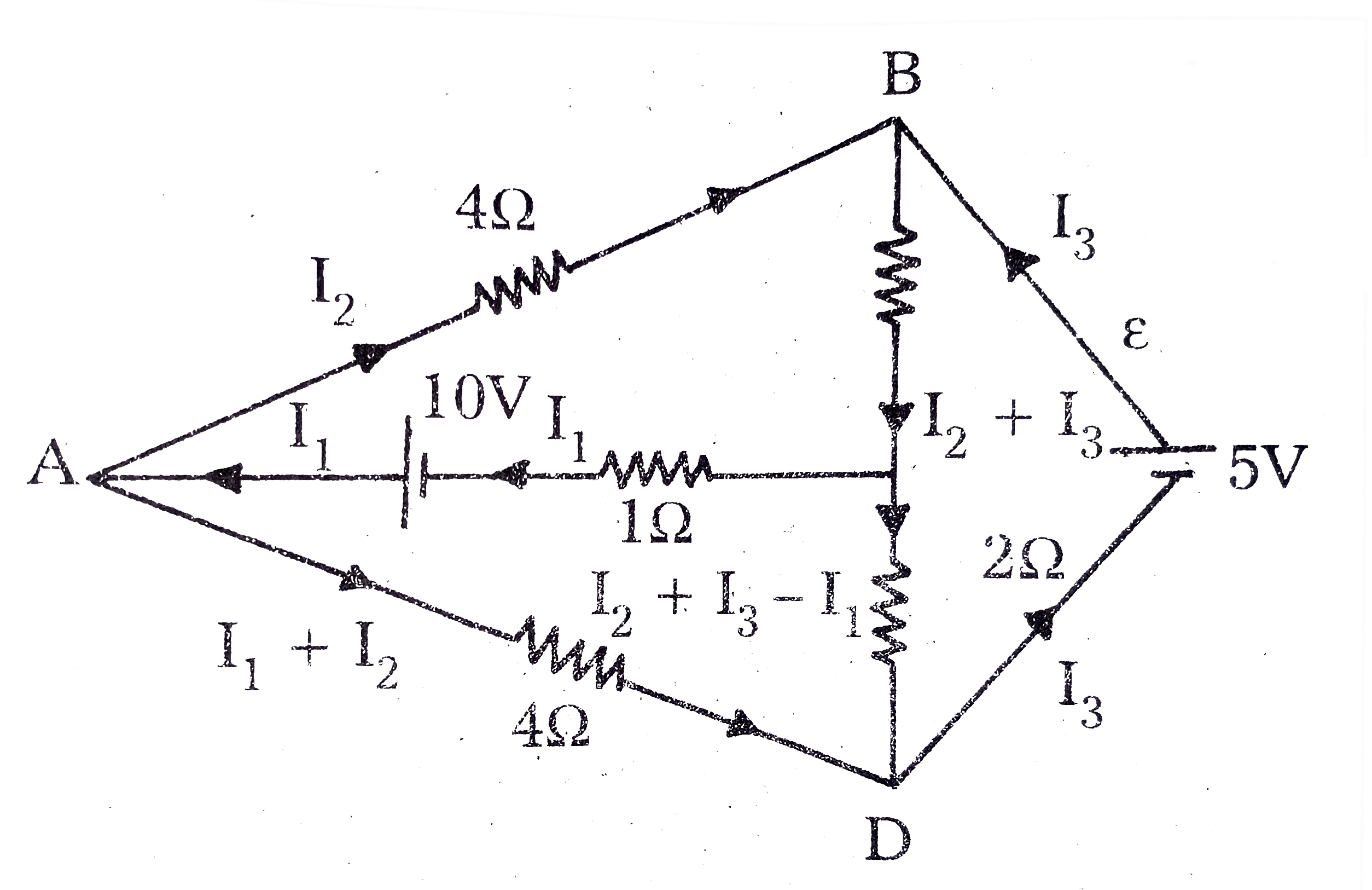
View SolutionDetermine the current in each branch of the network shown in fig.
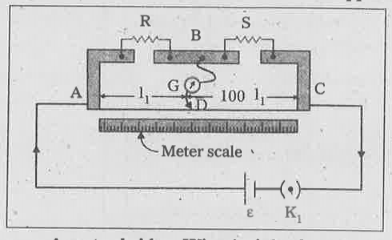
View SolutionIn a meter bridge the balance point is found to be at 39.5 cm from the end A, when the resistor Y is of `12.5 Omega`. Determine the resistance of x. why are the connections between resistors in a wheatstone or meter bridge made of thick copper strips?
View SolutionIn a meter bridge the balance point is found to be at `39.5` cm from the end A, when the resistor Y is of `12.5 Omega`. Determine the resistance of x. why are the connections between resistors in a wheat stone or meter bridge made of thick copper strips?
Determine the balance point of the bridge above if X and Y are interchanged.
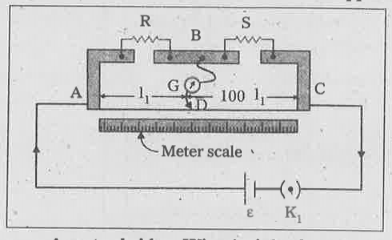
View SolutionIn a meter bridge the balance point is found to be at `39.5` cm from the end A, when the resistor Y is of `12.5 Omega`. Determine the resistance of x. why are the connections between resistors in a wheat stone or meter bridge made of thick copper strips?
What happens if the galvanometer and cell are interchanged at the balance point of the bridge ? Would the galvaometer show any current?
View SolutionA storage battery of emf 0.8 V and internal resistance `0.5 Omega` is being charged by a 120 V do supply using a series resistor of `15.5 Omega`. What is the terminal voltage of the battery during charging? What is the purpose of having a series resistor in the charging circuit?
View SolutionIn a potentiometer arrangment, a cell of emf 1.25 V gives a balance point at 35.0 cm length of the wire. If the cell is replaced by another cell and the balance point shifts to 63.0 cm`, what is the emf of the second cell ?
View SolutionThe number density of free electrons in a copper conductor estimated in textual example 6.1 is `8.5 xx 10^(28) m^(-3)`. How long does an electrons take to drift from one end of a wire 3.0 m long to its other end? The area of cross-section of the wire is `2.0 xx 10^(-6) m^(2)` and it is carrying a current of 3.0 A
View SolutionEstimate the average drift speed of conduction electrons in a copper wire of cross-sectional area `1.0 xx 10^(-7) m^(2)` carrying a current of 1.5 A. Assume that each copper atom contributes roughly one conduction electron. The density of copper is `9.0 xx 10^(3) kg//m^(3)` and its atomic mass is 63.5 u.
View SolutionCompare are drift speed obtained above with (i) thermal speeds of copper atoms at ordinary temperatures (ii) speeds of propagation of electric field along the conductor which causes the drift motion.
View SolutionIn Textual Example 1, the electron drift speed is estimated to be only a few `mm s^(-1)` for currents in the range of a few amperes? How then is current established almost the instant a circuit is closed?
View SolutionThe electron drift arises due to the force experienced by electrons in the electric field inside the condcutor. But force should cause acceleration. Why then do the electrons acquire a steady average drift speed?
View SolutionThe electron drift speed in metals is small `(~ ms^(-1))` and the charge of the electron is also very small `(~ 10^(-19)C)`, but we can still obtain a large amount of current in a metal. Why?
View Solutionwhen electrons drift in a metall from lower to higher potential does it mean that all the free electrons of the metal are moving in the same direction?
View SolutionAre the paths of electrons straight line between successive collisions (with the positive ions of the metal) in the (i) absence of electric field (ii) presnece of electric field?
View SolutionAn electric toaster uses nichrome for its heating element. When a negligibly small current passes through it, its resistance at room temperature `(27.0^(@)C)` is found to be `75.3 Omega`. When the toaster is connected to a 230 V supply, the current settles, after a few seconds, to a steady value of 2.68 A. what is the steady temperature of the nichrome element? The Temperature coefficient of resistance of nichrome averaged over the temperature range involved is `1.70 xx 10^(-4) .^(@)C^(-1)`.
View SolutionThe resistance of the platinum wire of a platinum resistance thermometer at the ice point is `5 Omega` and at steam point is `5.39 Omega`. When the thermometer is inserted in a hot bath, the resistance of the platinum wire is `5.795 Omega`. Calculate the temperature of the bath.
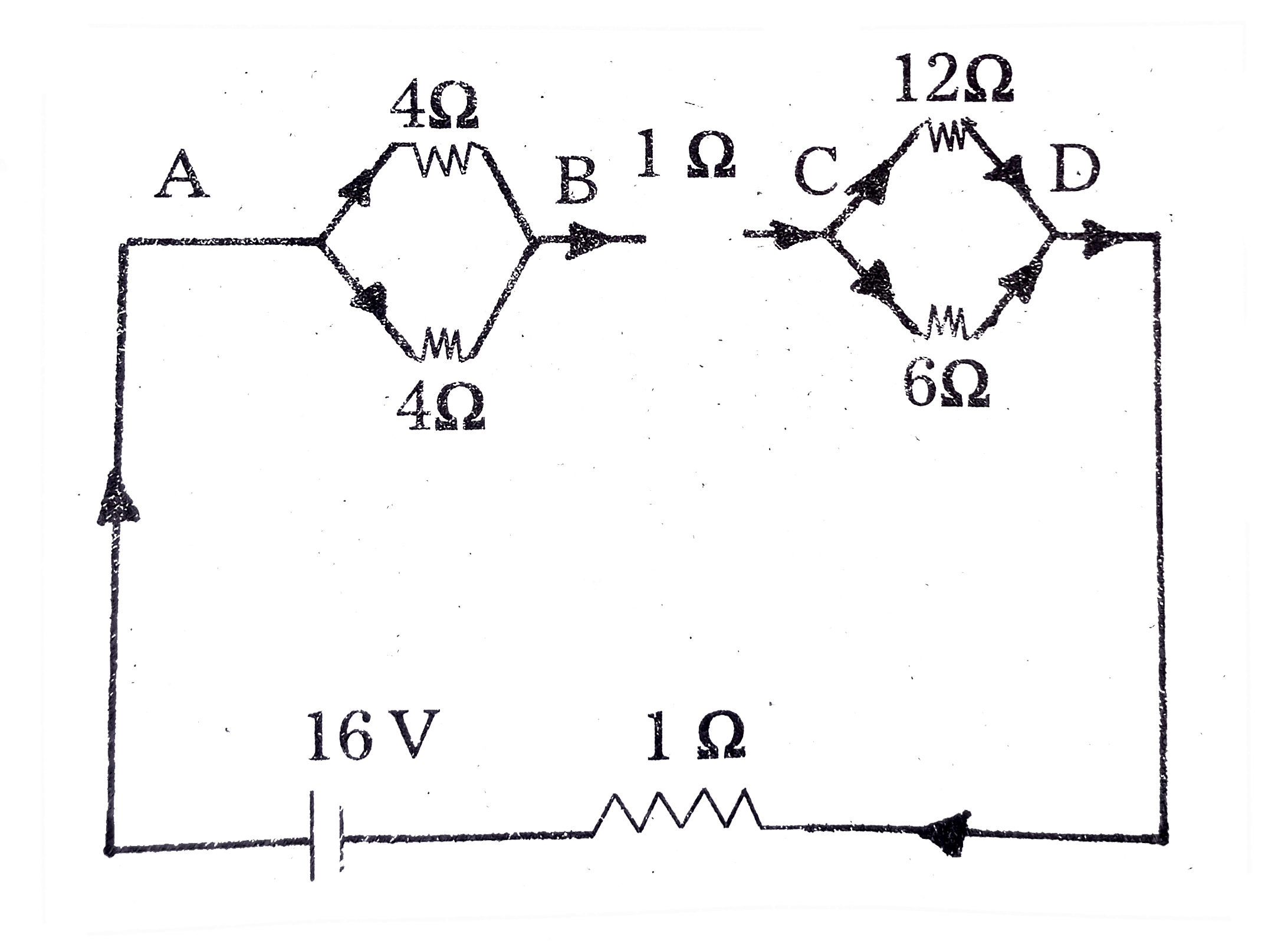
View SolutionA network of resistor is connected to a 16 V battery with internal resistance of `1 Omega`, a shown in fig.
(a) complete the equivalent resistance of the network.
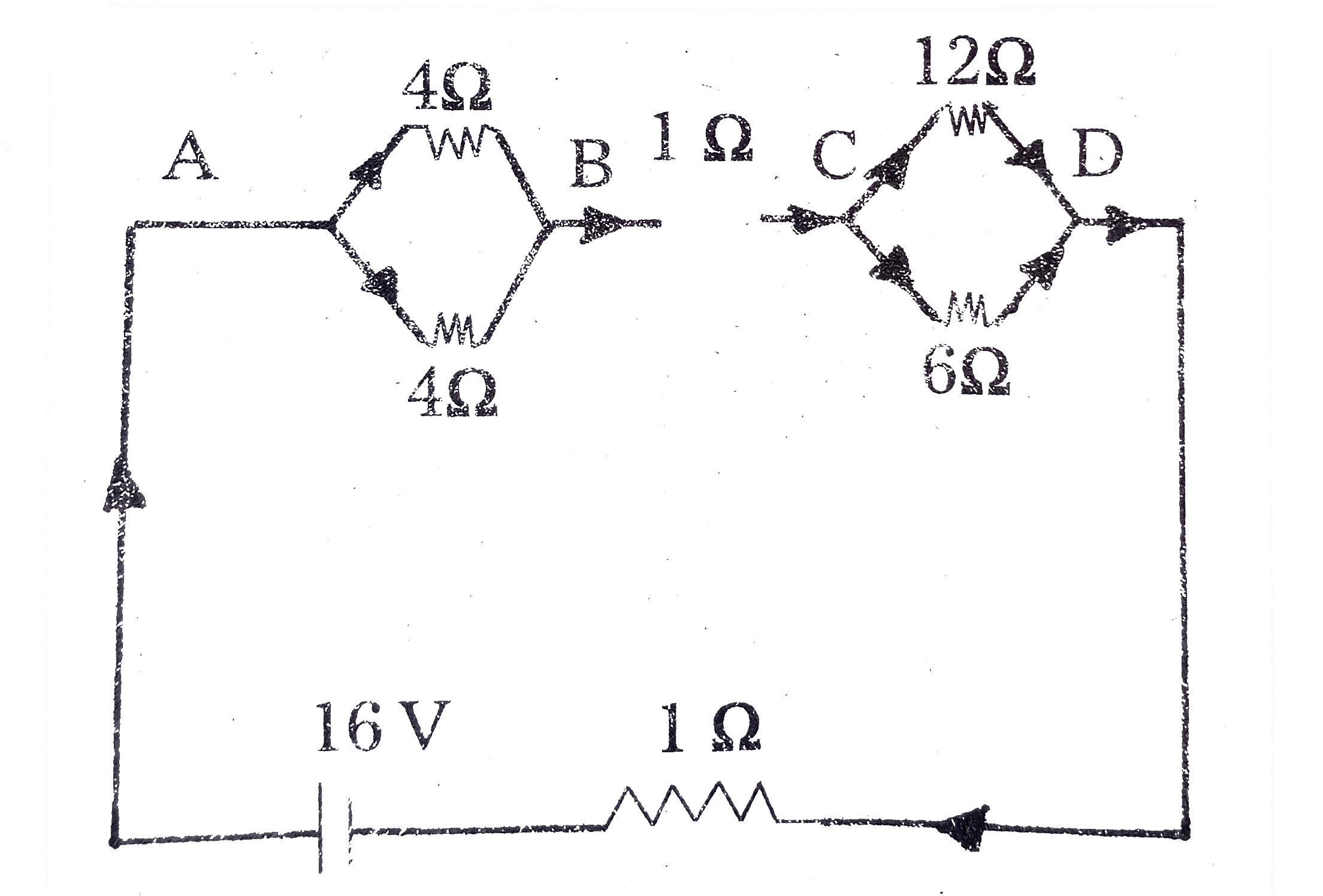
View SolutionA network of resistor is connected to a 16 V battery with internal resistance of `1 Omega`, a shown in fig.
Obtain the current in each resistor.
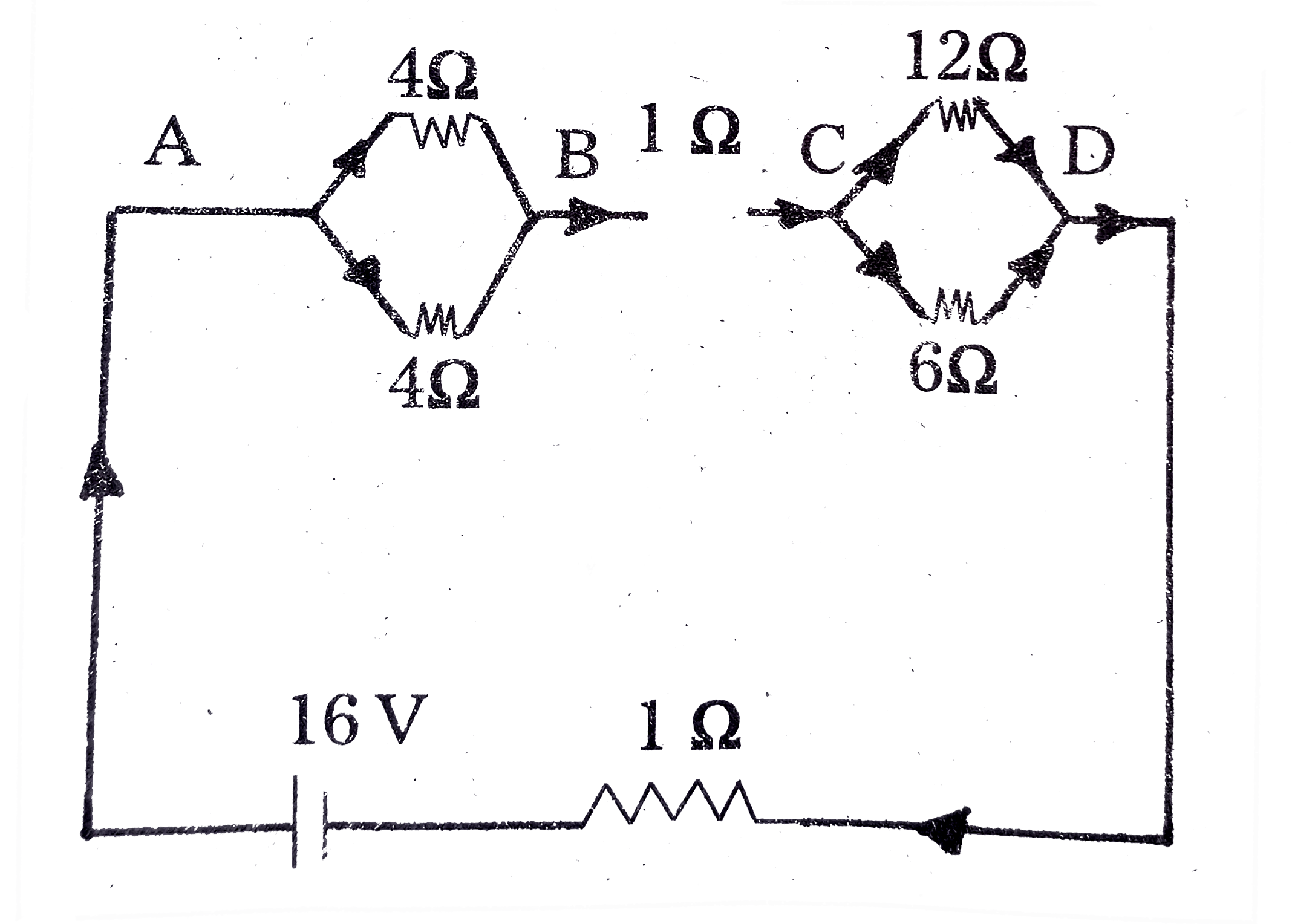
View SolutionA network of resistor is connected to a 16 V battery with internal resistance of `1 Omega`, a shown in fig.
Obtain the voltage drops `V_(AB), V_(BC)` and `V_(CD)`

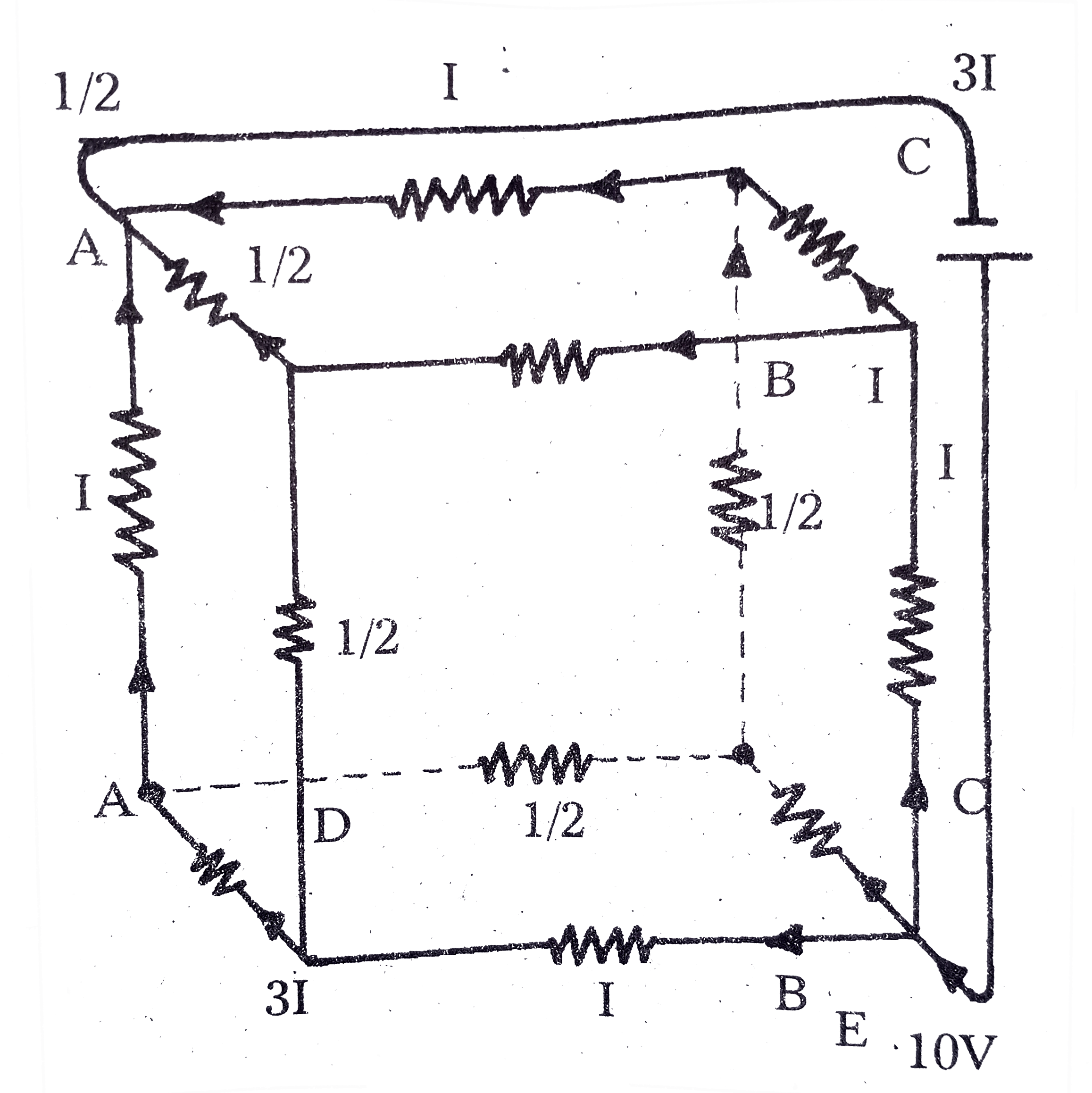
View SolutionA battery of 10 V and negligible internal resistance is connected across the diagonally opposite corners of a cubical network consisting of 12 resistors each of resistance `1 Omega` Fig. Determine the equivalent resistance of the network and the current along each edge of the cube.
View SolutionDetermine the current in each branch of the network shown in fig.
View SolutionThe four arms of a Wheatstone bridge have the following resistance :
`AB = 100 Omega, BC = 10 Omega, CD = 5 Omega`, and `DA = 60 Omega`
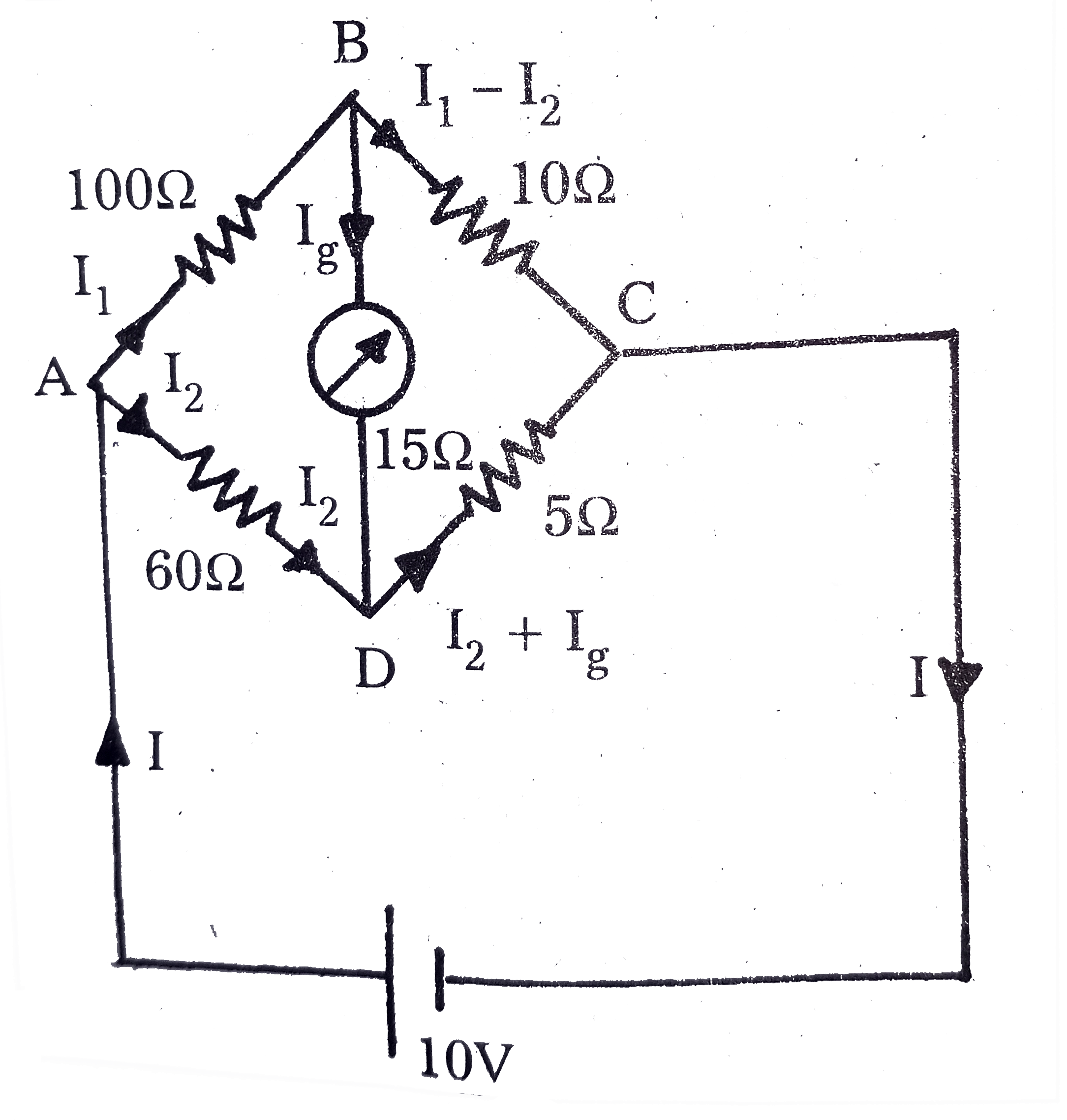
A galvanometer of `15 Omega` resistance is connected across BD. Calculate the current through the galvanometer when a potential difference of 10 V is maintained across AC.
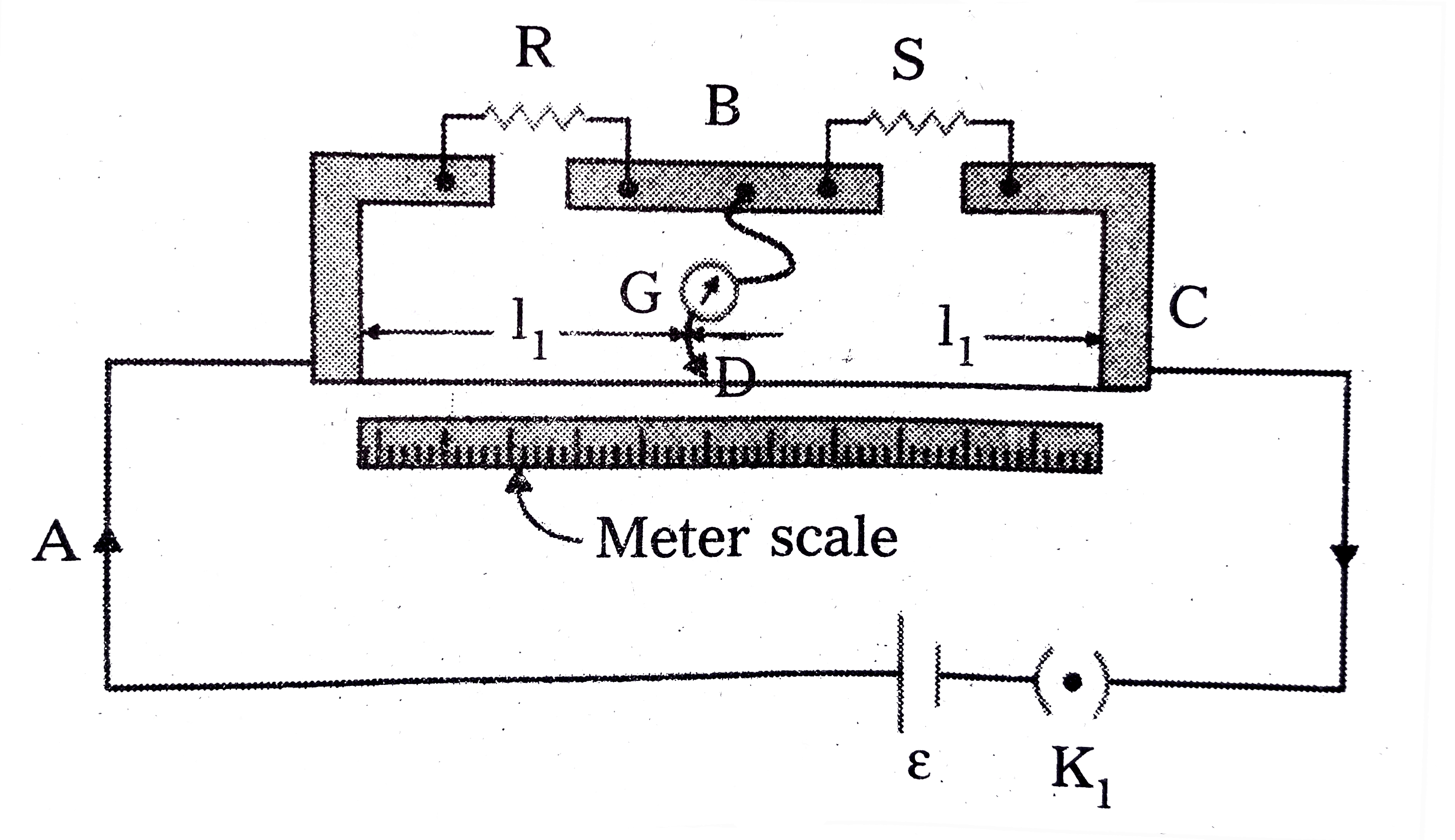
View SolutionIn a meter bridge , the null point is found at a distance of 36.7 cm from A. If now a resistance of `12 Omega` is connected in parallel with S, the null point occurs at 51.9 cm. Determine the value of R and S.
View SolutionA resistance of `R Omega` draws current from a potentiometer. The potentiometer has a total resistance `R_(0) Omega`. A voltage V is supplied to the potentiometer. Dervie can expression for the voltage across R when the sliding contact is in the middle of the potentiometer.

View Solution
TEXTUAL EXERCISES questions and complete solutions for chapter CURRENT ELECTRICITY of VIKRAM PUBLICATION ( ANDHRA PUBLICATION) of Class 12