Complete Solutions to SUBJECTIVE PROBLEMS of chapter CHEMICAL BONDING (BASIC) of Class 12 book with complete answers and questions
| Class 12 | CHEMISTRY |
| Chapter | CHEMICAL BONDING (BASIC) |
| VK JAISWAL | SUBJECTIVE PROBLEMS |
| Number Of Questions | 54 |
| Academic Year | 2023-24 |
Consider following compounds A to E :
(A) `XeF_(n) " " (B) XeF_((n+1))^(+) " " (C ) XeF_((n+1))^(-) " " (D) XeF_((n+2))`
(E ) `XeF_((n+4))^(2-)`,
If value of n is 4, then calculate value of `p div q` here, 'p' is total number of bond pair and 'q' is total number of lone pair on central atoms of compounds (A) to (E ).
View SolutionConsider the following five group (According to modern periodic table) of elements with their increasing order to atomic numbers :
Group `1 to A, B, C, D, E " " " Group" 2 to F,G,H,I,J`
Group `13 to K,L,M,N,O " " " Group" 15 to P,Q,R,S,T " " " Group " 17 to U,V,W,X,Y`
IF first and last element of each group belongs to 2nd and 6th period respectively and Z represents to carbonate ion `(CO_(3)^(2-))` then consider the following orders.
`(i) O^(+) gt H^(2+)` , Polarising power
(ii) `T^(3+) gt S^(3+) gt R^(3+)` , Stability of cation
`(iii) U^(-)(aq) gt V^(-)(aq) gt W^(-)(aq) gt X^(-)(aq)`, Size
(iv) `JV_(2) lt IV_(2) lt GV_(2) lt LV_(3)` , Covalent character
(v) `GZ gt IZ gt JZ` , Thermal stability
(vi) `AV gt BV gt CV gt DV gt EV` , Thermal stability
(vii) `C_(3)P gt B_(3)P gt A_(3)P` , Lattice energy
(viii) `KU_(3) lt KV_(3) lt KW_(3) lt KX_(3)`, Melting point
Then calculate value of `|p-q|^(2)` , here p and q are correct and incorrect orders in the given eight orders respectively.
View SolutionConsider the following species and find out total number of species which are polar and can act as Lewis acid
`C Cl_(4),CO_(2),SO_(2),AlCl_(3),HCHO,SO_(3),SiCl_(4),BCl_(3),CF_(4)`
View SolutionConsider the following table regarding interhalogen compounds, `XY_(n)` (where Y is more electronegative than X)

Thn according to given information calculate value of expression `P_(2)xx((P_(3)-P_(1))/((Q_(1)+Q_(2))`
View SolutionWhat is covalency of chlorine atom in second excited state ?
View SolutionSum of `sigma and pi` bonds in `NH_(4)^(+)` cation is ..
View SolutionCalculate the value ` X-Y` for `XeOF_(4)`. (`X=` Number of `sigma` bond pair and `Y=` Number of Ione pair on central atom).
View SolutionThe molecule `AB_n` is planar with six pairs of electrons around A in the valence shell. The value of n is
View SolutionCalculate value of `(X+Y+Z)/(10)`, here X is O-N-O bond angle in `NO_(3)^(-)` Y is O-N-O bond angle in `NO_(2)^(+)` and Z is F-Xe-F adjacent bond angle in `XeF_(4)`.
View SolutionCalculate x+y+z for `H_(3)PO_(3)` acid, where x is no. of lone pairs, y is no. of `sigma` bonds and z is no. of `pi` bonds.
View SolutionHow many right angle, bond angles are present in `TeF_(5)^(-)` molecular ion ?
View SolutionHow may possible `angle FSeF` bond angles are present in `SeF_(4)` molecule ?
View SolutionIn `IF_(6)^(-) and TeF_(5)^(-)`, sum of axial d-orbitals which are used in hybridisation in both species.
View SolutionAmong the following, total no. of planar species is :
(i) `SF_(4) " " (ii) BrF_(3) " " (iii) XeF_(2) " " (iv) IF_(5)`
(v)` SbF_(4)^(-) " " (vi) SF_(5)^(-) " " (vii) SeF_(3)^(+) " " (viii) CH_(3)^(+)`
(ix) `PCl_(4)^(+)`
View SolutionCalculate the value of " x+y-z" here x,y and z are total number of non-bonded electron pair (s),pie `(pi)` bond(s) and sigma `(sigma)` bonds in hydrogen phosphite ion respectively.
View SolutionConsider the following table
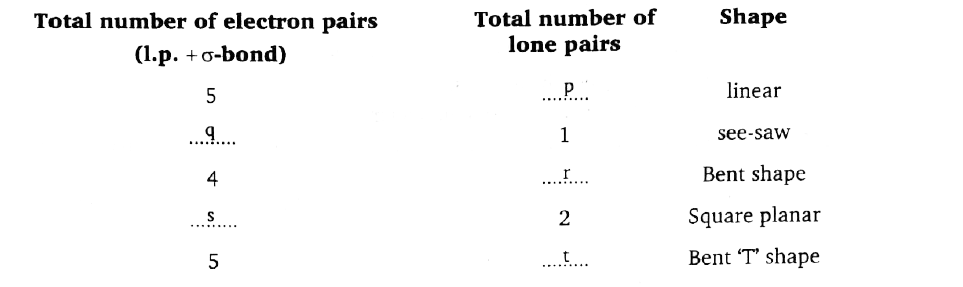
Then calculate value of "p+q+r-s-t".
View SolutionIn phosphorus acid, if X is number of non bonding electron pairs. Y is number of `sigma`-bonds and Z is number of `pi`- bonds. Then, calculate value of `Yxx Z -X`.
View SolutionCalculate the number of `p_(pi)-d_(pi)` bond(s) present in `SO_(4)^(2-)` :
View SolutionSum of `sigma and pi` bonds in `NH_(4)^(+)` cation is ..
View SolutionConsider the following orbitals (i)`3p_(x)` (ii)`4d_(x^(2))` (iii)`3d_(x^(2)-y^(2))` (iv)`3d_(yz)`
Then, calculate value of "`x+y-z`" here x is total number of gerade orbital and y is total number of ungerade orbitals and z is total number of axial orbitals in given above orbitals.
View SolutionCalculate value of |x-y|, here x and y are the total number of bonds in benzene and benzyne respectively which are formed by overlapping of hybridized orbitals.
View SolutionConsider the following compounds :
`(i) IF_(5) " " (ii) ClI_(4)^(-) " " (iii) XeO_(2)F_(2) " " (iv) NH_(2)^(-) `
`(v) BCl_(3) " " (vi) BeCl_(2) " " (vii) AsCl_(4)^(+) " " (viii) B(OH)_(3)`
(ix) `NO_(2)^(-) " " (x) ClO_(2)^(+)`
Then calculate value of "x+y-z", here, x,y and z are total number of compounds in given compounds in which central atom used their all three p-orbitals, only two p-orbitals and only one p-orbital in hybridisation respectively .
View SolutionTotal number of species which used all three p-orbitals in hybridisation of central atom and should be non-polar also.
`XeO_(2)F_(2), SnCl_(2), IF_(5), I_(3)^(+), XeO_(4),SO_(2),XeF_(7)^(+),SeF_(4)`
View SolutionConsider the following species `NO_(3)^(-), SO_(4)^(2-), ClO_(3)^(-),SO_(3), PO_(4)^(3-), XeO_(3),CO_(3)^(2-), SO_(3)^(2-)`
Then calculate value of |x-y|, where
x : Total number of species which have bond order 1.5 or greater than 1.5
y : Total number of species which have bond order less than 1.5
View SolutionConsider the following orbitals `3s, 2p_(x), 4d_(xy),4d_(z^(2)), 3d_(x^(2)-y^(2)),3p_(y),4s,4p_(z)` and find total number of orbital (s) having even number of nodal plane.
View SolutionFor the following molecules :
`PCl_(5), BrF_(3), IC l_(2)^(-), XeF_(5)^(-),NO_(3)^(-),XeO_(2)F_(2),PCl_(4)^(+),CH_(3)^(+)`
Calculate the value of `(a+b)/(c )`
a = Number of species having `sp^(3)` d-hybridisation
b= Number of species which are planar
c= Number of species which are non-planar
View SolutionFind out number of transformation among following which involves the change of hybridisation of underlined atom.
(a) `H_(2)underline(O)+H^(+) to H_(3)underline(O)^(+) " " (b) NH_(3)+underline(B)F_(3) to NH_(3). Underline(B)F_(3)`
(c ) `underline(X)eF_(6) to underline(X)eF_(5)^(+)+F^(-) " " (d) 2underline(P) Cl_(5) to underline(P)Cl_(4)^+)+PCl_(6)^(-)`
(e ) ` underline(C )H_(3)-CH_(3) to underline(C )H_(3)^(-)+CH_(3)^(+)`
View SolutionConsider following compounds A to E :
(A) `XeF_(n) " " (B) XeF_((n+1))^(+) " " (C ) XeF_((n+1))^(-) " " (D) XeF_((n+2))`
(E ) `XeF_((n+4))^(2-)`,
If value of n is 4, then calculate value of `p div q` here, 'p' is total number of bond pair and 'q' is total number of lone pair on central atoms of compounds (A) to (E ).
View SolutionConsider the following five group (According to modern periodic table) of elements with their increasing order to atomic numbers :
Group `1 to A, B, C, D, E " " " Group" 2 to F,G,H,I,J`
Group `13 to K,L,M,N,O " " " Group" 15 to P,Q,R,S,T " " " Group " 17 to U,V,W,X,Y`
IF first and last element of each group belongs to 2nd and 6th period respectively and Z represents to carbonate ion `(CO_(3)^(2-))` then consider the following orders.
`(i) O^(+) gt H^(2+)` , Polarising power
(ii) `T^(3+) gt S^(3+) gt R^(3+)` , Stability of cation
`(iii) U^(-)(aq) gt V^(-)(aq) gt W^(-)(aq) gt X^(-)(aq)`, Size
(iv) `JV_(2) lt IV_(2) lt GV_(2) lt LV_(3)` , Covalent character
(v) `GZ gt IZ gt JZ` , Thermal stability
(vi) `AV gt BV gt CV gt DV gt EV` , Thermal stability
(vii) `C_(3)P gt B_(3)P gt A_(3)P` , Lattice energy
(viii) `KU_(3) lt KV_(3) lt KW_(3) lt KX_(3)`, Melting point
Then calculate value of `|p-q|^(2)` , here p and q are correct and incorrect orders in the given eight orders respectively.
View SolutionConsider the following species and find out total number of species which are polar and can act as Lewis acid
`C Cl_(4),CO_(2),SO_(2),AlCl_(3),HCHO,SO_(3),SiCl_(4),BCl_(3),CF_(4)`
View SolutionConsider the following table regarding interhalogen compounds, `XY_(n)` (where Y is more electronegative than X)

Thn according to given information calculate value of expression `P_(2)xx((P_(3)-P_(1))/((Q_(1)+Q_(2))`
View SolutionWhat is covalency of chlorine atom in second excited state ?
View SolutionSum of `sigma and pi` bonds in `NH_(4)^(+)` cation is ..
View SolutionCalculate the value ` X-Y` for `XeOF_(4)`. (`X=` Number of `sigma` bond pair and `Y=` Number of Ione pair on central atom).
View SolutionThe molecule `ML_(x)` is planar with 6 electron pairs around M in the valence shell. The value of x is :
View SolutionCalculate value of `(X+Y+Z)/(10)`, here X is O-N-O bond angle in `NO_(3)^(-)` Y is O-N-O bond angle in `NO_(2)^(+)` and Z is F-Xe-F adjacent bond angle in `XeF_(4)`.
View SolutionCalculate x+y+z for `H_(3)PO_(3)` acid, where x is no. of lone pairs, y is no. of `sigma` bonds and z is no. of `pi` bonds.
View SolutionHow many right angle, bond angles are present in `TeF_(5)^(-)` molecular ion ?
View SolutionHow may possible `angle FSeF` bond angles are present in `SeF_(4)` molecule ?
View SolutionIn `IF_(6)^(-) and TeF_(5)^(-)`, sum of axial d-orbitals which are used in hybridisation in both species.
View SolutionAmong the following, total no. of planar species is :
(i) `SF_(4) " " (ii) BrF_(3) " " (iii) XeF_(2) " " (iv) IF_(5)`
(v)` SbF_(4)^(-) " " (vi) SF_(5)^(-) " " (vii) SeF_(3)^(+) " " (viii) CH_(3)^(+)`
(ix) `PCl_(4)^(+)`
View SolutionCalculate the value of " x+y-z" here x,y and z are total number of non-bonded electron pair (s),pie `(pi)` bond(s) and sigma `(sigma)` bonds in hydrogen phosphite ion respectively.
View SolutionConsider the following table

Then calculate value of "p+q+r-s-t".
View SolutionIn phosphorus acid, if X is number of non bonding electron pairs. Y is number of `sigma`-bonds and Z is number of `pi`- bonds. Then, calculate value of `Yxx Z -X`.
View SolutionCalculate the number of `p_(pi)-d_(pi)` bond(s) present in `SO_(4)^(2-)` :
View SolutionSum of `sigma and pi` bonds in `NH_(4)^(+)` cation is ..
View SolutionConsider the following orbitals (i)`3p_(x)` (ii)`4d_(x^(2))` (iii)`3d_(x^(2)-y^(2))` (iv)`3d_(yz)`
Then, calculate value of "`x+y-z`" here x is total number of gerade orbital and y is total number of ungerade orbitals and z is total number of axial orbitals in given above orbitals.
View SolutionCalculate value of |x-y|, here x and y are the total number of bonds in benzene and benzyne respectively which are formed by overlapping of hybridized orbitals.
View SolutionConsider the following compounds :
`(i) IF_(5) " " (ii) ClI_(4)^(-) " " (iii) XeO_(2)F_(2) " " (iv) NH_(2)^(-) `
`(v) BCl_(3) " " (vi) BeCl_(2) " " (vii) AsCl_(4)^(+) " " (viii) B(OH)_(3)`
(ix) `NO_(2)^(-) " " (x) ClO_(2)^(+)`
Then calculate value of "x+y-z", here, x,y and z are total number of compounds in given compounds in which central atom used their all three p-orbitals, only two p-orbitals and only one p-orbital in hybridisation respectively .
View SolutionTotal number of species which used all three p-orbitals in hybridisation of central atom and should be non-polar also.
`XeO_(2)F_(2), SnCl_(2), IF_(5), I_(3)^(+), XeO_(4),SO_(2),XeF_(7)^(+),SeF_(4)`
View SolutionConsider the following species `NO_(3)^(-), SO_(4)^(2-), ClO_(3)^(-),SO_(3), PO_(4)^(3-), XeO_(3),CO_(3)^(2-), SO_(3)^(2-)`
Then calculate value of |x-y|, where
x : Total number of species which have bond order 1.5 or greater than 1.5
y : Total number of species which have bond order less than 1.5
View SolutionConsider the following orbitals `3s, 2p_(x), 4d_(xy),4d_(z^(2)), 3d_(x^(2)-y^(2)),3p_(y),4s,4p_(z)` and find total number of orbital (s) having even number of nodal plane.
View SolutionFor the following molecules :
`PCl_(5), BrF_(3), IC l_(2)^(-), XeF_(5)^(-),NO_(3)^(-),XeO_(2)F_(2),PCl_(4)^(+),CH_(3)^(+)`
Calculate the value of `(a+b)/(c )`
a = Number of species having `sp^(3)` d-hybridisation
b= Number of species which are planar
c= Number of species which are non-planar
View SolutionFind out number of transformation among following which involves the change of hybridisation of underlined atom.
(a) `H_(2)underline(O)+H^(+) to H_(3)underline(O)^(+) " " (b) NH_(3)+underline(B)F_(3) to NH_(3). Underline(B)F_(3)`
(c ) `underline(X)eF_(6) to underline(X)eF_(5)^(+)+F^(-) " " (d) 2underline(P) Cl_(5) to underline(P)Cl_(4)^+)+PCl_(6)^(-)`
(e ) ` underline(C )H_(3)-CH_(3) to underline(C )H_(3)^(-)+CH_(3)^(+)`
View Solution
SUBJECTIVE PROBLEMS questions and complete solutions for chapter CHEMICAL BONDING (BASIC) of VK JAISWAL CHEMISTRY (HINGLISH) of Class 12
All Exercises from VK JAISWAL Class 12 CHEMISTRY CHEMICAL BONDING (BASIC)
- ASSERTION-REASON TYPE QUESTIONS
- Level 1
- Level 2
- Level 3 (Passive 1)
- Level 3 (Passive 10)
- Level 3 (Passive 11)
- Level 3 (Passive 12)
- Level 3 (Passive 2)
- Level 3 (Passive 3)
- Level 3 (Passive 4)
- Level 3 (Passive 5)
- Level 3 (Passive 6)
- Level 3 (Passive 7)
- Level 3 (Passive 8)
- Level 3 (Passive 9)
- MATCH THE COLUMN
- ONE OR MORE ANSWERS IS / ARE CORRECT
- SUBJECTIVE PROBLEMS