Complete Solutions to EXERCISE (MATCHING TYPE PROBLEMS) of chapter APPLICATION OF DERIVATIVES of Class 12 book with complete answers and questions
| Class 12 | MATHS |
| Chapter | APPLICATION OF DERIVATIVES |
| VK JAISWAL | EXERCISE (MATCHING TYPE PROBLEMS) |
| Number Of Questions | 8 |
| Academic Year | 2023-24 |
Column-1 gives pair of curves and column-II gives the angle `theta` between the curves at their intersection point.

View Solution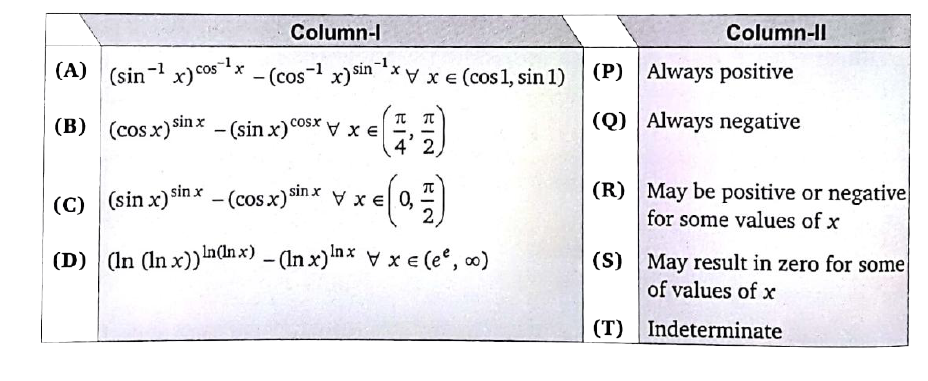
View SolutionLet `f (x) = (x ^(3) -4)/((x-1)^(3)) AA x ne 1, g (x)== (x ^(4) -2x ^(2))/(4) AA x in R, h (x) (x ^(3) +4)/((x+1)^(3)) AA x ne -1,`


View Solution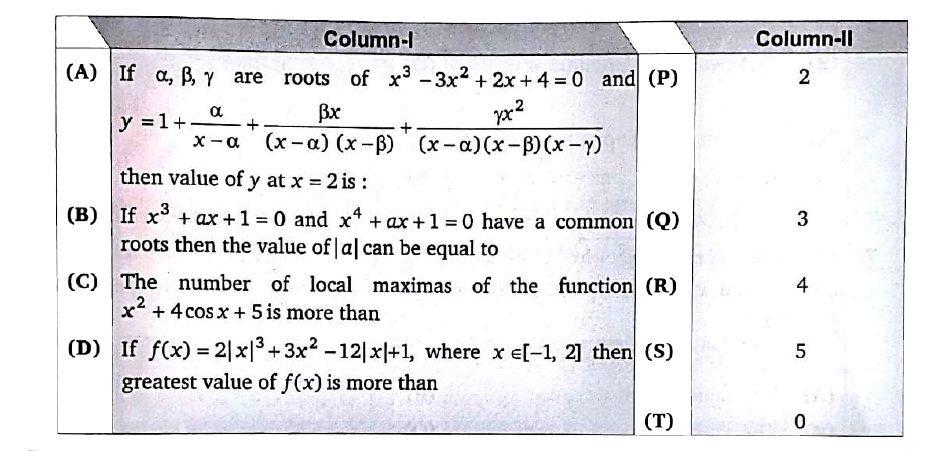
View Solution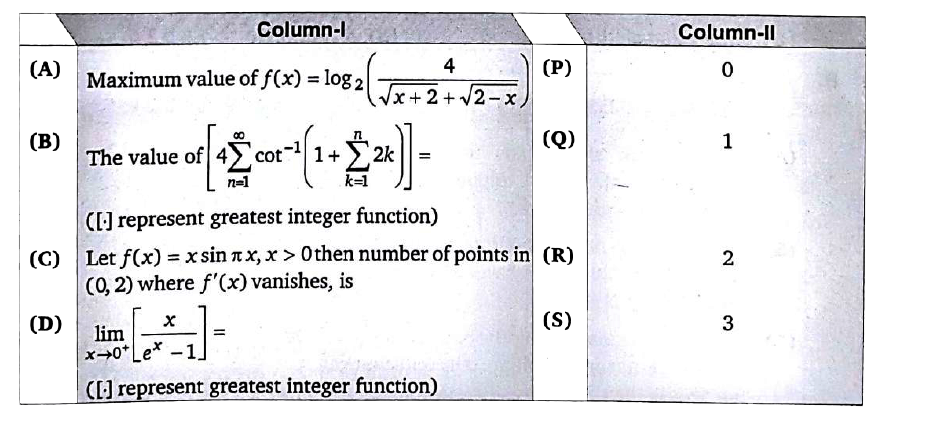
View SolutionConsider the functin `f (x) = (ln x)/(8) - ax +x ^(2) and a ge 0` is a real constant :

View SolutionThe function `f(x)=sqrt((a x^3+b x^2+c x+a))` ha sits non-zero local minimum and local maximum values at `x-2 and x = 2`, respectively. It 'a is a root of `x^2-x-6=0`
View Solution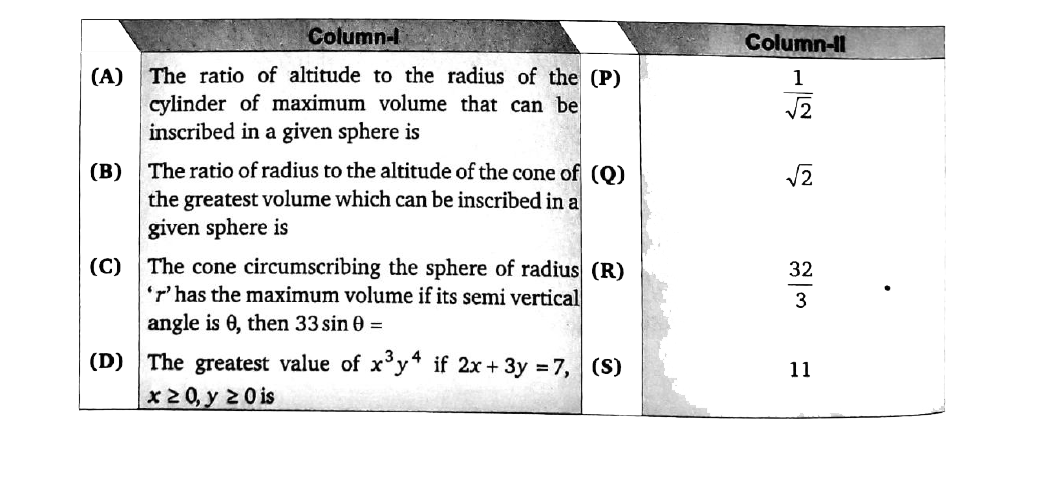
View Solution
EXERCISE (MATCHING TYPE PROBLEMS) questions and complete solutions for chapter APPLICATION OF DERIVATIVES of VK JAISWAL MATHS (HINGLISH) of Class 12