Complete Solutions to Examples of chapter PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE of Class 9 book with complete answers and questions
| Class 9 | PHYSICS |
| Chapter | PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE |
| SELINA PUBLICATION | Examples |
| Number Of Questions | 16 |
| Academic Year | 2023-24 |
A boy weighing 60 kgf is wearing shoes with heel of area of cross section `20 cm^(2)`, while a girl weighing 45 kgf is wearing sandals with heel of area of cross section `1.5 cm^(2)`. Compare the pressure exerted on ground by their heels when they stand on the heel of one foot.
View SolutionCalculate the pressure due to a water column of height 100 m. (Take `g=10 ms^(-2)` and density of water `=10^(3)kg m^(-3))`.
View SolutionAt what depth below the surface of water will pressure be equal to twice the atmospheric pressure ? The atmospheric pressure is `10N cm^(-2)` , density of water is `10^(3)kg m^(-3)` and `g=9.8 ms^(-2)`.
View SolutionA cube of each side 5 cm is placed inside a liquid. The pressure at the centre of one face of cube is 10 Pa. Calculate the thrust exerted by the liquid on this face.
View SolutionA square plate of side 10 m is placed horizontally 1 m below the surface of water. The atmospheric pressure is `1.013xx10^(5)Nm^(-2)`. Calculate the total thrust on the plate.
(Density of water `rho=10^(3)kg m^(-3), g=9.8ms^(-2))`
View SolutionA vessel of base area `100cm xx 60cm` and height `200 cm` is completely filled with a liquid of density `1.1xx10^(3)kg m^(-3)`.
(a) Ignoring the atmospheric pressure, find :
(i) the thrust at the bottom of the vessel,
(ii) the pressure at the bottom of the vessel,
(iii) The pressure at a depth of 5 cm from the free surface,
(iv) the net force experienced by a metal foil of area `10 cm^(2)` placed at a depth of 5 cm from the free surface,
(b) The thrust at the bottom of the vessel if the atmospheric pressure equal to `1xx10^(5)Nm^(-2)` is taken into account.
Take `g=9.8ms^(-2)` .
View SolutionIn Fig. `4.10`, a tube of length 200 cm filled with a liquid of density `0.90xx10^(3) kg m^(-3)` is placed inclined with the vertical such that the level A of liquid in the tube is at a vertical height 100 cm from its lowest point C. there is a point B in the tube below the point A at a vertical depth 60cm.
(a) Calculate the pressure at points
(i) A,
(ii) B and
(iii) C
(b) What will be the pressure at point C when the tube is made vertical ?
Take atmospheric pressure `=1.013xx10^(5)Nm^(-2)`
View SolutionA U tube is first partially filled with mercury. Then water is added in one arm and an oil is added in the other arm. Find the ratio of water and oil columns so that mercury level is same in both the arms of U tube. Given : Density of water `=10^(3)kg m^(-3)` , density of oil `=900kg m^(-3)`.
View SolutionFig. 4.11 shows a cube of each side 15 cm immersed in a tube containing water of density `10^(3) kg m^(-3)` such that its top surface is 20 cm below the free surface of water.
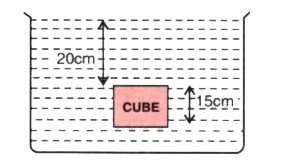
Calculate (i) the pressure at the top of cube,
(ii) the pressure at the bottom of cube,
(iii) the resultant pressure on cube.
(iv) the resultant thrust on cube.
Take atmospheric pressure = `10^(5)` Pa and `g=9.8 N kg^(-1)`.
View SolutionAn air bubble rises from the bottom of a lake of depth `10.34m` to its surface. Compare the pressure on bubble at the bottom to that on surface. (Atmospheric pressure `=0.76 m of Hg`, density of `Hg=13.6xx10^(3)Kg m^(-3)` and density of water `=10^(3)kg m^(-3))`.
View SolutionIn a hydraulic machine, the two pistons are of area of cross section in the ratio 1:10. What weight force in needed on the narrow piston to overcome a force of 100 N on the wide piston?
View SolutionThe area of cross section of press plunger of a hydraulic press is `4 m^2`. It is required to overcome a resistiveload of 400 kg on it. Calculate the force required on the pump plunger if the area of cross section of the pump plunger is `0.01 m^2`.
View SolutionWhat is meant by the statement “the atmospheric pressure at a place is 76 cm of Hg"? State its value in Pa.
View SolutionThe upper blood pressure of a patient is 160 cm of Hg whereas the normal blood pressure should be 120 cm of Hg. Calculate the extra pressure generated by the heart in S.I. unit . Take density of `Hg=13600 kg m^(-3)` and `g=9.8 ms^(-2)`.
View Solution(a) What length of water column is equivalent to `0.76` m of mercury column ? State the assumption made in your calculation.
(b) Can water be used as barometric liquid ? Give a reason.
View SolutionA mercury barometer reads 75cm. Now `3 cm^(3)` of atmospheric air is introduced into the tube. The mercury falls to a height of 65 cm and the length of air column above the mercury is found to be 15 cm Calculate the cross-sectional area of the barometer tube.
View Solution
Examples questions and complete solutions for chapter PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSURE of ICSE of Class 9