Complete Solutions to EXERCISE of chapter INTRODUCTION TO EUCLID'S GEOMETRY of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | INTRODUCTION TO EUCLID'S GEOMETRY |
| MODERN PUBLICATION | EXERCISE |
| Number Of Questions | 86 |
| Academic Year | 2023-24 |
Explain the difference between an axiom and a theorem.
View SolutionDefine the following
Ray?
View SolutionDefine the following
Line segment?
View SolutionDefine the following
Half-line?
View SolutionDefine the following
Parallel lines.
View SolutionDefine the following
Intersecting lines.
View SolutionDefine the following
Concurrent lines.
View SolutionDefine the following
Collinear points
View SolutionDefine the following terms
Planet
View SolutionHow many lines can pass through a given point?
View SolutionHow many circles can be drawn to pass through two given points?
View SolutionHow many points can be two distinct lines intersect at the most?
View SolutionIn the fig. AE=DF where E and F are mid-points of AB and DC respectively.Using Euclid's axiom, prove that AB=DC
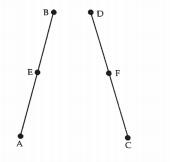
View SolutionIn the fig. name
six points, four line segments, four rays, three collinear points.
View SolutionHow many lines can pass through a given point?
View SolutionHow many lines can pass through
two given points?
View SolutionHow many points can be two distinct lines intersect at the most?
View SolutionHow many points can be two distinct lines intersect at the most?
View SolutionHow many planes can be made to pass through three distinct points?
View SolutionShow that every line segment has one and only one middle point.
View SolutionDefine parallel lines.
View SolutionDefine concurrent forces and describe equilibrium of concurrent forces.
View SolutionIf `l,m and n` are three straight lines such that `l||m` and `l||n` then prove that `m||n`.
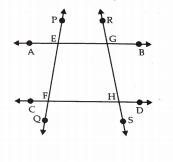
View SolutionIn the fig. if PR=QS prove that PQ=RS
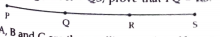
View SolutionA,B and C are three collinear points. How many line segment can be determined by them? Name these lines segments?
View SolutionEuclidean geometry is valid only for curved surfaces.
View SolutionWrite True or False and Justify Your answer The edges of a surface are curves.
View SolutionThe statements that are proved are called axioms.
View SolutionTwo distinct intersecting lines cannot be parallel to the same line.
View SolutionIf a quantity B is a part of another quantity A, then A can be written as the sum of B and some third quantity C.
View SolutionFill ups
A line segment has…………end points.
View SolutionFill ups
Rectilinear figures are formed by……………. .
View SolutionFill ups
Two lines in a plane not having any common points are called……………lines.
View SolutionFill ups
Concurrent lines……..passing through a given point.
View SolutionTwo distinct points in a plane determine a ............ Line.
View SolutionWho out of the following presented geometry in most systematic form?
View SolutionWhich out of the follwong mathematician gave first known proof?
View SolutionAxioms are
View SolutionPostulates are
a. self evident truths which are generaly sepcific
b. self evident truths which are applicable to mathematics in general
c. proofs
d. theorem.
View SolutionWhich of the following order is as per ascending order of dimensions.
View SolutionWhich of the follwong solid figure does not exist?
View SolutionEuclid's definitions of surface is that which has
View SolutionTerminated lines according to Eculid's postulates be produced
View SolutionPlayfair's axiom states that
View SolutionThales belongs to the country :
View SolutionEuclid belongs to the country :
View SolutionPythagoras was a student of :
View SolutionIn Indus Valley Civilisation (about 300 B.C.), the bricks used for construction work were having dimensions in the ratio :
View SolutionEuclid stated that all right angles are equal to each other in the form of :
View SolutionGreek’s emphasised on :
View SolutionThe three steps from solids to points are :
View SolutionThe number of dimensions, a solid has :
View SolutionThe number of dimensions, a surface has :
View SolutionThe number of dimensions, a point has :
View SolutionEuclid divided his famous treatise “The Elements” into :
View SolutionThe total number of propositions in the Elements are :
View SolutionBoundaries of solids are :
View SolutionBoundaries of surfaces are :
View SolutionIn Indus Valley Civilisation (about 300 B.C.), the bricks used for construction work were having dimensions in the ratio :
View SolutionA pyramid is solid figure, the base of which is :
View SolutionThe side faces of a pyramid are :
View SolutionIt is known that if x + y = 10 then x + y + z = 10 + z. The Euclid’s axiom that illustrates this statement is :
View SolutionIn ancient India, the shapes of altrars used for house hold rituals were :
View SolutionThe number of interwoven isosceles triangles in Sriyantra (in the Atharvaveda) is :
View SolutionGreek’s emphasised on :
View SolutionIn Ancient India, Altars with combination of shapes like rectangles, triangles and trapeziums were used for :
View SolutionEuclid belongs to the country :
View SolutionThales belongs to the country :
View SolutionPythagoras was a student of :
View SolutionWhich of the following needs a proof ?
View SolutionEuclid stated that all right angles are equal to each other in the form of :
View SolutionLines are parallel if they do not intersect is stated in the form of :
View SolutionIf the lines AB and AC are parallel to a line l, show that the points A,B and C are collinear.
View SolutionHow would you rewrite Euclid’s fifth postulate so that it would be easier to understand ?
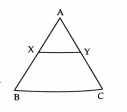
View SolutionIn the fig. `AX=1/2AB,AY=1/2AC` and AX=AY, prove that AB=AC
View SolutionTwo distinct lines in a plane can have two points in common.
View SolutionIt is known that x+y=10 and that x=z. show that z+y=10?
View SolutionLook at the figures. Show that the length AH> sum of lenghts of AB+BC+CD

View SolutionIn fig. `angleABC=angleACB` and `angle3=angle4`. Prove that `angle1=angle2`
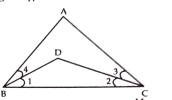
View SolutionIf a transversal intersects two parallel line then corresponding angles are an necessarily equal.
View SolutionIf a ray stands on a line, then the sum of angles so formed is equal to `10^@`. Is this system of axioms consistent? Justify your answer.
View SolutionIf two lines intersect, then vertically opposite angles are......... .
View SolutionIf a ray stands on a line, then the sum of the two adjacent angles so formed is............... .
View SolutionThe things which are double of the same thing are equal to one another.
View SolutionIf equals are added to equals the wholes are equal.
View SolutionThe things which are double of the same thing are equal to one another.
View Solution
EXERCISE questions and complete solutions for chapter INTRODUCTION TO EUCLID'S GEOMETRY of MODERN PUBLICATION of Class 9