Complete Solutions to LEVEL-2 of chapter STATISTICS of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | STATISTICS |
| PEARSON IIT JEE FOUNDATION | LEVEL-2 |
| Number Of Questions | 20 |
| Academic Year | 2023-24 |
If the ratio of mean and median of a certain data is 2:3, then find the ratio of its mode and mean.
View SolutionIf the mean of the following distribution is 13, then p =
`{:(x_(i) :,5,10,12,17,16,20),(f_(i) :,9,3,P,8,7,5):}`
View SolutionIf the ratio of mode and median of a certain data is 6:5, then find the ratio of its mean and median.
View SolutionIf the arithmetic mean of the following distribution is 8.2, then a =
`{:(x_(i) " :",1,3,5,9,11,13),(f_(i)" :",3,2,7,a,4,8):}`
View SolutionThe median of the series `8,12,15,7,x,19,22` lies in the interval
View SolutionThe mode of the following distribution is
{("Class interval",f),(" "1-5,4),(" "6-10,7),(" "11-15,10),(" "16-20,8),(" "21-25,6):}
View SolutionThe mean of the following data is
{("Class interval",f),(" "10-15,5),(" "15-20,7),(" "20-25,3),(" "25-30,4),(" "30-35,8):}
View SolutionThe median of the following frequency distribution is
`{:("Class interval",f),(" "0-10,5),(" "10-20,8),(" "20-30,7),(" "30-40,10),(" "40-50,20):}`
View SolutionFind the quartile deviation of the following discrete series.
`{:(x,7,5,4,8,12,10),(f,2,4,6,10,9,7):}`
View Solution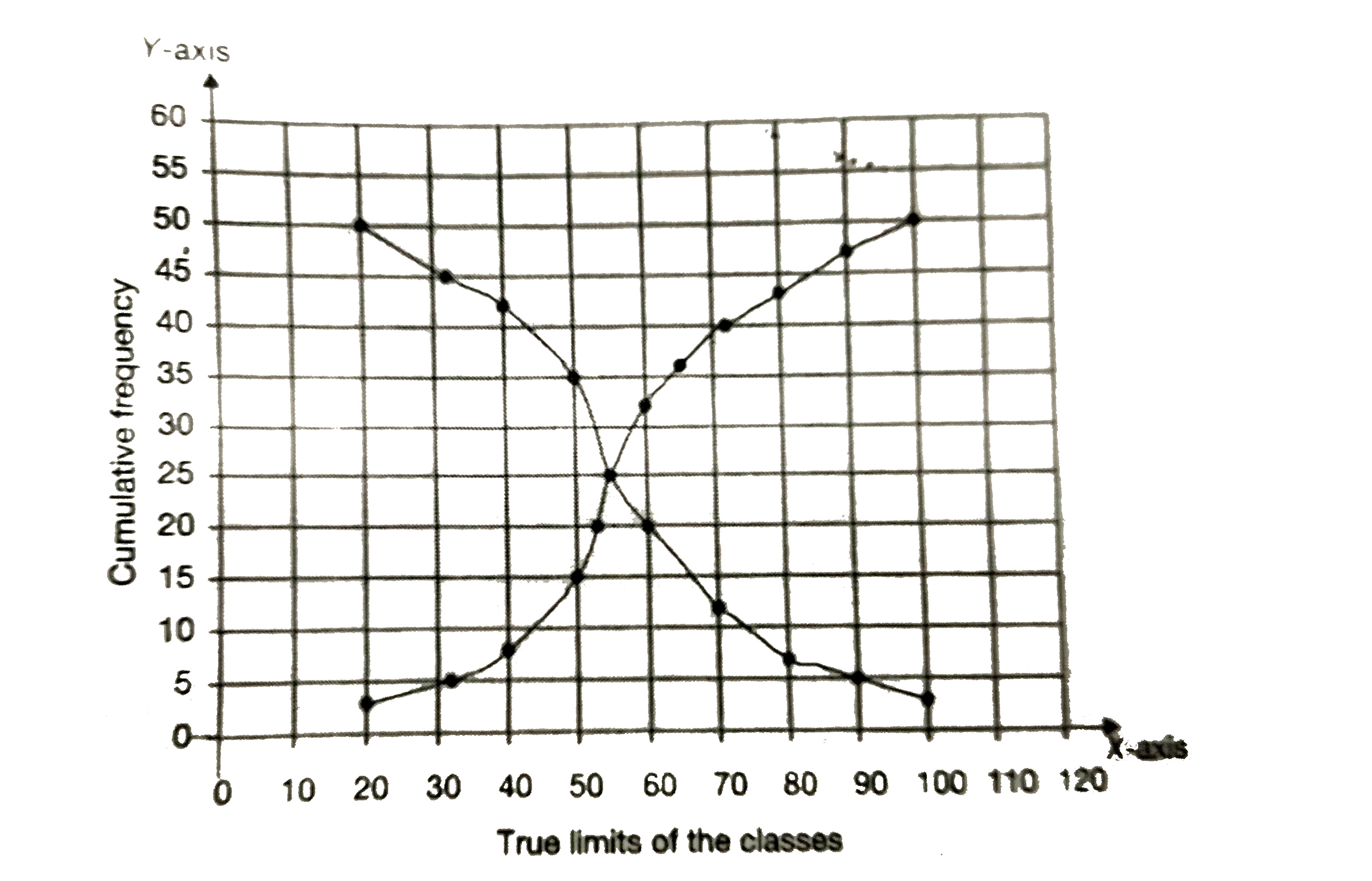
Find the number of students who scored less than or equal to 50% if marks.
View Solution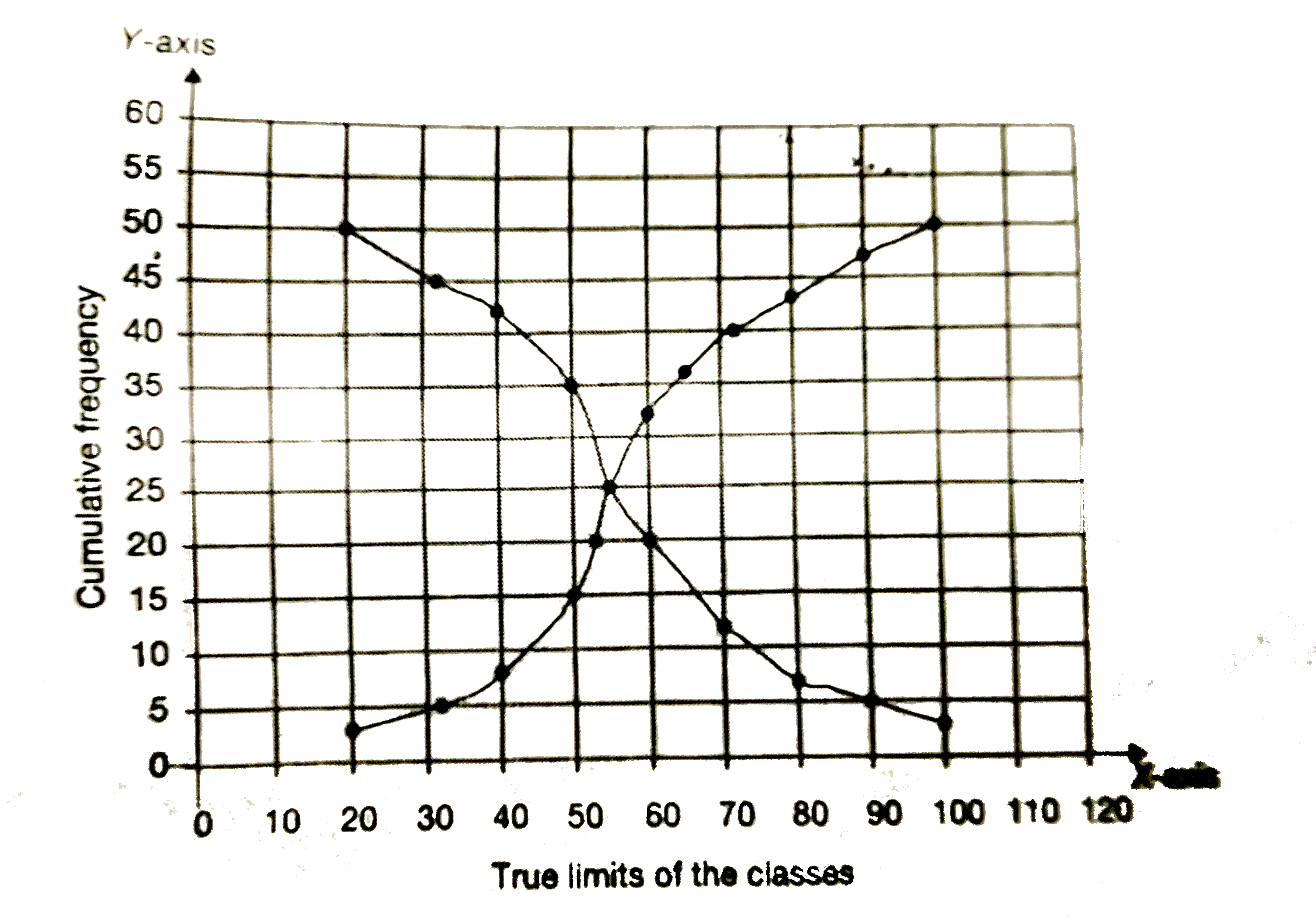
Find the number of students who scored greater than or equal to 90% of marks.
View SolutionIn a class of 15 students, on an average, each student got 12 books. If exactly two students received same number of books, and remaining student books average be an integer then which of the following could be the number of books received by each of the two students who received same number of books? 1.) 11 2.) 15 3.) 20 4.) 25
View SolutionFind the quartile deviation of the following discrete series.
`{:(x,3,5,6,8,10,12),(f,7,2,3,4,5,6):}`
View Solution`{:("Weight(in kg)","Number of students"),(" "20," "8),(" "22," "4),(" "24," "3),(" "25," "7),(" "30," "5):}`
Find the mean deviation (approximately) about the median for the above data.
View SolutionFind the mean deviation (approximately) about the mean for the following.
`{:("Class interval",f),(" "0-5,3),(" "5-0,4),(" "10-15,8),(" "15-20,10),(" "20-25,5):}`
View SolutionIf the average mark of 15 students is 60 and the average mark of another 10 students is 70, then find the average mark of 25 students.
The following are the steps involved in solving the above problem. Arrange them in sequential order.
(A) Average marks of 25 students `= (1600)/(25) = 64`
(B) The total marks of 15 students `= 15 xx 60 = 900`
The total marks of 10 students `= 10 xx 70 = 700`
(C) The total marks of 25 students = 900 + 700 = 1600
View SolutionIn a class of 25 boys and 20 girls, the mean weight of the boys is 40 kg and the mean weight of the girls is 35 kg. Find the mean weight of the class.
The following are the steps involved in solving the above problem. Arrange them in sequential order.
(A) The total weight of 25 boys `= 25 xx 40 = 1000` kg
The total weight of 20 girls `=20 xx 35 = 700` kg
(B) The mean weight of the class `= (1700)/(45) = 37(7)/(9)`kg
(C) The total weight of 45 students = 1000 kg + 700 kg = 1700 kg
View SolutionIf `p lt q lt 2p`, the median and mean of p, q and 2p are 36 and 31 respectively, then find the mean of p and q.
View SolutionIf `x lt y lt 2x`, the median and the mean of x, y and 2x are 27 and 33 respectively, then find the mean of x and y.
View SolutionThe mean of a set of 12 observations is 10 and another set of 8 observations is 12. The mean of combined set is ______.
View Solution
LEVEL-2 questions and complete solutions for chapter STATISTICS of PEARSON IIT JEE FOUNDATION of Class 9