Complete Solutions to EXERCISE 12.5 of chapter CIRCLES of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | CIRCLES |
| SUBHASH PUBLICATION | EXERCISE 12.5 |
| Number Of Questions | 12 |
| Academic Year | 2023-24 |
In Fig. 12.36 A, B and C are three points on a circle with centre O such that `BOC=30^@ and AOB = 60^@`. If D is a point on the circle other than the arc ABC, find ADC.
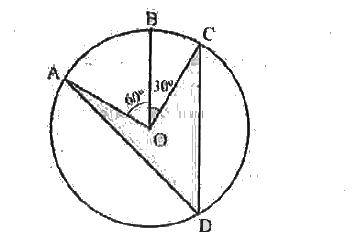
View SolutionA chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.

View SolutionIn the figure, `PQR=100^@`, where P, Q and R are points on a circle with centre O. Find OPR.
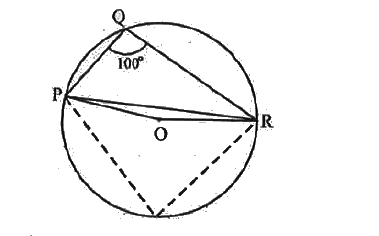
View SolutionIn the figure, `ABC=69^@, ACB=31^@`, find BDC.

View SolutionIn Fig, A, B, C and D are four points on a circle AC and BD intersect at a point E such that `BEC=130^@ and ECD=20^@`. Find BAC.
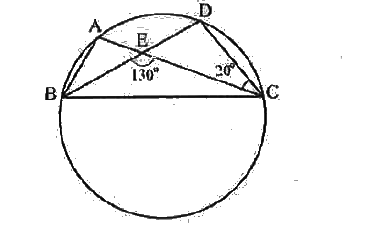
View SolutionABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If `angleDBC=70^@, angleBAC " is " 30^@`, find `angleBCD`.
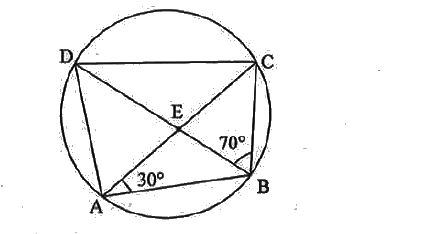
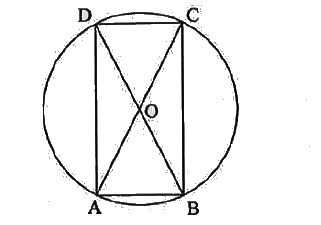
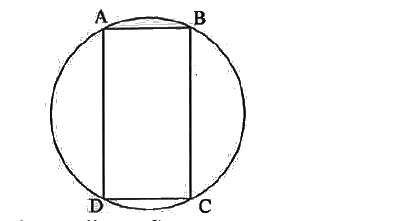
View SolutionIf diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral prove that it is a rectangle.
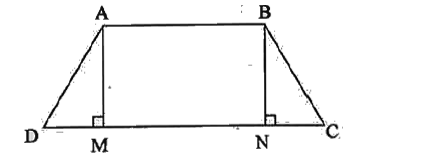
View SolutionIf the non-parallel sides of a trapezium are equal, prove that it is cyclic.
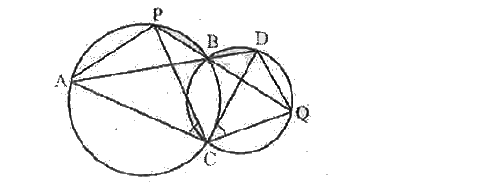
View SolutionTwo circle intersect at two points B and C. Through B, Two lines segment ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively. Prove that `ACP = QCD`.
View SolutionIf circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.

View SolutionABC and ADC are two right triangle with common hypotenuse AC. Prove that CAD = CBD.
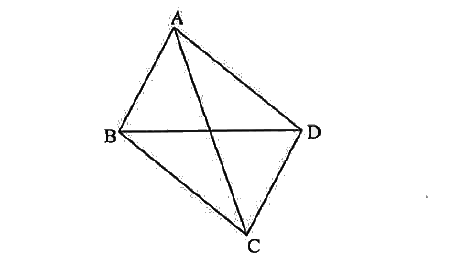
View SolutionProve that a cyclic parallelogram is a rectangle.
View Solution
EXERCISE 12.5 questions and complete solutions for chapter CIRCLES of VIDHYASANGAM - RAO'S ACADEMY MATHS (KANNADA ENGLISH) of Class 9