Complete Solutions to Question Bank of chapter POLYNOMIALS of Class 9 book with complete answers and questions
| Class 9 | MATHS |
| Chapter | POLYNOMIALS |
| V PUBLICATION | Question Bank |
| Number Of Questions | 24 |
| Academic Year | 2023-24 |
In rectangle with one side 1cm shorter than the other , take the length of the shorter side as x cm.
i) Taking their perimeters as p(x) cm, write the relation between p(x) and x as an equation.
ii) Taking their areas as a(x) sq.cm, write the relation between a(x) and x as an equation.
iii) Calculate p(1),p(2),p(3),p(4),p(5). Do you see any pattern?
iv) Calculate a(1),a(2),a(3),a(4),a(5).Do you see any pattern?05:00View SolutionFrom the four corners of a rectangle, small squares are cut off and the sides are folded up to make a box, as shown below:(fig)
i) Taking a side of the square as x cm, write the dimensions of the boxin terms of x.
ii) Taking the volume of the box as v(x) cubic cm, write the relation between v(x) and x as an equation.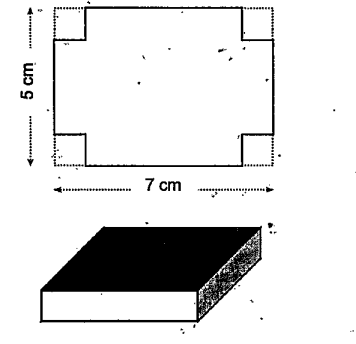 03:14View Solution
03:14View SolutionConsider all rectangles that can be made with a 1m long rope. Take one of its side as x cm and the area enclosed as a(x) sq.cm.
i) Write the relation between a(x) and xas in equation
ii) Why are the numbers a(10) and a(40) equal?
iii) To get the same number as a(x), for two different numbers as x, what must be the relation between the numbers?03:28View SolutionWrite each of the relations below in algebra and see if it gives a polynomial. Give reasons for your conclusions also.
i) A 1m wide path goes around a square ground. The relation between the length of a side of the ground and the area of the path.
ii) A liquid contains 7 litres of water and 8 litres of acid. More acid is added to it. The relation between the amount of acid added and the change in the percentage of acid in the liquid.
iii) Two poles of heights 3m and 4 m are erected upright on the ground, 5m apart. A rope is to be stretched from the top of one pole to some point on the groundand from there to the top of the other pole(fig).The relation between the distance of the point on the ground from the foot of a pole and the total length of the rope.
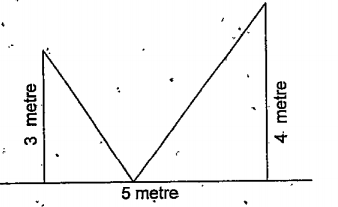 03:22View Solution
03:22View SolutionWrite each of the operations below as algebraic expressions, find out which are polynomials and explain why.
i) Sum of a number and its reciprocal.
ii) Sum of a number and its square root.
iii) Product of the sum and difference of a number and its square root.03:04View SolutionFind p(1) and p(10) in the following polynomials.
i) p(x)= 2x+5
ii) p(x)=`3x^ 2 + 6 x + 1 `
iii) p(x)=` 4 x^ 3 + 2 x^ 2 + 3 x + 7 `03:27View SolutionFind p(0), p(1) and p(-1) in the following polynomials.
i) p(x)= 3x+6
ii)p(x)= `3 x^ 2` + 6 x + 102:41View SolutionFind polynomial p(x) satisfying each set of conditions below.
i) First degree polynomial with p(1)=1 and p(2) =3.
ii) First degree polynomial with p(1) =-1 and p(-2)=3.04:31View SolutionWrite down the algebraic expression to find out each of the following. Discuss whether each is a polynomial and why?
The perimeter and area of a rectangle in which the length is 1cm more than the width?
The surface area and volume of a rectangular block in which the length is 1 cm more than the width and the height is 1cm more than the length.06:27View SolutionWhat is the algebraic expression denoting the hypotenuse of a right angled triangle when enlarging by increasing the perpendicular sides of length 4cm and 3cm equally?
04:40View SolutionThe area of a rectangle is 50 sq.cm. Write down the algebraic expression denoting the perimeter of such rectangles when changing its length and breadth without changing area?
03:01View SolutionFor p(x)=` 2 x^ 2 − 3 x + 1 `, find p(0),p(1),p(-1)
01:18View SolutionIf p(x)=` 3 x^ 2 − a x + 1 `and we want p(1) =2 what number should we take in the place of a?
00:55View SolutionIf p(x)=` 2 x^ 3 + a x^ 2 − 7 x + b ` and we want p(1)=3 and p(2)=19 what should be a and b?
02:04View SolutionA ball is thrown upwards with a speed of 40m/cm loses speed at the rate of 10m/cm every second. Write down the algebraic expression which gives the speed of the ball after x seconds? Find the speed when x=1,2,3…..?
03:43View SolutionIn the polynomial p(x)= ` 2 x^ 3 − 3 x^ 2 − 11 x − 6 ` find p(3),p(-2) and p(` 2 `). What is its peculiarity?
01:55View SolutionFor p(x)= `ax^3` + `b x^2` + c x + d .If p(1)=p(-1) prove that a+c=0
03:04View Solutiona)If p(x) =4x-4, find the numbers p(1) and p(2).
b) Write a first degree polynomial q(x) where q(1)=1 and q(2)=5.02:51View Solution`100xx17=1700`,isn't it? So, when 1700 is divided by 100 will get quotient 11.
What if 1718 is divided by 100?
Quotient 17 and remainder 18 will get. Similarly product of the polynomial x+1 and x+2 ix2+ 3 x + 2 `. So 1 added to the polynomial ` x 2 + 3 x + 2 `is divided by x+1 will get the quotient x+2 and remainder 1.
a) What is the quotient if 1718 is divided by17?
b) What number should be added to ` x 2 + 3 x + 2 ` gives the polynomial ` x 2 + 3 x `?
c) What should be the remainder when ` x 2 + 3 x `is divided by x+1?
d) What polynomial should be added to ` x 2 + 3 x + 2 ` will get ` x 2 + 4 x + 3 `?
e) What is the quotient when ` x 2 + 4 x + 3 `is divided by x+1?02:02View SolutionThe expression given below, which of them are polynomials.
i)` x^ 3 + 1 2 x^ 2 + 2 x − 5 `
ii) `x^2`+12x−2
iii)`x^2`+√x−3
iv) -3x+7
v) z^2+12z06:25View SolutionIn the polynomial p(x)=` 2 x^ 3 − 3 x^ 2 − 7 x + 3 `, find p(-1),p(-2),p(0),p(2). Of these which is the largest?
03:04View SolutionIn the polynomial p(x)=` a x^ 3 + b x^ 2 + c x + d ` if p(2)=p(-2), prove that 4a+c=0.
01:56View SolutionWrite down two third degree equations such that p(1)=p(-1).
04:33View SolutionLength of a rectangle is one less than two times its breadth.
a) If length is x, write its breadth.
b) Write the polynomial indicating its area and perimeter.03:55View Solution
Question Bank questions and complete solutions for chapter POLYNOMIALS of V PUBLICATION of Class 9