Topic 2 (Topic test 2) from ARIHANT PRAKASHAN PROBABILITY for Class 12
| Class 12 | MATHS |
| Chapter | PROBABILITY |
| ARIHANT PRAKASHAN | Topic 2 (Topic test 2) |
| Medium | English |
| Academic Year | 2023-2024 |
Question 5
Suppose that the probability that your alarm goes off in the morning is 0.9. If the alarm goes off, the probability is 0.8 that you attend your 8 a.m. class. If the alarm does not go to off, the probability that you make your 8 a.m.class is 0.5. Find the probability that you make your 8 a.m. class.View SolutionQuestion 9
Out of the adult population in a village 50% are farmers, 30% do business and 20% are service holders. It is known that 10% of the farmers, 20% of the business holders and 50% of service holders are above poverty line. What is the probability that a member chosen from any one of the adult population, selected at random, is above poverty line?View SolutionQuestion 14
A bag contains 5 white and 3 black balls, a second bag contains 4 white and 5 black balls, a third bag contains 3 white and 6 black balls. A bag is selected at rendom and a ball is drawn. Find the probability that the ball is black.
Do the problem assuming that the probability of choosing the first bag is twice as much as choosing the second bag, which is twice as much as choosing the third bag.View SolutionQuestion 17
From a survey conducted in a cancer hospital it is found that 10% of the patients were alcoholics, 30% chew gutka and 40% have no specific carcinogenic habits. If cancer strikes 80% of the smokers, 70% of alcoholics, 50% of the non specific, then estimateic the probability that a cancer patient chosen from any one of the above types, selected at random,
has no specific carcinogenic habits.View SolutionQuestion 20
In a class of 75 students, 15 are above average, 45 are average and the rest below average achievers. The probability that an above average achieving student fail is 0.005, that an average achieving student fails is 0.05 and the probability of a below average achieving student failing is 0.15. If a student is known to have passed, then what is the probability that he is a below average achiever?View SolutionQuestion 21
From a survey conducted in a cancer hospital it is found that 10% of the patients were alcoholics, 30% chew gutka and 40% have no specific carcinogenic habits. If cancer strikes 80% of the smokers, 70% of alcoholics, 50% of the non specific, then estimateic the probability that a cancer patient chosen from any one of the above types, selected at random,
has no specific carcinogenic habits.View SolutionQuestion 24
From a lot of 6 items containing 2 defective items, a sample of 4 items are drawn at random, Let the random variable X denote the number of defective items in the sample. If the sample is drawn without replacement, find
(i) The probability distribution of X
(ii) Mean of X
(ii) Variance of XView Solution
All Exercises from ARIHANT PRAKASHAN Class 12 MATHS PROBABILITY
- TOPIC 1 (TOPIC TEST 1)
- TOPIC 1 (PRACTICE QUESTIONS (1 MARK QUESTION))
- TOPIC 1 (PRACTICE QUESTIONS (4 MARK QUESTION))
- TOPIC 1 (PRACTICE QUESTIONS (6 MARK QUESTION))
- TOPIC 2 (TOPIC TEST 2)
- TOPIC 2 (PRACTICE QUESTION ( 1MARK QUESTION)
- TOPIC 2 (PRACTICE QUESTION ( 4 MARK QUESTION)
- TOPIC 2 (PRACTICE QUESTION ( 6 MARK QUESTION)
- CHAPTER TEST (1 MARK QUESTION)
- CHAPTER TEST (4 MARK QUESTION)
- CHAPTER TEST (6 MARK QUESTION)

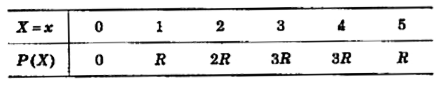 Determine
Determine