EXERCISE (COMPREHENSION TYPE PROBLEMS) from SHRI BALAJI MATHS (ENGLISH) APPLICATION OF DERIVATIVES for Class 12
| Class 12 | MATHS |
| Chapter | APPLICATION OF DERIVATIVES |
| SHRI BALAJI MATHS (ENGLISH) | EXERCISE (COMPREHENSION TYPE PROBLEMS) |
| Medium | English |
| Academic Year | 2023-2024 |
Question 3
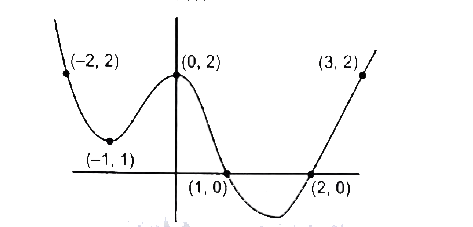
Let f(x)=⎡⎢ ⎢⎣1−x0≤x≤101<x≤2andg(x)=∫x0f(t)dt.(2−x)22<x≤3
Let the tangent to the curve y=g(x) at point P whose abscissa is 52 cuts x-axis in point Q.
Let the prependicular from point Q on x-axis meets the curve y=g(x) in point R .Find equation of tangent at to y=g(x) at P .Also the value of
g(1)=View SolutionQuestion 4
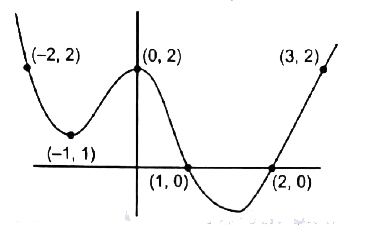
Let f(x)=⎡⎢ ⎢⎣1−x0≤x≤101<x≤2andg(x)=∫x0f(t)dt.(2−x)22<x≤3
Let the tangent to the curve y=g(x) at point P whose abscissa is 52 cuts x-axis in point Q.
Let the prependicular from point Q on x-axis meets the curve y=g(x) in point R .Find equation of tangent at to y=g(x) at P .Also the value of
g(1)=View SolutionQuestion 5
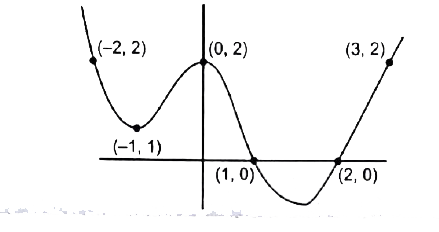
Let f(x)=⎡⎢ ⎢⎣1−x0≤x≤101<x≤2andg(x)=∫x0f(t)dt.(2−x)22<x≤3
Let the tangent to the curve y=g(x) at point P whose abscissa is 52 cuts x-axis in point Q.
Let the prependicular from point Q on x-axis meets the curve y=g(x) in point R .Find equation of tangent at to y=g(x) at P .Also the value of
g(1)=View SolutionQuestion 6
Let f(x)<0∀x∈(−∞,0)andf(x)>0,∀x∈(0,∞) also f(0)=0, Again f'(x)<0,∀x∈(−∞,−1)andf'(x)>0,∀x∈(−1,∞) also f'(−1)=0 given limx→−∞f(x)=0andlimx→∞f(x)=∞ and function is twice differentiable.
If f'(x)<0∀x∈(0,∞)andf'(0)=1 then number of solutions of equation f(x)=x2 is : (a) 1 (b) 2 (c) 3 (d) 4View SolutionQuestion 7
Let f(x)<0∀x∈(−∞,0)andf(x)>0,∀x∈(0,∞) also f(0)=0, Again f'(x)<0,∀x∈(−∞,−1)andf'(x)>0,∀x∈(−1,∞) also f'(−1)=0 given limx→−∞f(x)=0andlimx→∞f(x)=∞ and function is twice differentiable.
If f'(x)<0∀x∈(0,∞)andf'(0)=1 then number of solutions of equation f(x)=x2 is : (a) 1 (b) 2 (c) 3 (d) 4View SolutionQuestion 13
The differentiable function y=f(x) has a property that the chord joining any two points A(x1,f(x1)andB(x2,f(x2)) always intersects y-axis at (0,2x1x2). Given that f(1)=−1. then:
In which of the following intervals, the Rolle's theorem is applicable to the function F(x)=f(x)+x ? (a) [−1,0] (b) [0,1] (c) [−1,1] (d) [0,2]View Solution