DECIMAL for Class 6 MATHS
Introduction
Baccho aapne apni previous classes m parts and wholes ka aur unse related basic concepts pdhe hai. Aapne previous chapter m fractions k baare m bhi pdha aur ye jaana ki jab hume ek part aur ek whole ko represent krna hota hai to hum unhe fractions k form m krte hai. Decimal bhi ek aisa standard system hota hai jisse integers and non-integer numbers ko denote kia jaata hai. It is based on or counted in tens or tenths. Aapne apni day to day lives m decimals ko use hote hue dekha hoga. For example, Suresh scored 9.8 marks out of 10 in his maths exam. Or, a packet of chips contains 10.05 grams of fat.
In this chapter we will learn more about decimals and will build a better understanding on our previous topics. So let's get started!
DECIMALS
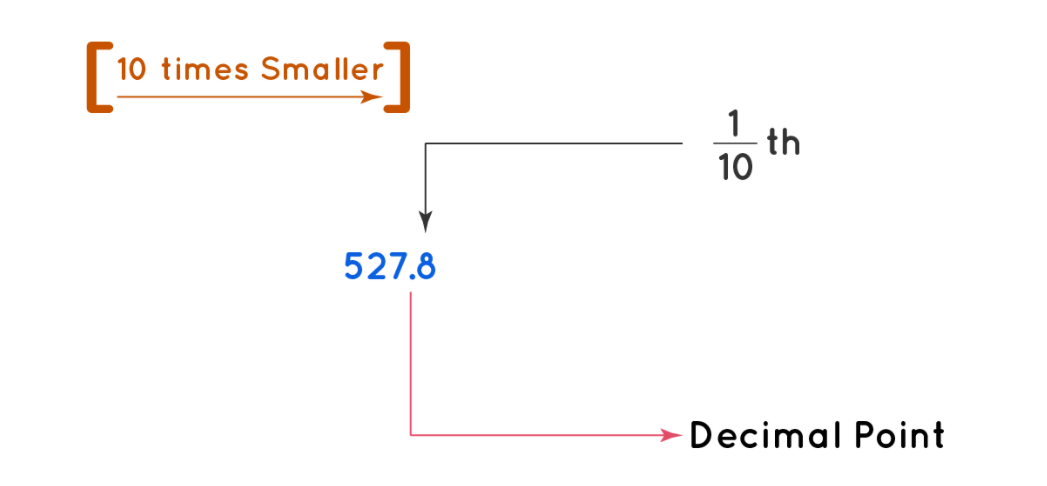
A decimal number system is used to express the whole number and fraction together. Here, we will separate the whole number from the fraction by inserting a ".", which is called a decimal point. The numbers to the left of the decimal point are the integers or whole numbers and the numbers to the right of the decimal point are called decimal numbers. If we go right from the ones' place, the next place will be (110) times smaller, which will be (110)th or one-tenth position. There are many real-life situations in which you might be using decimals without even realizing it. For example, let's say you are going to take a cone of ice cream. The vendor tells you that the price of ice cream is Rs. 20 and 50 paise. Now, if you want to express this whole amount in one figure, you will say that the price of the ice cream cone is Rs. 20.50.

TENTHS
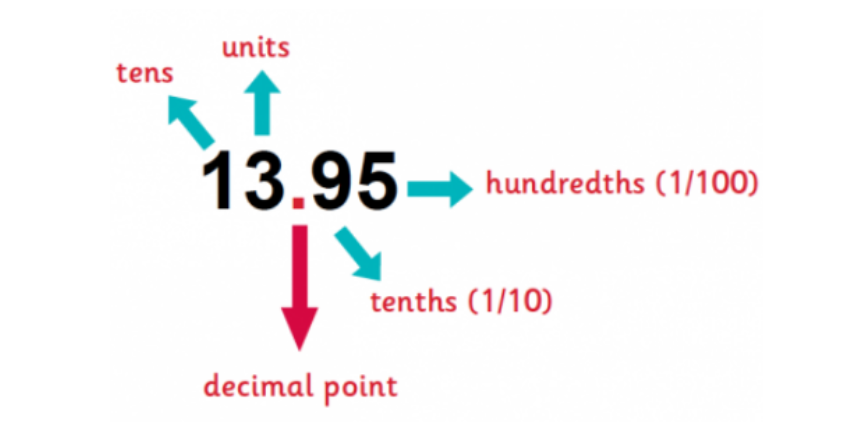
Baccho humne place value system k baare m previous chapters m pdha tha. Decimals k case m bhi same place value system hota hai for the whole number part of it (left of decimal point). But after the decimal point, there is a different world of numbers going on in which we use decimal fractions to represent the value. Jab hum left side move krte hai, each place is ten times greater than the previous place digit. So, to the right of one's place, we have tenths (110) and to the right of tenths, we have hundredths (1100), and so on.
Let's look at an example to see how we can express using decimals.
EXAMPLE: Ravi and Raju measured the lengths of their pencils. Ravi’s pencil was 7 cm 5mm long and Raju’s pencil was 8 cm 3 mm long. Can you express these lengths in centimeter using decimals?"
SOLUTION:
We know that 10mm=1cm
Therefore, 1mm=110cm or one-tenth cm =0.1cm
Now, length of Ravi’s pencil=7cm5mm
=7510cm i.e. 7cm and 5 tenths of a cm
=7.5cm
The length of Raju’s pencil =8cm3mm
=8310cm i.e. 8cm and 3 tenths of a cm
=8.3cm
Representing Decimals on number line
Decimals are another form of a fraction in which the whole number is separated from the fractional part using a dot. Baccho aapne previously fractions ko number line p represent krna seekha tha. Decimals on a number line are very similar to the representation of fractions on a number line except for its way of representation.
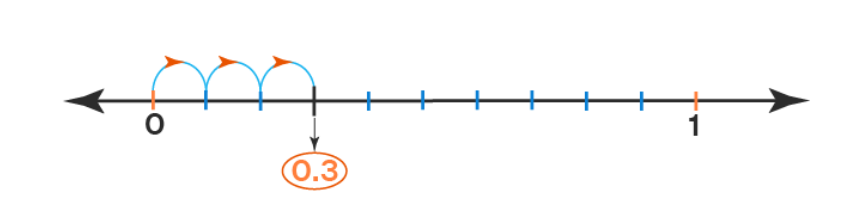
The right-hand side of 0 represents the positive region. Let us represent 0.3 on a number line using the following steps shown below.
Step I: Draw a number line by marking 0 as the reference.
Step II: Identify the integers between which the decimal lies and mark them. 0.3 lies between 0 and 1.
Step III: We will now be making 10 divisions between the integers 0 and 1.
Step IV: Now, we will be moving towards the right starting from 0 by the number of steps equivalent to the right-most digit value after the decimal point. For 0.3 we will be moving 3 places towards the right.
Inter-conversion of fractions and decimals
1. Fractions as decimals
Baccho humne seekha ki hum kisi fraction ko jiska denominator 10 ho usko decimals m kaise represent kr skte hai.
Let us now try to find decimal representation of
(a)
115
(b) 12
(a) We know that
115=2210=20+210+=2010+210=2+210=2.2
Therefore,
2210=2.2 (in decimal notation.)
(b) In 12 , the denominator is 2. For writing in decimal notation, the denominator should be 10. We already know
how to make an equivalent fraction. So,
12=1×52×5=510=0.5
Therefore, 12 is 0.5 in decimal notation.

2. Decimals as fractions
Baccho abhi tk humne seekha ki hum fractions ko jinke denominators 10,2 ya 5 ho, unko decimals m kaise represent krte hai. Let us now see how to write 1.2 as a fraction.
1.2=1+210=1010+210=1210
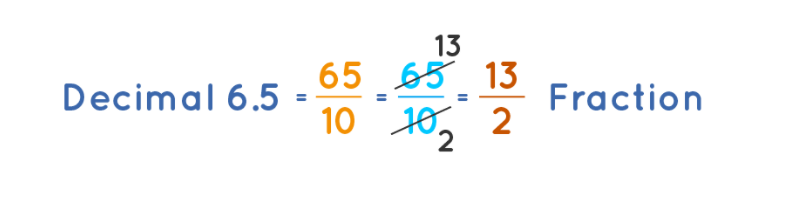
Example 1
Write each of the following as decimals :
(a) Seven-tenths
(b) Two tens and nine-tenths
(c) Fourteen point six
(d) One hundred and two ones
(e) Six hundred point eight
(a) Seven-tenths =710=0.7
(b) Two tens and nine-tenths =2×10+9×110=20.9
(c) Fourteen point six =14.60
(d) One hundred and two one =1×100+2×1=102.0
(e)Six hundred point eight =600.8
Example 2
Write each of the following as decimal :
(a) 30+6+210
(b) 600+2+810
(a)30+6+210
We have 3 tens, 6 ones and 2 tenths.
Therefore, the decimal representation is 36.2.
(b)600+2+810
Note that it has 6 hundreds, no tens, 2 ones and 8 tenths.
Therefore, the decimal representation is 602.8
HUNDREDTHS
The value of the digit at the second place after the decimal point is hundredths place value. The number in that place tells you how many hundredths are there. Hundredths can be calculated as 1 unit divided in 100 equal parts i.e 0.01.One block divided into 100 equal parts means each part is 1100 (one-hundredth) of a unit. It can be written as 0.01 in decimal notation.
Every fraction with denominator 100 can be written in decimal notation and vice-versa.
Let us consider a decimal number 0.5694 to see the different place values of each digit:
Let us look at the following examples to understand how to express decimals with hundredths.
Example 3
Write as fractions in lowest terms.
(a)0.60
(b)0.05
(c)0.75
(d)0.18
(e)0.25
(f)0.125
(g)0.066
(a) 0.60=60100=3×25×2=610=35
(b) 0.05=5100=5×120×5=120
(c) 0.75=75100=3×254×25=34
(d) 0.18=18100=9×250×2=950
(e) 0.25=25100=2525×4=14
(f) 0.125=1251000=1258×125=18
(g) 0.066=661000=33500
Example 4
Write each of the following as a decimal.
(a) Three hundred six and seven-hundredths.
(b) Eleven point two three five.
(c) Nine and twenty five thousandths
(a) Three hundred six and seven-hundredths =306+7100=306.07
(b) Eleven point two three five =11+.235=11.235
(c) Nine and twenty five thousandths =9+251000=9.025
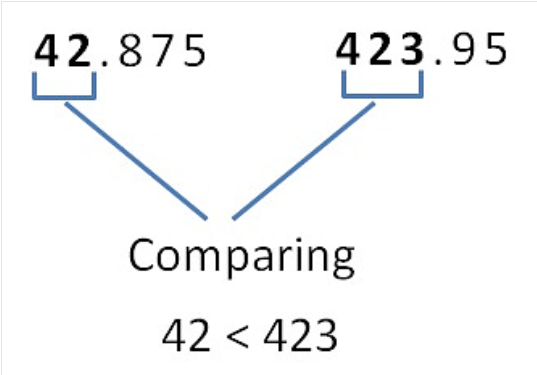
COMPARING DECIMALS
Baccho ab hum dekhenge ki hum decimals ko compare kaise krte hai. Hume do cheezon ka dhyaan rakhna hota hai while comparing decimals.
We repeat this process and keep on comparing digits to the right until we get the unequal digits.
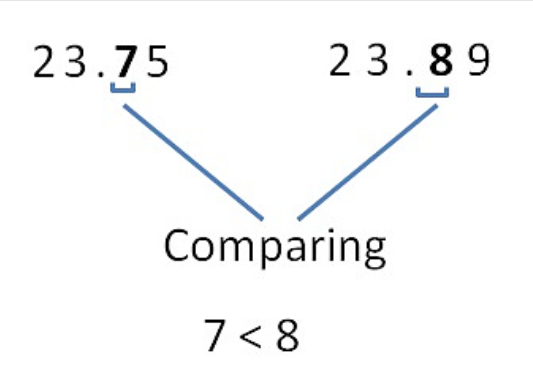
For example, let's compare 23.789 with 23.759. Here we see digits before the decimal point are equal which is 23=23. Now moving on to the tenths digits to compare. i.e., 23.789 with 23.759, we get 7=7. Both of them are equal. Now we move to the next term to the right of the tenths digit which is the hundredth digit. i.e., 23.789 with 23.759. Now, since 8>5, then we can say that 23.789>23.759.
∴23.789>23.759.
Example 5
Which is greater?
(a)0.3or0.4
(b)0.07or0.02
(c)3or0.8
(d)0.5or0.05
(e)1.23or1.2
(f)0.099or0.19
(g)1.5or1.50
(h)1.431or1.490
(i)3.3or3.300
(j)5.64or5.603
(a) 0.4>0.3
(b) 0.07>0.02
(c) 3>0.8
(d) 0.5>0.05
(e) 1.23>1.2
(f) 0.19>0.099
(g) 1.5=1.50
(h) 1.490>1.431
(i)3.3=3.300
(j)5.64>5.603
USING DECIMALS
We often use decimals in our daily life in multiple fields. Let us look at some of them:
1. MONEY
1 paisa =Rs.1100=Rs.0.01
85 paise =Rs.85100=Rs.0.85
125 paise =Rs.1 and 25 paise =Rs.1.25
65 paise =Rs.65100=Rs.0.65
2. LENGTH
1cm=1100m=0.01m
46cm=46100m=0.46m
126cm=100cm+26cm=1m+26100m=1.26m
3. WEIGHT
1g=11000kg=0.001kg
2650g=2000g+650g=20001000kg+6501000kg=2kg+0.650kg=2.650kg
Example 6
Express as meters using decimals.
(a)15cm
(b)6cm
(c)2m45cm
(d)9m7cm
(e)419cm
We know, 100 cm = 1 m
So,
(i) 15 cm =15100=0.15 m
(ii) 6 cm =6100=0.06 m
(iii) 2 m 45 cm =(2+45100)=2.45 m
(iv) 9 m 7 cm =(9+7100)=9.07 m
(v) 419 cm =419100=4.19 m
Example 7
Express as cm using decimals.
(a)5mm
(b)60mm
(c)164mm
(d)9cm8mm
(e)93mm
We know, 10 mm = 1 cm
So,
(i) 5 mm=510=0.5 cm
(ii) 60 mm=6010=6 cm
(iii) 164 mm=16410=16.4 cm
(iv)9 cm 8 mm=(9+810)=9.8 cm
(v) 93 mm=9310=9.3 cm
Example 8
Express as kg using decimals:
(a)2g
(b)100g
(c)3750g
(d)5kg8g
(e)26kg50g
We know, 1000 g = 1 kg
So,
(i) 2 g =21000=0.002 kg
(ii) 100 g =1001000=0.1 kg
(iii) 3750 g =37501000=3.75 kg
(iv) 5 kg 8 g =(5+81000)=5.008 kg
(v) 26 kg 50 g =(26+501000)=26.05 kg
Example 9
Express as rupees using decimals.
(a)5 paise
(b)75 paise
(c)20 paise
(d)50 rupees 90 paise
(e)725 paise
We know, 100 paise = 1 rupee
So,
(i) 5 paise = 5100 = 0.05 rupees
(ii) 75 paise = 75100 = 0.75 rupees
(iii) 20 paise = 20100 = 0.20 rupees
(iv) 50 rupees 90 paise = (50+90100) = 50.90 rupees
(v) 725 paise = 725100 = 7.25 rupees
ADDITION OF NUMBERS WITH DECIMALS
Baccho before learning how to add two decimal numbers we need to learn some basic rules or steps to do that.
For the operation of addition in decimals, follow the steps given below:
Step 1: Write the numbers, such that the decimals are lined perfectly.
Step 2: Write zeroes in the places wherever the length of decimal numbers is not the same.
Step 3: Now add the decimal numbers and find the output to addition.
Example: Add: 2.53+1.14.
Solution:
If we observe the given decimals, we see that they are like decimals, so, we will place them in order and do the regular addition.
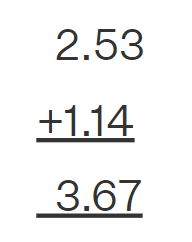
Therefore, after adding the decimals, we get the sum as 3.67
Example 10
Samson travelled 5 km 52 m by bus, 2 km 265 m by car and the rest 1km 30 m he walked. How much distance did he travel in all?
Distance travelled by Samson by bus = 5 km 52 m =5.052 km
Distance travelled by Samson by car = 2 km 265 m =2.265 km
Distance travelled by Samson by walking = 1 km 30 m =1.030 km
So, total distance travelled by Samson =5.052+2.265+1.030=8.347 km
Example 11
Rahul bought 4 kg 90 g of apples, 2 kg 60 g of grapes and 5 kg 300 g of mangoes. Find the total weight of all the fruits he bought
Weight of apples bought by Rahul = 4kg90g =4.090 kg
Weight of grapes bought by Rahul = 2kg60g =2.060 kg
Weight of mangoes bought by Rahul = 5kg300g =5.3 kg
So, total weight of fruits =4.090+2.060+5.300=11.450 kg
SUBTRACTION OF NUMBERS WITH DECIMALS
For the operation of subtraction in decimals, the rule of carryover is followed as in the normal whole numbers.
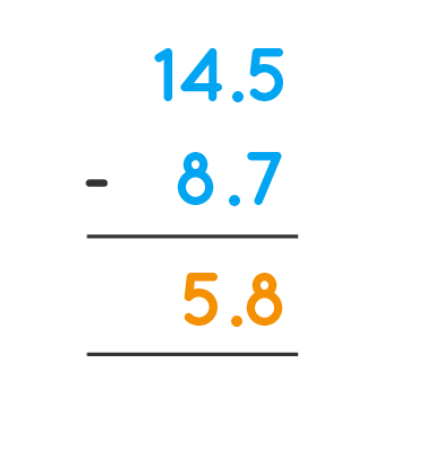
Let us understand this with an example:
14.5 - 8.7
. Here, we segregate the decimals and whole numbers and separately conduct the operation of subtraction.
14.5−8.7=(14+0.5)−(8+0.7)
=14+0.5−8−0.7
=(14−8)+(0.5−0.7)
=6−0.2
=5+1.0−0.2
=5+0.8
=5.8. Here the number 1 is taken from 6, to help for further subtraction. Also, the subtraction 1.0−0.2 is same as the subtraction 10−2=8. And a decimal is placed in the answer 0.8.
Example 12
Urmila’s school is at a distance of 5km350m from her house. She travels 1km70m on foot and the rest by bus. How much distance does she travel by bus?
Total distance=5.350km
distance traveled by on foot=1.070km
distance travelled by bus=total distance traveled - distance travelled by on foot
=5.35−1.07
=4.28km.
Example 13
Nasreen bought 3m20cm cloth for her shirt and 2m5cm cloth for her trouser. Find the total length of cloth bought by her.
Length of cloth bought for Nasreen shirt =3m20cm=3m+20100m=3.2m
Length of cloth bought for Nasreen trouser =2m5cm=2m+5100m=2.05m
So, total length of cloth bought by Nasreen =3.2+2.05=5.25m=5m25cm
SUMMARY
1. To understand the parts of one whole (i.e. a unit) we represent a unit by a block. One block divided into 10 equal parts means each part is 110 (one-tenth) of a unit. It can be written as 0.1 in decimal notation. The dot represents the decimal point and it
comes between the units place and the tenths place.
2. Every fraction with denominator 10 can be written in decimal notation and vice-versa.
3. One block divided into 100 equal parts means each part is 1100 (one-hundredth) of a unit. It can be written as 0.01 in decimal notation.
4. Every fraction with denominator 100 can be written in decimal notation and vice-versa.
5. In the place value table, as we go from left to the right, the multiplying factor becomes 110 of the previous factor.
The place value table can be further extended from hundredths to 110 of hundredths
i.e. thousandths (11000), which is written as 0.001 in decimal notation.
6. All decimals can also be represented on a number line.
7. Every decimal can be written as a fraction.
8. Any two decimal numbers can be compared among themselves. The comparison can start with the whole part. If the whole parts are equal then the tenth parts can be
compared and so on.
9. Decimals are used in many ways in our lives. For example, in representing units of money, length and weight.
Let's Revise
1. Baccho iss chapter m humne decimals k baare m seekha aur dekha ki hum apni day to day life m decimals ko kaha use krte hai.
2. Decimals ek aisa standard system hota hai jisse whole aur part ko saath m represent kia jaata hai. For example, One and a half can be written as 1.5.
3. Deceimals ko represent krne k lie hum whole aur part ko saath m likhte h by dividing them with a . between them. For example, 2.3,4.56,4.87,4245.343, etc.
4. Jab hum decimal point se right side move krte hai, to the first place is called tenths place and as we move forward the multiplying factor becomes 110 times the previous factor yaani ki tenths k baad we get hundredths, thousandths and so on.
5. Humne decimals ko number line p present krna bhi seekha. Number line p represent krne k lie hum do whole numbers k beech m ten divisions krte hai. For example, in order to represent 2.4 on number line, we'll show ten divisions between 2 and 3 and will mark the fourth division as 2.4.
6. Har decimal number fraction ki form m likha jaa skta hai. For example, 0.75 can be written as 34.
7. Humne decimals ko compare krna bhi seekha. In order to compare two decimals, we start with the whole part. If they are equal then we move to compare the tenths place, hundredths place and so on, until we find an inequality.
8. Hum decimals ko humari day to day life m bohot vastly use krte hai. For example, in money, we use terms like Rs. 70 and 50 paise which can be denoted as Rs. 70.50. We also use decimals in places like while measuring lengths (68.7cm,39.21m, etc) or while measuring weights like 73.4g,9.7kg, etc.