PRACTICAL GEOMETRY for Class 8 MATHS

Bachcho apne pichli class mein triangle ko draw karna sikha tha aur apne ye bhi dekha tha ki ek unique triangle ko draw karne ke lie $3$ measurements (of side and angle) ki jarurat hoti hai.
To ab question ye arise hota hai ki kya ek $4$ sided unique closed figure banane ke lie $4$ measurements sufficient hongi ? To es chiz ko samjhne ke lie chalo hum ek experiment perform karte hai jisme humare paas $4$ sticks hai jinme se $2$ sticks ki length $10$cm hai aur baki $2$ sticks ki length $8$cm hai. Agar hum unko perpendicularly join kare to hume ek rectangle $ABCD$ milega jiski length $10cm$ hogi aur width $8cm$. Agar hum us rectangle ko uski width ke along push karte hai to hume ek ||gm milega. Aur agar hum usi rectangle ko different direction mein push karte to hume ek different parallelogram milega jabki un $4$ sticks ki measurement same rahegi. To es experiment se hum ye conclude karte hai ki ek quadrilateral banane ke lie $4$ measurements sufficient nhi hai. To kya $5$ measurements sufficent hongi ? Let's see.
Humare paas jo rectangle $ABCD$ hai usme hum ek aur stick introduce karenge jiski length $BD$ yani diagonal of rectangle ke equal hogi aur us length ko hum $BD$ ke along tie kardenge. To ab hum ye dekh sakte hai ki rectangle ko open kiye bina hum usse push karke ek ||gm nhi bana sakte kyonki $BD$ ye nhi hone dega i.e., $5th$ stick ki wajh se hume ek unique rectangle mila aur isse hum kah sakte hai ki given sides se dusra koi quadrilateral formed nhi hoga.
To ab hum ye kah sakte hai ki $5$ measurements ek unique quadrilateral form kar sakte hai. Aap ye soch rhe honge ki kya $5$ measurements (of sides and angles) sufficient hongi ek unique quadrilateral banane ke lie ? Hum iss chapter mein yhi sab sikhne wale hai. So let's begin our study.

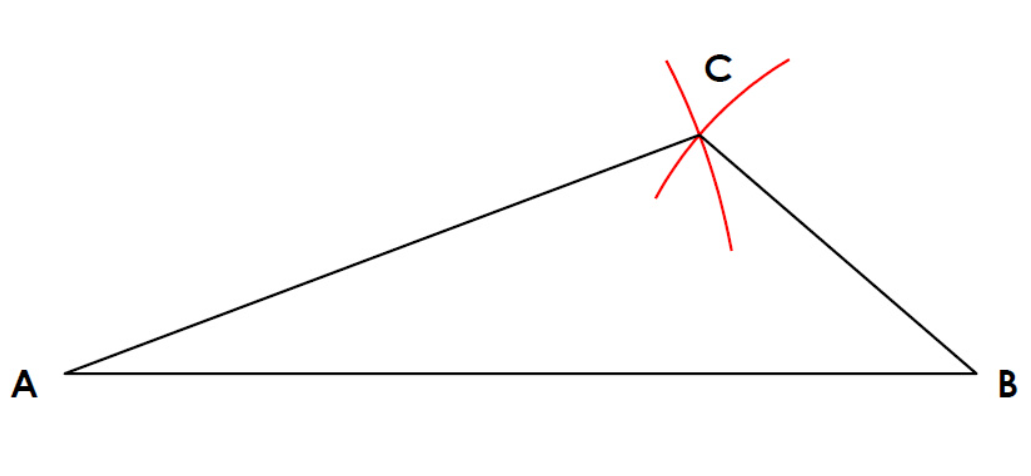
Baccho, before starting with construction of quadrilaterals, hum phle $SSS$ construction condition se triangle kaise draw karte hai wo recall karenge.
Bachcho kisi $\Delta ABC$ jiski sides, $AB=x$ cm, $BC=y$ cm aur $CA=z$ cm given ho usse hum in steps se construct ya draw kar sakte hai.

Bachcho ab hum dekhenge ki kaise ek unique quadrilateral ko draw kiya jata hai jab 5 measurement is tarh given ho,
• When four sides and one diagonal are given.
• When two diagonals and three sides are given.
• When two adjacent sides and three angles are given.
• When three sides and two included angles are given.
• When other special properties are known.

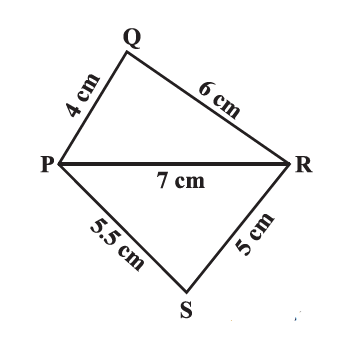
Bachcho maan lo ki hume ek quadrilateral $PQRS$ draw karna hai where $PQ=4$ cm, m, $QR=6$ cm, $RS=5$ cm, $PS=5.5$ cm aur $PR=7$ cm.
Step 1 : Bachcho sabse pahle hume ek rough sketch draw karna hai jisse hum quadrilateral ko visualize kar sake aur usme hum measurements ko mark kar denge.
Baccho, hume pta hai ki har quadrilateral do triangles m divide ho skta hai. To ab hum rough figure m quadrilateral ko do triangles ki tarah visualize krenge.

Step 2 : Ab hum $\Delta PQR$ construct krke using $SSS$ construction, jisme $PQ=4cm,QR=6cm,\text{and}PR=7cm$

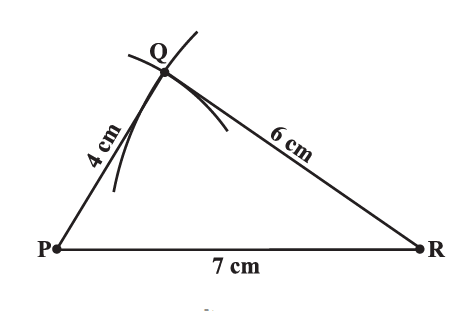
Baccho, traingle construct krne k baad hum ye dekh skte hai ki hume humare quadrilateral k three corner already mil chuke hai. Now we need the fourth corner to complete our quadrilateral.
Step 3 : Quadrilateral k fourth point $\left(S\right)$ ko locate krne k lie hum, $P$ and $R$ se do arcs draw krenge. Inn dono arcs ka radius humare quadrilateral ki bachi hui dono sides k length k jitna hoga.
Because $S$ is $5.5cm$ away from $P$, draw an arc from $P$ of radius $5.5cm$.

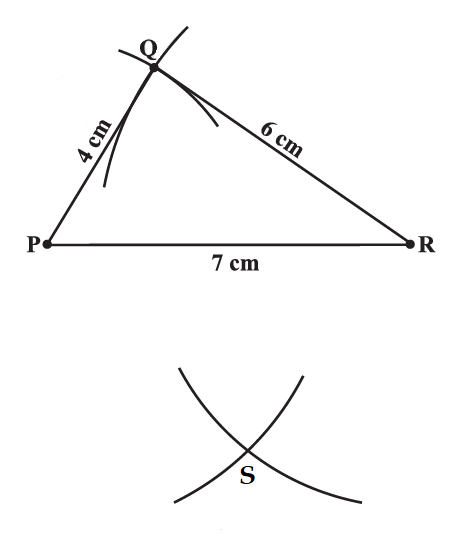
Step 5 : Similarly, kyunki $Q$ $5cm$ door hai $S$ se, we will draw an arc of radius $5cm$ from $Q$ and let it cut our previous arc at point $S$.
Ye humare quadrilateral ka fourth point bnjaaega.

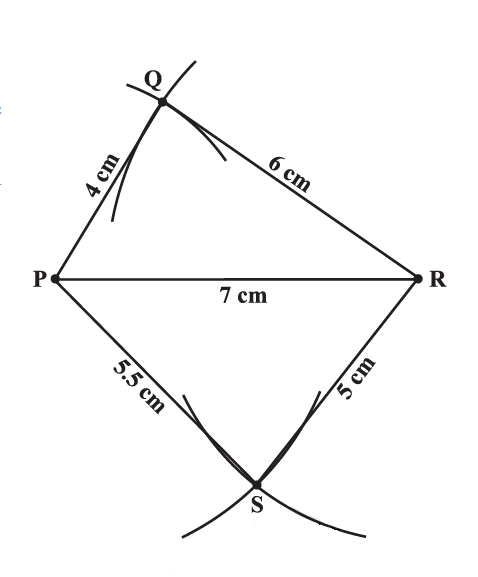
And with this, we constructed a quadrilateral when four sides and one diagonal were given.

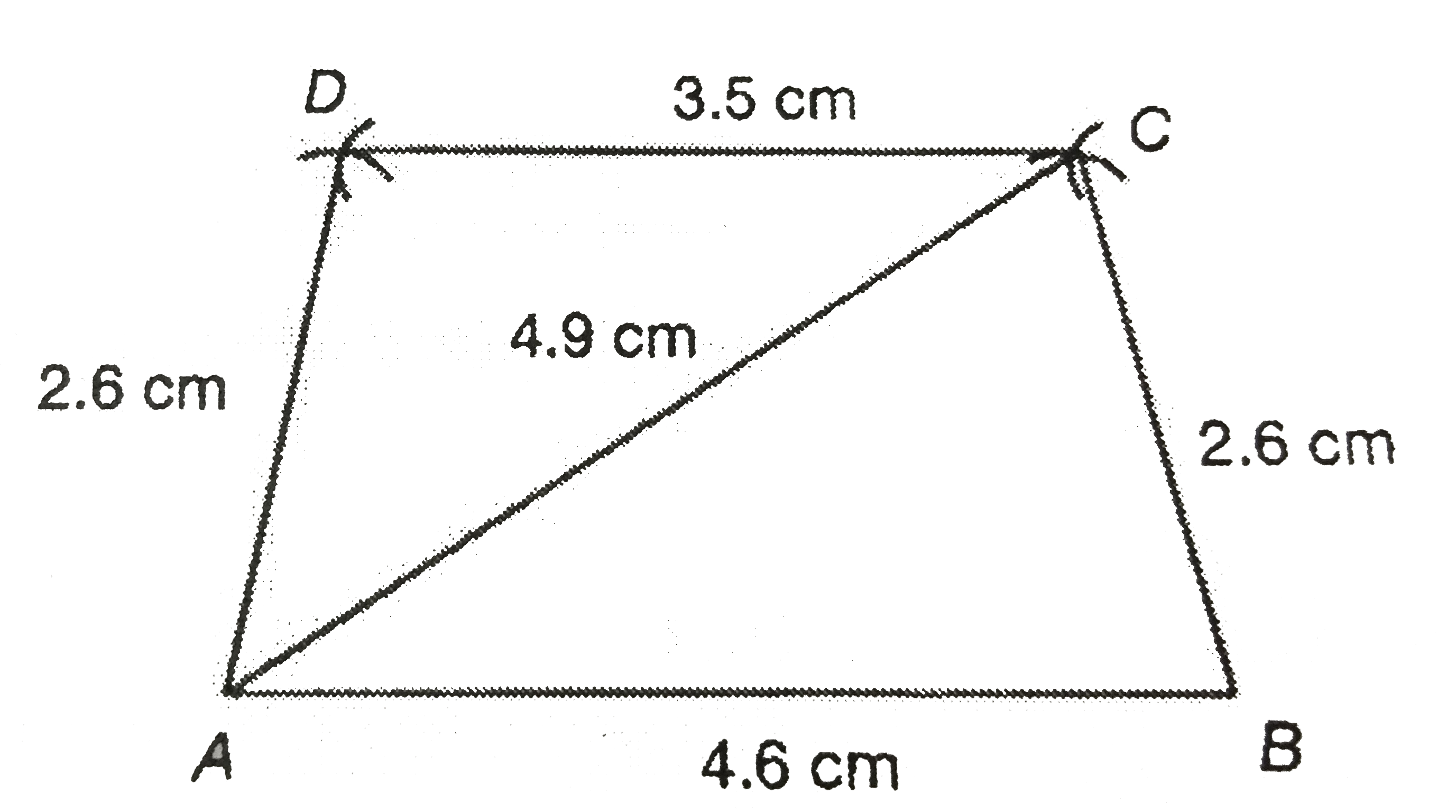
Construct a quadrilateral $ABCD$ in which $AB=4.6cm$, $BC=2.6cm$, $CD=3.5cm$, $AD=2.6cm$ and the diagonal $AC=4.9cm$.

Step 1: Draw a line segment AB=4.6 cm.
Step 2: With A and B as the centers,draw two arcs of radii 4.9 cm and 2.6 cm respectively to intersect each other at C.
Step 3: With C and A as the centers, draw two arcs of radii 3.5 cm and 2.6 cm respectively to intersect at D.
Step 4: Join BC, CD and AD to form quadrilateral ABCD.
ABCD is the required quadrilateral.

Baccho, humne abhi seekha ki hum kaise ek quadrilateral construct kr skte hai jab hume uski four sides aur diagonal ki measurements given ho yaani ki hume in total five measurements given thi. But can you construct a parallelogram when only three of it's measurements are given? Look at the following question:
Can you draw a parallelogram BATS where BA = 5 cm, AT = 6 cm and AS = 6.5 cm? Why?
Baccho, hum jaante hai ki parallelogram ($\mid {\mid }^{\text{gm}}$) ki opposite sides equal hoti hai, to agar hume ek parallelogram ki do adjacent sides given ho, to hum baaki dono sides bhi pta laga skte hai.
$\therefore$ In the given question, since $BA=5cm,\text{and}AT=6cm$,
$\Rightarrow$ $TS=BA=5cm\text{and}BS=AT=6cm$
Now we have all the five measurements and we can easily construct this parallelogram. Try it out!
Baccho, ye same concept rectangles and squares m bhi apply ho skta hai. If you are given two adjacent sides of a rectangle and its diagonal, you can construct it. And if you are given one side and the diagonal of a square, you can easily construct it too kyunki square ki saari sides equal hoti hai.

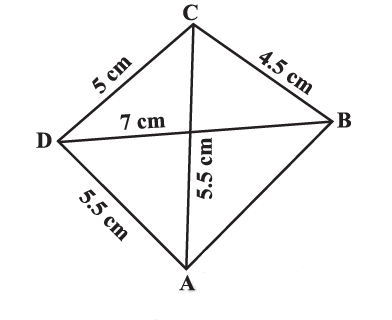
Bachcho ab hum seekhenge ki agar hume ek quadrilateral k do diagonals aur teen sides ki measurements given ho to hum use kaise construct krenge. So let's consider the following measurements:
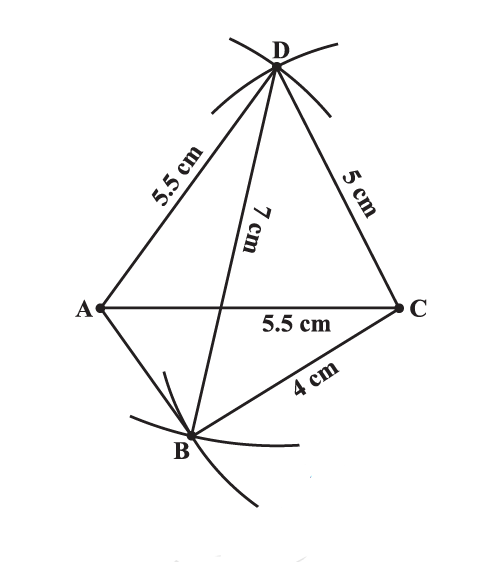
In quadrilateral $ABCD,BC=4.5cm,AD=5.5cm,CD=5cm$ the diagonal $AC=5.5cm$ and diagonal $BD=7cm$
Step 1 : Bachcho firse hum pahle ek rough sketch draw krenge with measurements for better visualization.

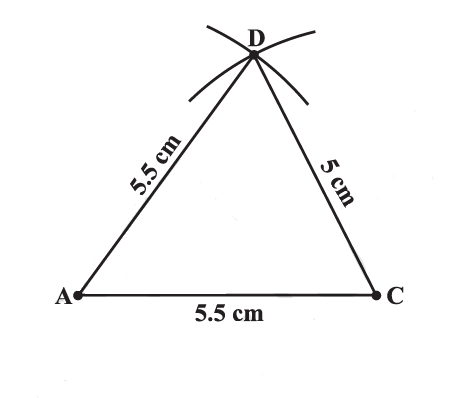
Step 2 : Ab hum $△ACD$ construct krenge using $SSS$ construction, jisme $AC=5.5cm,CD=5cm\text{and}AD=5.5cm$
Baccho, iss triangle ko construct krne k baad ab hume quadrilateral k fourth point $B$ locate krna hai.

Step 3 : To locate point $B$, hum $D$ ko centre lekr ek $7.7cm$ k radius ki arc draw krenge

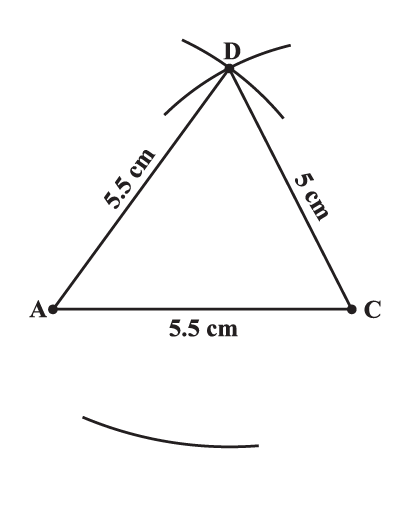
Step 4 : Baccho, kyunki hume third side $BC=4.5cm$ given hai, hum $C$ ko centre lekr ek $4.5cm$ k radius ki arc draw krenge which would cut our previous arc at $B$.

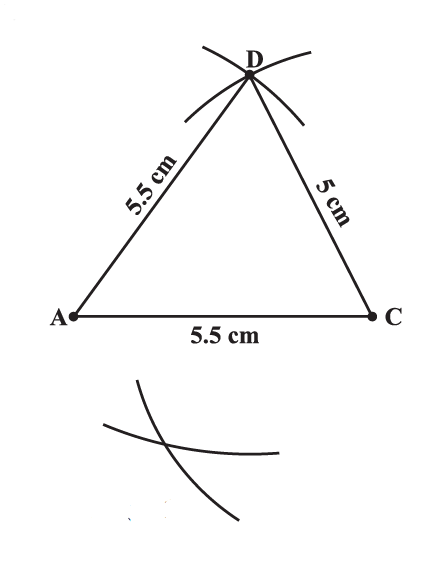
Step 5 : Join $AB\text{and}BC$, to complete the quadrilateral $ABCD$.

Construct Quadrilateral $ABCD$ , in which
$BC=4.5cm$
$AD=5.5cm$
$CD=5cm$
$AC=5.5cm$
$BD=7cm$

Refer to the video for the solution.

Bachcho ab hum seekhenge ki agar hume ek quadrilateral k do diagonals aur teen sides ki measurements given ho to hum use kaise construct krenge. So let's consider the following measurements:
In quadrilateral $ABCD,BC=4cm,AD=5.5cm,CD=5cm$ the diagonal $AC=5.5cm$ and diagonal $BD=7cm$
Step 1 : Bachcho firse hum pahle ek rough sketch draw krenge with measurements for better visualization.
Step 2 : Ab hum $△ACD$ construct krenge using $SSS$ construction, jisme $AC=5.5cm,CD=5cm\text{and}AD=5.5cm$
Baccho, iss triangle ko construct krne k baad ab hume quadrilateral k fourth point $B$ locate krna hai.

Step 3 : To locate point $B$, hum $D$ ko centre lekr ek $7cm$ k radius ki arc draw krenge

Step 4 : Baccho, kyunki hume third side $BC=4cm$ given hai, hum $C$ ko centre lekr ek $4cm$ k radius ki arc draw krenge which would cut our previous arc at $B$.

Step 5 : Join $AB\text{and}BC$, to complete the quadrilateral $ABCD$.

Construct a quadrilateral $ABCD$ , where $AB=3.5cm,BC=6.5cm,\angle A={75}^0,\angle B={105}^0\text{and}\angle C={120}^0$

Refer to the video for the solution.

Bachcho ab hum seekhenge ki agar hume three sides aur two included angles given ho to hum ek quadrilateral kaise construct krskte hai. Let;s consider the following question :
Construct a quadrilateral $ABCD$, where $AB=4cm,BC=5cm,CD=6.5cm\text{and}\angle DB=105°\text{and}\angle DC=80°.$
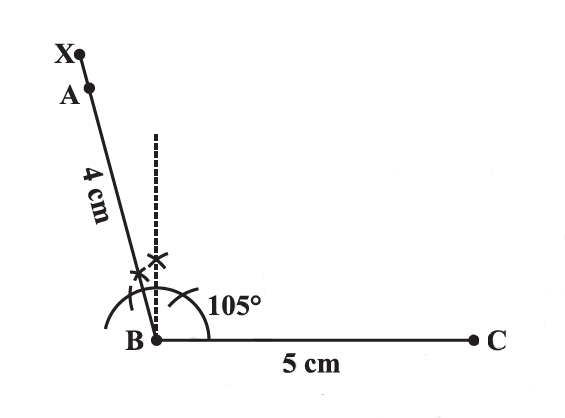
Step 1 : Hum sabse phle ek rough figure bnaaenge and saari measurements clearly mark krlenge.

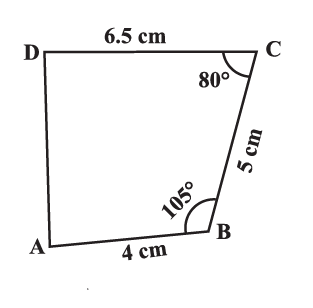
Step 2 : Baccho, we'll draw a line segment $BC$ of length $5cm$. Uske baad we'll construct an angle $\angle CBX=105°$ On ray $BX$, mark $A$ at a distance of $4cm$ away from $B$. Now, we have three corners of the quadrilateral $(A,B,C)$.

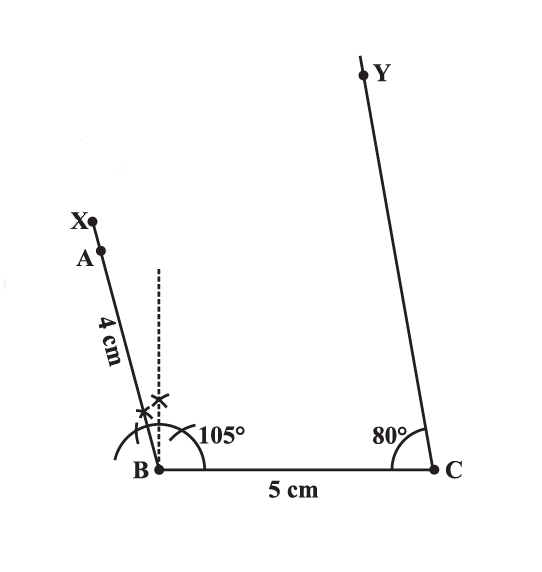
Step 3 : Fourth point $D$ ko locate krne k lie, hum point $C$ par $\angle BCY=80°$ construct krenge.

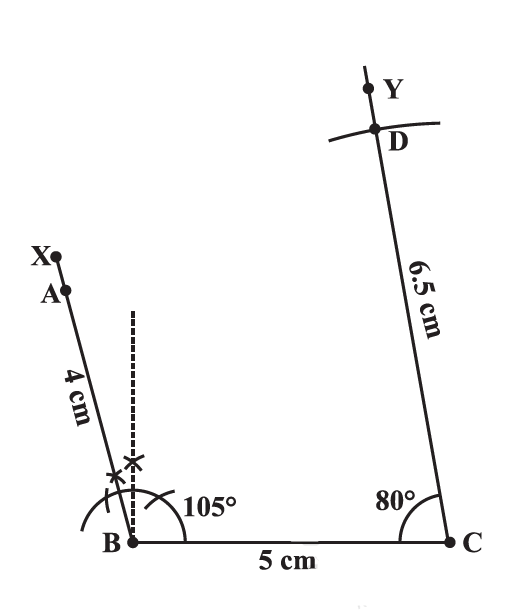
Step 4 : Now, on ray $CY$, we'll mark $D$ at a distance of $6.5cm$ away from $C$.

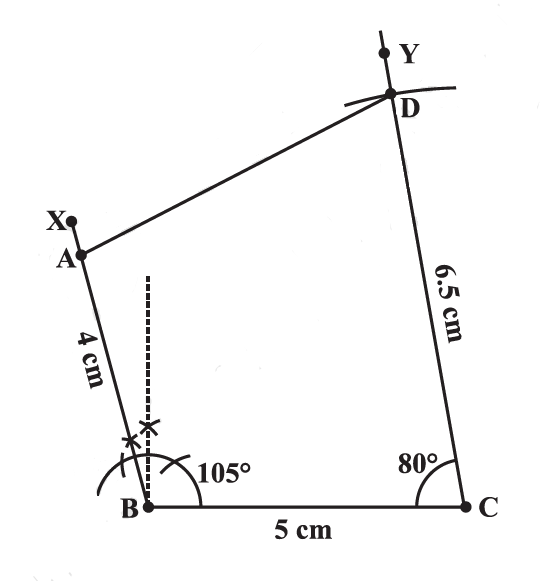
Step 5 : Join $A$ and $D$ to get the fourth side of the quadrilateral $ABCD$.

Construct a quadrilateral $PQRS$, in which $\angle Q={45}^0,\angle R={90}^0,QR=5cm,PQ=9cm\text{and}RS=7cm$

Refer to the video for the solution.

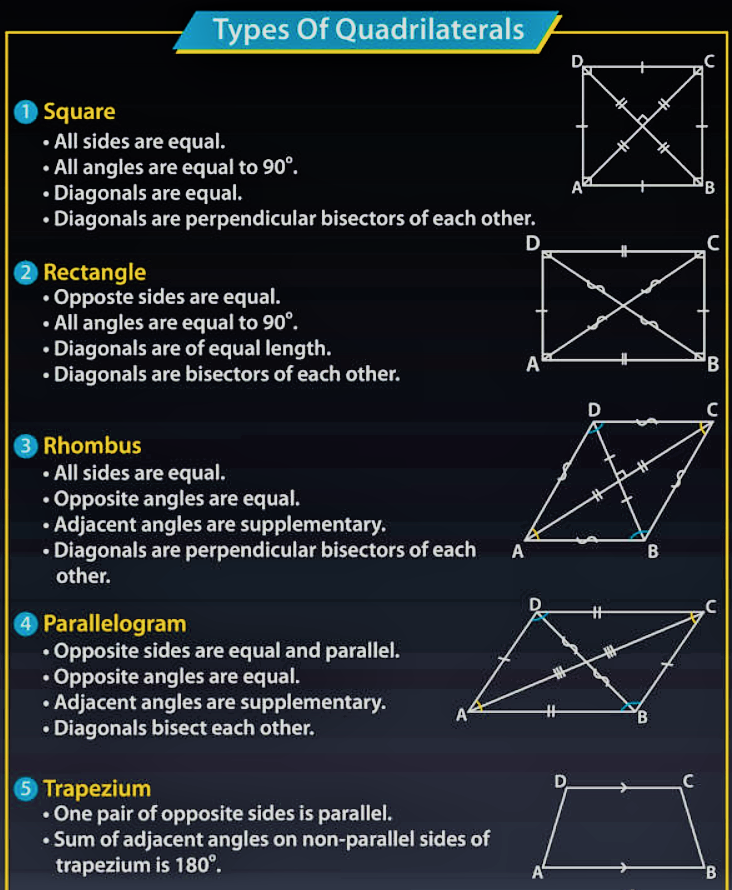
Baccho now we'll consider some special cases of constructing quadrilaterals. Before starting let us revise the different types of quadrilaterals.

Baccho, abhi tk humne dekha ki we were given various measurements of sides and angles of the quadrilateral. But what if we're given only a few measurements of a specific quadrilateral? Let's consider the following examples :
$1)$ Draw a square $ABCD$ of side 4.5 cm.

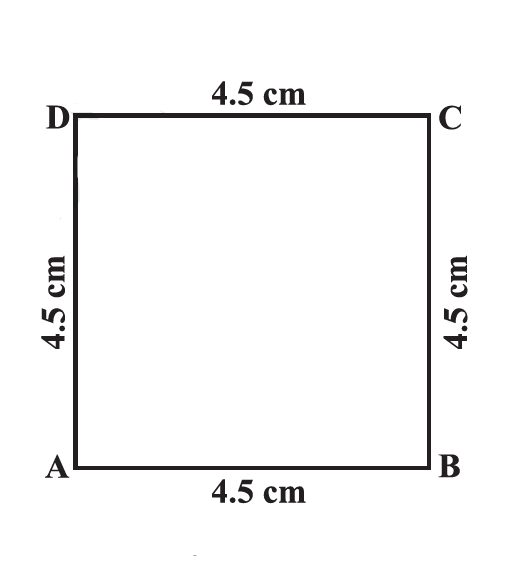
Baccho, as you can see, we're given only one measurement but also, we are given that the quadrilateral is a square and we know that a square has all its sides equal and all its angles equal to 90°. So let's see how to construct it!
Step 1 : Draw a line segment $AB$ of length $4.5cm$.
Step 2 : At $B$, construct an angle equal to 90°. On the other arm of the angle, mark $C$ at a distance of $4.5cm$ away from $B$. Baccho, jab aap $A\text{and}C$ ko join krenge, you'll see that you have got a $△ABC$ using $SAS$ rule.
Step 3 : Baccho ab aap easily point $D$ locate kr skte hai by drawing two arcs of radii $4.5cm$ from points $A\text{and}C$.
Step 4 : Join all the points and you'll have a square $ABCD$.
$2)$ Construct a rhombus $ABCD$ where $AC=6cm\text{and}BD=7cm$

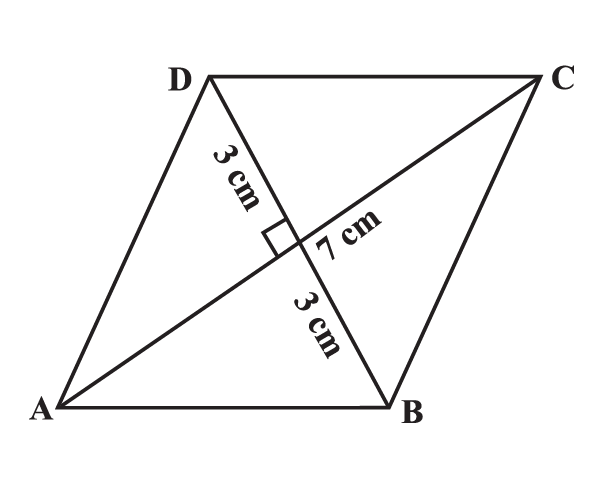
Baccho, iss case m bhi hume sirf rhombus k dono diagonals given hai. We are not given any measurements of sides or its angles, so how will we construct it? We know that $ABCD$ is a rhombus and rhombus ki saari sides equal hoti hai and the diagonals of a rhombus are perpendicular bisectors of each other!. So let's construct this :
Step 1 : Draw a line segment $BD=7cm$.
Step 2 : Baccho, ab hum $BD$ ka perpendicular bisector construct krenge.
Step 3 : To get our other diagonal, we'll cut the perpendicular bisector with an arc of radius $3cm\left(\frac{6}{2}\right)$ on either sides of $BD$ and we'll mark both the points as $A$ and $C$. Isse hume humara dusra diagonal $AC$ miljaaega.
Step 4 : Join the end points of both the diagonals $(A,B,C\text{and}D)$ to get rhombus $ABCD$.

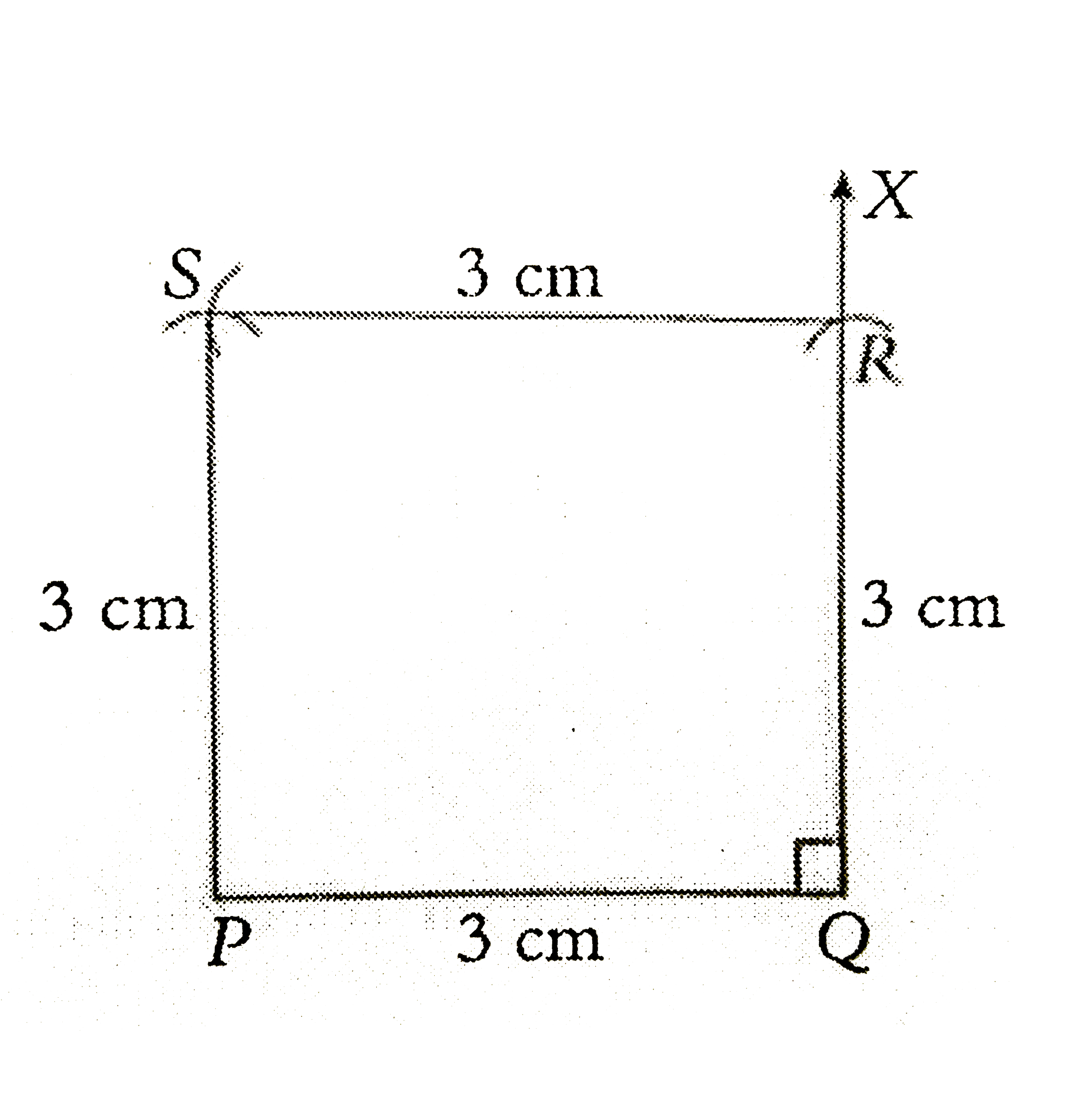
Construct a square of side $3cm$.

Steps:
1. Draw a line segment $PQ=3cm$.
2. Construct $\angle PQX={90}^{\circ }$.
3. Mark the point $R$ on $\overrightarrow{QX}$ such that $QR=3cm$.
4.With $R$ and $P$ as centres and with radii of $3cm$ each , draw two arcs to intersect each other at $S$.
5. Join $PS$ and $RS$ to form the required square $PQRS$.

• Five measurements can determine a quadrilateral uniquely.


• A quadrilateral can be constructed uniquely if the lengths of its four sides and a diagonal is given.


• A quadrilateral can be constructed uniquely if its two diagonals and three sides are known.


• A quadrilateral can be constructed uniquely if its two adjacent sides and three angles are known.


• A quadrilateral can be constructed uniquely if its three sides and two included angles are given.

$1$) Baccho iss chapter m humne seekha ki ek unique quadrilateral ko bnane k lie five measurements ki zaroorat pdhti hai. We learnt how to construct different quadrilaterals jisme kahi p sides aur kahi p angles k measurements given hote hai using only compass and ruler.
$2$) Humne seekha ki agar kisi quadrilateral ki four sides aur ek diagonal ka measurement given ho to hum kaise use construct kr skte hai by constructing a triangle first using $SSS$ construction condition.
$3$) We also learnt the construction of a quadrilateral jiski hume sirf teen sides aur do diagonals ki measurements given ho.
$4$) Then we learnt about the construction of a quadrilateral where we are given its two adjacent sides and three angles.
$5$) Fir humne seekha ki agar ek quadrilateral ki sirf teen sides aur do included angles given ho to use kaise construct kia jaata hai.
$6$) Finally, we revised our types of quadrilaterals and learnt special cases where only a few of the details are given.